
Preparing for a formal assessment can often feel like a daunting task, but understanding the core principles of problem-solving can significantly boost your confidence. When it comes to evaluating your ability to handle quantitative challenges, focusing on a few key strategies will make the process more manageable.
Solving problems efficiently requires not only practice but also a clear understanding of fundamental techniques. This section will guide you through various exercises that focus on enhancing your skills, ensuring that you’re ready to approach any challenge calmly and competently.
Understanding the right methods is essential for success. With the right strategies in place, you can confidently tackle even the most difficult questions. Our comprehensive approach will help you understand where to focus your energy and how to avoid common pitfalls that often lead to confusion.
Understanding numerical assessments is essential for anyone looking to improve their problem-solving capabilities. These evaluations focus on measuring your proficiency in various areas, including calculations, logical reasoning, and applying formulas to solve practical challenges. The goal is to develop the skills necessary for success in a variety of situations where quantitative reasoning is needed.
Key Areas of Focus
In most evaluations, the primary focus lies on several fundamental topics. These include working with fractions, decimals, percentages, and solving equations. Recognizing the patterns within these subjects will help you navigate questions effectively. Consistency and practice are crucial for mastering these areas and building confidence.
Problem-Solving Techniques
Approaching problems strategically is vital. Breaking down complex questions into simpler parts and applying systematic methods will often lead to more accurate results. The ability to stay organized and focus on the most relevant details ensures that even tricky problems can be solved efficiently.
Key Topics in Basic Math Assessments
When preparing for a quantitative evaluation, there are several important areas to focus on. Mastery of these fundamental concepts will provide a solid foundation for tackling various challenges. Whether it’s handling numbers, understanding relationships, or applying formulas, each topic plays a crucial role in ensuring success.
Core Concepts to Master
It’s important to be familiar with key principles that frequently appear in assessments. These include operations involving integers, ratios, and proportions, as well as understanding variables and solving for unknowns. Developing a strong grasp of these topics ensures that you can approach problems with clarity and precision.
Common Types of Questions
There are several question types that consistently appear in tests, each requiring different problem-solving techniques. Below is a breakdown of some of the most common formats you will encounter:
| Question Type | Description |
|---|---|
| Simple Calculations | These questions test your ability to perform basic arithmetic operations accurately and quickly. |
| Word Problems | These problems require you to interpret and translate a real-world scenario into mathematical terms. |
| Algebraic Equations | These require solving for unknown variables using established algebraic rules and techniques. |
| Geometry | Geometry questions involve calculating areas, volumes, and other properties of shapes and figures. |
Strategies for Solving Math Problems
Approaching numerical challenges can often seem overwhelming, but with the right strategies, solving problems becomes more manageable. Developing a step-by-step method helps break down complex tasks into simpler, more solvable parts. By focusing on a structured approach, you can increase accuracy and efficiency in finding the correct solutions.
Step-by-Step Problem-Solving Techniques
One of the best ways to solve problems efficiently is to follow a structured process. Here are some steps you can follow:
- Understand the Problem: Carefully read the question to make sure you know what is being asked.
- Identify Key Information: Highlight the important values, variables, and relationships given in the problem.
- Choose the Right Approach: Determine the best mathematical method to apply, whether it’s arithmetic, algebra, or geometry.
- Work Through the Problem: Solve the problem step by step, keeping track of your calculations.
- Review Your Solution: Check your work to ensure there are no errors and that you’ve answered the question correctly.
Common Techniques for Different Types of Problems

Different types of challenges require different strategies. Here are some common techniques for various problem types:
- For Simple Calculations: Use mental math or paper to quickly calculate values. Double-check your work to avoid simple mistakes.
- For Word Problems: Translate the words into mathematical expressions and equations, then proceed with solving as usual.
- For Algebraic Problems: Isolate variables and apply algebraic rules to solve for unknowns.
- For Geometry Questions: Familiarize yourself with formulas for areas, perimeters, and volumes to apply the correct calculations.
Common Errors to Avoid During Exams
While solving quantitative problems under time pressure, it’s easy to make mistakes. However, recognizing the most common errors can help you avoid them and improve your performance. These mistakes often arise from carelessness, misunderstanding the question, or rushing through the process. Being mindful of these pitfalls will ensure more accurate results and greater success.
Careless Calculation Mistakes
One of the most frequent errors occurs when performing basic operations such as addition, subtraction, multiplication, or division. These small mistakes can lead to incorrect answers. To prevent this, always double-check your calculations, especially in more complex problems where several steps are involved.
Misinterpreting the Question
Many errors stem from not fully understanding what the problem is asking. Sometimes, key information is missed, or a different approach is needed than the one initially considered. Take a moment to carefully read and analyze the question before beginning the solution process to ensure you’re addressing it correctly.
Skipping Steps in the Process
In the rush to complete the task, it’s easy to skip crucial steps or over-simplify parts of the problem. Following each step methodically ensures that no critical detail is overlooked. Always work through the problem step by step, and don’t skip any stages of the solution process.
Overlooking Units and Conversions
For problems that involve measurements, neglecting to convert units or using incorrect units can lead to significant errors. Always ensure that the units in your answer match the ones requested in the problem, and remember to convert where necessary.
Mastering the Right Approach to Solutions
To successfully solve any quantitative problem, having a well-planned approach is essential. The right mindset and methods can make all the difference in delivering accurate solutions under time constraints. Developing a clear strategy for approaching problems ensures that you remain organized, focused, and confident throughout the process.
Breaking Down the Problem Step by Step
One of the most effective ways to approach any question is to break it down into smaller, more manageable parts. Start by identifying the knowns and the unknowns, then look for relationships between them. Applying logical reasoning in a step-by-step fashion ensures that you follow the most efficient path to the correct answer.
Using the Correct Tools and Techniques
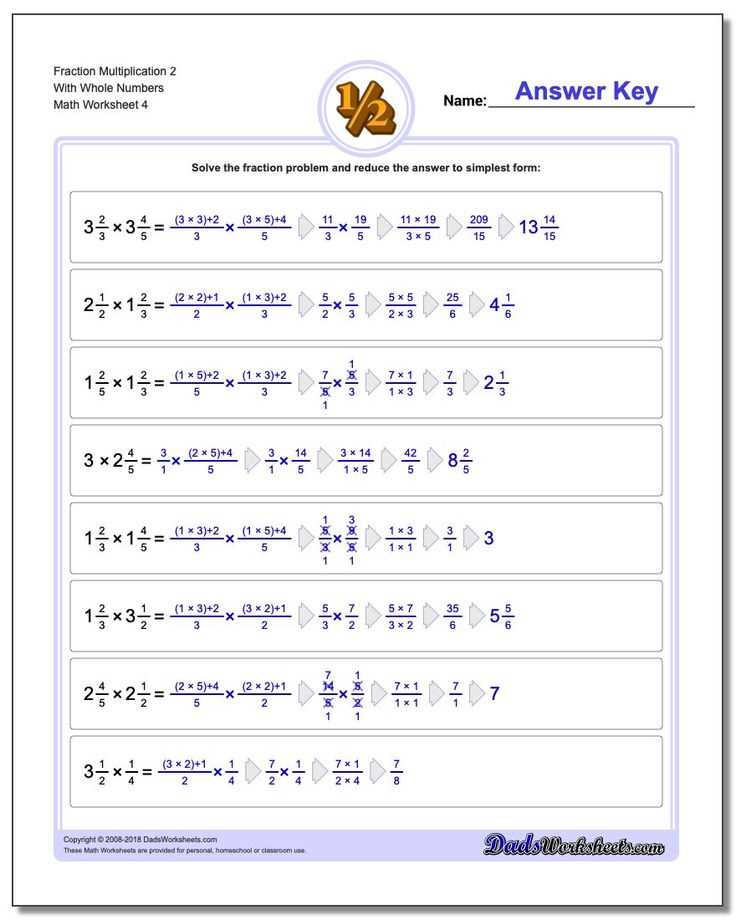
Having the right mathematical tools at your disposal is key. Whether it’s basic arithmetic, algebraic manipulation, or geometric principles, each problem requires a specific approach. Make sure you’re familiar with the techniques and formulas needed for solving different types of questions, and apply them where appropriate.
Effective Ways to Prepare for Exams
Preparation is key to performing well in any assessment. A well-organized study plan, coupled with the right techniques, can significantly improve your ability to tackle problems effectively. By focusing on the core concepts and practicing regularly, you can enhance your problem-solving skills and boost your confidence ahead of the test.
Creating a Study Schedule

To make the most of your preparation time, it’s important to develop a structured study schedule. Allocate specific time slots for each topic or skill, and stick to your plan. Consistent study habits will allow you to gradually master the material without feeling rushed as the exam approaches.
Practicing with Sample Problems
One of the best ways to prepare is by solving sample questions. Practicing various types of problems will help you become familiar with the format and identify areas that require further review. This hands-on approach reinforces learning and allows you to refine your skills over time.
Double-Checking Your Solutions for Precision
Ensuring the accuracy of your solutions is a critical part of the problem-solving process. Often, small mistakes go unnoticed during the first attempt, which can lead to incorrect results. Taking the time to review your work carefully can catch these errors and help you achieve the correct answer.
Key Areas to Focus on During Review
When reviewing your solutions, it’s essential to focus on the following areas:
- Recheck Calculations: Ensure that all arithmetic operations are performed correctly and no numbers are accidentally skipped or miscalculated.
- Verify Units: Double-check that all measurements are in the correct units and conversions have been applied accurately.
- Check Steps: Review each step of your work to ensure that the correct methods and formulas have been applied throughout the process.
Methods for Effective Verification
To verify your solutions effectively, consider using the following strategies:
- Work Backwards: Start from your final solution and retrace your steps to confirm the accuracy of each calculation.
- Use Estimation: Compare your solution to a rough estimate to see if the result is reasonable.
- Cross-Check with Another Method: If possible, solve the problem using an alternative method to see if both approaches yield the same result.