
In this section, we will explore essential concepts that play a crucial role in solving complex problems often encountered in AP assessments. By understanding the fundamental principles behind these exercises, students can approach challenges with confidence and clarity. Our focus will be on key mathematical ideas that are frequently tested, ensuring that you have a strong grasp of the necessary techniques for success.
As you work through the exercises, it’s important to break down each problem step-by-step, identifying patterns and applying relevant formulas. This method will not only help you find solutions more efficiently but also deepen your understanding of how these concepts interrelate. With dedicated practice, you can improve your ability to solve even the most challenging questions.
Understanding Random Variables in AP Statistics
In any mathematical context, certain quantities can be uncertain, fluctuating, or subject to change based on underlying conditions. Grasping how these uncertain quantities behave is a vital skill in tackling questions that require interpretation of numerical data. These concepts form the foundation for evaluating outcomes that depend on chance, allowing students to calculate expectations and probabilities effectively.
Exploring the Concept of Uncertain Quantities
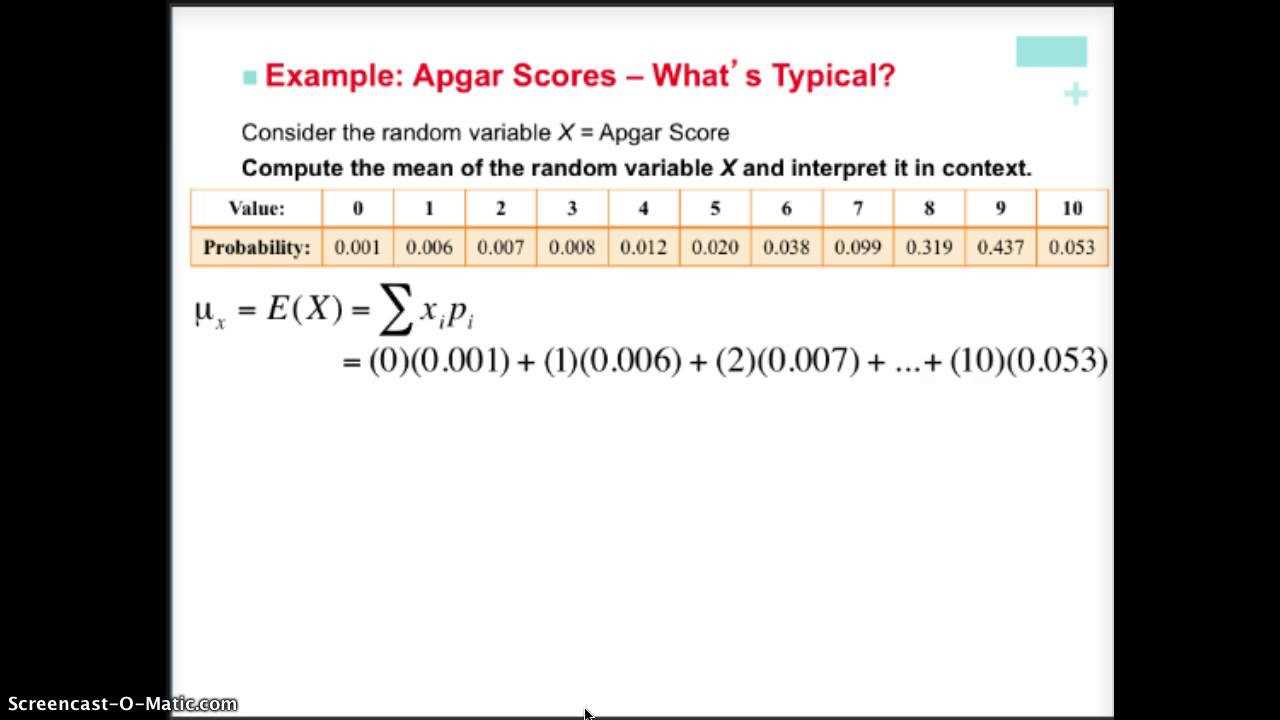
One of the first steps in approaching these exercises is to recognize that the values you’re working with are not fixed. Instead, they may change depending on different situations or experiments. Understanding this uncertainty is essential when predicting or calculating likely outcomes. The goal is to focus on how different scenarios could lead to varying results.
Application of Mathematical Models
Once the nature of the fluctuating quantities is understood, the next task is to apply appropriate mathematical models. These models allow you to analyze patterns in data and make sense of the relationships between different factors. By mastering these models, you can calculate the likelihood of certain results and predict future occurrences with greater accuracy.
Key Concepts for Chapter 6 Review

In this section, we will focus on the fundamental principles needed to approach questions in the sixth section of your coursework. Mastering these core ideas will give you the tools to solve problems effectively and confidently. A deep understanding of the core concepts allows for clearer problem-solving strategies and ensures you are well-prepared for any related challenges.
Understanding Distributions and Their Applications
The first important concept to grasp is how different types of distributions are used to model real-world scenarios. Whether you’re dealing with frequency distributions or probability distributions, it is essential to understand how data behaves and how to calculate outcomes based on this information. Recognizing the structure of a distribution helps in making informed predictions about potential results.
Calculating Expected Values and Probabilities
Another crucial element involves calculating expected values. These values give you an idea of the average outcome over numerous trials, providing insight into what can be expected in different situations. Alongside this, calculating probabilities is key for determining the likelihood of various outcomes, allowing you to assess risks and possibilities effectively.
Steps to Solve Random Variable Problems
Solving problems that involve uncertain quantities requires a systematic approach. By following a set of steps, you can break down complex scenarios and make informed decisions. This method not only helps in organizing your thoughts but also ensures that you don’t overlook key components of the problem.
Identify the Relevant Information
The first step is to identify and understand all relevant details provided in the problem. Pay attention to the given values, conditions, and the specific question being asked. Knowing what information you have and what you’re looking for will guide the entire process.
Apply the Right Mathematical Tools
Once you’ve identified the relevant information, the next step is applying the correct formulas and techniques. This could involve calculating averages, probabilities, or other measures that help in analyzing the given data. The right mathematical tools will allow you to work through the problem step by step and reach an accurate solution.
Common Mistakes in AP Statistics Tests

When working through challenging mathematical problems, it’s easy to overlook important details or make simple errors. These mistakes can significantly impact your results and understanding of the material. Recognizing and avoiding common pitfalls is essential for improving accuracy and ensuring you tackle problems with confidence.
Misinterpreting the Problem
A frequent mistake occurs when the problem is not fully understood. This could involve missing key information or misinterpreting the question’s requirements. It’s essential to read each question carefully, identifying the specific task at hand, whether it’s finding a value, comparing results, or interpreting data in a specific context.
Incorrect Application of Formulas
Another common error involves using the wrong formula or applying it incorrectly. Many students struggle with remembering the exact formula needed for different scenarios, leading to confusion and incorrect results. Reviewing and practicing the correct application of each formula can help ensure you use them properly when needed.
How to Approach Practice Test Questions
When faced with a set of problems designed to assess your understanding of key concepts, it’s important to develop an effective strategy. A clear approach helps you manage time and ensures you tackle each question with a systematic method. By breaking down each task into manageable steps, you increase your chances of finding accurate solutions.
The first step is to carefully read each question, highlighting key information and noting what is being asked. This helps you focus on the important aspects and avoid rushing into a solution. Once the question is clear, identify the most suitable method to solve it. If needed, start with simpler calculations before working your way to more complex ones, ensuring that your approach is logical and organized.
Effective Study Tips for AP Statistics
To excel in this subject, having a well-structured study routine is essential. It’s not just about reading the material but also about applying what you learn through various methods. Here are some practical strategies to improve your understanding and performance:
- Understand the Concepts: Don’t just memorize formulas–focus on the reasoning behind them. Knowing why a formula works will help you apply it more effectively in different situations.
- Practice Regularly: Consistent practice helps reinforce the concepts and allows you to become more comfortable with solving complex problems. The more problems you tackle, the easier they will become.
- Review Mistakes: Take the time to go over any errors you make during practice. Understanding why you made a mistake will help you avoid it in the future and deepen your comprehension of the topic.
In addition to these techniques, consider forming a study group with classmates or seeking help from a tutor if necessary. Discussing challenging topics with others can provide new insights and reinforce your knowledge. Lastly, don’t forget to take breaks to avoid burnout and maintain a steady pace in your studies.