As you approach the culmination of your studies, it’s important to have a solid understanding of the material you’ve encountered throughout the course. This section will help you consolidate your knowledge and sharpen your skills for the upcoming assessment. By focusing on key concepts and problem-solving techniques, you’ll be better equipped to tackle any challenges that arise.
Within this guide, you’ll find various strategies for mastering critical topics, from equations and functions to more complex mathematical structures. It’s essential to practice applying these methods in different scenarios to ensure a thorough grasp of the content.
With dedication and the right preparation, success is within reach. Stay focused, utilize the materials provided, and approach each problem with confidence. Your hard work will pay off as you move forward in your mathematical journey.
Algebra 2 Semester 1 Review Guide
In this section, we will focus on strengthening your understanding of the essential topics covered during the first part of the course. Whether you need to reinforce foundational concepts or dive deeper into more complex problems, this guide will provide you with the tools to succeed.
To effectively prepare, it is crucial to address key concepts and practice problem-solving techniques. Here are the major areas to focus on:
- Solving linear and quadratic equations
- Graphing functions and interpreting their behavior
- Working with polynomials and factoring techniques
- Understanding rational expressions and their properties
- Exploring systems of equations and solutions
- Analyzing inequalities and their graphical representations
For each of these areas, make sure you practice applying various methods and strategies. The more problems you work through, the more comfortable you will become with the material. Remember to focus on understanding the reasoning behind each solution, not just memorizing steps.
Additionally, reviewing past problems and identifying areas that need more attention will help you focus your efforts where they are most needed. Regular practice and revisiting challenging topics will ensure a solid foundation as you move forward.
Key Topics for Algebra 2 Exam
In this section, we will focus on the most important areas that require attention before the assessment. These are the core concepts that will play a significant role in the overall understanding and application of mathematical techniques. Mastering these topics will ensure that you are well-prepared for any questions that may arise.
Essential Concepts to Focus On
- Understanding and solving different types of equations
- Graphing and interpreting functions and their transformations
- Factoring expressions and simplifying polynomials
- Working with rational expressions and their manipulation
- Systems of equations and methods of solving them
- Analysis and solving of inequalities
Mathematical Problem-Solving Strategies

- Practice solving problems step-by-step to improve clarity
- Review different techniques for solving systems, including substitution and elimination
- Master graphing techniques and transformations
- Ensure a strong understanding of factoring and its applications
- Focus on recognizing patterns and applying appropriate methods to simplify complex expressions
By concentrating on these fundamental topics and applying various strategies, you will gain a deeper understanding of the material and be better equipped for the challenges ahead.
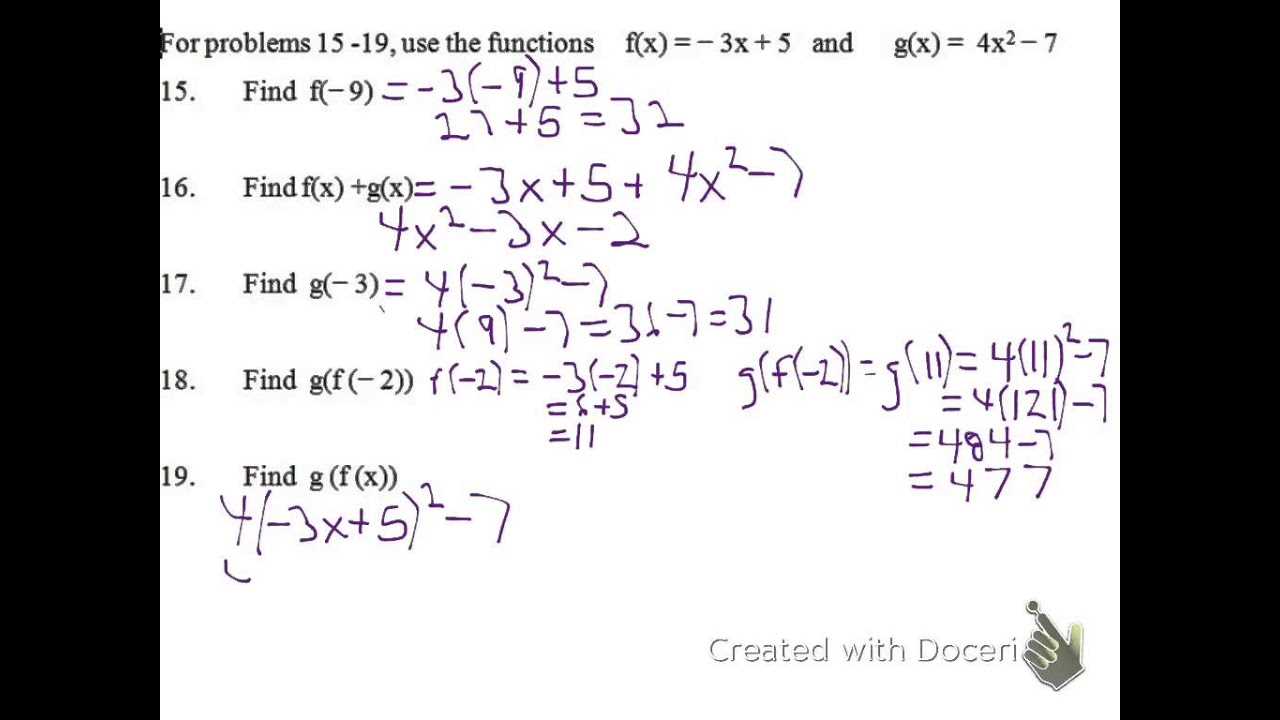
Solving Equations and Inequalities

Understanding how to solve both equations and inequalities is a fundamental skill in mathematics. Whether dealing with linear, quadratic, or rational expressions, the process of finding the solution involves identifying the unknown and isolating it using appropriate methods. Mastery of these techniques will provide you with the tools necessary to approach complex problems with confidence.
Solving Equations
When solving equations, it is important to follow a structured approach. Start by simplifying both sides of the equation if necessary. Then, apply inverse operations to isolate the variable. Here are some key strategies to remember:
- Simplify both sides of the equation
- Combine like terms where possible
- Use inverse operations to move terms to opposite sides
- Check your solution by substituting it back into the original equation
Solving Inequalities
Unlike equations, inequalities involve ranges of solutions rather than a single answer. When solving inequalities, it is essential to keep track of the direction of the inequality sign. Some important steps include:
- Isolate the variable by performing inverse operations
- Reverse the inequality sign when multiplying or dividing by a negative number
- Express the solution set in interval notation or graphically
By consistently applying these techniques and practicing different types of problems, you will become proficient in solving both equations and inequalities, allowing you to tackle more complex scenarios with ease.
Polynomials and Rational Expressions
Working with expressions that involve multiple terms, such as polynomials and rational forms, requires understanding how to manipulate and simplify them. These expressions are a crucial part of mathematics, appearing frequently in various problem-solving scenarios. Developing a strong grasp of these topics will allow you to handle a wide range of equations and inequalities with ease.
Polynomials are algebraic expressions made up of terms that are added or subtracted, where each term consists of a constant multiplied by a variable raised to a non-negative integer power. To work with them effectively, it is essential to learn how to combine like terms, factor expressions, and perform polynomial division.
Rational expressions are ratios of two polynomials. Simplifying and solving these expressions often involves factoring both the numerator and the denominator, finding common factors, and reducing the expression to its simplest form. Understanding how to handle such expressions is key to solving complex problems.
By practicing techniques such as factoring, simplifying, and performing operations on polynomials and rational expressions, you’ll improve your ability to solve advanced problems with confidence.
Understanding Functions and Graphs
Functions and their graphical representations are central to understanding relationships between variables in mathematics. By analyzing how one quantity changes in relation to another, you gain valuable insights into real-world applications and mathematical problems. Mastering the concept of functions is essential for solving a variety of equations and understanding their behavior visually.
A function is a relationship where each input is paired with exactly one output. The key to working with functions is understanding their notation, domain, and range. The graph of a function represents these relationships, providing a visual interpretation of how the output changes as the input varies. The shape of the graph gives important clues about the nature of the function itself.
Graphing different types of functions–linear, quadratic, cubic, and more–allows you to identify key characteristics such as intercepts, slopes, and points of symmetry. By practicing the interpretation and sketching of graphs, you can enhance your ability to solve problems involving different functions and their properties.
Through consistent practice and understanding of function behavior, you will become proficient in analyzing and solving function-based problems with confidence.
Systems of Equations and Matrices
Systems of equations and matrices are powerful tools for solving complex problems involving multiple variables. These mathematical structures allow you to represent and solve several equations simultaneously, making them essential for tackling real-world situations that require finding unknowns across various constraints. Understanding how to work with these systems will enhance your problem-solving abilities and prepare you for more advanced concepts.
A system of equations consists of two or more equations that share common variables. Solving these systems requires finding the values of the variables that satisfy all the equations at the same time. Different methods, such as substitution, elimination, and graphical solutions, can be used to find the solution.
Matrices provide a more efficient way of solving systems of equations, especially when dealing with larger sets of equations. A matrix is a rectangular arrangement of numbers that can represent a system of linear equations. Matrix operations, such as multiplication and row reduction, offer a systematic approach to solving these systems, often with the use of specialized algorithms.
Mastering both systems of equations and matrices will enable you to solve more complex mathematical problems and gain a deeper understanding of how variables relate to one another in multi-equation scenarios.
Tips for Successful Exam Preparation
Effective preparation for assessments requires a combination of organization, strategic practice, and a focused mindset. By adopting the right approach, you can enhance your ability to grasp challenging concepts and perform at your best during the test. Following a structured plan ensures that you cover all necessary material while managing stress and time efficiently.
Effective Study Habits
Building strong study habits is essential for success. Regular study sessions, instead of cramming, help reinforce understanding and retention. Make use of various techniques such as summarizing key points, practicing problems, and discussing complex topics with peers. Additionally, it’s important to stay organized by breaking down topics into manageable sections and setting specific goals for each study session.
Time Management Strategies
Time management plays a vital role in exam preparation. Prioritize the most challenging subjects or areas you struggle with and allocate more time to those. Creating a study schedule and sticking to it ensures a balanced review of all topics. Avoid multitasking and allow breaks to maintain focus and mental clarity.
| Time Management Tips | Benefits |
|---|---|
| Break study sessions into blocks | Improved focus and retention |
| Focus on weak areas first | Stronger overall understanding |
| Set realistic goals for each study period | Increased productivity |
By implementing these strategies, you will be better equipped to handle the material with confidence, ensuring optimal performance when it’s time to apply your knowledge.