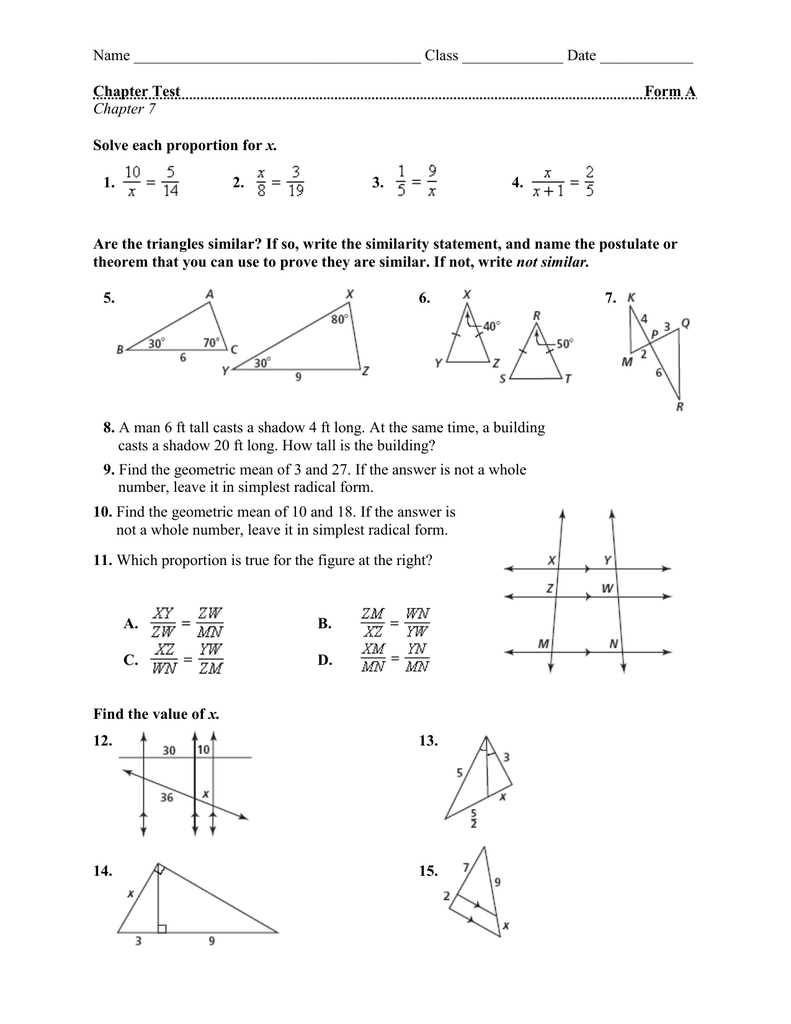
Mastering Essential Skills
In this section, we focus on the core principles that will help you effectively tackle problems related to shapes, angles, and measurements. By mastering these skills, you can confidently navigate through various mathematical situations that require logical reasoning and spatial understanding.
Breaking Down the Problems
To approach the problems efficiently, it’s essential to first understand the key concepts involved. Whether you are dealing with angles, lines, or areas, breaking each problem into smaller, manageable steps allows for clearer solutions. Analyzing each component before solving will help ensure accuracy.
Common Pitfalls to Avoid
Even experienced individuals make errors in understanding or interpreting a problem. It’s crucial to pay attention to common issues such as misidentifying angles, using incorrect formulas, or forgetting crucial steps. Recognizing these pitfalls can help you stay on track.
Effective Problem-Solving Techniques
- Understand the given data: Identify all the known variables and what needs to be solved.
- Apply appropriate formulas: Use the correct mathematical rules for each problem.
- Double-check calculations: Verify each step to ensure the accuracy of your solution.
Practical Tips for Success
When faced with these types of challenges, consistency and practice are key. Ensure that you are familiar with the foundational concepts and spend time solving different types of problems. Additionally, always keep track of your work and understand each step you take in the process.
Review and Reinforcement
Once you have solved the problems, review your work to see if there are alternative methods or quicker solutions. This helps reinforce the learning process and prepares you for similar problems in the future.
Understanding Key Mathematical Concepts
In this section, we will explore essential principles necessary for solving complex problems related to shapes, angles, and measurements. By gaining a solid grasp of these fundamental ideas, you’ll be able to tackle various challenges with confidence and clarity.
Having a clear understanding of the problem is crucial. Knowing what is being asked and recognizing the given data will guide your approach. Ensure you break the problem down into smaller, easier-to-handle parts, which will allow for a more straightforward solution process.
Step-by-Step Guide for Chapter 3 Assessment
The first step is to carefully review the information provided in the question. Identify key details, such as the type of shapes or relationships between the components. From there, apply the most appropriate mathematical techniques to determine the unknown values.
In the process, it’s helpful to visualize the problem with diagrams or sketches. This will help you better understand the spatial relationships and make it easier to see where different components fit together.
Common Errors in Problems
Even with a solid understanding of the concepts, mistakes can happen. A common error is misinterpreting the question or overlooking important details. Another frequent issue is applying the wrong formula or making simple arithmetic errors during calculations.
To avoid these pitfalls, always double-check your work and ensure that each step aligns with the instructions provided. Additionally, take time to verify the accuracy of your calculations before finalizing your solution.
Tips for Solving Challenges
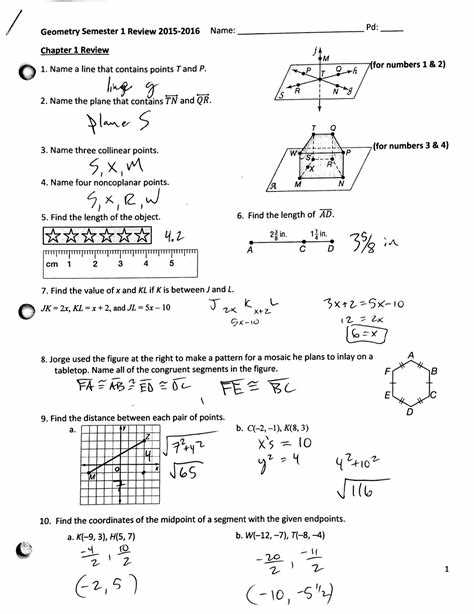
One useful technique is to work backward. Starting from the solution and retracing your steps can sometimes reveal where errors occurred or offer alternative ways to approach the problem. Another tip is to simplify complex problems by breaking them into smaller parts and solving them sequentially.
Also, practice regularly with different types of problems to become more familiar with various methods and strategies. The more problems you solve, the more confident you will become in your ability to handle increasingly difficult tasks.
How to Verify Your Solutions

After completing a problem, it’s important to review your solution to ensure that it makes sense. Double-check each step of the calculation and cross-reference your results with known formulas or rules. You can also test your solution by applying it in different scenarios to see if it remains consistent.
Reviewing Theorems and Postulates
Understanding the underlying principles behind mathematical rules is vital for successfully solving problems. Take time to revisit the relevant theorems and postulates, as these serve as the foundation for many solutions. Familiarity with these core ideas allows for a more efficient approach and reduces the likelihood of errors.
By consistently reviewing these concepts, you will develop a deeper understanding and be better prepared for challenges in the future.