
Understanding the properties of real numbers is essential for mastering the concepts of algebra and calculus. To help students practice and solidify their understanding of these properties, real numbers worksheets with answers in PDF format are an invaluable resource.
These worksheets provide a comprehensive review of the key properties of real numbers, including commutative, associative, and distributive properties, as well as the properties of zero and one. The worksheets are designed to challenge students at various levels, from basic understanding to more advanced application of the properties.
Each worksheet includes a variety of exercises and problems that cover different aspects of the properties of real numbers. These exercises provide students with opportunities to apply the properties in real-world contexts, such as solving equations and simplifying expressions.
The answers to the worksheets are included in the PDF file, allowing students to check their work and receive immediate feedback. This enables students to identify and correct any misconceptions or errors they may have made, enhancing their understanding of the properties of real numbers.
By providing students with a collection of real numbers worksheets with answers in PDF format, educators can support their learning and mastery of these fundamental algebraic concepts. These worksheets can be used as homework assignments, in-class practice, or as review material before exams, helping students build their skills and confidence in working with real numbers.
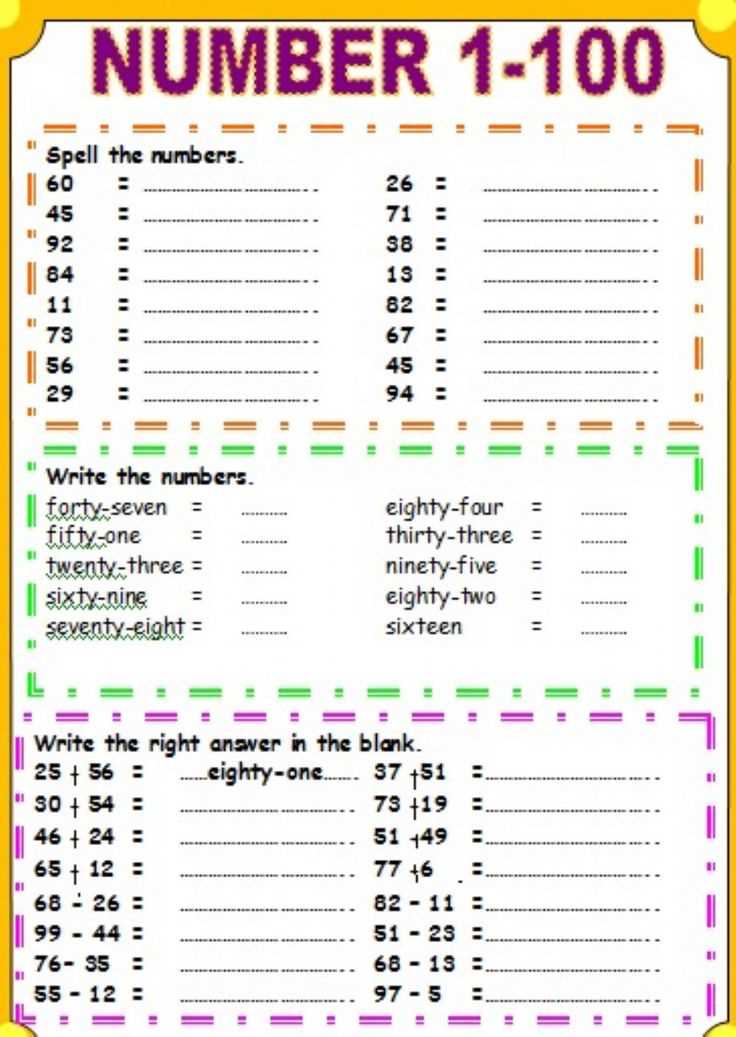
Properties of Real Numbers Worksheet with Answers PDF
If you are studying real numbers in mathematics, chances are you will come across worksheets that require you to practice and apply the properties of real numbers. These properties are fundamental rules that govern the behavior of real numbers, and understanding them is crucial for solving algebraic equations and word problems.
A great resource for practicing these properties is a worksheet with answers provided in a PDF format. This allows you to work through the problems on your own and then check your answers to ensure accuracy and understanding. Having the answers readily available also makes it easier to self-assess and identify areas where further practice might be needed.
Typically, a properties of real numbers worksheet will include a variety of problems that cover different aspects of the properties, such as the commutative, associative, and distributive properties. These problems may require you to simplify expressions, perform operations, or prove statements using the properties.
In addition to the problems, the worksheet will also include step-by-step solutions or explanations for each problem. This helps you understand the process and logic behind each solution, enabling you to apply the same concepts to similar problems in the future.
Overall, using a properties of real numbers worksheet with answers in PDF format is an effective way to practice and reinforce your understanding of these fundamental concepts. It provides an opportunity for independent learning and self-assessment, allowing you to improve your skills and confidence in working with real numbers.
Classifying Real Numbers
Understanding the classification of real numbers is an important concept in mathematics. Real numbers can be divided into several different categories based on their properties and characteristics. By classifying real numbers, we can better understand their relationships and use them in various mathematical operations.
Whole Numbers: Whole numbers are non-negative integers, including zero. They do not contain any fractions or decimals. Examples of whole numbers include 0, 1, 2, 3, and so on.
Natural Numbers: Natural numbers are positive integers greater than zero. They are also known as counting numbers because they are used to count objects. Examples of natural numbers include 1, 2, 3, 4, and so on.
Integers: Integers include both positive and negative whole numbers, including zero. They do not contain any fractions or decimals. Examples of integers include -3, -2, -1, 0, 1, 2, 3, and so on.
Rational Numbers: Rational numbers can be expressed as the ratio or fraction of two integers. They can be either terminating or recurring decimals. Examples of rational numbers include 1/2, 3/4, 0.5, 0.333…, and so on. Rational numbers also include integers and whole numbers.
Irrational Numbers: Irrational numbers cannot be expressed as the ratio or fraction of two integers. They are non-terminating and non-repeating decimals. Examples of irrational numbers include √2, π (pi), and e (Euler’s number). Irrational numbers are infinite and cannot be expressed as a fraction or ratio.
By understanding the classification of real numbers, we can solve various mathematical problems and equations, as well as explore the connections between different types of numbers. It is essential to have a strong foundation in classifying real numbers to excel in higher-level mathematics.
Operations with Real Numbers
In mathematics, real numbers are one of the fundamental concepts that are used extensively in various mathematical operations. Real numbers include both rational and irrational numbers, such as integers, fractions, decimals, and square roots. Understanding how to perform operations with real numbers is crucial in solving mathematical problems and equations.
Addition and Subtraction: The addition and subtraction of real numbers follow the same rules as with whole numbers. When adding or subtracting real numbers with the same sign, simply add or subtract the absolute values and keep the sign the same. For example, (-3) + (-5) = -8 and (-7) – (-2) = -5.
Multiplication and Division: To multiply or divide real numbers, multiply or divide the absolute values and apply the rules for the signs. Positive multiplied by positive gives a positive result, negative multiplied by negative gives a positive result, and positive multiplied by negative gives a negative result. For example, (-6) x 4 = -24 and (-12) ÷ (-3) = 4.
Order of Operations: Just like in other mathematical operations, the order of operations applies when performing calculations with real numbers. The order is as follows: parentheses, exponents, multiplication and division (from left to right), and addition and subtraction (from left to right). For example, in the expression 3 + 2 x 5, multiplication should be done before addition, resulting in the answer 13.
Properties of Real Numbers: Real numbers have various properties that help in performing calculations. These include the commutative property, associative property, distributive property, and identity properties. The commutative property states that the order of addition or multiplication does not affect the result. The associative property states that the grouping of numbers in addition or multiplication does not affect the result. The distributive property states that multiplication can be distributed over addition or subtraction. The identity properties state that adding or multiplying a number by 1 does not change its value. Understanding and applying these properties can simplify calculations with real numbers.
In conclusion, operations with real numbers involve addition, subtraction, multiplication, and division. Following the rules for these operations and understanding the properties of real numbers are essential for solving mathematical problems effectively. Practicing these operations and properties can greatly improve one’s mathematical skills.
Commutative Property of Addition
The commutative property of addition is one of the fundamental properties of real numbers. It states that the order in which two numbers are added does not affect the sum.
In other words, no matter how we rearrange the order of the numbers being added, the result will always be the same. This property can be stated as:
a + b = b + a
Where a and b are any real numbers. This property can be easily understood with simple arithmetic examples:
- If we consider the numbers 4 and 7, the commutative property of addition tells us that 4 + 7 is the same as 7 + 4, both of which result in 11.
- Similarly, if we consider the numbers -2 and 5, the commutative property of addition tells us that -2 + 5 is the same as 5 + (-2), both of which result in 3.
This property is particularly useful when simplifying expressions or solving equations involving addition. It allows us to freely rearrange the terms and still arrive at the correct solution.
Associative Property of Addition

The associative property of addition states that the way in which numbers are grouped when added together does not affect the sum. In other words, the order in which you group the numbers does not change the result of the addition.
For example, if we have three numbers, a, b, and c, the associative property of addition allows us to rearrange the grouping of these numbers. We can either add a and b first, and then add the sum to c, or we can add b and c first, and then add the result to a. The final sum will be the same in both cases.
The associative property can be written as:
- (a + b) + c = a + (b + c)
This property is a fundamental concept in mathematics and is used extensively in solving equations and simplifying expressions.
To illustrate the associative property, let’s consider the following example:
| Example: | Associative Property | Result |
|---|---|---|
| (2 + 3) + 4 | a + (b + c) | 9 |
| 2 + (3 + 4) | (a + b) + c | 9 |
As you can see, regardless of how the numbers are grouped, the sum remains the same.
The associative property of addition allows us to simplify and manipulate expressions by rearranging the grouping of numbers. It is an essential tool in algebra and helps in solving complex mathematical problems.
Commutative Property of Multiplication
The commutative property of multiplication is an essential property of real numbers that allows us to rearrange the factors in a multiplication expression without changing the result. This property states that the order of the factors does not matter in multiplication. In other words, if we multiply two real numbers, the result will be the same regardless of the order in which we multiply them.
For example, let’s consider the multiplication expression 3 × 5. According to the commutative property of multiplication, we can also express it as 5 × 3. Both expressions will result in the product of 15. This property holds true for any real numbers, not just for 3 and 5.
This property is particularly useful when solving equations or simplifying expressions. It allows us to rearrange the terms in an expression to make calculations easier or to find patterns. For example, if we have an equation like 2x = 10, we can use the commutative property of multiplication to rewrite it as x(2) = 10. This makes it easier to solve for x by dividing both sides of the equation by 2.
In summary, the commutative property of multiplication states that the order of the factors does not affect the result of multiplication. This property is a fundamental concept in mathematics and is used extensively in various applications.
Associative Property of Multiplication
The associative property of multiplication is an important property in mathematics that deals with the grouping of numbers during multiplication. This property states that the grouping of numbers in a multiplication does not change the product. In other words, changing the order in which numbers are grouped does not change the result of the multiplication.
For example, let’s consider the expression (2 × 3) × 4. According to the associative property of multiplication, we can rewrite this expression by changing the grouping as 2 × (3 × 4). No matter which way we group the numbers, the result will always be the same. In this case, both expressions evaluate to 24.
- (2 × 3) × 4 = 6 × 4 = 24
- 2 × (3 × 4) = 2 × 12 = 24
This property is particularly useful when working with larger numbers or when simplifying algebraic expressions. It allows us to rearrange the grouping of numbers to make calculations easier or to find equivalent expressions. The associative property of multiplication is a fundamental concept that helps us to understand the relationships between numbers and how they behave under different operations.
Distributive Property
The distributive property is a fundamental property of real numbers that allows us to simplify expressions and perform calculations more efficiently. It states that for any real numbers a, b, and c, the following equation holds true:
a * (b + c) = a * b + a * c
This means that when we distribute a value outside of a parentheses that contains addition or subtraction, we can multiply each term inside the parentheses separately and then add or subtract the results. This property is extremely useful in algebraic expressions and equations.
For example, let’s say we have the expression 2 * (3 + 4). By using the distributive property, we can rewrite it as:
- 2 * (3 + 4) = 2 * 3 + 2 * 4
- = 6 + 8
- = 14
So, using the distributive property, we simplified the expression 2 * (3 + 4) to the value 14. This property is particularly helpful when working with polynomials, as it allows us to expand and simplify expressions efficiently.
In summary, the distributive property is a powerful tool that allows us to distribute a value outside of parentheses over the terms inside, simplifying expressions and calculations in the process.