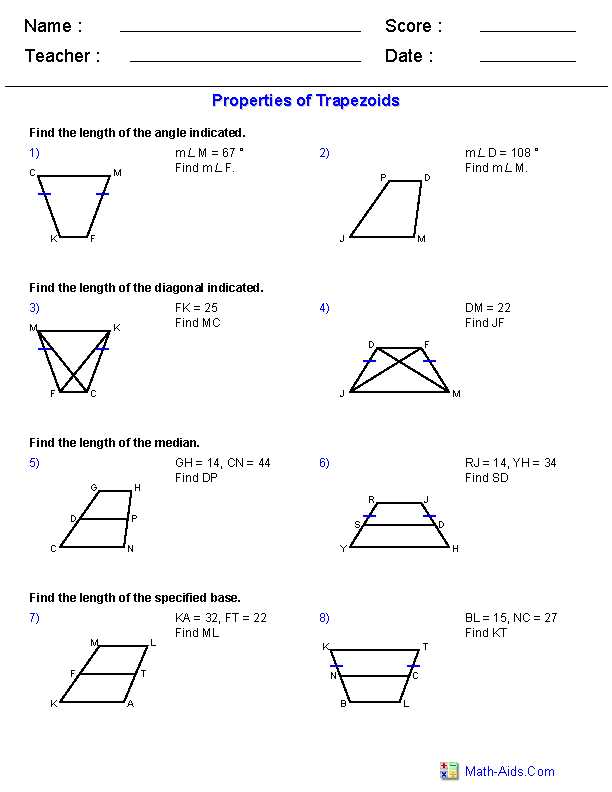
Rectangles are one of the fundamental shapes in geometry, and understanding their properties is crucial for solving problems involving area, perimeter, and other related concepts. A properties of rectangles worksheet is a valuable tool for students to practice their skills and reinforce their knowledge.
This worksheet typically includes a variety of questions that cover different aspects of rectangles, such as finding the length, width, area, and perimeter of a given rectangle. It also tests the understanding of various properties and relationships, including the diagonals, angles, and symmetry of rectangles.
By working through the answers to this worksheet, students can gain a deeper understanding of the geometry of rectangles. They can develop the ability to visualize and manipulate these shapes mentally, which is essential for solving more complex problems in mathematics and other fields.
Understanding Rectangles
Rectangles are one of the most common and basic shapes in geometry. They are four-sided polygons with opposite sides that are equal in length and four right angles. Understanding the properties of rectangles is essential for solving geometry problems and applying mathematical concepts in real-life situations.
Properties of Rectangles:
- Sides: A rectangle has four sides, with opposite sides being equal in length.
- Angles: All four angles in a rectangle are right angles, measuring 90 degrees.
- Diagonals: The diagonals of a rectangle are congruent and bisect each other.
- Area: The area of a rectangle is calculated by multiplying the length and width of the rectangle.
- Perimeter: The perimeter of a rectangle is calculated by adding the lengths of all four sides.
Rectangles have various applications in everyday life, such as in construction, architecture, and design. With their straight sides and right angles, they are often used to create stable structures and define boundaries. Architects and designers use rectangles to plan buildings, rooms, and furniture. Additionally, rectangles are used in measurements and calculations, such as determining the size and area of a wall or floor.
Understanding the properties of rectangles is not only important for mathematical purposes but also in practical scenarios. By knowing the characteristics of a rectangle, one can effectively utilize its properties to solve problems and make informed decisions related to shapes, sizes, and dimensions.
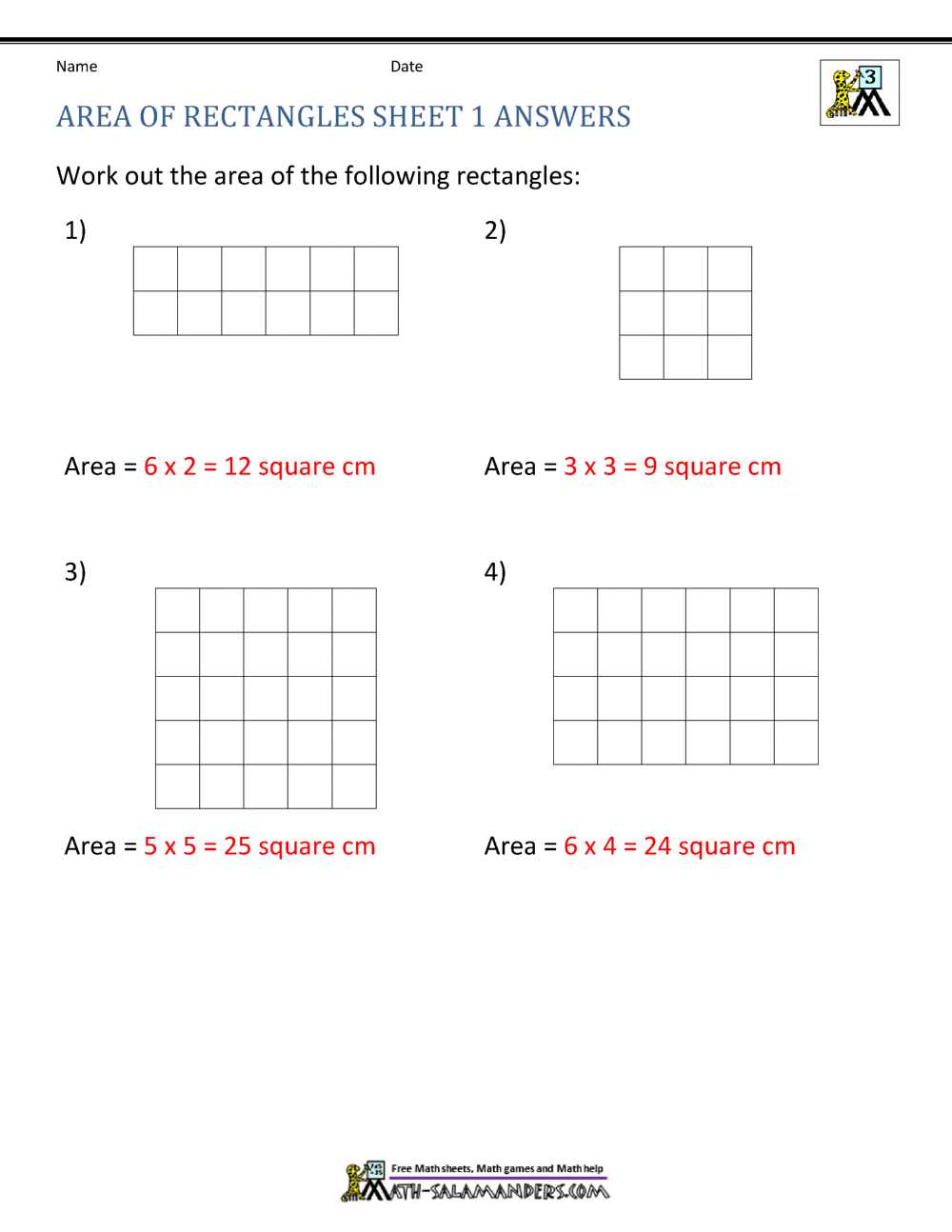
Calculating the Area of a Rectangle
The area of a rectangle is a measure of the space enclosed within its boundaries. It is found by multiplying the length and the width of the rectangle. The formula to calculate the area of a rectangle is:
Area = Length × Width
For example, let’s say we have a rectangle with a length of 8 units and a width of 4 units. To find the area, we would multiply the length by the width:
| Length | Width | Area |
|---|---|---|
| 8 units | 4 units | 32 square units |
So, the area of this rectangle is 32 square units.
Remember, the units used for length and width should be the same, and the resulting area will be in square units. The area of a rectangle is always a positive value, as it represents the amount of space enclosed within the shape.
Finding the Perimeter of a Rectangle
In geometry, a rectangle is a quadrilateral with four right angles. It is a special type of parallelogram, where the opposite sides are equal in length. One of the important properties of a rectangle is that its opposite sides are parallel.
To find the perimeter of a rectangle, you need to add up the lengths of all its sides. Since a rectangle has two pairs of equal sides, you can find the perimeter by using the formula: P = 2(l + w), where P represents the perimeter, l is the length, and w is the width of the rectangle.
For example, if the length of a rectangle is 5 units and the width is 3 units, you can find its perimeter by substituting these values into the formula: P = 2(5 + 3) = 2(8) = 16 units. Therefore, the perimeter of the rectangle is 16 units.
It is important to note that the perimeter of a rectangle is always greater than its area. This is because the perimeter is the total length of the outer boundary, while the area is the measure of the region enclosed by the rectangle.
In summary, the perimeter of a rectangle is found by adding the lengths of all its sides using the formula P = 2(l + w). It is always greater than the area of the rectangle. Understanding the concept of perimeter is crucial in various real-life applications, such as determining the amount of fencing needed for a rectangular garden or the length of borders in graphic design.
The Relationship Between Perimeter and Area
When studying rectangles, it is important to understand the relationship between perimeter and area. These two measurements are closely connected and can provide valuable information about the shape.
The perimeter of a rectangle is the sum of all its sides. It can be found by adding the lengths of the four sides together. The formula for finding the perimeter of a rectangle is: P = 2l + 2w, where P represents the perimeter, l represents the length, and w represents the width.
The area of a rectangle, on the other hand, is the measurement of the space inside the shape. It can be found by multiplying the length and width of the rectangle. The formula for finding the area of a rectangle is: A = l × w, where A represents the area, l represents the length, and w represents the width.
The relationship between perimeter and area can be observed by comparing the formulas. Since the perimeter involves adding the lengths of all sides, it is affected by the length and width of the rectangle. However, the area is determined by multiplying the length and width, resulting in a different effect. As a result, changing one measurement can have different effects on the two measurements.
For example, if the length of a rectangle is increased while the width remains the same, both the perimeter and area will increase. This is because the increased length adds to both the perimeter and the area. However, if the length remains the same but the width is increased, only the perimeter will be affected. The area will remain the same since it is determined by the product of length and width.
Understanding the relationship between perimeter and area is crucial when working with rectangles. It allows us to analyze and manipulate the shape to achieve desired results in various applications, such as construction, design, and geometry.
Diagonals of a Rectangle
A rectangle is a quadrilateral with four right angles. One of the important properties of a rectangle is that its diagonals are congruent. This means that the diagonals have the same length.
Let’s consider a rectangle ABCD. The two diagonals of this rectangle are AC and BD. Both diagonals intersect at the center of the rectangle, which is point O. The diagonal AC divides the rectangle into two congruent triangles, namely triangle AOC and triangle COD. Similarly, the diagonal BD divides the rectangle into two congruent triangles, triangle BOA and triangle BDC.
The length of the diagonal AC, which is denoted as dAC, can be found using the Pythagorean theorem:
dAC = √(AB2 + BC2)
Since a rectangle has opposite sides that are equal in length, we can rewrite this as:
dAC = √(AB2 + AB2) = √2AB2 = AB√2
Therefore, the length of the diagonal AC is equal to the length of one side of the rectangle multiplied by the square root of 2. Similarly, the length of the diagonal BD, denoted as dBD, is also equal to AB√2.
In summary, the diagonals of a rectangle are congruent and their lengths can be found using the formula AB√2, where AB is the length of one side of the rectangle.
Properties of Opposite Sides in a Rectangle
A rectangle is a quadrilateral with four right angles. One of the key properties of a rectangle is that its opposite sides are congruent. This means that if we have a rectangle ABCD, then sides AB and CD are equal in length, as well as sides BC and AD.
Let’s take a closer look at this property using a diagram:
| Rectangle ABCD | Opposite Sides |
 |
|
This property can be explained by the fact that a rectangle can be divided into two congruent right triangles. Each right triangle has a hypotenuse that is equal to the length of one of the rectangle’s sides, and two legs that are equal to the lengths of the rectangle’s adjacent sides. Therefore, we can conclude that opposite sides of a rectangle are congruent.
This property is useful in many applications, such as finding missing side lengths or solving problems involving areas and perimeters of rectangles. By knowing that opposite sides of a rectangle are congruent, we can make calculations and solve problems more easily.
Properties of Opposite Angles in a Rectangle
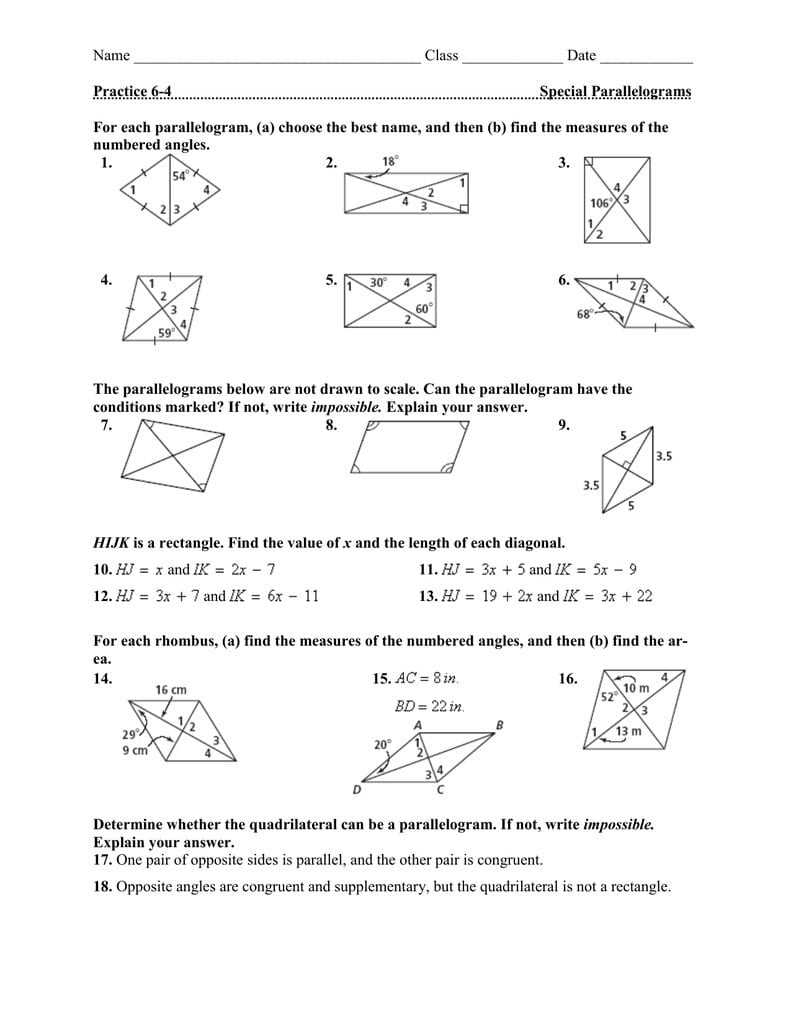
In a rectangle, opposite angles are congruent. This means that if we have a rectangle ABCD, then angle A and angle C are equal, and angle B and angle D are equal. The measure of angle A is the same as the measure of angle C, while the measure of angle B is the same as the measure of angle D.
This property can be proven using the fact that the opposite sides of a rectangle are parallel. Since opposite sides are parallel, the transversal formed by the diagonal of the rectangle creates a pair of corresponding angles. By the corresponding angle postulate, these corresponding angles are congruent, which implies that opposite angles in a rectangle are also congruent.
- Angle A = Angle C
- Angle B = Angle D
This property of opposite angles in a rectangle is useful when solving problems involving angles in rectangles. By knowing that opposite angles are congruent, we can find the measure of one angle using the measure of its opposite angle.
Applications of Rectangles in Real Life
The concept of rectangles is not limited to the confines of a geometry class; in fact, rectangles can be found in various aspects of our daily lives. From architecture to engineering to design, rectangles play a crucial role in different areas, showcasing their versatility and practicality.
One of the most obvious applications of rectangles is in the field of architecture. Buildings, houses, and structures are often designed and constructed using rectangular shapes. The four sides of a rectangle provide stability and symmetry, making it an ideal choice for creating strong and structurally sound edifices. Additionally, the rectangular shape allows for efficient use of space, maximizing the available area for different purposes within the building.
In engineering, rectangles are frequently utilized in the design of machines, tools, and equipment. Their uniform sides and right angles make them easy to work with and integrate into various mechanical systems. From conveyor belts to circuit boards, rectangles are often used as the basic building blocks of these complex systems, allowing for efficient manufacturing and assembly processes.
Rectangles also find their way into the world of design and aesthetics. From paintings to graphic design to product packaging, rectangles are commonly used to create visually appealing compositions. The straight lines and symmetrical nature of rectangles provide a sense of order and balance, often serving as a framework for other design elements. Whether it’s a book cover or a logo, rectangles are often used to convey professionalism, stability, and precision.
Overall, the applications of rectangles in real life are vast and varied. Their practicality, versatility, and aesthetic appeal make them an integral part of our daily lives, from the buildings we live in to the products we use. Understanding the properties of rectangles not only helps us in mathematical problem-solving but also enables us to appreciate and recognize their significance in the world around us.