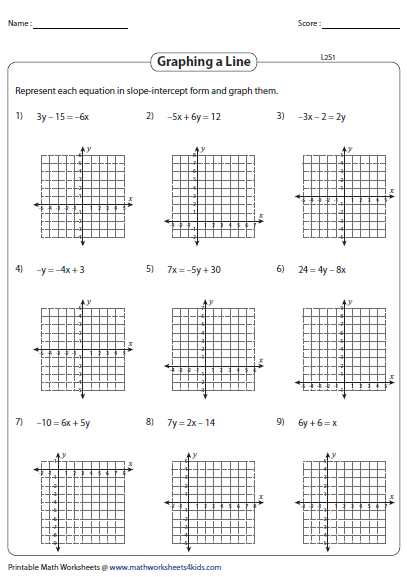
The Point Slope Form is an essential concept in algebra that helps us understand the relationship between two variables on a graph. In Course 3, students are introduced to the Point Slope Form and its applications. To reinforce the understanding of this topic, Kuta Software provides an answer key that enables students to check their work and practice solving various problems.
The Point Slope Form is expressed as y – y1 = m(x – x1), where m represents the slope of the line and (x1, y1) is a specific point on the line. By using this form, students can easily determine the equation of a line when provided with the slope and a point. Kuta Software’s answer key for Course 3 includes a step-by-step explanation of how to use the Point Slope Form to find the equation of a line, making it a valuable resource for students and teachers alike.
With the help of the Kuta Software answer key, students can practice solving problems that involve finding the equation of a line given the slope and a point, as well as problems that involve graphing lines using the Point Slope Form. This comprehensive answer key provides detailed solutions and explanations, allowing students to develop a deeper understanding of the concept and improve their problem-solving skills.
Point Slope Form Course 3 Answer Key Kuta Software
In Course 3, the concept of point-slope form is introduced as a method for representing linear equations in one variable. This form is particularly useful when working with equations that involve a known point on the line and the slope of the line. The answer key provided by Kuta Software helps students check their work and verify the correctness of their solutions.
The point-slope form of a linear equation is given by the equation y – y1 = m(x – x1), where (x1, y1) is a point on the line and m is the slope of the line. To solve problems using this form, students need to substitute the values of the point and slope into the equation and simplify the expression.
With the help of the Kuta Software answer key, students can compare their solutions to the correct answers provided. This allows them to identify any mistakes they may have made and make corrections accordingly. By going through the answer key, students can gain a better understanding of the concepts and techniques involved in solving problems using point-slope form.
Additionally, the answer key can be a helpful tool for teachers as well. It allows them to quickly assess their students’ understanding of the topic and provide targeted feedback. Teachers can use the answer key to identify common misconceptions or areas where students may be struggling, and adjust their instruction accordingly.
In conclusion, the point-slope form course 3 answer key provided by Kuta Software is a valuable resource for both students and teachers. It helps students check their work, verify the correctness of their solutions, and gain a deeper understanding of the topic. For teachers, the answer key serves as a valuable assessment tool and allows for targeted instruction. Together, these resources support effective learning and mastery of the point-slope form concept in Course 3.
What is Point Slope Form?
Point slope form is particularly useful when we know a point on the line and its slope, and we want to write an equation to represent that line. By plugging in the values of the point and slope into the equation, we can easily find the equation of the line in point slope form.
Example:
Let’s say we have a line that passes through the point (2, 4) and has a slope of 3. Using point slope form, the equation of the line would be y – 4 = 3(x – 2). This equation represents the given line on the coordinate plane.
Point slope form provides a clear and concise way to represent linear equations, making it easier to analyze and solve problems involving lines. It allows us to easily identify the slope and point on the line, which can be helpful in various applications such as finding the equation of a line, graphing lines, or solving word problems related to slope.
Understanding the Components of Point Slope Form
The point slope form allows us to easily determine the equation of a line if we know its slope and one point on the line. This form is particularly useful when working with real-world applications, as it allows us to easily represent and solve problems involving the relationship between two variables.
To better understand the components of point slope form, let’s break it down:
- y – y1: The difference between the y-coordinate of a point on the line and the y-coordinate of the given point (x1, y1).
- =: Represents the equality between the left side and the right side of the equation.
- m: The slope of the line, which determines how steep or shallow the line is.
- (x – x1): The difference between the x-coordinate of any point on the line and the x-coordinate of the given point (x1, y1).
By substituting the known values for (x1, y1) and m into the equation, we can find the equation of the line represented by point slope form. This equation can then be used to analyze and solve various problems involving the line.
Overall, understanding the components of point slope form is essential for working with linear equations and graphing straight lines. It allows us to easily represent and solve problems involving the relationship between variables, providing a valuable tool for mathematical analysis.
How to Write an Equation in Point Slope Form
To write an equation in point-slope form, you first need to know the slope of the line and the coordinates of a point on the line. The slope represents how steep the line is, while the point gives you a specific location on the line.
Once you have these values, you can plug them into the point-slope form equation. Subtract the y-coordinate of the point (y1) from both sides of the equation. Then, distribute the slope (m) to the x-coordinate of the point (x1) and subtract it from both sides of the equation.
Example:
- Slope: m = 2
- Point: (3, 5)
Plugging these values into the equation, we get:
| y – 5 | = | 2(x – 3) |
Simplifying the equation, we have:
| y – 5 | = | 2x – 6 |
Finally, we can rearrange the equation to get it in the standard point-slope form:
| y | = | 2x – 1 |
Now we have successfully written the equation in point-slope form!
Point-slope form is very helpful when you need to find the equation of a line given a point and the slope. It allows you to easily write the equation without having to calculate the y-intercept.
Step-by-Step Examples of Solving Equations using Point Slope Form
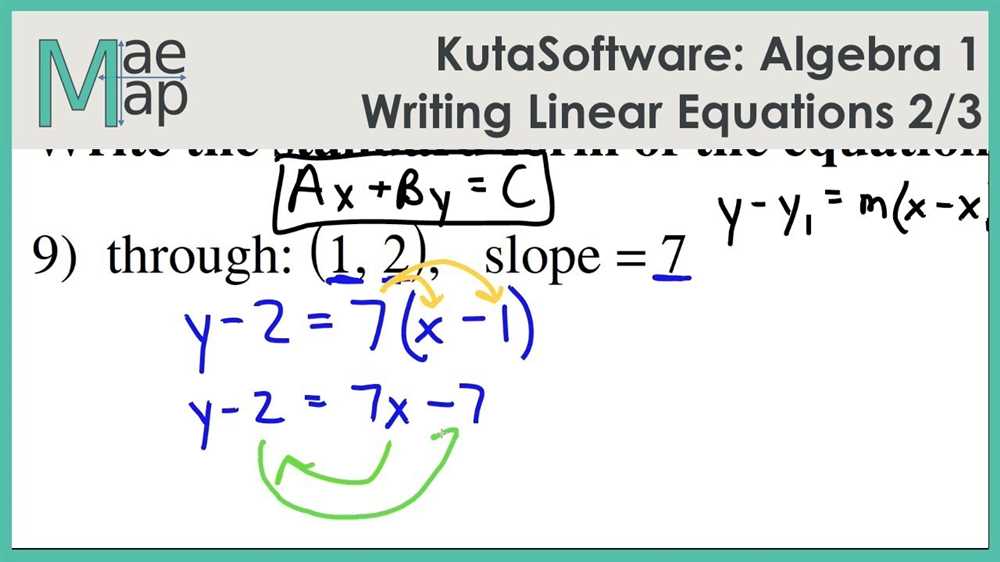
Here are some step-by-step examples of solving equations using point slope form:
- Example 1: Find the equation of a line that passes through the point (2, 4) and has a slope of 3.
- Start with the point slope form equation: y – y1 = m(x – x1).
- Substitute the values of x1, y1, and m into the equation: y – 4 = 3(x – 2).
- Distribute 3 to the terms inside the parentheses: y – 4 = 3x – 6.
- Add 4 to both sides of the equation to isolate y: y = 3x – 2. This is the equation of the line that passes through the point (2, 4) with a slope of 3.
- Example 2: Find the equation of a line that passes through the point (-1, 5) and is parallel to the line y = 2x + 3.
- Start with the given line equation: y = 2x + 3.
- Since the desired line is parallel to y = 2x + 3, it will have the same slope of 2.
- Use the point slope form equation and substitute the values of x1, y1, and m into the equation: y – 5 = 2(x + 1).
- Distribute 2 to the terms inside the parentheses: y – 5 = 2x + 2.
- Add 5 to both sides of the equation to isolate y: y = 2x + 7. This is the equation of the parallel line that passes through the point (-1, 5).
By following these step-by-step examples, you can easily solve equations using point slope form and find the equation of a line given a point and its slope.
Common Mistakes to Avoid when Using Point Slope Form
Point slope form is a commonly used equation format in algebra to represent a linear equation. While it is a straightforward concept, there are some common mistakes that students often make when using point slope form. By being aware of these mistakes, students can avoid errors and improve their understanding and accuracy.
1. Misunderstanding the meaning of the slope
One common mistake is misinterpreting the meaning of the slope in point slope form. The slope represents the rate of change of the dependent variable with respect to the independent variable. Students sometimes confuse the slope with the y-coordinate of a point, leading to incorrect calculations and solutions.
2. Using the wrong point coordinates

Another common mistake is using the wrong coordinates for the given point in the equation. Point slope form requires the coordinates of a single point on the line, usually denoted as (x₁, y₁). Students may mistakenly interchange the x-coordinate with the y-coordinate or use the wrong values altogether, resulting in incorrect equations and solutions.
3. Incorrectly isolating the y-variable
In point slope form, the equation is typically written as y – y₁ = m(x – x₁), where m is the slope and (x₁, y₁) is a point on the line. Students often make the mistake of isolating the x-variable instead of the y-variable when rearranging the equation. This can lead to confusion and incorrect solutions if not corrected.
4. Miscalculating the slope
Calculating the slope accurately is crucial in point slope form. The slope is determined by finding the ratio of the change in y-coordinates to the change in x-coordinates between two points on the line. Students may miscalculate the slope by taking the difference of the y-coordinates instead of the change, or by mistakenly switching the signs of the differences. These errors can result in incorrect equations and solutions.
5. Forgetting to simplify the equation
After setting up the equation in point slope form, it is important to simplify it further if possible. Students may forget to simplify the equation before using it to solve problems, leading to unnecessary complexity and potential errors in calculations. Simplifying the equation can make it easier to work with and ensure more accurate solutions.
By being aware of these common mistakes, students can improve their understanding and application of point slope form in solving linear equations. Regular practice and attention to detail are key to avoiding these errors and achieving success in algebra.
Using Point Slope Form to Find Equations from Graphs
When given a graph, we can use the point slope form to find the equation of a line. First, we need to identify a point on the line and the slope of the line. Once we have these values, we can substitute them into the point slope form equation. By simplifying and rearranging the equation, we can determine the equation of the line.
For example, let’s say we have a graph with a line passing through the point (2, 4) and have a slope of 3. Using the point slope form equation, we substitute these values into the equation: y – 4 = 3(x – 2). By simplifying and rearranging the equation, we get y = 3x – 2. Thus, the equation of the line is y = 3x – 2.
The point slope form is a useful tool in analyzing and solving problems involving linear equations. It allows us to easily find the equation of a line given a point and the slope. By understanding and applying this form, we can better understand the relationship between points and lines in the coordinate plane.
Applying Point Slope Form in Real-Life Scenarios
The point slope form, also known as the slope-intercept form, is a fundamental concept in algebra that is widely used in real-life scenarios. It allows us to describe the equation of a straight line in terms of its slope and a single point on the line. Understanding point slope form is essential for solving a variety of problems in fields such as physics, engineering, and economics.
One real-life application of point slope form is in calculating the growth rates of populations and industries. For example, a biologist studying a population of birds can use point slope form to determine the rate at which the population is increasing or decreasing over time. By measuring the population size at two different points in time and knowing the average growth rate, the biologist can create an equation in point slope form to predict future population sizes.
In the field of economics, point slope form can be used to analyze the relationship between two variables, such as price and quantity demanded. By plotting the data points on a graph and fitting a line through them using point slope form, economists can determine the slope of the line, which represents the rate of change in quantity demanded for a given change in price. This information is crucial for making informed business decisions and predicting consumer behavior.
Another practical application of point slope form is in engineering and construction. Engineers often need to design structures such as bridges or buildings that are supported by straight lines. By using point slope form, they can calculate the slopes and coordinates of these lines, ensuring that the structure is stable and structurally sound. Point slope form also allows engineers to make adjustments to the design in case there are changes in the slope or position of the lines.
In conclusion, point slope form is a powerful tool that has numerous real-life applications. Whether it is used to analyze population growth, study economic trends, or design structures in engineering, point slope form allows us to express the equation of a straight line in a concise and meaningful way. By understanding this concept, we can solve a wide range of problems and make informed decisions in various fields.
Finding the Answer Key for Point Slope Form Exercises using Kuta Software

Point slope form is a popular method for writing linear equations. It is often used in algebra courses to teach students how to graph linear equations or to find the equation of a line given a point and the slope. Students are usually given a set of exercises to practice this concept, and having access to the answer key can be very helpful in understanding and correcting mistakes.
One way to find the answer key for point slope form exercises is by using Kuta Software. Kuta Software is an online resource that provides various math worksheets and answer keys. To find the answer key for point slope form exercises on Kuta Software, you can follow these steps:
- Go to the Kuta Software website and navigate to the algebra section.
- Look for the specific topic of point slope form or linear equations.
- Select the worksheet that corresponds to the exercises you want the answer key for.
- Once you open the worksheet, you should be able to see the answer key along with the exercises. The answer key is usually located at the bottom of the worksheet.
By accessing the answer key on Kuta Software, students can easily check their work, identify areas where they need more practice, and gain a better understanding of the point slope form concept. It is important to note that while answer keys can be helpful, they should not be used as a substitute for learning and understanding the material. Answer keys should be used as a tool to verify and reinforce knowledge.