Complex numbers can seem intimidating at first, but with practice, they become easier to understand and work with. To simplify complex numbers, it’s important to have a solid grasp of basic arithmetic operations such as addition, subtraction, multiplication, and division. This worksheet provides an answer key to help you practice simplifying complex numbers and check your work.
The answer key in this worksheet will guide you through the process of simplifying complex numbers step by step. It will help you break down complex numbers into their real and imaginary parts and perform the necessary operations to simplify them. By using this answer key, you will be able to practice solving various problems involving complex numbers and build your confidence in working with them.
Simplifying complex numbers is an essential skill in many fields, including mathematics, engineering, and physics. It allows you to simplify complex calculations and express them in a more manageable form. By mastering the skill of simplifying complex numbers, you will be able to solve equations, graph complex functions, and analyze complex systems more effectively.
Whether you are a student studying complex numbers for the first time or a professional looking to brush up on your skills, this worksheet’s answer key will be a valuable resource for you. Use it to practice simplifying complex numbers, gain a deeper understanding of their properties, and enhance your problem-solving abilities in various mathematical contexts.
Simplifying Complex Numbers Worksheet Answer Key: A Comprehensive Guide
When it comes to simplifying complex numbers, having an answer key can be incredibly helpful. It allows students to check their work and verify that they have correctly simplified the given expressions. In this comprehensive guide, we will walk through some common types of problems and provide an answer key for each.
Simplifying Complex Numbers with Rationalizing Denominators
One common type of problem involves simplifying complex numbers with rationalizing denominators. This occurs when there is a radical in the denominator of the complex number. To simplify these expressions, we multiply both the numerator and denominator by the conjugate of the radical. The conjugate is obtained by changing the sign of the imaginary part of the number. Here’s an example:
- Simplify: (3 – 2i) / (3 + √2i)
To rationalize the denominator, we multiply both the numerator and denominator by the conjugate of √2i, which is √2i. We then simplify the expression:
- (3 – 2i) * (√2i) / (3 + √2i) * (√2i)
- (3√2i + 2i√2 – 2i^2) / (3^2 + (√2i)^2)
- (3√2i + 2i√2 + 2) / (9 + 2i^2)
- (3√2i + 2i√2 + 2) / (9 – 2)
- (3√2i + 2i√2 + 2) / 7
So, the simplified form of the expression is (3√2i + 2i√2 + 2) / 7.
Simplifying Complex Numbers with Imaginary Units

Another type of problem involves simplifying complex numbers with imaginary units. In these expressions, we have powers of i raised to different exponents. To simplify these expressions, we use the fact that i^2 = -1 and i^3 = -i. Here’s an example:
- Simplify: (2i)^3 – 2i^2 + i – 4
We can simplify this expression by applying the rules of exponents and the fact that i^2 = -1 and i^3 = -i:
- (2i)^3 – 2i^2 + i – 4 = 8i^3 – 2(-1) + i – 4
- 8(-i) – 2(-1) + i – 4 = -8i + 2 + i – 4
- -8i + 2 + i – 4 = -7i – 2
So, the simplified form of the expression is -7i – 2.
In this comprehensive guide, we have covered two common types of problems that involve simplifying complex numbers. By following the steps and using the provided answer key, students can check their work and ensure that they have correctly simplified the given expressions.
What are Complex Numbers?
Complex numbers are mathematical entities that consist of a real part and an imaginary part. They are expressed in the form a + bi, where a and b are real numbers, and i represents the imaginary unit (i.e., the square root of -1). Complex numbers are an extension of the real number system, allowing for the representation of quantities that cannot be expressed solely with real numbers.
In a complex number, the real part (a) represents the horizontal component, and the imaginary part (bi) represents the vertical component. The imaginary unit (i) is used to express numbers that do not exist in the real number system, such as the square root of -1.
Complex numbers have many applications in various fields of mathematics and science. They are particularly useful in electrical engineering, physics, and computer science. In electrical engineering, complex numbers are used to represent AC circuits, with the real part representing the resistive component and the imaginary part representing the reactive component. In physics, complex numbers are used in quantum mechanics to represent wave functions. In computer science, complex numbers are used in algorithms that involve complex calculations, such as signal processing and image compression.
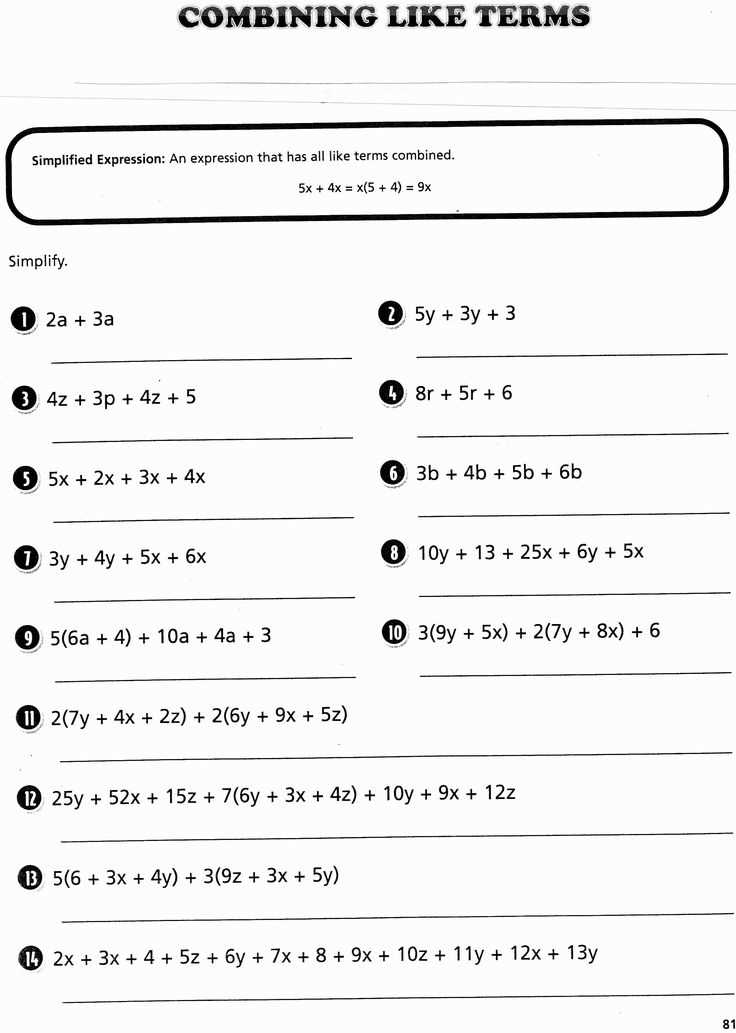
When simplifying complex numbers, it is important to combine like terms and separate the real and imaginary parts. This can be done by adding or subtracting the real parts and the imaginary parts separately. The simplification process involves performing operations such as addition, subtraction, multiplication, and division, following the rules of arithmetic for complex numbers.
Overall, complex numbers provide a powerful mathematical tool for solving problems and representing quantities that go beyond the realm of real numbers. They allow for the exploration of abstract concepts and have practical applications in various scientific and technological fields.
Simplifying Complex Numbers: Basic Rules and Concepts
Simplifying complex numbers is an important concept in mathematics. Complex numbers are numbers that consist of a real part and an imaginary part. They are often written in the form a + bi, where a represents the real part and bi represents the imaginary part.
When simplifying complex numbers, there are a few basic rules and concepts to keep in mind. One of the main rules is that the square root of -1 is represented by the imaginary unit i. This allows us to combine the real and imaginary parts of a complex number into a single expression.
To simplify a complex number, we can use the basic operations of addition, subtraction, multiplication, and division. When adding or subtracting complex numbers, we simply combine the real parts and the imaginary parts separately. For example, to simplify (3 + 2i) + (2 + 4i), we add 3 and 2 for the real part, and 2i and 4i for the imaginary part.
Multiplication and division of complex numbers can be done using the distributive property and basic algebraic techniques. For example, to simplify (2 + 3i)(4 + 5i), we multiply the real parts (2 * 4) and the imaginary parts (3i * 5i), and then combine them.
Overall, simplifying complex numbers involves applying these basic rules and concepts to combine the real and imaginary parts into a simplified expression. Practice and familiarity with these rules can help students become more comfortable with working with complex numbers and solving equations involving them.
Worksheet Exercises: Simplifying Complex Numbers
Complex numbers can be intimidating at first, but with practice and understanding, they can become much simpler to work with. This worksheet is designed to help students practice simplifying complex numbers. By simplifying complex numbers, students will be able to express them in a standard form: a + b*i, where “a” and “b” are real numbers and “i” is the imaginary unit.
The worksheet exercises include a variety of problems, ranging from basic simplification to more complex expressions. Students will be required to perform operations such as addition, subtraction, multiplication, and division on complex numbers. They will also need to combine like terms and simplify expressions with both real and imaginary parts. Additionally, some exercises may involve rationalizing the denominator or simplifying square roots.
Each exercise provides students with a complex number expression and asks them to simplify it. The answer key, available separately, provides step-by-step solutions to each problem, ensuring that students can check their work and learn from their mistakes. This way, students can practice simplifying complex numbers effectively and gain confidence in their abilities.
- Exercise 1: Simplify (-3 + 2i) – (5 – 4i)
- Exercise 2: Simplify (2 + 3i)(4 – 5i)
- Exercise 3: Simplify (3 + 2i)^2
- Exercise 4: Simplify (4 + 5i) / (2 – i)
- Exercise 5: Simplify sqrt(-6 + 8i)
By practicing these exercises, students will become more comfortable with simplifying complex numbers and gain a deeper understanding of their properties. They will also develop critical thinking skills, as simplifying complex numbers requires careful attention to detail and understanding of mathematical rules and operations. With continued practice, students will be able to confidently solve more complex problems involving complex numbers.
Step-by-Step Solutions: Worksheet Answer Key
Welcome to the step-by-step solutions for the Simplifying Complex Numbers worksheet. This answer key will provide you with a clear and concise explanation for each question on the worksheet. Whether you’re a student looking to check your work or a teacher wanting to guide your students through the process, this answer key is here to help.
Before we dive into the solutions, let’s review what we mean by simplifying complex numbers. A complex number is a number of the form a + bi, where a and b are real numbers and i is the imaginary unit. To simplify a complex number, we want to rewrite it in a simpler form by combining like terms.
Now, let’s go through the step-by-step solutions for each question on the worksheet:
Question 1:
Simplify the complex expression (3 + 2i) + (4 – 5i).
To simplify this expression, we can combine the real parts and the imaginary parts separately:
- Add the real parts: 3 + 4 = 7.
- Add the imaginary parts: 2i – 5i = -3i.
- Combine the real and imaginary parts: 7 – 3i.
Therefore, the simplified form of (3 + 2i) + (4 – 5i) is 7 – 3i.
Question 2:
Simplify the complex expression (2 – 3i) – (5 + 4i).
To simplify this expression, we can distribute the negative sign and then combine the real parts and the imaginary parts separately:
- Distribute the negative sign: 2 – 3i – 5 – 4i = -3 – 7i.
- Combine the real parts: -3 – 5 = -8.
- Combine the imaginary parts: -3i – 4i = -7i.
- Combine the real and imaginary parts: -8 – 7i.
Therefore, the simplified form of (2 – 3i) – (5 + 4i) is -8 – 7i.
Question 3:
Simplify the complex expression (6 + 2i)(3 – 4i).
To simplify this expression, we can use the FOIL method (First, Outer, Inner, Last) to distribute the terms:
- Multiply the first terms: 6 * 3 = 18.
- Multiply the outer terms: 6 * -4i = -24i.
- Multiply the inner terms: 2i * 3 = 6i.
- Multiply the last terms: 2i * -4i = -8i^2.
- Combine like terms: 18 – 24i + 6i – 8i^2.
- Replace i^2 with -1: 18 – 24i + 6i – 8(-1).
- Simplify: 18 – 24i + 6i + 8 = 26 – 18i.
Therefore, the simplified form of (6 + 2i)(3 – 4i) is 26 – 18i.
Continue following the step-by-step solutions to simplify the remaining questions on the worksheet. Remember to take your time and double-check your work as you go. Happy simplifying!
Common Mistakes to Avoid
When simplifying complex numbers, it’s important to be aware of common mistakes that can lead to incorrect answers. By avoiding these mistakes, you can ensure the accuracy of your solutions.
Forgetting to distribute the real and imaginary parts is a common error. When simplifying a complex number, it’s crucial to distribute any coefficients to both the real and imaginary parts. For example, if you have the expression 3(2 + 4i), you should distribute the 3 to both the 2 and the 4i, resulting in 6 + 12i.
Confusing the square root of negative numbers is another common mistake. When simplifying complex numbers, you’ll often encounter the square root of a negative number, represented by the imaginary unit i. Remember that i^2 is equal to -1, so when you square i, you obtain -1. Be careful to correctly simplify expressions involving i.
Forgetting to combine like terms is an error that can lead to incorrect answers. When simplifying complex numbers, it’s important to combine like terms, just like you would in algebra. For example, if you have the expression (3 + 2i) + (-2 – i), you should combine the real parts (3 + (-2) = 1) and the imaginary parts (2i + (-i) = i), resulting in 1 + i.
Not following the correct order of operations can also lead to mistakes. When simplifying complex numbers, it’s important to follow the correct order of operations, just like you would in any other mathematical expression. This means performing multiplication and division before addition and subtraction. Be sure to double-check your steps to ensure that you’re following the correct order.
Using incorrect formulas or rules is a mistake that can result in incorrect simplifications. When simplifying complex numbers, make sure you’re using the appropriate formulas and rules. For example, the formula for multiplying complex numbers is (a + bi)(c + di) = ac + adi + bci + bdi^2. Using the incorrect formula could lead to incorrect simplifications.
- Forgetting to distribute coefficients to both the real and imaginary parts
- Confusing the square root of negative numbers
- Forgetting to combine like terms
- Not following the correct order of operations
- Using incorrect formulas or rules
Advanced Techniques for Simplifying Complex Numbers
When working with complex numbers, it is often necessary to simplify them in order to make calculations more manageable. There are several advanced techniques that can be used to simplify complex numbers, making them easier to manipulate and understand.
1. Rationalizing Denominators: One common technique is to rationalize the denominators of complex numbers. This involves multiplying both the numerator and denominator of the complex number by the conjugate of the denominator. By doing this, the resulting complex number will have a rational denominator, making it easier to simplify further.
2. Simplifying Expressions: Another technique is to simplify complex expressions by combining like terms and performing arithmetic operations. This can involve adding or subtracting the real and imaginary parts separately, and then combining them to get the final simplified expression.
3. Using Exponential Form: Complex numbers can also be simplified using exponential form, where the complex number is written as a product of a magnitude and a complex exponential. This form can be useful for simplifying complex numbers in calculations involving powers, roots, or other exponential operations.
4. Factoring Quadratic Equations: Complex numbers can also be simplified by factoring quadratic equations. By factoring the quadratic equation into its roots, the complex numbers can be expressed in a simpler form, making them easier to work with.
These are just a few advanced techniques for simplifying complex numbers. It is important to understand and practice these techniques in order to efficiently work with complex numbers and perform calculations accurately. By simplifying complex numbers, mathematicians and scientists can work with them more easily and gain a deeper understanding of their properties and applications.