
In Lesson 10 4 of Geometry, students are presented with a set of exercises to practice their skills in solving geometric problems. This article will provide answers and explanations for Practice B of Lesson 10 4, helping students to check their work and deepen their understanding of the concepts being taught.
The exercises in Practice B of Lesson 10 4 involve solving problems related to angles, triangles, and other geometric shapes. By providing the answers and explanations for these exercises, students can compare their own solutions and reasoning with the correct ones, identifying any areas where they may need to review or seek further clarification.
Geometry is a branch of mathematics that deals with the properties and relationships of lines, angles, shapes, and spaces. It is an essential subject for anyone interested in fields such as architecture, engineering, or physics. By mastering the skills and concepts taught in Lesson 10 4, students will be better equipped to tackle more complex geometric problems in the future.
Overall, this article provides a valuable resource for students studying Geometry and working through Lesson 10 4 Practice B. By checking their answers and understanding the explanations provided, students can enhance their learning and improve their problem-solving skills in the realm of geometry.
Understanding the concept and objectives of Lesson 10 4 in geometry
In Lesson 10 4 in geometry, students will focus on the concept of congruent triangles and the various methods to prove their congruency. The objective of this lesson is to develop an understanding of congruent triangles and how they can be used to solve problems and prove geometric theorems.
Congruent triangles are triangles that have the same size and shape. They have congruent corresponding sides and congruent corresponding angles. In Lesson 10 4, students will learn about the different criteria that can be used to prove the congruence of triangles, including side-side-side (SSS), side-angle-side (SAS), angle-side-angle (ASA), angle-angle-side (AAS), and hypotenuse-leg (HL).
Throughout the lesson, students will explore various properties and theorems related to congruent triangles, such as the CPCTC theorem (Corresponding Parts of Congruent Triangles are Congruent), which states that if two triangles are congruent, then their corresponding parts (sides and angles) are also congruent.
By understanding the concept of congruent triangles and knowing how to prove their congruency using different criteria, students will be able to solve problems involving triangles and apply geometric theorems. The lesson also aims to enhance students’ logical reasoning and problem-solving skills within the context of geometry.
Lesson 10-4 in geometry focuses on the concept of trigonometric ratios and their applications. Trigonometric ratios are mathematical functions that relate the angles of a triangle to the lengths of its sides. This lesson provides a detailed explanation of the three main trigonometric ratios: sine, cosine, and tangent.
Sine: The sine of an angle in a right triangle is defined as the ratio of the length of the side opposite the angle to the length of the hypotenuse. It is represented as sin(A) = opposite/hypotenuse.
Cosine: The cosine of an angle in a right triangle is defined as the ratio of the length of the adjacent side to the length of the hypotenuse. It is represented as cos(A) = adjacent/hypotenuse.
Tangent: The tangent of an angle in a right triangle is defined as the ratio of the length of the side opposite the angle to the length of the adjacent side. It is represented as tan(A) = opposite/adjacent.
In Lesson 10-4, students are introduced to the steps for finding the trigonometric ratios of an angle. These steps involve identifying the given angle, labeling the sides of the triangle, and using the appropriate trigonometric ratio to find the unknown side length.
The lesson also covers the applications of trigonometric ratios in real-life scenarios, such as finding the height of a tree or the length of a shadow. Students learn how to set up and solve trigonometric equations to find the unknown values in these situations.
Overall, Lesson 10-4 provides a comprehensive understanding of trigonometric ratios and their practical applications, allowing students to apply these concepts to solve various types of geometry problems.
Step-by-step guide to solving geometry problems in Lesson 10 4
Geometry problems can sometimes be challenging, but with a step-by-step approach, you can easily solve them. In Lesson 10 4, we will focus on specific types of problems and their solutions. Let’s go through the process:
1. Read the problem: Begin by carefully reading the problem and understanding the given information. Identify what the problem is asking and the specific figures or shapes involved.
2. Draw a diagram: Visualize the problem by drawing a clear and accurate diagram. Include all the given information and label the different parts of the figure.
3. Identify known and unknown variables: Determine what information is already given in the problem and what needs to be found. This will help you set up the necessary equations or relationships.
4. Apply appropriate theorems or formulas: Use the geometric theorems or formulas relevant to the problem. Make sure you understand how to apply them correctly.
5. Solve the equations or relationships: Use the known and unknown variables to set up and solve the necessary equations or relationships. Show all the steps clearly and carefully.
6. Check your solution: After finding the solution, double-check your work to ensure that it makes sense in the context of the problem. Verify that your solution satisfies all the given conditions.
7. Communicate your answer: Finally, clearly state your answer in a way that makes sense in the problem’s context. Use appropriate units of measurement and provide any additional explanations if necessary.
By following these steps, you can approach geometry problems in Lesson 10 4 with confidence and successfully find the solutions. Practice these steps with various problem types to enhance your problem-solving skills.
Common Challenges Faced while Practicing Lesson 10.4 in Geometry
When practicing Lesson 10.4 in geometry, students often encounter several common challenges that can hinder their understanding and progress in the subject. These challenges can range from difficulties with the concepts presented in the lesson to struggles with problem-solving and application of the learned material.
One common challenge is understanding the basic properties and characteristics of quadrilaterals, such as rectangles, squares, and parallelograms. Students may struggle to differentiate between these shapes and accurately identify their defining features. This can impact their ability to solve problems related to these figures and hinder their overall understanding of geometric concepts.
- Another challenge is comprehending the various theorems and postulates that are introduced in Lesson 10.4. These theorems, such as theorem 10.4.1, which states that opposite sides of a rectangle are congruent, can be complex and require careful attention to detail. Students may struggle to apply these theorems correctly in problem-solving situations, leading to incorrect answers and confusion.
- Problem-solving itself can also pose a challenge during the practice of Lesson 10.4. Some students may struggle with identifying the appropriate steps and strategies to solve geometry problems effectively. They may find it difficult to visualize the given information and apply the relevant theorems or formulas to solve the problem accurately.
- Additionally, time management can be a concern while practicing Lesson 10.4 in geometry. Students may find themselves spending too much time on individual problems, causing them to rush through the remaining exercises or not have enough time to fully understand and absorb the lesson’s content.
- Identify the given information: Start by carefully reading the problem and identifying all the information that is provided. Look for given measurements, angles, or specific conditions that are mentioned.
- Determine your goal: Understand what the problem is asking you to find or prove. This will help you focus on the relevant information and steps needed to reach the solution.
- Apply the appropriate formulas and theorems: Geometry is based on a set of rules and principles. Use the appropriate formulas and theorems to solve for the unknowns. This may involve finding angles, side lengths, or congruent figures.
- Draw accurate diagrams: Visualization is key in geometry. Draw accurate diagrams that represent the given information and the relationships between different elements. This will help you visualize the problem and identify any additional information needed.
- Use logical reasoning: Geometry problems often require logical reasoning skills. Think through the problem step by step, making logical deductions and using deductive reasoning to arrive at the solution.
- Check your answers: Once you have found a solution, always double-check your work and make sure your answer makes sense. If possible, re-read the problem and see if your solution aligns with the given information and the question being asked.
- Overall, Lesson 10 4 introduces important formulas and concepts related to geometry that are essential for solving various geometric problems.
- These include the Pythagorean Theorem, similarity, the area of a triangle, congruence, the volume of a cylinder, the angle sum property, perpendicular bisectors, and similar triangles.
- Understanding and applying these formulas and concepts will help in solving geometry problems and exploring the properties of different geometric figures.
Overall, it is important for students to actively engage with the material, seek clarification when needed, and practice regularly to overcome these challenges and develop a strong foundation in geometry.
Strategies and Tips to Overcome Challenges in Lesson 10 4 Practice
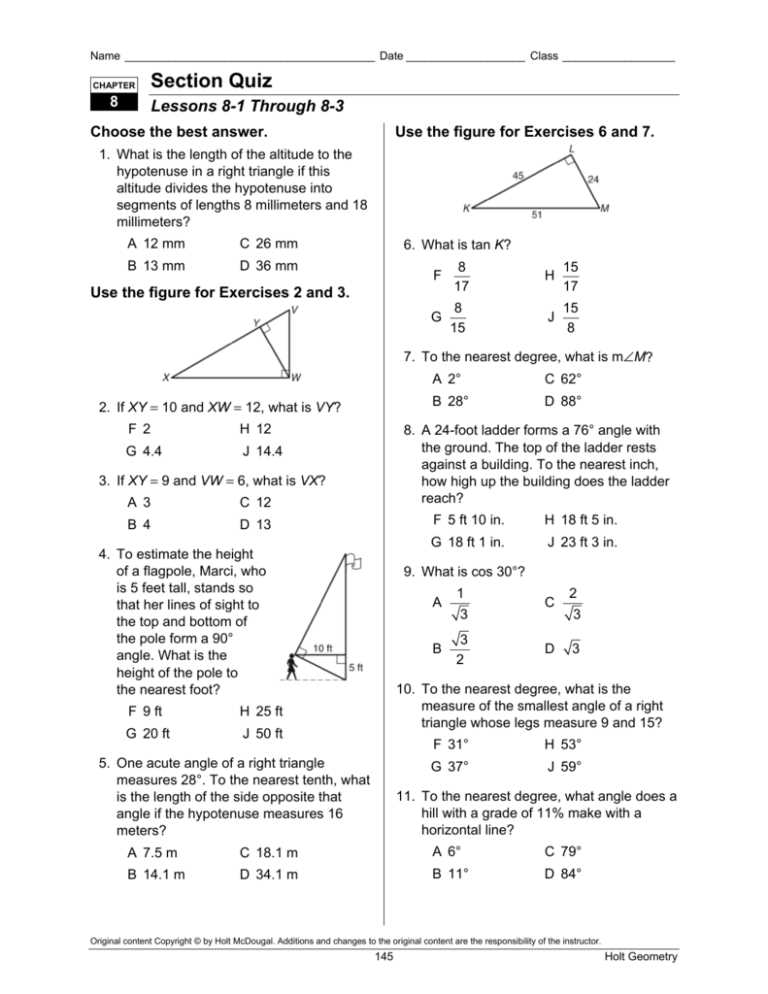
When facing challenges in Lesson 10 4 practice, it is important to approach the problems with a strategic mindset. By following certain tips and techniques, you can improve your understanding and tackle the difficulties more effectively.
1. Understand the problem: Before you begin solving the practice questions, take the time to fully comprehend the problem statement. Highlight any key information or clues that can help you determine the appropriate approach to solve it.
2. Review relevant concepts: Lesson 10 4 practice may require a solid understanding of certain concepts and formulas. Take a moment to review these concepts before attempting the questions. This will ensure that you have a strong foundation and can apply the correct principles to solve the problems.
3. Break down complex problems: Sometimes, the questions in Lesson 10 4 practice may seem overwhelming due to their complexity. In such cases, break down the problem into smaller, more manageable steps. This will make the problem-solving process easier and help you identify any potential solutions more effectively.
4. Practice regularly: Consistency is key when it comes to improving your problem-solving skills. Make it a habit to practice regularly, not just during Lesson 10 4 practice but also throughout your geometry studies. This will allow you to reinforce your understanding and become more comfortable with various problem-solving techniques.
5. Seek help if needed: If you find yourself stuck on a particular problem or concept in Lesson 10 4 practice, don’t hesitate to seek help. Ask your teacher or classmates for clarification or additional guidance. Sometimes, an outside perspective can provide new insights and help you overcome challenges more effectively.
6. Analyze your mistakes: After completing Lesson 10 4 practice, take the time to review your answers and analyze any mistakes you made. Understanding your errors can help you identify areas where you need improvement and allow you to adjust your study strategy accordingly.
7. Stay positive and persistent: Overcoming challenges in Lesson 10 4 practice may require time and effort. Stay positive and persistent, even if you encounter difficulties. Believe in your ability to improve and approach each problem with determination and a growth mindset.
Key points to remember while solving Lesson 10 4 practice problems in geometry
When tackling the practice problems in Lesson 10 4 of geometry, there are several key points to remember. These points will help guide your thinking and approach to solving the problems, ensuring that you arrive at the correct answers:
By keeping these key points in mind, you will be better equipped to solve the practice problems in Lesson 10 4 of geometry. Remember to take your time, think through each step, and always review and check your work for accuracy.
Important Formulas and Concepts related to Lesson 10 4
Lesson 10 4 introduces important formulas and concepts related to geometry. These formulas and concepts are essential for understanding and solving various geometric problems.
1. Pythagorean Theorem: The Pythagorean Theorem states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. It can be written as a^2 + b^2 = c^2, where a and b are the lengths of the two legs of the right triangle and c is the length of the hypotenuse.
2. Similarity: Similarity is a concept in geometry that refers to the relationship between two figures that have the same shape but different sizes. Two figures are said to be similar if their corresponding angles are congruent and the ratios of their corresponding sides are equal.
3. Area of a Triangle: The area of a triangle can be calculated using the formula A = (1/2) * base * height, where A is the area, base is the length of the base of the triangle, and height is the perpendicular distance from the base to the opposite vertex.
4. Congruence: Congruence is a concept in geometry that refers to the relationship between two figures that have the same shape and size. Two figures are said to be congruent if their corresponding angles and sides are congruent.
5. Volume of a Cylinder: The volume of a cylinder can be calculated using the formula V = π * r^2 * h, where V is the volume, π is a mathematical constant approximately equal to 3.14159, r is the radius of the base of the cylinder, and h is the height of the cylinder.
6. Angle Sum Property: The angle sum property states that the sum of the interior angles of a triangle is always equal to 180 degrees. This property is useful for solving problems that involve finding the measure of an unknown angle in a triangle.
7. Perpendicular Bisector: A perpendicular bisector is a line or line segment that intersects another line segment at its midpoint and forms a right angle with it. The perpendicular bisector of a line segment is useful for finding the midpoint of the segment, as well as for constructing congruent angles or triangles.
8. Similar Triangles: Similar triangles are triangles that have the same shape but different sizes. Two triangles are said to be similar if their corresponding angles are congruent and the ratios of their corresponding sides are equal.