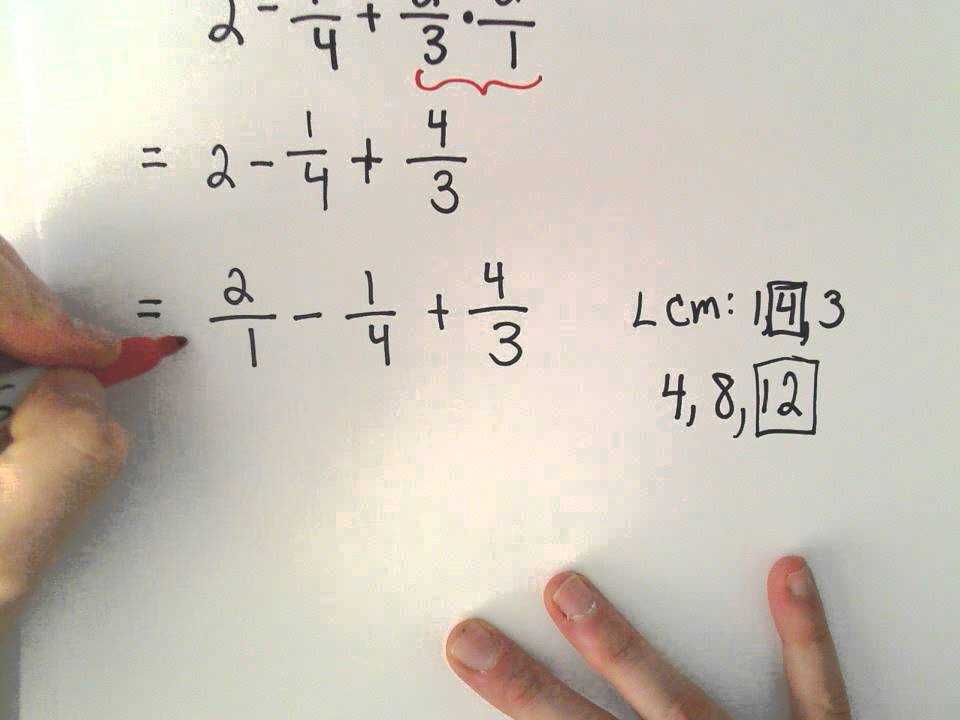
In Week 1 of your math course, you have covered decimal and fraction operations, and now it’s time to check your answers with the answer key. This answer key will help you evaluate your understanding of the topics covered and identify any areas where you may need additional practice.
The answer key includes solutions to various exercises and problems that you have encountered in Week 1. It provides step-by-step explanations and calculations, allowing you to compare your own answers and methods with the correct ones.
By using the answer key, you can verify if you have performed the operations correctly and grasp the concepts involved in decimal and fraction operations. This key serves as a valuable resource to help you improve your math skills and build confidence in working with decimals and fractions.
Week 1 Decimal and Fraction Operations Answer Key
In the first week of our decimal and fraction operations lesson, we covered various topics such as adding, subtracting, multiplying, and dividing decimals and fractions. This answer key provides the correct solutions for the practice problems given in the week’s assignments. Let’s take a look at some of the key concepts and solutions.
1. Adding Decimals:
- To add decimals, align the decimal points and add the numbers as usual.
- For example, to add 3.78 and 2.56, we align the decimals and get 6.34 as the sum.
2. Subtracting Fractions:
- To subtract fractions, find a common denominator and then subtract the numerators.
- For example, to subtract 1/2 from 3/4, we need a common denominator of 4. So the solution is (3-2)/4 = 1/4.
3. Multiplying Decimals:
- To multiply decimals, ignore the decimal points and multiply the numbers as if they were whole numbers.
- Count the total number of digits after the decimal points in the factors and place the decimal point accordingly in the product.
- For example, to multiply 2.5 and 0.4, we ignore the decimal points and get 25 x 4 = 100. Since there is one digit after the decimal point in the factors, we place the decimal point in the product two places from the right, resulting in 1.00.
4. Dividing Fractions:
- To divide fractions, multiply the first fraction by the reciprocal of the second fraction.
- For example, to divide 2/3 by 4/5, we multiply 2/3 by 5/4 (the reciprocal of 4/5) and get (2/3) x (5/4) = 10/12. Simplifying this fraction gives us 5/6 as the solution.
This answer key provides the correct solutions for various types of decimal and fraction operations. It is important to practice these concepts to gain proficiency in working with decimals and fractions. Make sure to check your answers with this answer key to ensure accuracy in your work.
Adding Decimals and Fractions
Adding decimals and fractions involves different methods and steps, but both processes are essential in everyday life and various mathematical calculations. By understanding the concepts of decimals and fractions and learning the proper techniques, we can effectively add these numbers and solve complex problems.
When adding decimals, it is crucial to align the decimal points before carrying out the addition. Start by lining up the decimal points of the numbers being added. Then, add the digits in each column, starting from the rightmost column. If there is a carry, it should be added to the next column. Once all the columns have been added, the sum can be expressed as a decimal. It is important to remember to retain the decimal point in its correct position.
Example:
Add 1.25 and 3.6
| 1 | . | 2 | 5 | |
| + | 3 | . | 6 | 0 |
| = | 4 | . | 8 | 5 |
Adding fractions follows a different process. When adding fractions with the same denominator, simply add the numerators and keep the denominator the same. If the fractions have different denominators, they need to be converted to the same denominator before addition. This can be done by finding the least common denominator (LCD) of the fractions and adjusting the numerators accordingly. Once the fractions have the same denominator, the numerators can be added, and the sum can be expressed as a fraction.
Example:
Add 3/4 and 1/5
| 3/4 | + | 1/5 | = | ? |
To find the LCD, we need to identify the least common multiple (LCM) of 4 and 5, which is 20. We then adjust the numerators accordingly:
| 3/4 | + | 1/5 | = | 15/20 | + | 4/20 |
Adding the adjusted numerators, we get:
| 15/20 | + | 4/20 | = | 19/20 |
Therefore, the sum of 3/4 and 1/5 is 19/20.
Subtracting Decimals and Fractions
Subtracting decimals and fractions can be a challenging task for students, but with practice and understanding, it becomes easier. The key to successfully subtracting decimals and fractions is to have a clear understanding of the concept and to follow a systematic approach.
When subtracting decimals, it is important to align the decimal points before performing the subtraction. This ensures that the numbers are correctly lined up and the decimal points are properly placed in the answer. Once the decimals are aligned, subtraction can be performed as usual, starting from the rightmost digit.
For example, if we have the problem 5.72 – 3.46, we would align the decimal points as follows:
| 5 | . | 7 | 2 | |
| – | 3 | . | 4 | 6 |
Subtracting the numbers from right to left, we get the answer 2.26.
When subtracting fractions, it is important to have a common denominator before performing the subtraction. This allows us to subtract the numerators directly. If the denominators are not the same, we need to find a common denominator by finding the least common multiple (LCM) of the denominators.
For example, if we have the problem 3/4 – 1/6, we need to find a common denominator. The LCM of 4 and 6 is 12, so we can rewrite the fractions with the common denominator:
- 3/4 = 9/12
- 1/6 = 2/12
Now, we can subtract the numerators: 9 – 2 = 7. The denominator remains the same, so the final answer is 7/12.
By understanding the concepts and following a systematic approach, subtracting decimals and fractions becomes a manageable task, helping students improve their math skills.
Multiplying Decimals and Fractions
In mathematics, multiplying decimals and fractions is a fundamental operation that is commonly encountered in various real-life scenarios. This operation involves multiplying a decimal number and a fraction to find their product.
To multiply a decimal and a fraction, we can first convert the decimal to a fraction by placing it over a denominator of 1 followed by the required number of zeros. Then, we can multiply the fractions using the standard method of multiplying fractions: multiplying the numerators and multiplying the denominators. Finally, we simplify the resulting fraction, if necessary.
For example, let’s consider the problem of multiplying 0.5 with the fraction 2/3. We can convert 0.5 to a fraction by writing it as 5/10. Now, we can multiply the fractions: (5/10) * (2/3). Multiplying the numerators, we get 10, and multiplying the denominators, we get 30. Therefore, the product of 0.5 and 2/3 is equal to 10/30.
In some cases, it may be necessary to convert the resulting fraction to a decimal. This can be done by dividing the numerator by the denominator. In our example, 10 divided by 30 equals 0.3333… or approximately 0.33 when rounded to two decimal places.
Overall, multiplying decimals and fractions is a straightforward process that involves converting decimals to fractions, multiplying the fractions, and simplifying the result if needed. This operation is essential in various mathematical and real-world contexts, such as calculating discounts, finding percentages, and solving measurement problems.
Dividing Decimals and Fractions

Dividing decimals and fractions is an important skill in mathematics that allows us to solve real-world problems and perform calculations with precision. By understanding and mastering the concepts of division, we can accurately determine how much of something is contained within another quantity.
When dividing decimals, we follow similar rules as when dividing whole numbers. However, we need to pay attention to the placement of the decimal point. We can start by moving the decimal point in the divisor to the right until it becomes a whole number. Next, we move the decimal point in the dividend the same number of places to the right. Finally, we divide the modified dividend by the modified divisor to obtain the quotient.
When it comes to dividing fractions, we can use the concept of reciprocal or the “flipping” method. This means that we take the reciprocal of the divisor by flipping the numerator and the denominator. Then, we multiply the dividend by the reciprocal of the divisor. This process simplifies the division of fractions and allows us to obtain the quotient.
Overall, dividing decimals and fractions requires attention to detail and a thorough understanding of the principles of division. By applying the proper techniques and following the rules, we can accurately divide decimals and fractions and solve various mathematical problems.
Converting Decimals to Fractions
Converting decimals to fractions is a fundamental skill in mathematics. It allows us to represent decimal numbers as fractions, which can sometimes make calculations or comparisons easier. To convert a decimal to a fraction, we need to understand the relationship between decimals and fractions.
A decimal is a way of expressing a fraction or a part of a whole using a base-10 system. It consists of a whole number part followed by a decimal point and a fractional part. The digits to the right of the decimal point represent smaller parts of the whole number or fraction.
To convert a decimal to a fraction, we can use a simple process. We first write down the decimal as a fraction with the decimal part as the numerator and the place value of the last digit as the denominator. Then, we simplify the fraction if possible by canceling out common factors between the numerator and denominator.
For example, let’s convert the decimal 0.75 to a fraction. We can write it as 75/100 because the last digit is in the hundredths place. Simplifying the fraction, we find that 75/100 is equal to 3/4.
Converting decimals to fractions is important in various mathematical operations, such as adding, subtracting, multiplying, and dividing. It allows us to perform calculations with decimals more easily, as working with fractions often provides simpler or more intuitive results.
Overall, understanding how to convert decimals to fractions is a valuable skill that can enhance our mathematical abilities and aid in solving problems in various contexts.
Converting Fractions to Decimals
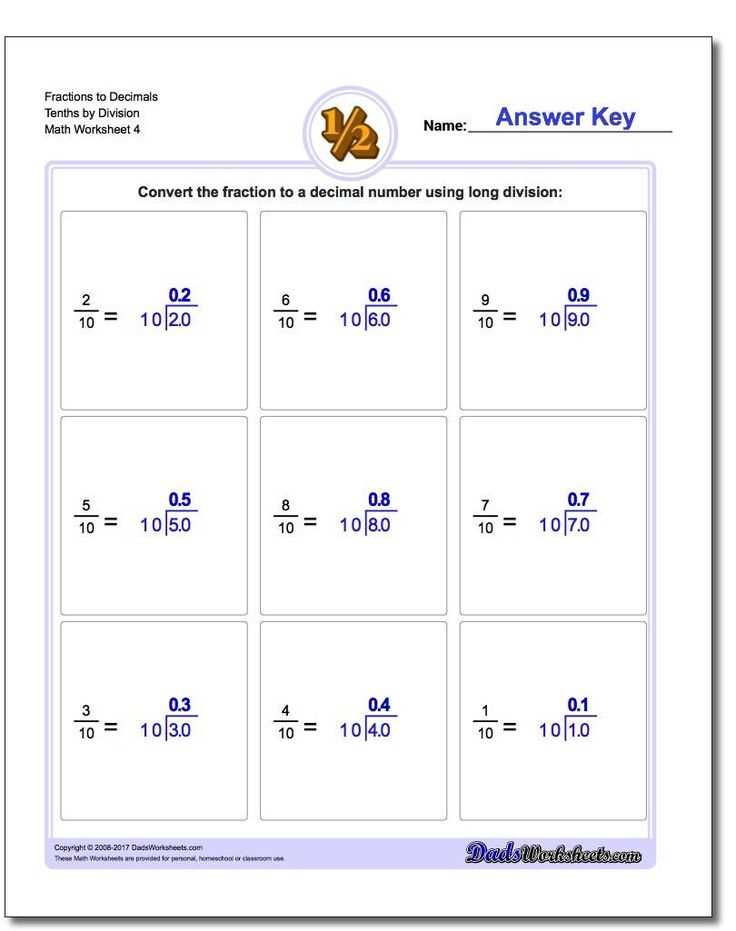
Converting fractions to decimals is an important skill in mathematics. Fractions represent a part of a whole, while decimals represent a number in the base-10 system. In order to convert a fraction to a decimal, we need to divide the numerator (the top number) by the denominator (the bottom number). The result of this division will be the decimal representation of the fraction.
For example, let’s convert the fraction 3/4 to a decimal. We divide 3 by 4, which gives us 0.75. Therefore, the decimal equivalent of 3/4 is 0.75. We can also express this as a percentage, which would be 75%. It’s important to note that fractions can have terminating decimals, like 3/4, or repeating decimals, like 1/3.
To convert a fraction to a decimal, we can follow a few simple steps. First, we divide the numerator by the denominator. If the division is not exact, we can continue dividing until we reach the desired level of decimal places or until the division repeats. It’s also common to round the decimal at a certain decimal place, depending on the context or the desired level of precision.
In summary, converting fractions to decimals involves dividing the numerator by the denominator. This allows us to represent fractions as decimals, which can be useful in various mathematical calculations and real-life situations. Understanding this process is essential for building a strong foundation in mathematics.
Simplifying Fractions
When working with fractions, it is often necessary to simplify them to their simplest form. Simplifying fractions involves finding the equivalent fraction with the smallest possible numerator and denominator. This makes it easier to work with and compare fractions.
In order to simplify a fraction, you need to find the greatest common divisor (GCD) of the numerator and denominator. The GCD is the largest number that divides both the numerator and denominator evenly. Once you have found the GCD, you can divide both the numerator and denominator by this number to simplify the fraction.
For example, let’s say we have the fraction 8/12. The GCD of 8 and 12 is 4. So, we can divide both the numerator and denominator by 4 to get 2/3, which is the simplified form of the fraction.
It is important to simplify fractions because it allows for easier calculations and comparisons. Simplified fractions are also easier to understand and interpret in everyday situations. For example, if you have a recipe that calls for 1/2 cup of sugar, it is much easier to measure out 1/2 cup than it is to measure out 4/8 or 6/12 cup.
Overall, simplifying fractions is an essential skill when working with fractions. It allows for easier calculations, comparisons, and understanding of fractions in various contexts.