
Geometry is a branch of mathematics that deals with the properties and relationships of points, lines, and shapes in space. It is a subject that is widely studied in schools and universities around the world. In lesson 1 7 practice a geometry answers, students are introduced to various concepts and principles in geometry, and are required to apply their knowledge and problem-solving skills to find solutions to different mathematical problems.
This lesson focuses on answering questions related to geometric figures and their properties. Students are given a set of problems which require them to find missing angles, measure lengths, calculate areas and volumes, and apply geometric formulas. By solving these problems, students not only reinforce their understanding of geometric concepts, but also develop their critical thinking and analytical skills.
One of the key skills that students learn in lesson 1 7 practice a geometry answers is how to use logical reasoning to derive conclusions and solve problems. They are encouraged to use deductive reasoning to analyze the given information, make connections between different geometric properties, and arrive at a logical solution. This process of problem-solving helps students develop their problem-solving abilities and prepares them for more complex mathematical concepts in the future.
In conclusion, lesson 1 7 practice a geometry answers provides students with the opportunity to deepen their understanding of geometry and develop their problem-solving skills. Through solving a variety of geometric problems, students learn to apply their knowledge of geometric concepts and principles to find solutions. This lesson is an important stepping stone in their journey to mastering the subject of geometry.
Exploring the Basics of Geometry
In mathematics, geometry is a branch that deals with the properties and relationships of shapes, sizes, and angles. It is an essential field of study that provides a foundation for various scientific and technological advancements. By understanding the basics of geometry, we can analyze and solve real-world problems in fields such as architecture, engineering, and art.
One fundamental concept in geometry is the notion of points, lines, and planes. A point is a location represented by a dot, and it has no size or shape. A line is a straight path that extends indefinitely in both directions, composed of an infinite number of points. A plane is a flat surface that extends infinitely in all directions. These building blocks allow us to construct and analyze more complex geometric figures.
Another important aspect of geometry is angles. An angle is formed by two rays that share a common endpoint, called the vertex. The size of an angle is measured in degrees, with a full circle measuring 360 degrees. Angles are classified based on their measure, ranging from acute angles (less than 90 degrees) to obtuse angles (more than 90 degrees) and right angles (exactly 90 degrees).
Geometry also involves exploring different types of polygons, which are closed shapes with straight sides. Some common polygons include triangles, quadrilaterals, pentagons, and hexagons. Each polygon has its own unique properties and formulas that allow us to calculate their areas, perimeters, and angles.
Overall, studying the basics of geometry provides us with a framework for understanding the relationships, properties, and measurements of shapes and angles. It allows us to analyze and solve problems in various fields, making it an essential subject in mathematics and beyond.
Understanding Angle Relationships

In geometry, angles play a crucial role in understanding the relationships between different shapes and figures. By studying angle relationships, we can make connections and solve various problems related to angles.
One important concept in angle relationships is the concept of complementary angles. Complementary angles are two angles that add up to 90 degrees. For example, if one angle measures 30 degrees, then its complementary angle would measure 60 degrees. Understanding complementary angles helps us solve problems involving angles in different shapes.
Another concept related to angle relationships is supplementary angles. Supplementary angles are two angles that add up to 180 degrees. For instance, if one angle measures 120 degrees, then its supplementary angle would measure 60 degrees. Recognizing supplementary angles allows us to find missing angles and solve equations involving angles.
Angle relationships can also be seen in parallel lines and transversals. When a transversal intersects two parallel lines, various angle relationships are formed. Some key angle relationships include corresponding angles, alternate interior angles, alternate exterior angles, and consecutive interior angles. Understanding these angle relationships helps us prove geometric properties and determine the measures of unknown angles.
In conclusion, understanding angle relationships is essential in geometry. By knowing the properties and relationships of angles, we can solve problems, make connections, and prove geometric concepts. Whether it is finding complementary or supplementary angles, or understanding angle relationships in parallel lines and transversals, a solid grasp of angle relationships is fundamental in the study of geometry.
Solving Equations Involving Parallel Lines
When dealing with parallel lines, it is important to understand the relationships between the angles formed by these lines. Parallel lines have a unique property that allows us to solve equations involving their angles.
One important relationship is the corresponding angles formed by a transversal line intersecting two parallel lines. Corresponding angles are congruent, meaning they have the same measure. This property can be used to solve equations by setting the measures of corresponding angles equal to each other.
For example, consider the following scenario: Line l and line m are parallel, and line t is a transversal intersecting both l and m. Angle A is a corresponding angle to angle B. If we know the measure of angle A is 60 degrees, we can set up the equation:
60 = x
where x represents the measure of angle B. By solving this equation, we can find the measure of angle B.
Another relationship involving parallel lines is the alternate interior angles. Alternate interior angles are also congruent, meaning they have the same measure. This property can be used to solve equations when given the measures of other alternate interior angles.
By understanding these relationships and using algebraic equations, we can solve for the measures of angles involved in parallel lines. This knowledge is crucial in many areas of geometry and can help us solve a wide range of problems.
Applying Properties of Triangles
When studying geometry, one of the fundamental shapes we encounter is the triangle. Triangles have unique properties that help us analyze and solve various geometric problems. By understanding these properties, we can apply them to different situations and make accurate deductions about the triangle’s angles, sides, and relationships with other shapes.
One of the essential properties of a triangle is the sum of its interior angles. According to the Triangle Sum Theorem, the sum of the three interior angles of any triangle is always 180 degrees. This property allows us to find missing angles in a triangle by subtracting the given angles from 180 degrees. It also helps us classify triangles based on their angle measures, such as acute, right, and obtuse triangles.
Another crucial property of triangles is the relationship between their sides and angles. In a right triangle, for example, one of the angles is always 90 degrees, and the side opposite to this right angle is called the hypotenuse. The Pythagorean Theorem allows us to find the length of the hypotenuse or the other two sides if we know the lengths of the other two sides. Additionally, the Law of Sines and Law of Cosines provide formulas to find missing side lengths or angles in any triangle.
To further analyze triangles, we can apply different properties and theorems, such as the Angle Bisector Theorem, perpendicular bisectors, medians, altitudes, and the Triangle Inequality Theorem. These properties help us determine the relationship between various parts of a triangle and highlight important characteristics that can be used to solve complex problems.
In conclusion

Understanding and applying the properties of triangles is essential in geometry. By knowing the sum of interior angles, relationships between sides and angles, and utilizing different theorems, we can analyze and solve a wide range of geometric problems. These properties provide us with a solid foundation to explore more complex concepts and further our understanding of geometry.
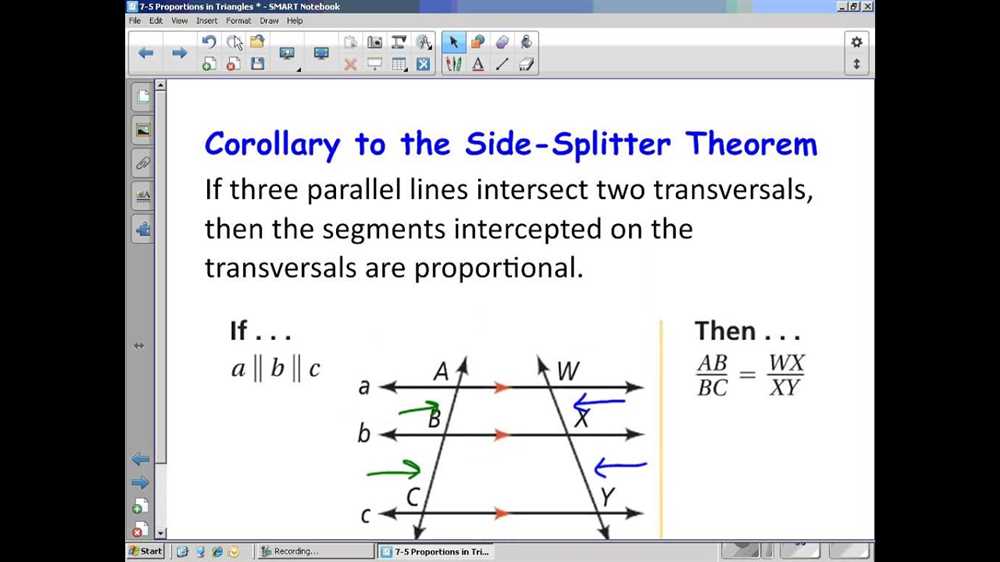
Finding Measures of Similar Figures
When dealing with similar figures, it is important to understand how to find the measures of corresponding sides and angles. Similar figures have the same shape but may differ in size. To find the measures of corresponding sides, you can use the concept of proportional reasoning. This means that the ratios of the lengths of corresponding sides in similar figures are equal.
For example, if you have two triangles that are similar, you can set up a proportion to find the measure of a corresponding side. If the lengths of the corresponding sides are represented by the variables x and y, you can set up the proportion x/y = a/b, where a and b are the lengths of the corresponding sides in the other triangle. By cross-multiplying, you can solve for x or y.
In addition to finding the measures of corresponding sides, you can also find the measures of corresponding angles in similar figures. The angles in similar figures are congruent, which means they have the same measure. This is true for corresponding angles, as well as for interior angles and exterior angles.
Overall, finding the measures of similar figures involves using proportional reasoning to find the lengths of corresponding sides, and recognizing that the angles in similar figures are congruent. By understanding these concepts and applying them to different geometric shapes, you can accurately determine the measures of similar figures.
Calculating Perimeter and Area

The calculation of perimeter and area is an essential aspect of geometry. Perimeter is the distance around the boundary of a shape, while area represents the amount of space enclosed by a shape. These measurements are often used in real-world scenarios to determine the amount of material needed for construction or to estimate the size of a space.
To calculate the perimeter of a shape, you need to find the sum of the lengths of all its sides. For example, in a rectangle with sides measuring 3 meters and 5 meters, the perimeter would be 16 meters (3 + 5 + 3 + 5). Similarly, the perimeter of a circle is calculated using the formula P = 2πr, where r is the radius of the circle.
The area of a shape, on the other hand, is the measure of the space enclosed within its boundaries. It is usually expressed in square units, such as square meters or square feet. The formula for calculating the area of a rectangle is A = length × width. For instance, if a rectangle measures 4 meters in length and 6 meters in width, the area would be 24 square meters (4 × 6). For a circle, the area is found using A = πr^2, where r is the radius of the circle.
By accurately calculating the perimeter and area of various shapes, we can make informed decisions in fields like architecture, landscaping, and engineering. These calculations allow us to optimize resource allocation, estimate costs, and create precise plans for construction projects. Whether it’s determining the amount of paint needed to cover a room or planning the layout of a garden, understanding how to calculate perimeter and area is a fundamental skill in geometry.
Understanding Volume and Surface Area
The concepts of volume and surface area are fundamental to geometry and have important applications in various fields such as engineering, architecture, and physics. Understanding these concepts is essential for solving problems related to the measurement, design, and analysis of three-dimensional objects.
Volume refers to the amount of space occupied by a three-dimensional object. It is measured in cubic units. The formula to calculate the volume of common shapes such as cubes, rectangular prisms, and cylinders involves multiplying the length, width, and height of the object. By understanding this formula, one can determine the amount of space within an object and make informed decisions regarding its usage, storage, or transportation.
Surface area, on the other hand, represents the total area of all the surfaces of a three-dimensional object. It is measured in square units. The formula to calculate the surface area varies depending on the shape of the object. For example, the surface area of a cube is found by multiplying the length of one side by itself, and then multiplying the result by six (since a cube has six identical square faces). Understanding surface area is crucial for determining the amount of material required to cover or construct an object, as well as for analyzing heat transfer or pressure distribution on the object’s surface.
By developing a solid understanding of volume and surface area, individuals can effectively analyze and manipulate three-dimensional objects in various contexts. This knowledge enables them to solve real-world problems, design efficient structures, and make informed decisions regarding space utilization, material usage, and other important considerations related to three-dimensional objects.
Reviewing and Applying Concepts with Practice Problems
In geometry, it is important to regularly review and apply the concepts learned in order to reinforce understanding and improve problem-solving skills. Practice problems provide an opportunity to test knowledge and build confidence in solving various types of geometric problems. By systematically working through practice problems, students become more familiar with the different concepts and develop strategies for approaching different types of questions.
One useful approach to practicing geometry is to start with basic concepts and gradually progress to more advanced topics. This ensures a solid foundation and allows for a smooth transition to more complex problems. The practice problems should cover a wide range of concepts, such as angles, triangles, quadrilaterals, circles, and three-dimensional figures.
When practicing geometry problems, it is helpful to identify the key information given in each problem and determine what is being asked. This helps in selecting the appropriate methods and formulas to use. It is also important to understand the relationships and properties of different geometric figures, as these can often be used to solve problems more efficiently.
It is advisable to work through practice problems systematically, starting with easier ones and gradually moving on to more challenging ones. This allows for a gradual progression and builds confidence along the way. It is also helpful to review the solutions and explanations for the problems, as this allows for a deeper understanding of the concepts and techniques used.
By regularly reviewing and applying the concepts learned through practice problems, students can improve their problem-solving skills and become more comfortable with geometry. This not only helps in achieving better grades but also prepares students for more advanced mathematical studies that may require a strong foundation in geometry.