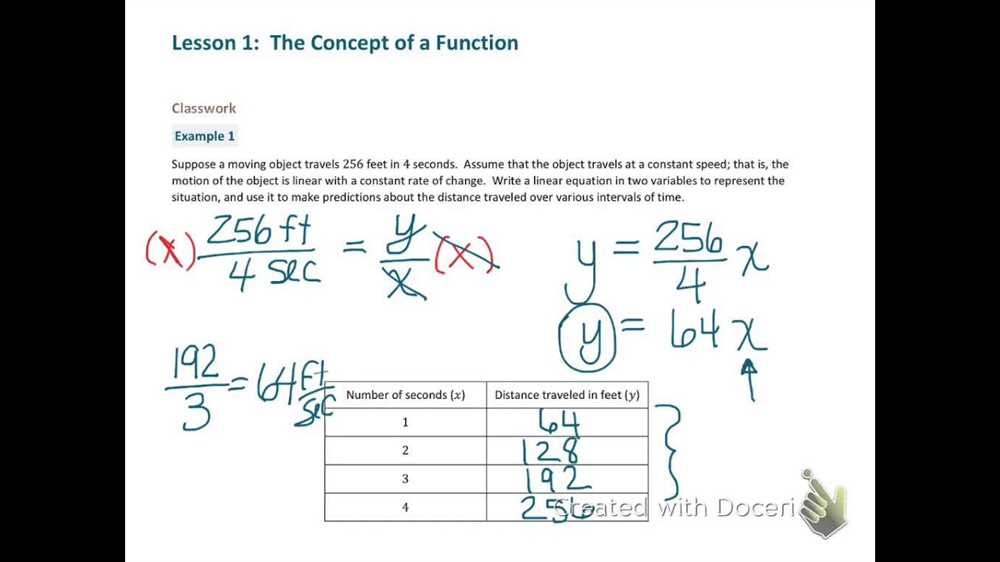
In Secondary Math 2 module 5, students delve deeper into the study of advanced mathematical concepts. This module focuses on various topics, including functions, exponential and logarithmic functions, and sequences and series. It is essential for students to understand and master these concepts to excel in higher mathematics and prepare for college-level courses.
As students progress through this module, they are introduced to complex equations and problems that require critical thinking and logical reasoning. They learn to apply mathematical principles to real-world situations, developing problem-solving skills that are invaluable in many professions.
The answer key for Secondary Math 2 module 5 is a valuable resource for both students and teachers. It provides step-by-step solutions to the exercises and problems presented in the module, allowing students to check their answers and understand the concepts better. Teachers can also use the answer key as a guide to assess their students’ progress and identify areas that may require additional instruction.
Overview of Secondary Math 2

Secondary Math 2 is a comprehensive course that builds on the foundational mathematics skills acquired in Secondary Math 1. It is designed to introduce students to more advanced concepts and problem-solving strategies in algebra, geometry, and statistics. This course is typically taken in the second year of high school, and it serves as a bridge between the fundamental concepts covered in Secondary Math 1 and the more advanced topics explored in Secondary Math 3.
The main goal of Secondary Math 2 is to deepen students’ understanding of mathematical concepts and strengthen their ability to apply these concepts in real-world situations. The course covers a wide range of topics, including linear equations and inequalities, exponential and logarithmic functions, quadratic functions, geometric transformations, probability, and data analysis. Throughout the course, students are encouraged to think critically, analyze data, and make connections between different mathematical ideas.
Key topics covered in Secondary Math 2 include:
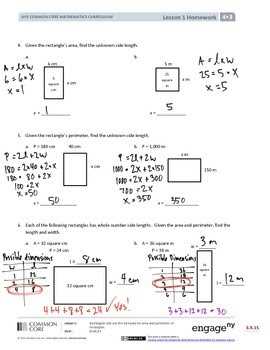
- Linear equations and inequalities
- Systems of linear equations and inequalities
- Exponential and logarithmic functions
- Quadratic functions
- Geometric transformations
- Properties of circles and polygons
- Probability and statistics
- Data analysis and interpretation
Secondary Math 2 provides students with the necessary skills and knowledge to excel in higher-level math courses and prepares them for college and career success. It emphasizes critical thinking, problem-solving, and mathematical reasoning, which are essential skills in today’s rapidly evolving world. By mastering the concepts and techniques covered in this course, students will become confident and capable mathematicians who can tackle complex mathematical problems with ease.
The Importance of Module 5
Module 5 of the Secondary Math 2 curriculum is a critical component in the development of a student’s mathematical understanding. This module focuses on quadratic functions and their applications, which are fundamental concepts in higher level math and real-world problem-solving.
Quadratic functions: In Module 5, students explore the properties and characteristics of quadratic functions, including vertex form, factoring, and graphing. Understanding quadratic functions is essential as they appear in various fields such as physics, engineering, economics, and computer science. These functions provide a framework for modeling and analyzing real-world phenomena.
Problem-solving skills: Module 5 not only teaches students about quadratic functions but also develops their problem-solving skills. By applying quadratic functions to real-life situations, students learn how to analyze data, make predictions, and solve complex problems. These skills are valuable not only in math but also in other academic subjects and future career paths.
Mathematical reasoning: Module 5 challenges students to think critically and reason mathematically. They learn how to analyze patterns, make connections between different mathematical concepts, and justify their reasoning. These skills promote a deeper understanding of mathematical principles and help students become more confident and proficient in their mathematical abilities.
Preparation for further math courses: Module 5 acts as a foundation for advanced math courses. The concepts and skills taught in this module, such as graphing quadratic functions and solving quadratic equations, are building blocks for more complex mathematical topics. Taking the time to fully grasp these concepts in Module 5 will set students up for success in future math courses.
In conclusion, Module 5 of the Secondary Math 2 curriculum is of utmost importance. It not only introduces students to quadratic functions but also equips them with problem-solving skills, strengthens their mathematical reasoning abilities, and prepares them for future math courses. Investing time and effort into mastering Module 5 will greatly benefit students in their academic and professional journeys.
Understanding Key Concepts in Secondary Math 2 Module 5
In Secondary Math 2 Module 5, students delve into the world of trigonometry and explore the relationships between angles, sides, and ratios of right triangles. This module builds upon the foundational concepts from previous modules and extends them to solve real-world problems using trigonometric functions.
One of the key concepts in Module 5 is the relationship between angles and the ratios of the sides of right triangles. Students learn about the sine, cosine, and tangent ratios and how they can be used to find missing side lengths or angle measures in a right triangle. They also learn about the reciprocal trigonometric ratios, cosecant, secant, and cotangent, and how they relate to the original trigonometric ratios.
Another important concept in this module is the application of trigonometric functions in real-world scenarios. Students learn how to use trigonometry to solve problems involving distances, heights, angles of elevation and depression, as well as other situations that can be modeled using right triangles. They will apply their knowledge of trigonometry to calculate distances between objects, determine the height of buildings or trees, and solve navigation problems.
Throughout Module 5, students will also explore the connections between trigonometry and other branches of mathematics, such as algebra and geometry. They will use their algebraic skills to manipulate trigonometric equations and solve for unknown variables. Additionally, they will apply geometric principles to analyze and prove trigonometric identities and properties.
This module is an important stepping stone in the study of mathematics, as it lays a solid foundation for future topics in advanced calculus, physics, and engineering. By understanding the key concepts in Secondary Math 2 Module 5, students will be equipped with the necessary skills to apply trigonometry in various real-world settings and further their understanding of the mathematical world.
Exploring Functions and Equations
Functions and equations are fundamental concepts in mathematics, particularly in the study of algebra. Functions are a way to describe how one quantity depends on another, while equations provide a way to represent relationships between variables. In the context of secondary math 2, module 5, students will delve deeper into exploring functions and equations, building upon their previous knowledge and skills.
One key aspect of exploring functions and equations is understanding the concept of a function’s domain and range. The domain of a function refers to the set of all possible input values, or x-values, while the range represents the set of all possible output values, or y-values. By examining the values within the domain and range, students can gain insights into the behavior and characteristics of a function.
Students will also explore how to analyze and represent functions graphically. Graphs provide a visual representation of the relationship between variables and can help students identify patterns and make predictions. By examining the shape, intercepts, and slopes of graphs, students can further understand the behavior of functions.
Another important concept in exploring functions and equations is solving equations. Students will learn how to solve linear equations, including those with one variable as well as systems of equations with multiple variables. This skill allows them to find the values of variables that satisfy a given equation or set of equations.
Overall, exploring functions and equations in secondary math 2 module 5 equips students with the necessary tools to analyze, interpret, and solve mathematical problems involving functions. Through a combination of algebraic manipulation, graphical analysis, and problem-solving techniques, students will develop their mathematical reasoning and critical thinking.
Solving Systems of Equations
Solving systems of equations involves finding the values of the variables that make both equations true simultaneously. This is typically done by using one of the three methods: substitution, elimination, or graphing.
In the substitution method, one equation is solved for one variable, and then that expression is substituted into the other equation. This allows us to solve for the other variable. The process repeats until the values for both variables are found. The substitution method is useful when one equation is already solved for a variable.
In the elimination method, the goal is to eliminate one variable by adding or subtracting the equations. The equations are manipulated so that when they are combined, one variable is eliminated. Then, the resulting equation with a single variable can be solved to find its value. The elimination method is useful when neither equation is already solved for a variable.
Graphing involves graphing the two equations on the coordinate plane and finding the point where they intersect. The x-coordinate and y-coordinate of this point represent the values of the variables that satisfy both equations. This method is useful when the equations are linear and easy to graph.
Each method has its advantages and disadvantages, and the choice of method depends on the specific problem and personal preference. Practicing these methods and becoming proficient in solving systems of equations can greatly improve problem-solving skills in algebra and beyond.
Investigating Quadratic Functions
A quadratic function is a type of polynomial function with a degree of 2. It can be expressed in the form f(x) = ax^2 + bx + c, where a, b, and c are constants. Investigating quadratic functions involves exploring their properties and behaviors, such as the vertex, axis of symmetry, and the shape of the graph.
One way to investigate quadratic functions is by analyzing their graphs. The graph of a quadratic function is a parabola, which is a U-shaped curve. The vertex of the parabola is a key point on the graph that can be used to find important information about the function.
To determine the vertex of a quadratic function, first find the x-coordinate by using the formula x = -b/2a. Substituting this value into the function will give you the corresponding y-coordinate. The vertex represents the minimum or maximum point on the graph, depending on whether the coefficient a is positive or negative.
Another important property of quadratic functions is the axis of symmetry. The axis of symmetry is a vertical line that divides the parabola into two symmetrical halves. It passes through the vertex of the parabola and is described by the equation x = -b/2a. This line is a useful tool for determining the symmetry and shape of the graph.
Investigating quadratic functions also involves analyzing the roots or zeros of the function. These are the x-values where the graph intersects the x-axis. The roots can be found by setting the function equal to zero and solving for x using factoring, completing the square, or the quadratic formula.
Overall, investigating quadratic functions allows us to understand their properties, graph their behavior, and solve problems related to them. By studying these functions, we can gain insights into various real-world applications, such as physics, engineering, and finance.
Secondary Math 2 Module 5 Answer Key
In the study of secondary math, module 5 focuses on various topics including exponential functions, logarithmic functions, and their applications. The answer key for module 5 provides solutions and explanations for the problems and exercises encountered throughout the module.
The answer key starts with an introduction to exponential functions, explaining the basic properties and equations involving exponential growth and decay. It then moves on to logarithmic functions, exploring their inverse relationship with exponential functions and their role in solving equations and inequalities.
The answer key also includes examples and step-by-step solutions for problems related to exponential and logarithmic functions. These examples cover a range of applications, including population growth, compounding interest, and exponential and logarithmic modeling.
Additionally, the answer key may provide tips and strategies for tackling more challenging problems and concepts within the module. It serves as a valuable resource for both students and teachers in understanding the material and assessing learning progress.
Overall, the secondary math 2 module 5 answer key is a comprehensive guide that helps students navigate and master the concepts and problems related to exponential and logarithmic functions. It provides clarity and support in the learning process, ensuring a strong foundation in these fundamental mathematical concepts.
Step-by-Step Solutions for Functions and Equations
Solving functions and equations is an essential skill in secondary math. Understanding the step-by-step process to solve these problems is crucial for success in the subject. By following a systematic approach, students can easily navigate through complex equations and functions and arrive at the correct answers.
One of the initial steps in solving functions and equations is to identify the variables and constants involved. This allows us to clearly define what values we’re looking for and what values are given in the problem. By understanding the problem’s context, we can determine the appropriate mathematical operations and rules to apply.
After identifying the variables and constants, the next step is to simplify the equation or function. This involves combining like terms, applying the order of operations, and using algebraic manipulations to rearrange the equation or function into a more manageable form. By simplifying the problem, we can eliminate unnecessary complexity and focus on the core mathematical operations required.
Once the equation or function is simplified, the next step is to solve for the unknown variable. This may involve isolating the variable on one side of the equation or using an appropriate function to find the desired value. By carefully following the rules of algebra and applying the appropriate operations, we can solve for the unknown variable and find a solution.
Finally, it is crucial to check the solution and verify its accuracy. This involves substituting the found value back into the original equation or function and ensuring that both sides of the equation or function are equal. By double-checking our work, we can confirm that our solution is correct and complete.
Overall, solving functions and equations requires a systematic approach that involves identifying variables and constants, simplifying the problem, solving for the unknown variable, and checking the solution. By following these steps and practicing regularly, students can develop a strong foundation in solving functions and equations and excel in secondary math.