Algebra 2 can be a challenging subject for many students, but with practice and the right resources, it can become more manageable. In this article, we will explore five algebra 2 exercises and provide step-by-step solutions to help you improve your understanding of the subject.
The first exercise focuses on solving quadratic equations. You will learn how to use the quadratic formula to find the roots of a quadratic equation. By following the provided step-by-step solution, you will gain insight into the process and be able to apply it to similar problems in the future.
The second exercise dives into the topic of graphing exponential functions. You will be shown how to identify the key features of an exponential function, such as the base and the y-intercept, and how to use these features to graph the function accurately. The step-by-step solution will guide you through this process, making it easier to comprehend.
Next, we will tackle an exercise on solving systems of linear equations. You will learn different methods, such as substitution and elimination, to find the values of the variables that satisfy both equations. The provided solution will demonstrate each step clearly, allowing you to apply these techniques to other systems of equations.
The fourth exercise focuses on solving logarithmic equations. You will be introduced to logarithmic properties and how to use them to solve equations involving logarithms. The step-by-step solution will guide you through the process, helping you become more comfortable with logarithmic equations.
Finally, we will explore an exercise on finding the inverse of a function. You will learn how to determine whether a function has an inverse and how to find it if it exists. The provided solution will walk you through the process, helping you deepen your understanding of inverse functions.
By working through these five algebra 2 exercises and understanding their solutions, you will be better equipped to tackle more challenging problems in algebra 2. Remember, practice is key, so keep practicing and seeking additional resources to reinforce your skills.
Understanding Algebra 2: Exercises and Answers
Algebra 2 is an advanced level of mathematics that builds upon the concepts learned in Algebra 1. It delves deeper into the study of algebraic functions, equations, and inequalities, requiring students to apply complex problem-solving skills. To develop a strong understanding of Algebra 2, it is essential to practice a variety of exercises and check the answers to assess your progress.
Exercises in Algebra 2 cover a range of topics, including polynomial functions, logarithmic functions, rational expressions, and trigonometry. These exercises may involve factorization, simplification, solving equations, and graphing functions. By practicing these exercises, students can reinforce their understanding of the concepts and enhance their problem-solving abilities.
1. Polynomial Functions: Solve problems involving polynomial functions, such as finding zeroes, determining end behavior, and graphing polynomial equations. Use the exercises to practice factoring, long division, and synthetic division to simplify polynomial expressions.
2. Logarithmic Functions: Explore logarithmic functions and their properties. Practice solving logarithmic equations, simplifying expressions using logarithmic rules, and graphing logarithmic functions.
3. Rational Expressions: Master the techniques of simplifying rational expressions, performing operations with rational expressions, and solving equations involving rational expressions. These exercises will help you understand the concepts of rational functions and their applications.
4. Trigonometry: Dive into trigonometric functions, identities, and equations. Practice solving trigonometric equations, graphing trigonometric functions, and applying trigonometric concepts to real-world problems.
By consistently practicing exercises in Algebra 2, you can strengthen your problem-solving skills, improve your understanding of complex algebraic concepts, and gain confidence in solving challenging mathematical problems. Remember to check the answers to these exercises to evaluate your progress and identify any areas that require further attention. With dedication and practice, you can master Algebra 2 and excel in your math studies.
What is Algebra 2?
Algebra 2 is a branch of mathematics that builds upon the concepts learned in Algebra 1. It is a more advanced level of algebra that delves deeper into topics such as equations, inequalities, functions, and graphs. In Algebra 2, students learn to work with more complex equations and expressions, using strategies like factoring, completing the square, and solving quadratic equations.
One of the main goals of Algebra 2 is to develop students’ problem-solving skills and critical thinking abilities. By studying algebraic concepts and applying them to real-world situations, students learn to analyze and solve problems in various contexts, such as finance, engineering, and physics. Algebra 2 also helps students develop logical reasoning and deductive thinking skills, which are valuable in many fields beyond mathematics.
Some specific topics covered in Algebra 2 include polynomial functions, exponential and logarithmic functions, matrices and determinants, conic sections, and sequences and series. These concepts and techniques form the foundation for more advanced mathematical studies, such as calculus and linear algebra.
In summary, Algebra 2 is an essential course that builds upon the foundation of Algebra 1 and prepares students for higher-level mathematics. It equips students with important problem-solving skills and mathematical reasoning abilities, making it an integral part of a well-rounded education.
The Importance of Algebra 2 Skills

Algebra 2 is a valuable branch of mathematics that builds upon the foundational concepts learned in algebra 1. It introduces students to more advanced topics and helps develop critical mathematical thinking and problem-solving skills. Having a strong understanding of algebra 2 is essential for success in various fields, including science, engineering, finance, and computer science.
One of the key reasons why algebra 2 skills are important is their practical application in real-world situations. Many complex problems in the fields of physics, chemistry, and engineering can be solved using algebraic equations and functions. Additionally, algebra 2 skills are crucial for analyzing and interpreting data, making predictions, and conducting statistical analyses. These skills are highly valued in research, data analysis, and decision-making roles.
Algebra 2 also lays the foundation for higher-level mathematics courses, such as calculus and linear algebra. These courses are required for many STEM (science, technology, engineering, and mathematics) programs in colleges and universities. Without a solid understanding of algebra 2 concepts, students may struggle to grasp the more advanced topics in these courses, hindering their academic and career prospects.
Moreover, algebra 2 helps develop logical reasoning and problem-solving abilities. Through solving equations, factoring polynomials, and graphing functions, students learn to analyze problems, break them down into smaller, manageable parts, and apply appropriate problem-solving strategies. These skills are not only important in academia but also in everyday life, as they enable individuals to make informed decisions, solve practical problems, and think critically about complex issues.
In conclusion, algebra 2 skills are essential for success in various academic and professional pursuits. They provide a solid mathematical foundation, improve problem-solving abilities, and have practical applications in numerous fields. By mastering the concepts and skills taught in algebra 2, students can enhance their academic performance, broaden their career opportunities, and develop valuable skills that can be applied in various aspects of their lives.
Types of Exercises in Algebra 2

In Algebra 2, students encounter various types of exercises that help them develop their understanding of mathematical concepts and problem-solving skills. These exercises cover a wide range of topics, including equations, inequalities, functions, and graphs.
1. Solving Equations: One common type of exercise in Algebra 2 is solving equations. Students are given an equation and are required to find the value(s) of the variable that satisfy the equation. These exercises often involve linear equations, quadratic equations, or systems of equations. Students learn different techniques, such as factoring, completing the square, or using the quadratic formula, to solve these equations.
2. Graphing: Another type of exercise in Algebra 2 involves graphing equations and functions. Students learn how to plot points, draw lines, and graph various types of functions, including linear, quadratic, exponential, and logarithmic functions. They also learn about symmetry, transformations, and the behavior of functions as x approaches positive or negative infinity.
3. Solving Inequalities: Inequalities are another important concept in Algebra 2, and students practice solving inequalities through various exercises. They learn how to graph inequalities on a number line and determine the solution set. They also solve systems of inequalities and explore the concept of interval notation to represent the solution.
4. Operations with Functions: Algebra 2 includes exercises that involve operations with functions. Students learn how to add, subtract, multiply, and divide functions, as well as compose and find the inverse of functions. These exercises help them gain a deeper understanding of the properties of functions and how they can be manipulated.
5. Word Problems: Word problems are a crucial component of Algebra 2 exercises as they help students apply their algebraic skills to real-world situations. These problems require students to translate words into algebraic expressions or equations and then solve them to find the solution. They cover a wide range of topics, including rate problems, mixture problems, and distance-time problems.
Overall, the exercises in Algebra 2 aim to reinforce the fundamental concepts of algebra, enhance problem-solving abilities, and prepare students for more advanced mathematical concepts in higher-level courses.
Equations and Inequalities
Equations and inequalities are essential concepts in algebra, as they allow us to solve problems and find unknown values. An equation is a mathematical statement that asserts the equality of two expressions, while an inequality compares two expressions using the symbols < (less than), > (greater than), ≤ (less than or equal to), or ≥ (greater than or equal to).
Solving equations involves finding the value of the variable that makes the equation true. This is done by performing operations on both sides of the equation to isolate the variable. Commonly used operations include addition, subtraction, multiplication, and division. For example, in the equation 2x + 5 = 13, we can subtract 5 from both sides to get 2x = 8, and then divide both sides by 2 to find that x = 4.
Inequalities, on the other hand, represent a range of possible values for the variable. Solving inequalities involves finding the set of values that make the inequality true. Similar to equations, we can use various operations to transform the inequality and determine its solution. For instance, in the inequality 3x – 7 ≤ 5, we can add 7 to both sides to get 3x ≤ 12, and then divide both sides by 3 to find that x ≤ 4.
Equations and inequalities are used in various fields such as physics, engineering, economics, and many other areas of science and mathematics. Understanding how to solve equations and inequalities allows us to model real-world situations, make predictions, and solve problems efficiently.
Here are some examples of exercises on equations and inequalities in algebra 2:
- Solve the equation 2(x + 3) = 10 for x
- Solve the inequality 4x – 5 > 3x + 2 for x
- Find the solutions to the equation x^2 – 9 = 0
- Determine the range of values for x in the inequality 2x + 1 ≥ 7
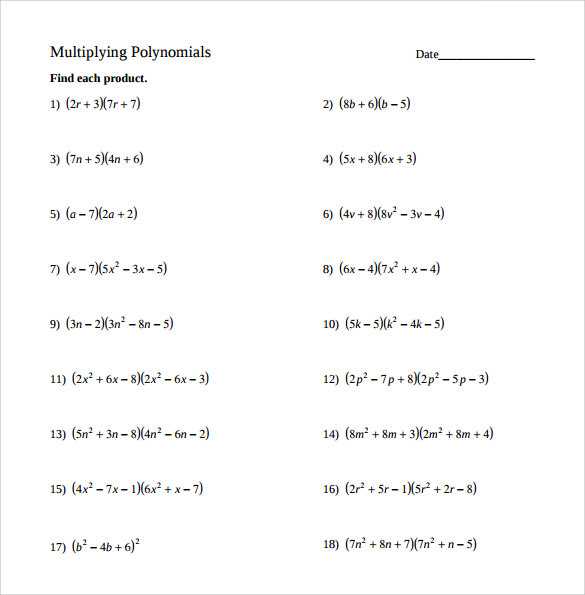
Polynomials and Factoring
A polynomial is a mathematical expression that consists of variables, coefficients, and exponents. It can be divided into terms, which are separated by addition or subtraction signs. The highest power of the variable in a polynomial determines its degree.
Factoring is the process of breaking down a polynomial into its constituent parts. By factoring a polynomial, we can write it as a product of its factors. Factoring polynomials is crucial in algebra as it helps us solve equations, simplify expressions, and analyze the behavior of functions.
Methods of Factoring:
- Common Factors: When a polynomial has a common factor, we can factor it out by dividing each term by the common factor.
- Factoring by Grouping: In some polynomials, we can group terms with common factors and then factor out the common factors from each group.
- Factoring by Reverse FOIL: This method involves finding two numbers that multiply to give the constant term and add up to give the coefficient of the linear term, which are then used to factor the polynomial.
- Factoring Quadratic Trinomials: Quadratic trinomials can be factored using methods such as the product-sum method, square root method, or by applying the quadratic formula.
- Factoring Special Forms: Certain polynomials have special forms that can be factored easily, such as the difference of squares and perfect square trinomials.
Applications of Factoring:
Factoring polynomials have various applications in real-life situations and mathematical problems. Some common applications include:
- Solving quadratic equations and finding the roots of a polynomial.
- Simplifying algebraic expressions and reducing fractions to lowest terms.
- Finding the x-intercepts and y-intercepts of a polynomial function.
- Graphing polynomial functions and determining their behavior.
- Solving word problems involving algebraic expressions and equations.
In conclusion, understanding polynomials and factoring is essential in algebraic problem-solving. By mastering factoring techniques, we can manipulate and simplify polynomials to analyze their properties and solve various types of equations and problems.