
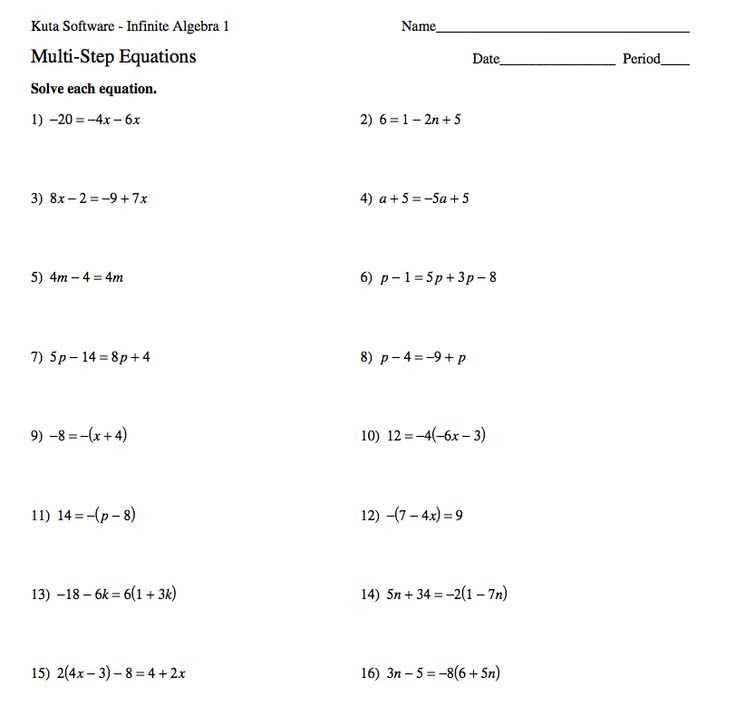
In Lesson 5, we will be practicing solving two-step equations. Two-step equations are algebraic equations that require two operations to solve for the unknown variable. By following a few simple steps, we can find the solution to these equations.
The answer key for this skills practice will provide step-by-step solutions to the various two-step equations. It will help you understand the process and allow you to check your work. Remember, practice makes perfect, so don’t hesitate to keep working on these equations until you feel confident in your abilities.
By reviewing the answer key, you will also be able to identify any mistakes you may have made and learn from them. Mistakes are a natural part of the learning process, and recognizing and correcting them is essential for improvement.
So grab your pencil and paper and get ready to dive into Lesson 5 Skills Practice More Two-Step Equations. With a bit of practice and perseverance, you’ll be solving these equations like a pro in no time!
Lesson 5 Skills Practice: More Two-Step Equations Answer Key
In this lesson, we will review the key concepts and skills related to solving two-step equations. By the end of this practice, you should have a clear understanding of how to solve these types of equations and find the solution.
To solve a two-step equation, we follow a specific set of steps. First, we isolate the variable by performing the opposite operation of what is being done to the variable. Then, we simplify both sides of the equation by combining like terms. Finally, we solve for the variable by performing the necessary operations to find its value. It is important to remember that whatever operation we do to one side of the equation, we must do to the other side as well in order to maintain equality.
For example, let’s consider the equation 3x + 2 = 8. To isolate the variable, we need to subtract 2 from both sides of the equation. This gives us 3x = 6. Next, we divide both sides of the equation by 3 to solve for x. The simplified equation becomes x = 2. Therefore, the solution to this equation is x = 2.
Throughout this lesson’s practice problems, you will encounter a variety of two-step equations. Remember to carefully follow the steps outlined above in order to solve each equation and find the correct answer. Pay attention to signs, coefficients, and order of operations to avoid any mistakes. With practice, you will become more confident in solving two-step equations and be able to tackle more complex problems in the future.
Below is the answer key to the practice problems in this lesson. Refer to this key to check your solutions and understand any mistakes you may have made. Use this as an opportunity to learn from your errors and improve your problem-solving skills.
Answer Key:
- x = 5
- x = -2
- x = 4
- x = 3
- x = -1
Continue practicing and reviewing two-step equations to solidify your understanding of this important algebraic concept. With time and effort, you will become proficient in solving equations and be well-prepared for more advanced math topics.
Overview
In Lesson 5, we will continue to practice solving two-step equations. This skill is essential in algebra and is necessary for solving more advanced equations in the future. We will focus on equations that involve addition, subtraction, multiplication, and division.
The lesson begins with a review of the basic concepts of two-step equations. We will discuss the importance of isolating the variable and using inverse operations to undo the operations applied to the variable. This will help us find the value of the variable that makes the equation true.
We will then move on to more complex equations that require multiple steps to solve. We will explore examples of equations with variables on both sides and equations with fractions or decimals. We will learn how to simplify expressions, combine like terms, and apply the distributive property to solve these equations.
Throughout the lesson, we will practice solving a variety of two-step equations through guided examples and interactive exercises. These exercises will help reinforce the concepts and techniques we have learned, and provide an opportunity for you to apply your knowledge in different scenarios.
By the end of this lesson, you will have a solid understanding of solving two-step equations and be able to confidently tackle more complex equations in the future. These skills will be valuable not only in algebra, but also in other areas of math and problem-solving.
Understanding Two-Step Equations
A two-step equation is a mathematical equation that requires two separate steps to solve for the variable. These equations involve performing two different mathematical operations in order to isolate the variable on one side of the equation. By following the appropriate steps, you can find the value of the variable and solve the equation.
One common type of two-step equation involves addition or subtraction followed by multiplication or division. It is important to remember that when solving these equations, you must perform the operations in the correct order and maintain equality on both sides of the equation throughout the process.
To solve a two-step equation, start by isolating the variable term by undoing any addition or subtraction. If the variable has a term being added or subtracted, you must perform the opposite operation to both sides of the equation. This will cancel out the addition or subtraction and leave the variable term alone on one side of the equation.
After isolating the variable term, the next step is to undo any multiplication or division. If the variable has a term being multiplied or divided, you must perform the opposite operation to both sides of the equation. This will cancel out the multiplication or division and leave the variable alone on one side of the equation, which will allow you to determine its value.
Understanding and correctly solving two-step equations is an essential skill in mathematics. It allows you to find the value of unknown variables and solve real-world problems that involve multiple mathematical operations. By following the correct steps and maintaining equality, you can confidently solve two-step equations and gain a deeper understanding of mathematical concepts.
Solving Two-Step Equations
Two-step equations are mathematical expressions that require two separate operations to solve. They involve both addition/subtraction and multiplication/division, and solving them requires following a specific sequence of steps.
To solve a two-step equation, the first step is to isolate the variable term by undoing any addition or subtraction operations. This is done by performing the opposite operation on both sides of the equation. For example, if the equation has addition, subtract the same value from both sides. If it has subtraction, add the same value to both sides.
After isolating the variable, the second step is to undo any multiplication or division operations by performing the opposite operation again. If the equation has multiplication, divide both sides of the equation by the same value. If it has division, multiply both sides by the same value.
It is important to remember that the goal is to get the variable term by itself on one side of the equation and find its value. The equal sign serves as a balance, and whatever actions are performed on one side must also be performed on the other side to maintain balance.
By following these steps, it is possible to solve two-step equations and find the value of the variable. Practicing these skills will not only strengthen mathematical abilities but also build problem-solving skills that can be applied to a variety of real-life situations.
Practice Problems
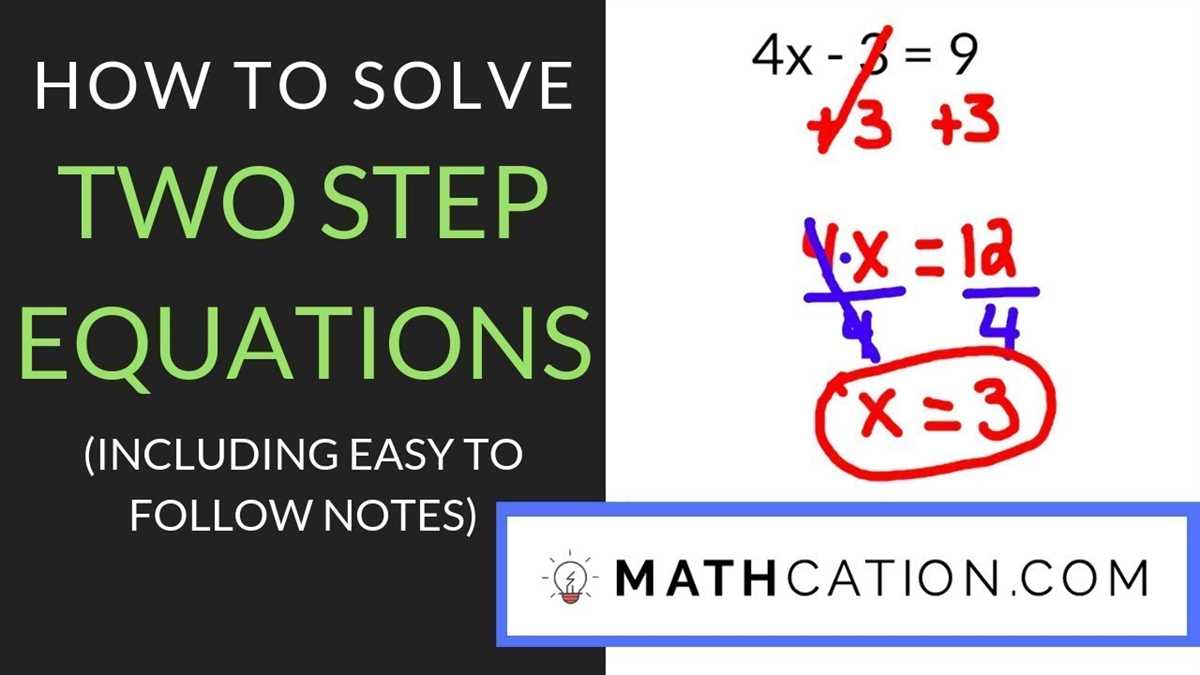
In this lesson on two-step equations, we will review some practice problems to reinforce your understanding of the concept. By solving these problems, you will gain confidence in solving equations that involve both addition/subtraction and multiplication/division.
Problem 1: Solve the equation 3x + 5 = 17.
To solve this equation, we need to isolate the variable x. We can do this by performing inverse operations. To eliminate the addition of 5, we subtract 5 from both sides of the equation:
- 3x + 5 – 5 = 17 – 5
- 3x = 12
Next, we need to eliminate the multiplication of 3. To do this, we divide both sides of the equation by 3:
- (3x)/3 = 12/3
- x = 4
Therefore, the solution to the equation 3x + 5 = 17 is x = 4.
Problem 2: Solve the equation 2(x – 3) = 10.
To solve this equation, we need to distribute the 2 to both terms inside the parentheses:
- 2*x – 2*3 = 10
- 2x – 6 = 10
Next, we isolate the variable x by performing inverse operations. Addition of 6 is the inverse of subtraction of 6, so we add 6 to both sides of the equation:
- 2x – 6 + 6 = 10 + 6
- 2x = 16
Finally, we eliminate the multiplication of 2 by dividing both sides of the equation by 2:
- (2x)/2 = 16/2
- x = 8
Therefore, the solution to the equation 2(x – 3) = 10 is x = 8.
By practicing more two-step equations, you will become more proficient in solving them. Remember to apply the same steps of isolating the variable by performing inverse operations. Keep practicing to improve your skills!
Answer Key for Practice Problems

Here is the answer key for the practice problems on two-step equations:
- Problem 1: x = 4
- Problem 2: x = -1
- Problem 3: x = 6
- Problem 4: x = 9
- Problem 5: x = -5
Remember, when solving two-step equations, it’s important to follow the order of operations and isolate the variable by performing inverse operations.
Practice makes perfect! Keep practicing solving two-step equations to improve your skills. Good luck!