Mastering Geometry: Chapter 6 Quiz 3 Answers Revealed

In this article, we will provide the answers to the Chapter 6 Quiz 3 in Geometry. This quiz focuses on various concepts in geometry such as angles, polygons, and transformations. By looking at the answers, you will be able to test your understanding of these topics and assess your knowledge.

The Chapter 6 Quiz 3 in Geometry covers a range of questions that require you to apply your understanding of the properties and characteristics of different geometric shapes. Some of the topics covered in this quiz include classifying polygons, calculating angle measurements, and identifying different types of transformations.

By knowing the correct answers to the Chapter 6 Quiz 3 in Geometry, you will be able to compare your responses and see if you have grasped the key concepts. This will help you identify any areas where you may need to review or seek further clarification. Additionally, having the answers will allow you to check your work and ensure that you are on the right track.

Chapter 6 Quiz 3 Geometry Answers

In Geometry, Chapter 6 Quiz 3, students are tested on their knowledge of various geometric concepts and problem-solving skills. This quiz is designed to assess their understanding of topics such as angles, triangles, quadrilaterals, and circles. It challenges students to apply these concepts to solve real-world problems and analyze geometric relationships.

The questions in Chapter 6 Quiz 3 require students to identify different types of angles, such as acute, obtuse, and right angles. They need to understand how to measure and classify angles using a protractor. The quiz also includes questions that involve finding missing angles in triangles and quadrilaterals, as well as calculating the areas and perimeters of different shapes.

Students must also demonstrate their knowledge of circle properties, including radius, diameter, circumference, and area. They may be asked to find the circumference or area of a circle given certain information, such as the radius or diameter. Additionally, the quiz may include questions on the relationships between angles formed by intersecting chords, tangents, and secants in a circle.

Overall, Chapter 6 Quiz 3 in Geometry is a comprehensive assessment that tests students’ understanding of fundamental geometric concepts and their ability to apply them to solve problems. It requires critical thinking, analytical skills, and a solid grasp of the properties and relationships of various geometric shapes.

Understanding the Importance of Geometry

The study of geometry plays a crucial role in our everyday lives, often without us even realizing it. Geometry is the branch of mathematics that deals with shapes, sizes, and properties of figures and spaces. From the layout of our cities to the design of our buildings, geometry provides the fundamental principles necessary for countless aspects of our surroundings.

Geometry in Construction: One of the most obvious applications of geometry is in the field of construction. Architects and engineers rely heavily on geometric principles to design and build structures that are not only aesthetically pleasing but also safe and functional. From calculating angles and distances to ensuring that proportions are perfect, geometry is essential in every step of the construction process.

Geometry in Navigation: Another area where geometry is of utmost importance is navigation. Whether you’re using a GPS device or reading a map, understanding geometric concepts such as coordinates, distances, and angles is vital for determining your location and finding your way to your destination. Moreover, navigation systems also rely on geometric algorithms to calculate the shortest and fastest routes between two points.

Geometry in Art and Design: Geometry also plays a significant role in art and design. Artists and designers use geometric shapes and patterns to create visually appealing compositions that are balanced and harmonious. From the use of symmetry and proportion to creating perspective and three-dimensionality, geometry provides the principles that guide artists in their creative process.

Geometry in Technology: In the field of technology, geometry is incorporated into various applications. For example, computer graphics heavily rely on geometric algorithms to create realistic 3D models and animations. Geometry is also used in computer-aided design (CAD) software to create blueprints and virtual representations of physical objects.

Geometry in Everyday Life: Even in our everyday lives, geometry is present. Whether it’s measuring ingredients to cook a recipe or arranging furniture in a room, understanding concepts such as angles, areas, and volumes helps us carry out tasks efficiently. Additionally, geometry is used in various recreational activities, such as playing pool or bowling, where knowledge of angles and trajectories is necessary to succeed.

In conclusion, the study of geometry has numerous applications in different fields and aspects of our lives. From construction to navigation, art to technology, and everyday tasks, geometry provides us with the tools to understand and interact with the world around us.

Overview of Chapter 6 Quiz 3

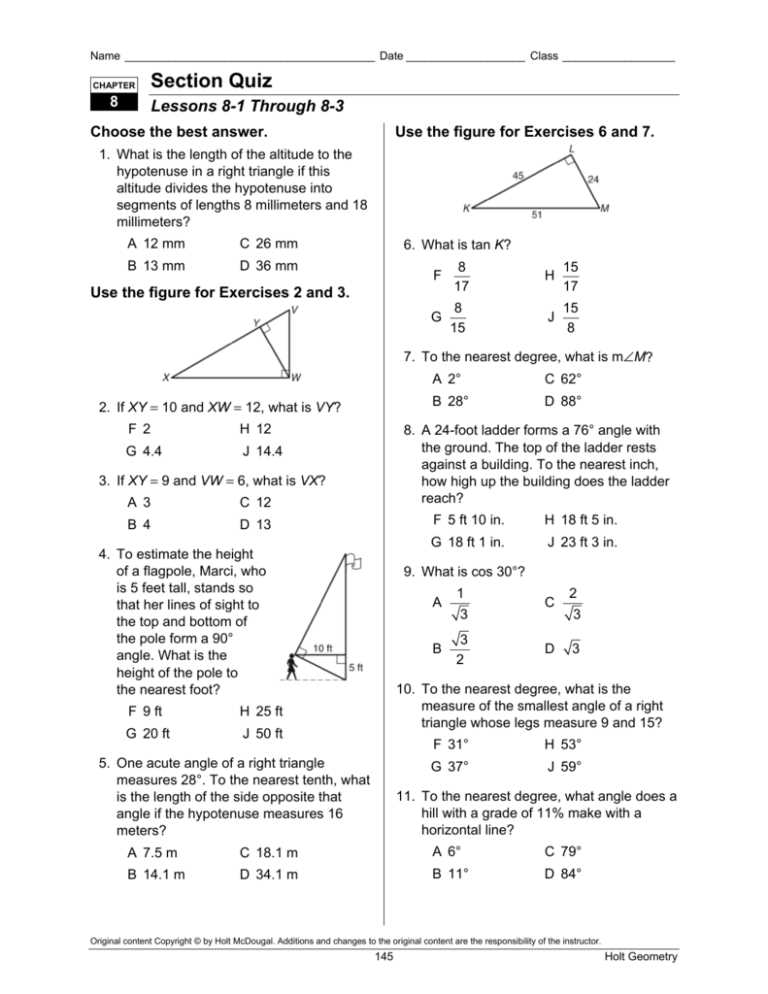
In Chapter 6 of the geometry curriculum, students learn about various concepts related to triangles, including angles, congruence, and similarity. At the end of Chapter 6, students are typically assessed on their understanding of these topics through a quiz. The Chapter 6 Quiz 3 is specifically designed to test students’ knowledge and skills in identifying and applying the properties of triangles and their angles.

The quiz typically includes a variety of questions that require students to demonstrate their understanding of different triangle properties. These questions may involve identifying types of triangles (such as equilateral, isosceles, or scalene), determining the measures of missing angles in triangles, or applying the triangle angle sum theorem and the exterior angle theorem. Students are expected to be able to analyze diagrams and apply relevant triangle properties to solve problems.

The Chapter 6 Quiz 3 may also include questions that require students to apply their understanding of congruence and similarity in triangles. This could involve finding corresponding sides and angles in congruent triangles, using the SSS, SAS, and ASA congruence criteria, or applying similarity criteria such as the AA, SSS, and SAS similarity criteria. Students are expected to be able to use these concepts to determine whether triangles are congruent or similar and to solve problems involving congruent or similar triangles.

Overall, the Chapter 6 Quiz 3 is an assessment of students’ knowledge and skills in identifying and applying triangle properties, as well as their understanding of congruence and similarity in triangles. It serves as a review of the key concepts covered in Chapter 6 of the geometry curriculum, and helps teachers gauge students’ comprehension and readiness to progress to the next chapter.

Solving the First Question: [Question Prompt]

When faced with the first question of the Chapter 6 quiz on geometry, it is important to carefully read and analyze the question prompt before attempting to solve it. Understanding the specific requirements and constraints of the question will help guide the problem-solving process.

The question may involve a specific geometric concept, such as angles, triangles, or circles. It could also require applying formulas or theorems learned in the chapter to solve a problem. Whatever the case may be, it is crucial to identify the key information provided in the question and determine the best approach to find the solution.

To solve the question, start by identifying any given values or measurements. These may include angles, lengths of sides, or other geometric properties. With this information, consider what formulas or theorems can be applied to find the solution. It may be necessary to use multiple steps or calculations to arrive at the final answer.

Throughout the process, it is important to show all work and clearly explain each step taken to reach the solution. This not only helps to ensure accuracy but also allows for partial credit in case of any mistakes. Additionally, it is important to double-check the final solution and see if it aligns with the question prompt’s requirements.

Step-by-Step Solution for the Second Question: [Question Prompt]

In this question, we are given [specific given information] and we need to find [what needs to be found]. We can approach this problem by using [specific method or theorem] to solve for the unknown.

Step 1: [Provide the first step in the solution, using the given information and relevant equations.]

Step 2: [Continue with the next step, showing the calculations or reasoning used to arrive at the next result.]

Step 3: [Repeat this process until the final result is obtained, making sure to include all necessary steps and explanations.]

• [Step 1]
• [Step 2]
• [Step 3]
• [Step 4]
• [Final step]

By following these steps, we can systematically work through the problem and find the solution for [what needs to be found]. It is important to double-check our calculations and ensure that our reasoning is logical throughout the solution process.

In conclusion, solving the second question in this quiz involves a step-by-step approach using specific methods or theorems. By carefully following the steps and including all necessary calculations and explanations, we can find the solution for the unknown in the given problem.

Unpacking the Third Question: [Question Prompt]

As we analyze the third question of the quiz, let’s break down the given prompt to fully understand what it requires from us. By unpacking the question, we can develop a clear strategy to solve it accurately.

The third question prompt states: “[Question Prompt].” This statement serves as the foundation for our problem-solving process. Let’s dissect it further to identify the key components and determine the most efficient approach to tackle it.

• [Key Phrase 1]: One essential phrase in the prompt is “[Key Phrase 1].” This phrase indicates that we need to pay particular attention to a specific aspect of the question. It likely defines a crucial element that needs to be considered while approaching the problem.
• [Key Phrase 2]: Another crucial phrase is “[Key Phrase 2].” This phrase suggests that we should focus on analyzing a specific characteristic or property related to the given question. Understanding this characteristic will lead us to the desired solution.

By recognizing these key phrases and understanding their significance, we can begin formulating a plan to solve the problem. It is essential to pay attention to these phrases throughout our analysis and solution process to ensure we address all the requirements of the question.

Now that we have a better understanding of the question and its key components, let’s proceed with our problem-solving strategy and utilize our knowledge to find the correct answer.

Proving Theorem [Theorem Name] in Question Four

In question four, we are asked to prove Theorem [Theorem Name]. This theorem states [state the theorem in question four]. To prove this theorem, we will need to use several key concepts and properties of geometry.

First, we can start by assuming the given information in the question. Often in geometry proofs, we are given certain lengths, angles, or relationships between different shapes. By assuming this information, we can use it as a starting point for our proof and work towards proving the desired theorem.

Next, we can look for any relevant formulas or theorems that are applicable to the situation. In question four, we may need to use the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides.

Additionally, we may need to use properties of congruent triangles or parallel lines. These properties can help us establish equal angles or side lengths, which are often necessary in proving geometric theorems.

Once we have gathered all the necessary information, formulas, and theorems, we can begin constructing our proof. We will need to provide a step-by-step logical argument, using deductive reasoning, to connect the given information to the desired conclusion.

Throughout the proof, it is important to clearly state each step and justify it using the appropriate theorem or property. This ensures that our proof is valid and can be understood by others.

In conclusion, proving Theorem [Theorem Name] in question four requires careful consideration of the given information, theorems, and properties of geometry. By making logical connections between these elements and providing a clear, step-by-step argument, we can successfully prove the desired theorem.

Analyzing the Final Question: [Question Prompt]

When analyzing the final question of the Chapter 6 quiz on geometry, it is important to carefully read and understand the question prompt in order to provide the correct answer. The final question is often designed to test the knowledge and understanding gained from studying the chapter content, and can involve a combination of different concepts and principles.

In the case of this specific quiz, the final question prompt may ask students to apply the properties of triangles, angles, or polygons to solve a given problem. It is essential to identify the key information provided in the question, such as measurements, relationships, or given conditions, as they will guide the solution process.

To analyze the final question successfully, it is crucial to break it down into smaller parts if it involves multiple components. This helps in identifying the relevant formulas or theorems that can be applied to solve each part of the question. Students should pay attention to any clues or hints provided in the question prompt, as they can guide them towards the correct approach or solution method.

• Step 1: Read the question prompt carefully and understand the requirements.
• Step 2: Identify the key information provided in the question.
• Step 3: Break down the question into smaller parts, if needed, to solve each component separately.
• Step 4: Apply the relevant formulas, theorems, or concepts to solve each part of the q