
In mathematics, linear inequalities are expressions that compare two linear equations using the symbols <, >, ≤, or ≥. These inequalities help us understand relationships between different variables and find solutions that satisfy certain conditions. In the practice exercise “6 5 Practice Linear Inequalities Form G Answers,” we explore the process of solving linear inequalities with example problems to sharpen our skills.
The practice exercise provides a series of linear inequalities questions in the form of equations, where we need to determine the range or values that satisfy the given conditions. By understanding the properties of inequalities and applying the correct operations, we can find the correct solutions and answers to each problem.
This exercise also helps to reinforce our understanding of graphing linear inequalities on a coordinate plane. By plotting the corresponding linear equation, shading the appropriate side of the line, and considering the inequality symbol, we can visually determine the range of solutions. With “6 5 Practice Linear Inequalities Form G Answers,” we can practice these concepts and build confidence in solving linear inequalities.
Understanding Linear Inequalities: Solution Guide
In mathematics, linear inequalities play a crucial role in understanding and solving various problems, from algebraic equations to real-world applications. A linear inequality is an inequality in which the highest power of the variable is 1. It can be represented graphically on a coordinate plane as a shaded region bounded by a straight line. Solving linear inequalities involves finding the values of the variable that satisfy the given inequality.
To solve linear inequalities, it is important to follow a systematic approach. First, identify the inequality symbol (>, <, ≥, or ≤) and the variable that needs to be solved. Next, isolate the variable on one side of the inequality sign. This can be done by performing operations such as addition, subtraction, multiplication, or division on both sides of the inequality. However, it is important to note that when multiplying or dividing by a negative number, the direction of the inequality symbol should be reversed.
After isolating the variable, the solution to the inequality can be determined by graphing the corresponding linear equation on a coordinate plane and shading the appropriate region. The solution can also be expressed as an interval notation or as a set of ordered pairs. For example, if the solution to the inequality is x > 3, it can be represented as (3, ∞) or x , where ∞ represents infinity.
It is important to always check the solution to the linear inequality by substituting the values back into the original inequality. This ensures that the solution is valid and satisfies the given inequality. In cases where the inequality involves absolute values, the solution may need to be considered in two separate cases, depending on whether the expression inside the absolute value is positive or negative.
Practice and understanding of linear inequalities are essential in various fields such as economics, engineering, and physics. They provide a mathematical tool for solving optimization problems, analyzing constraints, and making informed decisions. By following a systematic approach and understanding the concepts behind linear inequalities, one can confidently tackle complex problems and arrive at accurate solutions.
How to Solve Linear Inequalities: Step-by-Step Explanation
In mathematics, linear inequalities are equations that involve a variable and one or more constants, along with comparison symbols such as greater than or less than. Solving linear inequalities involves finding the values of the variable that satisfy the inequality.
The following are step-by-step instructions for solving linear inequalities:
- Step 1: Identify the inequality sign and the variables involved. For example, if the inequality is 2x + 3 > 5, the inequality sign is > and the variable is x.
- Step 2: Simplify the inequality by performing any necessary operations. This may involve combining like terms and/or moving terms to one side of the inequality symbol. In the example 2x + 3 > 5, subtracting 3 from both sides gives 2x > 2.
- Step 3: Divide both sides of the inequality by the coefficient of the variable. In the example 2x > 2, dividing by 2 gives x > 1.
- Step 4: Graph the solution on a number line. For the inequality x > 1, plot an open circle at 1 and shade the region to the right.
- Step 5: Write the solution in interval notation, if necessary. In the example x > 1, the solution can be written as (1, ∞) to represent all values of x greater than 1.
These steps can be applied to any linear inequality. By following this process, you can accurately solve linear inequalities and find the values that satisfy the given conditions.
Practice Makes Perfect: 5 Exercises to Solve Linear Inequalities
Linear inequalities are mathematical expressions that compare two variables and determine a range of possible values that satisfy the inequality. Solving linear inequalities is an important skill in algebra and is often used in various real-life scenarios, such as budgeting, optimization problems, and decision-making.
To master the art of solving linear inequalities, it is crucial to practice. Here are five exercises that can help you sharpen your skills:
- Solve for x: Solve the inequality 2x + 3 > 7 for x. Start by isolating x on one side of the inequality, then divide by the coefficient of x to find the solution.
- Graph the inequality: Graph the inequality y ≤ -3x + 2 on a coordinate plane. Use a solid line for the inequality symbol ≤, and shade the region below the line to represent the solutions.
- Write the solution: Write the solution set for the inequality 4x + 5 < 3x - 2. Start by subtracting 3x from both sides, then isolate x on one side. Finally, express the solution set using interval notation or set builder notation.
- Solve a compound inequality: Solve the compound inequality -1 < 2x + 3 < 5. Break down the compound inequality into two separate inequalities, solve each one individually, and then combine the solutions to find the final solution set.
- Word problem: Solve the following word problem using linear inequalities: “Sam has at most $100 to spend on groceries. The price of each item cannot exceed $20. Write an inequality to represent this situation and find the maximum number of items Sam can purchase.”
Remember, practice is key when it comes to mastering the skills required to solve linear inequalities. By consistently working through exercises like these, you will build your confidence and proficiency in handling these mathematical expressions.
Checking Your Answers: Common Mistakes in Solving Linear Inequalities
In solving linear inequalities, it is important to check our answers to ensure accuracy. There are several common mistakes that can occur during the solving process, which may lead to incorrect solutions. By being aware of these mistakes, we can avoid them and improve the reliability of our answers.
1. Failing to reverse the inequality symbol when multiplying or dividing by a negative number: When multiplying or dividing both sides of an inequality by a negative number, it is necessary to reverse the inequality symbol. This is because multiplying or dividing by a negative number changes the direction of the inequality. For example, if we have the inequality 3x < 6 and we divide both sides by -3, the correct solution is x > -2. However, if we forget to reverse the symbol, we may mistakenly write x < -2 as the solution.
2. Forgetting to distribute the negative sign: When distributing a negative sign to terms within parentheses, it is important to remember that the sign applies to each term individually. For example, if we have the inequality -2(x – 4) > 8 and we forget to distribute the negative sign, we may mistakenly write -2x – 4 > 8 as the resulting inequality.
3. Neglecting to isolate the variable: In order to solve a linear inequality, we need to isolate the variable on one side of the inequality symbol. This involves performing the necessary operations on both sides of the inequality to move all the variable terms to one side. For example, if we have the inequality 4x – 5 > 3x + 2 and we forget to subtract 3x from both sides, we may mistakenly solve the inequality as x – 5 > 2.
To avoid these common mistakes, it is important to double-check our work and carefully follow the steps in solving linear inequalities. By being mindful of these potential errors, we can achieve accurate solutions and improve our understanding of linear inequalities.
Form G Answers: Solutions to the Linear Inequalities Practice Problems
Linear inequalities are equations that involve inequalities (>, <, ≥, ≤) and linear expressions. Solving these inequalities involves finding the values of the variable that make the inequality true. Form G provides practice problems to help students understand and master the concept of solving linear inequalities.
In Form G, there are various types of linear inequalities such as “less than”, “greater than”, “less than or equal to”, and “greater than or equal to”. Students are required to solve these inequalities and represent the solution on a number line or using interval notation.
When solving linear inequalities, it is essential to perform operations on both sides of the inequality sign in order to isolate the variable. The goal is to find the range of values that satisfy the inequality and represent it graphically. Students can also check their solutions by substituting the values back into the inequality and verifying if it holds true.
The Form G answers provide step-by-step solutions to the practice problems, allowing students to understand the process and learn from their mistakes. It is important for students to carefully review the answers and understand the reasoning behind each step in order to improve their problem-solving skills.
Overall, practicing linear inequalities using Form G helps students develop a strong foundation in solving and understanding linear inequalities. By mastering this concept, students can apply it to real-world situations where inequalities are commonly used to represent constraints or conditions.
Mastering Linear Inequalities: Tips and Tricks for Success

Linear inequalities are an important topic in mathematics, and mastering them is crucial for success in various areas such as algebra, calculus, and real-world applications. However, understanding and solving linear inequalities can be challenging for many students. In this article, we have provided some valuable tips and tricks to help you improve your skills in dealing with linear inequalities.
1. Understand the Basics
Before diving into more complex problems, make sure you have a strong understanding of the basics of linear inequalities. Familiarize yourself with the symbols used in representing inequalities (such as ‘<' and '>‘), as well as the concepts of inequality and inequality signs. This foundation will help you build confidence and tackle more advanced problems.
2. Graphical Representation
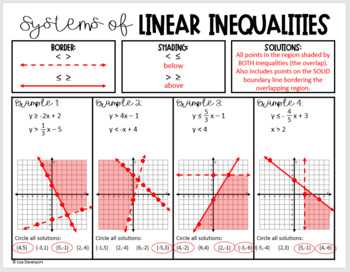
Visualizing linear inequalities on a graph can often make them easier to understand and solve. Plotting the inequalities on a coordinate plane can give you a clear picture of their solution sets. Practice sketching graphs for different types of inequalities, such as linear equations and quadratic inequalities, to strengthen your graphical understanding.
3. Know the Properties
Understanding the properties of linear inequalities will help you manipulate and solve them more efficiently. Familiarize yourself with properties such as multiplication/division, addition/subtraction, and combining like terms. These properties will allow you to simplify and transform inequalities, making them easier to solve.
4. Use Algebraic Techniques
Mastering algebraic techniques is essential for solving linear inequalities. Use techniques such as adding or subtracting the same value on both sides, multiplying or dividing by a positive or negative number, and isolating the variable. By applying these techniques correctly, you can transform complex inequalities into simpler forms and find their solutions more effectively.
5. Practice, Practice, Practice
As with any mathematical concept, consistent practice is the key to mastering linear inequalities. Solve a variety of problems with different levels of difficulty to enhance your skills. Work on exercises from textbooks, online resources, or seek guidance from your teacher or tutor. The more you practice, the more familiar you will become with the different types of inequalities and their solution methods.
By following these tips and tricks, you can improve your understanding and proficiency in solving linear inequalities. Remember, mastering linear inequalities takes time and effort, but with consistent practice and a solid understanding of the concepts, you can become a pro in no time!