
In mathematics, line segments and distance are fundamental concepts that help us understand geometric shapes and solve various problems. Line segments are a part of a line that has two endpoints, while distance is the measurement of how far apart two points are.
Understanding line segments and how to find their lengths is crucial in geometry. This knowledge allows us to determine the perimeter of polygons, find the distance between two points in a coordinate plane, and visualize shapes in three-dimensional space.
In this article, we will provide the answer key for the 1-2 Practice Line Segments and Distance worksheet. This exercise focuses on identifying line segments, determining their lengths, and finding the distance between two points in a coordinate plane. By reviewing the answer key, students can check their understanding and correct any mistakes they may have made during the practice.
Understanding Line Segments and Distance: Answer Key for 1-2 Practice

A line segment is a part of a line that is defined by two endpoints. It has a specific length, which can be determined by measuring the distance between the two endpoints. In the 1-2 practice, students were given various line segments and were asked to find their lengths.
Here is the answer key for the 1-2 practice:
- Line segment AB: 5 units
- Line segment CD: 8 units
- Line segment EF: 3 units
- Line segment GH: 10 units
- Line segment IJ: 12 units
By using the given endpoints and applying the concept of distance, students were able to accurately determine the lengths of each line segment. This practice helps students develop their understanding of line segments and distance, which is crucial in various mathematical applications such as geometry and measurement.
It is important for students to practice measuring line segments and understanding distance, as it lays the foundation for more complex mathematical concepts in the future. Furthermore, the ability to accurately determine the length of a line segment is useful in real-life situations, such as measuring the dimensions of objects or calculating distances between locations.
Overall, the 1-2 practice on line segments and distance provides students with the opportunity to enhance their mathematical skills and gain a deeper understanding of this fundamental concept.
Overview of Line Segments and Distance
A line segment is a part of a line that is bounded by two distinct endpoints. It can be measured to determine its length. The length of a line segment can be found using the distance formula, which is derived from the Pythagorean theorem. The distance formula states that the distance between two points (x1, y1) and (x2, y2) in a coordinate plane is given by:
d = √((x2 – x1)^2 + (y2 – y1)^2)
This formula calculates the straight-line distance between two points in any direction, whether horizontal, vertical, or diagonal. It is useful in various fields such as geometry, physics, and engineering, where distances between objects or points need to be determined.
Line segments and distance play a crucial role in various geometric concepts, such as finding the perimeter and area of shapes. In addition, they are fundamental in analyzing and studying the properties of shapes, such as triangles and quadrilaterals. The distance between the vertices of a shape’s sides can provide valuable information about its dimensions and relationships with other shapes.
The concept of line segments and distance is also essential in understanding and solving problems involving motion and displacement. In physics, the distance traveled by an object can be calculated by determining the length of its path, which can often be represented by a line segment. This allows scientists and engineers to analyze the motion of objects and make predictions based on their understanding of distance and line segments.
In conclusion, line segments and distance are fundamental concepts in geometry and physics. They allow for the measurement and analysis of lengths and distances between points, objects, and shapes. Understanding how to calculate and work with line segments and distance is crucial in various fields and can provide practical applications for solving real-world problems.
Key Concepts in Line Segments
A line segment is a part of a line that is bounded by two distinct endpoints. It is a fundamental concept in geometry and is commonly used to measure distances and define shapes. Understanding key concepts related to line segments is crucial in solving problems involving lengths, positions, and intersections.
Endpoint: An endpoint is the starting or ending point of a line segment. It is typically represented by a dot, and its position determines the length of the line segment.
Length: The length of a line segment is the distance between its two endpoints. It can be measured in various units, such as inches, feet, or centimeters. The length of a line segment can be determined using various methods, including counting units, using a ruler or measuring tape, or through mathematical calculations.
Midpoint: The midpoint of a line segment is the point that divides the line segment into two equal halves. It is located at an equal distance from both endpoints and is commonly denoted by the letter “M”. The midpoint of a line segment can be found by using a ruler or by applying a formula that calculates the average of the coordinates of the endpoints.
Parallel Lines: Parallel lines are two or more lines that never intersect. They have the same slope but are located at different positions in space. In a line segment, if two lines are parallel, their respective endpoints also form a line segment with parallel sides.
Perpendicular Lines: Perpendicular lines are two lines that intersect to form right angles. In a line segment, if two lines are perpendicular, their respective endpoints also form a line segment that is perpendicular to another line segment.
- In summary, key concepts related to line segments include endpoints, length, midpoint, parallel lines, and perpendicular lines.
- These concepts are used to measure distances, define shapes, and solve geometric problems.
- Understanding these concepts is essential in geometry and other fields that involve spatial reasoning.
- Applying formulas and using visual tools such as rulers and graphs can help in calculating and visualizing line segments.
Exploring Distance in Line Segments
Distance is a fundamental concept in mathematics, and it plays a crucial role in geometry. When we talk about distance in line segments, we are referring to the length between two points on a straight line. This length is always positive and can be measured in various units such as centimeters, inches, or meters.
In order to determine the distance between two points on a line segment, we can use the distance formula, which is based on the Pythagorean theorem. The formula states that the square of the distance between two points (x1, y1) and (x2, y2) is equal to the sum of the squares of the differences in their x- and y-coordinates.
To illustrate this concept, let’s consider an example. Imagine we have a line segment with two endpoints, A (2, 4) and B (6, 8). To find the distance between these two points, we can apply the distance formula: d = √[(x2 – x1)² + (y2 – y1)²]. Plugging in the coordinates, we get: d = √[(6 – 2)² + (8 – 4)²]. Simplifying this expression, we find that the distance between points A and B is √20 or approximately 4.47 units.
Understanding distance in line segments is essential in various areas of mathematics, physics, and engineering. It allows us to measure distances accurately, calculate areas and volumes, and solve problems involving geometric shapes. By exploring distance in line segments, we gain a deeper understanding of the concepts of length and magnitude and their practical applications in real-life situations.
Approaching Practice Problems in 1-2
When it comes to practicing line segments and distance problems, it is important to approach them with a systematic and organized strategy. These types of problems often involve finding the length of a line segment or calculating the distance between two points on a coordinate plane. By following a step-by-step process, you can simplify these problems and increase your chances of finding the correct solution.
Step 1: Identify the Given Information
- Start by carefully reading the problem and identifying all the information that is given. This may include specific points on a coordinate plane, lengths of line segments, or any other relevant details.
- Make sure to note down all the given information in a clear and organized manner.
Step 2: Determine the Objective
- Next, determine what the problem is asking you to find. Are you looking for the length of a line segment, the distance between two points, or something else?
- Clearly define your objective and make a note of it so that you can stay focused throughout the problem-solving process.
Step 3: Choose a Suitable Method
- Based on the given information and the objective of the problem, choose a suitable method to solve it.
- For example, if you are asked to find the distance between two points on a coordinate plane, you can use the Pythagorean theorem or the distance formula.
Step 4: Apply the Chosen Method
- Once you have selected a method, apply it to the given information to find the solution.
- Pay attention to any equations or formulas that need to be used, and make sure to show all your work clearly.
Step 5: Check Your Answer
- After finding a solution, take the time to check your answer to ensure its accuracy.
- Double-check your calculations and make sure they align with the given information and the objective of the problem.
By following these steps and approaching practice problems in a systematic manner, you can improve your problem-solving skills and become more proficient in dealing with line segments and distance problems.
Answer Key for Practice Problems 1-2
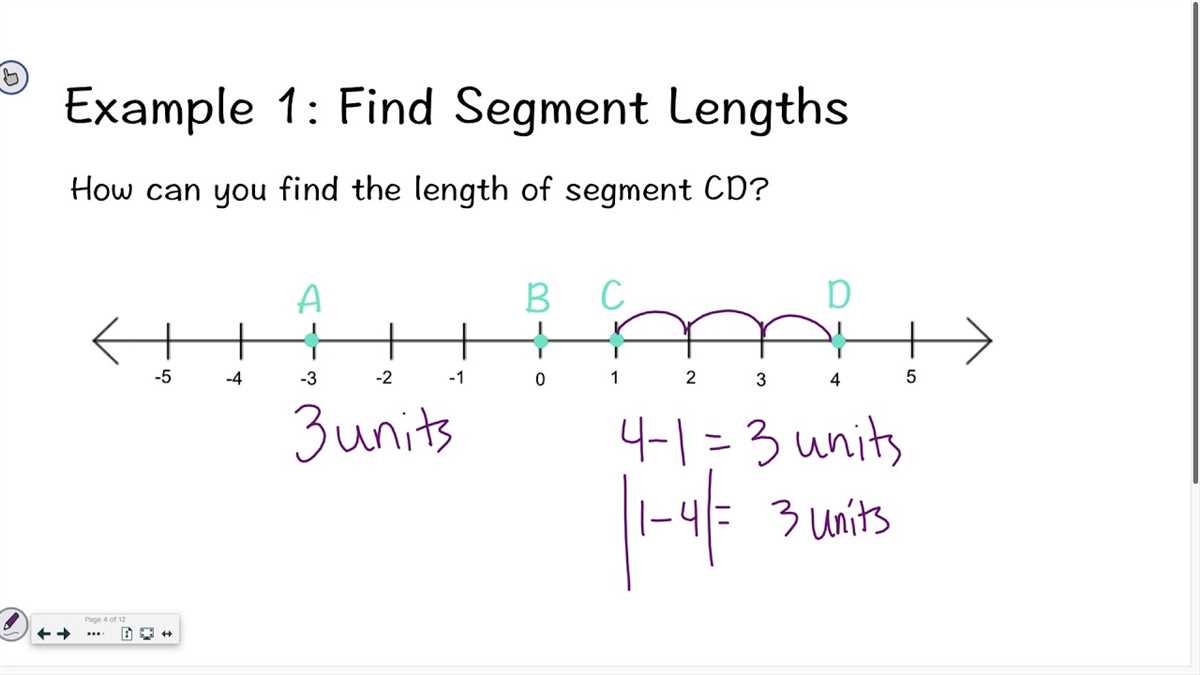
Below is the answer key for the practice problems 1-2 on line segments and distance:
- Problem 1: Find the distance between points A(2, 3) and B(5, 7).
- Answer: Using the distance formula, we have:
√[((5-2)^2) + ((7-3)^2)] = √[3^2 + 4^2] = √[9 + 16] = √25 = 5 units.
- Problem 2: Find the coordinates of the midpoint of the line segment with endpoints C(1, -2) and D(7, 5).
- Answer: Using the midpoint formula, we have:
x-coordinate: (1 + 7) / 2 = 8 / 2 = 4.
y-coordinate: (-2 + 5) / 2 = 3 / 2 = 1.5.
Therefore, the coordinates of the midpoint are (4, 1.5).
In summary, the distance between points A(2, 3) and B(5, 7) is 5 units, and the midpoint of the line segment with endpoints C(1, -2) and D(7, 5) is (4, 1.5).