
In mathematics, linear functions play a crucial role as they represent a straight line on a graph. These functions can be transformed by various operations, such as shifting, stretching, and reflecting. Understanding these transformations is essential in solving equations and graphing linear functions. In this article, we will explore the answer key to the transformations of linear functions in section 6.4.
First, let’s define the basic form of a linear function, which is in the form y = mx + b. Here, m represents the slope of the line, and b is the y-intercept, which is the point where the line intersects the y-axis. The slope determines the steepness of the line, while the y-intercept represents the point where the line crosses the y-axis at x = 0.
Now, let’s dive into the transformations of linear functions. The first transformation we will explore is shifting. Shifting a linear function involves moving the entire graph horizontally or vertically. It can be done by adding or subtracting a constant value to the equation. For example, if we have the equation y = mx + b and we want to shift it upward by 2 units, we can modify the equation to y = mx + (b + 2). This will result in the entire graph moving 2 units higher.
Understanding Linear Functions
Linear functions are one of the fundamental concepts in mathematics. They describe a relationship between two variables that can be represented by a straight line on a graph. In the context of transforming linear functions, it is important to have a strong understanding of how these functions work.
At its core, a linear function can be written in the form y = mx + b, where m represents the slope of the line and b represents the y-intercept. The slope determines how steep the line will be, and the y-intercept represents where the line crosses the y-axis. By analyzing the equation of a linear function, we can gather important information about its behavior and characteristics.
When transforming a linear function, certain changes can be made to the equation to shift or scale the line. For example, adding a constant to the equation will shift the entire line up or down, while multiplying the equation by a constant will scale the line vertically. These transformations can also be combined to create more complex changes to the line.
In order to effectively understand and work with linear functions, it is important to have a solid grasp of key concepts such as slope, y-intercept, and the effects of transformations. By studying and practicing these concepts, students can develop a strong foundation in algebra and problem-solving skills that will serve them well in various disciplines.
Exploring Transformations of Linear Functions

In mathematics, linear functions are often used to model relationships between two variables. These functions have the form y = mx + b, where m represents the slope of the line and b represents the y-intercept. Linear functions can be transformed in various ways, leading to different characteristics and patterns.
One common transformation is vertical translation, which involves shifting the entire graph up or down. This is achieved by adding or subtracting a constant term to the equation of the linear function. For example, adding 3 to the equation y = 2x + 5 will result in y = 2x + 8, shifting the graph 3 units higher. Vertical translations can affect the y-intercept and the steepness of the line.
Another transformation is horizontal translation, which involves shifting the entire graph left or right. This is accomplished by adding or subtracting a constant term to the x-variable in the equation. For instance, adding 2 to the x-variable in the equation y = 2x + 5 will yield y = 2(x+2) + 5, shifting the graph 2 units to the left. Horizontal translations do not alter the slope or the y-intercept of the line.
Other transformations of linear functions include vertical and horizontal stretches or compressions, reflection across the x-axis or y-axis, and rotation. These transformations can be achieved by multiplying or dividing the coefficients in the equation and applying the appropriate rules. Each transformation affects the characteristics of the linear function in unique ways, altering its slope, y-intercept, or both.
By exploring and understanding the different transformations of linear functions, mathematicians gain insights into the behavior and properties of these functions. This knowledge is crucial in various applications, such as modeling real-world scenarios and solving problems in fields like economics, physics, and engineering.
Using Transformations to Graph Linear Functions
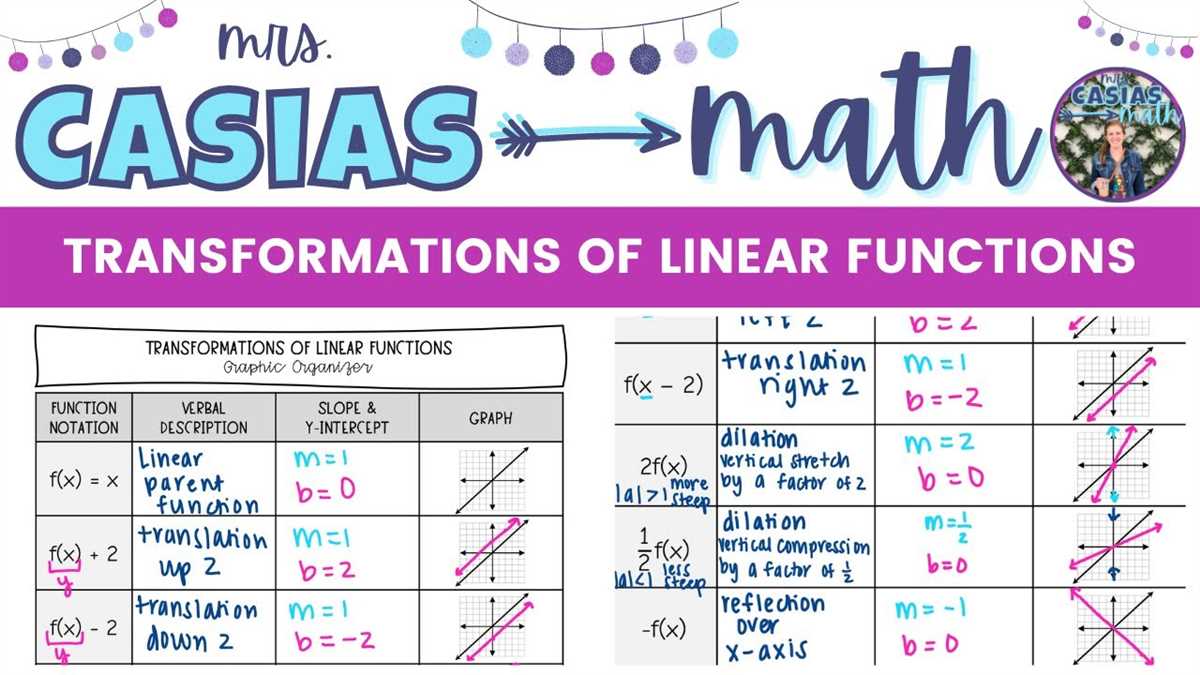
Linear functions are a fundamental concept in mathematics, and graphing them can provide valuable insight into their behavior. Transformations allow us to modify the graph of a linear function, helping us understand how changes in the equation affect its shape and position on the coordinate plane.
There are several types of transformations that can be applied to a linear function. Translation involves shifting the entire graph horizontally or vertically. For example, adding a constant to the x or y values of the function will shift the graph left, right, up, or down. This can be useful for understanding how changes in the equation affect the position of the linear function on the coordinate plane.
Another type of transformation is scaling, which involves stretching or compressing the graph horizontally or vertically. Multiplying the x or y values of the function by a constant will stretch or compress the graph accordingly. This transformation can help us understand how changes in the equation affect the shape of the linear function.
Using transformations, we can not only graph linear functions, but also analyze their behavior and make predictions. By applying translations and scalings to the original graph, we can determine how changes in the equation will affect the position and shape of the linear function. This allows us to explore the relationship between the equation and the graph, and gain a deeper understanding of linear functions and their properties.
Applying Transformations to Solve Equations
The concept of applying transformations to solve equations involves manipulating a given equation by applying specific transformations to both sides of the equation, in order to isolate the variable and find its value. The transformations used include addition and subtraction of constants, multiplication and division by constants, and applying inverse operations.
One of the key strategies when applying transformations to solve equations is to perform the same transformation to both sides of the equation in order to maintain equality. For example, if we have an equation like 2x + 5 = 15, we can isolate the variable x by subtracting 5 from both sides of the equation. This will yield 2x = 10. Then, dividing both sides of the equation by 2 will give us the solution x = 5.
Transformations can also be applied to more complex equations with multiple terms and variables. In these cases, it is important to identify which terms need to be eliminated or combined in order to isolate the desired variable. By applying the appropriate transformations, we can simplify the equation and solve for the variable.
Overall, applying transformations to solve equations is a fundamental skill in algebra. It allows us to manipulate equations in order to find the values of unknown variables and solve real-world problems. By understanding the concept of transformations and practicing their application, we can become proficient in solving various types of equations.
Analyzing the Effects of Transformations on Linear Functions
Linear functions can be transformed in various ways, resulting in changes to their graphs and equations. These transformations can include shifts, stretches, compressions, and reflections. By analyzing these effects, we can gain a deeper understanding of how these transformations impact the behavior and characteristics of linear functions.
One common transformation is a shift, which involves moving the entire graph of a linear function horizontally or vertically. A horizontal shift affects the equation by changing the value of the constant term, while a vertical shift changes the value of the slope. These shifts can result in the graph moving to the left or right, or up or down. By analyzing the equation and graph before and after the shift, we can observe how these changes impact the function’s intercepts, slope, and overall position.
Another transformation is a stretch or compression, which involves changing the slope of the linear function. A stretch occurs when the slope is multiplied by a value greater than 1, resulting in a steeper graph. Conversely, a compression occurs when the slope is multiplied by a value between 0 and 1, resulting in a flatter graph. By analyzing the resulting equations and graphs, we can observe how these changes affect the steepness or flatness of the function, as well as the intercepts and overall position.
In addition to shifts and stretches, linear functions can also be reflected across the x-axis or y-axis. A reflection across the x-axis changes the sign of the y-coordinates, while a reflection across the y-axis changes the sign of the x-coordinates. By studying the equations and graphs before and after the reflection, we can observe how these transformations impact the symmetry and orientation of the function.
In conclusion, analyzing the effects of transformations on linear functions allows us to gain a deeper understanding of how these changes impact the behavior and characteristics of the functions. By studying the resulting equations and graphs, we can observe how shifts, stretches, compressions, and reflections affect the intercepts, slope, steepness or flatness, symmetry, and overall position of the linear function.
Identifying and Describing Transformations in Real-world Scenarios
In real-world scenarios, linear functions can be transformed in various ways to model different situations or phenomena. These transformations involve changes in the slope and/or the y-intercept of the linear function, resulting in shifts, stretches, or compressions of the graph. By identifying and describing these transformations, we can better understand and analyze the relationship between variables in the real world.
Types of Transformations
There are several types of transformations that can occur in real-world scenarios:
- Vertical translation: This transformation occurs when the y-intercept of the linear function is changed. It shifts the graph vertically up or down.
- Vertical stretch or compression: This transformation occurs when the slope of the linear function is changed. It stretches or compresses the graph vertically.
- Horizontal translation: This transformation occurs when the x-values of the linear function are changed. It shifts the graph horizontally left or right.
- Horizontal stretch or compression: This transformation occurs when the x-values of the linear function are scaled. It stretches or compresses the graph horizontally.
Example Real-world Scenarios
Let’s consider two example scenarios to demonstrate how transformations can be identified and described:
- In a retail setting, the price of a product may be modeled by a linear function. If the y-intercept of the function is increased, it represents a vertical translation upwards, indicating a price increase. If the slope of the function is increased, it represents a vertical compression, indicating that the price is increasing at a faster rate.
- In a manufacturing environment, the production rate of a machine may be modeled by a linear function. If the x-values of the function are shifted to the right, it represents a horizontal translation, indicating a delay in production. If the x-values are scaled by a factor greater than 1, it represents a horizontal compression, indicating that the machine is producing faster.
Conclusion
By examining real-world scenarios and identifying the transformations present in linear functions, we can gain a deeper understanding of how different variables are related and how they change over time. These transformations help us analyze and make predictions about the behaviors and trends observed in various real-world situations.