
Velocity is an important concept in physics and is defined as the rate of change of an object’s position with respect to time. It is a vector quantity, meaning it has both magnitude and direction. To calculate velocity, we need to know both the distance an object has traveled and the time it took to travel that distance. Practice problems can help students solidify their understanding of velocity and gain confidence in applying the relevant formulas.
In this article, we will provide answers to a set of velocity practice problems. It is important to note that the practice problems should be attempted first before referring to the answers, as they serve as a learning tool. By practicing and working through these problems, students can better grasp the concepts of velocity and enhance their problem-solving skills.
The answers to the practice problems will be provided step-by-step, outlining the formulas used and the calculations made. Each problem will be explained thoroughly, allowing students to understand the reasoning behind each answer. Additionally, any relevant concepts or equations related to velocity will be discussed, ensuring a comprehensive understanding of the topic.
Velocity Practice Problems Worksheet Answers
Velocity practice problems can be challenging, but with the right approach and understanding of the concepts, they can become easier to solve. Here are some answers to common velocity practice problems that you may encounter:
1. A car travels at a velocity of 60 kilometers per hour for 3 hours. What is the distance traveled by the car?
To find the distance traveled, we can use the formula:
Distance = Velocity x Time
In this case, the velocity is 60 kilometers per hour and the time is 3 hours. Substituting these values into the formula:
Distance = 60 km/h x 3 h = 180 kilometers
Therefore, the car has traveled a distance of 180 kilometers.
2. An object is thrown vertically upwards with an initial velocity of 20 meters per second. How high does the object go?
To find the maximum height reached by the object, we can use the formula:
Height = (Velocity^2)/(2 x Acceleration)
In this case, the initial velocity is 20 meters per second and the acceleration is the acceleration due to gravity, which is approximately 9.8 meters per second squared. Substituting these values into the formula:
Height = (20^2)/(2 x 9.8) = 200/19.6 = 10.2 meters
Therefore, the object reaches a maximum height of 10.2 meters.
3. A train accelerates uniformly from rest to a velocity of 80 kilometers per hour in 10 seconds. What is the acceleration of the train?
To find the acceleration of the train, we can use the formula:
Acceleration = (Final Velocity – Initial Velocity)/Time
In this case, the final velocity is 80 kilometers per hour, the initial velocity is 0 kilometers per hour (since the train starts from rest), and the time is 10 seconds. Substituting these values into the formula:
Acceleration = (80 km/h – 0 km/h)/10 s = 8 km/h^2
Therefore, the acceleration of the train is 8 kilometers per hour squared.
These examples demonstrate how to apply the formulas for distance, height, and acceleration in velocity practice problems. By understanding these concepts and using the appropriate formulas, you can solve velocity problems more effectively.
What Are Velocity Practice Problems?
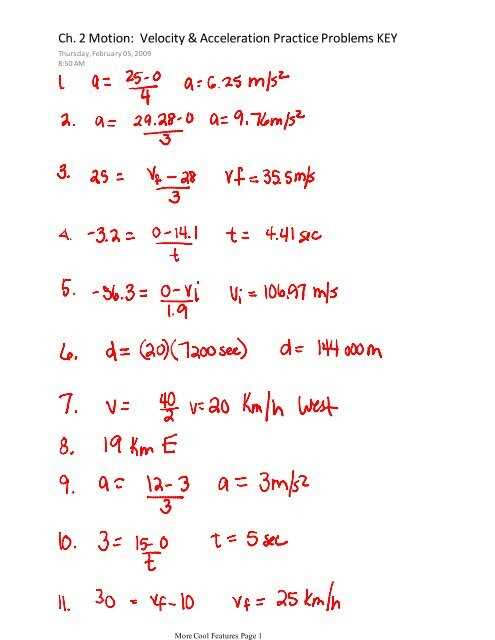
Velocity practice problems are exercises designed to help students understand and apply the concept of velocity in physics. Velocity is a measure of how fast an object is moving in a certain direction, taking into account both speed and direction. These practice problems provide students with the opportunity to calculate velocity using different formulas and analyze various scenarios to deepen their understanding of this fundamental concept.
In velocity practice problems, students are often given information about an object’s speed, direction, and time, and they are asked to calculate the object’s velocity. They may need to convert units, apply the formula v = d/t (where v is velocity, d is distance, and t is time), or use other relevant equations depending on the specific problem. These exercises not only help students develop their mathematical skills, but also enhance their problem-solving abilities and critical thinking.
By practicing velocity problems, students can strengthen their comprehension of key concepts such as displacement, average velocity, and instantaneous velocity. They can also gain insight into real-world applications of velocity, such as analyzing the motion of vehicles, understanding the speed of projectiles, or predicting the trajectory of moving objects. These problems can be found in textbooks, online resources, or provided by teachers as part of their coursework to assess students’ understanding and mastery of velocity concepts.
To effectively solve velocity practice problems, students need to have a solid understanding of basic physics principles, including distance, time, speed, and vectors. They should also be familiar with relevant formulas and equations, and be able to manipulate units of measurement. By consistently practicing these problems, students can improve their problem-solving skills, reinforce their understanding of velocity, and become more confident in applying physics concepts to real-world situations.
Why Are Velocity Practice Problems Important?
Velocity practice problems play a crucial role in helping students grasp the concept of velocity and develop their problem-solving skills in physics. Velocity, defined as the rate at which an object changes its position, is a fundamental concept in physics that is applicable to a wide range of real-world scenarios. By working on velocity practice problems, students are able to apply the principles they have learned in class to solve practical problems, enhancing their understanding of the subject matter.
Firstly, practicing velocity problems allows students to become familiar with the various equations and formulas associated with velocity, such as the formula for average velocity (v = Δx/Δt). By repeatedly using these formulas in different problem-solving scenarios, students gain a deeper understanding of their application and become more comfortable utilizing them in future physics problems.
Secondly, velocity practice problems help students develop critical thinking and analytical skills. Physics is a discipline that requires logical reasoning and problem-solving abilities. By tackling velocity problems, students are challenged to think critically about the given information and devise a systematic approach to solve the problem. This process allows them to hone their analytical skills and improve their ability to break down complex problems into manageable steps.
Furthermore, velocity practice problems provide students with an opportunity to apply mathematical concepts to real-world situations. Physics, as an experimental science, aims to explain natural phenomena using mathematical models and principles. By solving velocity problems, students are able to see the practical application of their mathematical knowledge and gain a deeper appreciation for the power of physics in understanding the world around them.
In conclusion, velocity practice problems are important for students to reinforce their understanding of velocity, enhance their problem-solving abilities, and develop their critical thinking skills. By engaging in these practice problems, students are able to apply theoretical concepts to real-world scenarios and improve their overall comprehension of physics.
How to Solve Velocity Practice Problems

Solving velocity practice problems involves understanding the concepts and equations related to velocity, as well as applying mathematical calculations to solve for unknown variables. Here are step-by-step instructions on how to solve velocity practice problems:
1. Identify the given information: Read the problem carefully and identify the values given to you. Look for key variables such as initial velocity (u), final velocity (v), acceleration (a), time (t), or distance (d).
2. Determine the unknown variable: Determine which variable you need to solve for based on the given information and what the problem is asking for. It could be any of the aforementioned variables.
3. Use the appropriate equation: Depending on the nature of the problem, you’ll need to choose the appropriate equation to solve for the unknown variable. Some common equations related to velocity include:
- Velocity equation: v = u + at
- Acceleration equation: a = (v – u) / t
- Distance equation: d = ut + (1/2)at^2
- Time equation: t = (v – u) / a
4. Rearrange the equation: Rearrange the equation to isolate the unknown variable on one side of the equation. This may involve performing mathematical operations such as addition, subtraction, multiplication, or division.
5. Substitute the values: Substitute the given values into the rearranged equation, ensuring units are consistent. Plug in the values and perform the necessary calculations to solve for the unknown variable.
6. Check your answer: Once you have solved for the unknown variable, double-check your answer to ensure it makes sense in the context of the problem. Also, calculate any related variables if necessary to verify consistency with the problem’s given information.
By following these steps and practicing different velocity problems, you can develop a better understanding of velocity concepts and improve your problem-solving skills.
Common Mistakes in Solving Velocity Practice Problems
In order to correctly solve velocity practice problems, it is important to avoid common mistakes that can lead to incorrect answers or confusion. One common mistake is not properly identifying the given variables and their units. It is crucial to clearly understand what information is given in the problem and ensure that the units are consistent.
Another mistake is not using the correct formulas or equations. Velocity problems often involve distance, time, and speed, so it is important to use the appropriate formulas such as velocity equals distance divided by time. Using the wrong formula can lead to incorrect answers.
Confusion can also arise from not understanding the difference between average velocity and instantaneous velocity. Average velocity is calculated over a specific time interval, while instantaneous velocity refers to the velocity at a specific moment in time. It is important to correctly interpret the problem and determine which type of velocity is being asked for.
Additionally, neglecting to convert units can also lead to errors. It is important to convert units, such as miles to kilometers or seconds to minutes, when necessary in order to ensure consistency and accuracy in calculations.
Lastly, rounding errors can occur if calculations are not done with enough precision. It is important to carry out calculations with an appropriate number of decimal places and round the final answer to the appropriate number of significant digits.
By avoiding these common mistakes, students can improve their accuracy and understanding when solving velocity practice problems.
Sample Velocity Practice Problems with Explanations
In this section, we will provide a few sample velocity practice problems along with explanations to help you understand the concept better.
Problem 1:
An object is moving with a constant velocity of 10 m/s. If it travels for 5 seconds, what is the total distance covered?
Answer:
The formula to calculate distance is:
Distance = Velocity × Time
Distance = 10 m/s × 5 s
Distance = 50 meters
Therefore, the total distance covered by the object is 50 meters.
Problem 2:
An athlete runs with a velocity of 8 m/s for 10 seconds. What is the total distance covered?
Answer:
Using the same formula as above:
Distance = Velocity × Time
Distance = 8 m/s × 10 s
Distance = 80 meters
Therefore, the total distance covered by the athlete is 80 meters.
These sample problems demonstrate how to calculate distance using velocity and time. Remember to always multiply the velocity by the time to find the total distance covered. Velocity practice problems can vary in complexity, but the basic concept remains the same.
By practicing more velocity problems, you will become more comfortable with the concept and be able to apply it in real-world scenarios. Keep practicing and keep improving your understanding of velocity!