
Momentum conservation is a fundamental law of physics that states that the total momentum of a closed system remains constant if no external forces act on it. This principle is based on the law of conservation of momentum, which states that the total momentum before an event is equal to the total momentum after the event. To understand and apply this concept, students often complete worksheet exercises that help them practice calculating and analyzing momentum in various scenarios.
These momentum conservation worksheets provide students with a series of problems and questions that test their understanding of the topic. The worksheets typically include scenarios like collisions between objects and the interaction of objects with different masses and velocities. By solving these problems, students can apply the principles of momentum conservation and develop their problem-solving skills.
The answers to these momentum conservation worksheets are essential for students to check their work and understand the correct calculations. These answers help students verify their solutions and learn from any mistakes they may have made in their calculations. By comparing their answers to the given answers, students can identify and correct any misconceptions or errors in their approach to solving the problems.
Momentum Conservation Worksheet Answers
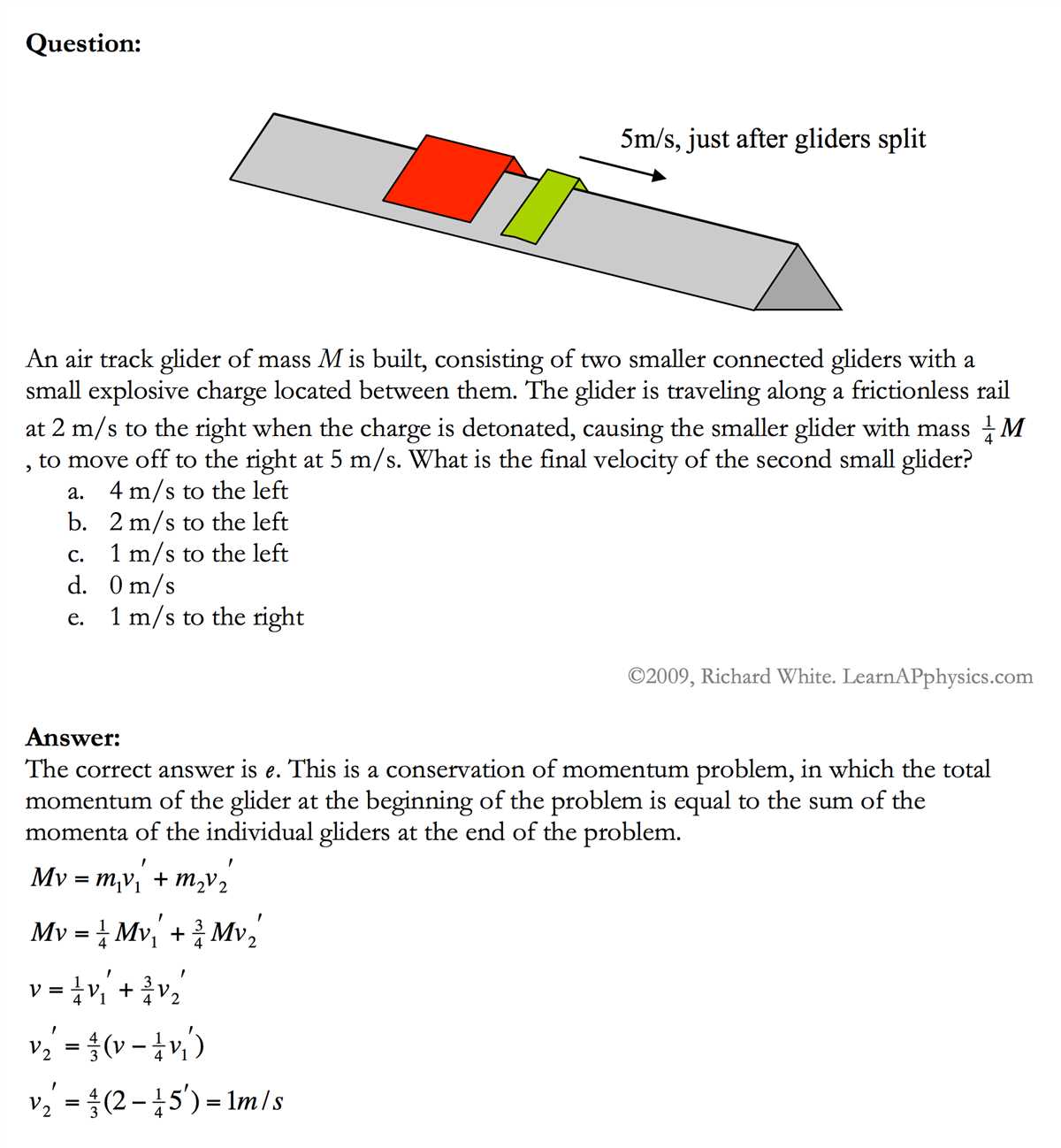
In the study of physics, the principle of momentum conservation plays a crucial role in understanding the behavior of objects in motion. This principle states that the total momentum of a system remains constant if no external forces act on it. To better comprehend this concept, students often complete worksheets that involve analyzing various scenarios and applying the principle of momentum conservation to solve problems.
Here are some example answers to a momentum conservation worksheet:
- Scenario 1: A 2 kg car traveling at 10 m/s collides with a stationary 3 kg car. The two cars stick together after the collision. Calculate their final velocity.
- Scenario 2: A 0.5 kg puck slides across the ice with an initial velocity of 4 m/s. It collides with a stationary 1 kg puck and bounces off in the opposite direction. Calculate the final velocity of each puck.
In this scenario, we can apply the principle of momentum conservation, which states that the total momentum before the collision is equal to the total momentum after the collision.
The momentum before the collision can be calculated as:
Initial momentum = (mass of car 1 × velocity of car 1) + (mass of car 2 × velocity of car 2)
Initial momentum = (2 kg × 10 m/s) + (3 kg × 0 m/s) = 20 kg·m/s
Since the cars stick together after the collision, their masses can be added together:
Total mass = mass of car 1 + mass of car 2 = 2 kg + 3 kg = 5 kg
The final velocity can be calculated as:
Final velocity = total momentum / total mass = 20 kg·m/s / 5 kg = 4 m/s
In this scenario, we can again apply the principle of momentum conservation to solve the problem.
The momentum before the collision can be calculated as:
Initial momentum = (mass of puck 1 × velocity of puck 1) + (mass of puck 2 × velocity of puck 2)
Initial momentum = (0.5 kg × 4 m/s) + (1 kg × 0 m/s) = 2 kg·m/s
Since the first puck bounces off in the opposite direction, the final velocity of the first puck can be calculated as:
Final velocity of puck 1 = -initial velocity of puck 1 = -4 m/s
The final velocity of the second puck can be calculated as:
Final velocity of puck 2 = (initial momentum – (mass of puck 1 × final velocity of puck 1)) / mass of puck 2
Final velocity of puck 2 = (2 kg·m/s – (0.5 kg × -4 m/s)) / 1 kg = 6 m/s
These answers demonstrate the application of the principle of momentum conservation in various scenarios and highlight the importance of understanding this concept in the study of physics.
What is Momentum Conservation?
Momentum conservation is a fundamental principle in physics that states that the total momentum of a closed system remains constant if no external forces act on it. In other words, the total momentum before a collision or interaction is equal to the total momentum after the collision or interaction.
This principle is derived from Newton’s laws of motion and is based on the concept of momentum, which is the product of an object’s mass and velocity. Momentum is a vector quantity, which means it has both magnitude and direction. Therefore, in order to account for the conservation of momentum, both the magnitude and direction of momentum must be taken into consideration.
When two objects collide or interact, the momentum of each object can change. However, according to the law of momentum conservation, the total momentum of the system will remain the same. This means that if one object gains momentum, the other object will lose an equal amount of momentum in the opposite direction.
The principle of momentum conservation has many practical applications in various fields of physics, such as mechanics, fluid dynamics, and electromagnetism. It is used to analyze and calculate the motion and interactions of objects in different scenarios, such as collisions, explosions, and fluid flow. By applying the principle of momentum conservation, scientists and engineers can predict and understand the behavior of systems and develop solutions to real-world problems.
Principles of Momentum Conservation
The principle of momentum conservation states that the total momentum of an isolated system remains constant before and after any interaction takes place. Momentum is a vector quantity that measures the motion of an object, defined as the product of its mass and velocity. Whenever two or more objects interact, their individual momenta may change, but the total momentum of the system remains the same.
According to Newton’s third law of motion, for every action, there is an equal and opposite reaction. This principle plays a crucial role in the conservation of momentum. When two objects collide, the forces they exert on each other are equal in magnitude and opposite in direction. As a result, the total momentum of the system before and after the collision is conserved.
The conservation of momentum can be applied to various scenarios, such as collisions between objects, explosions, and even rocket propulsion. In a collision, the total momentum of the system can be calculated by adding the individual momenta of the objects involved. If the collision is elastic, both the kinetic energy and momentum of the system are conserved. If it is inelastic, kinetic energy may not be conserved, but momentum still remains constant.
Understanding the principles of momentum conservation is crucial in analyzing and predicting the motion of objects in various physical systems. It allows us to determine the outcomes of interactions between objects and helps us explain various phenomena, such as the recoil of firearms or the motion of planets and satellites. By utilizing the concept of momentum conservation, scientists and engineers can design more efficient systems and devices, improving our understanding of the natural world and advancing technological progress.
Worksheet Questions

Here are the questions from the worksheet on momentum conservation, along with their answers:
1. A 0.5 kg ball is moving with a velocity of 2 m/s. It collides with a wall and bounces back with a velocity of -2 m/s. What is the change in momentum of the ball?
The change in momentum of the ball can be calculated by subtracting its initial momentum from its final momentum. The initial momentum can be calculated by multiplying the mass of the ball with its initial velocity, while the final momentum can be calculated by multiplying the mass of the ball with its final velocity. Therefore, the change in momentum is:
Change in momentum = (0.5 kg * -2 m/s) – (0.5 kg * 2 m/s) = -2 kg m/s – 1 kg m/s = -3 kg m/s
2. Two objects of masses 1 kg and 2 kg are moving with velocities of 3 m/s and -2 m/s respectively. They collide and stick together. What is their final velocity?
When two objects collide and stick together, their masses combine and their final velocity is determined by the conservation of momentum. The total initial momentum can be calculated by adding the individual momenta of the two objects. Therefore:
Total initial momentum = (1 kg * 3 m/s) + (2 kg * -2 m/s) = 1 kg m/s – 4 kg m/s = -3 kg m/s
The total final momentum is equal to the total initial momentum, due to momentum conservation. Therefore, the final velocity can be calculated using the formula:
Final velocity = Total final momentum / (total mass of the combined objects)
Final velocity = -3 kg m/s / (1 kg + 2 kg) = -1 kg m/s
3. A car with a mass of 1000 kg is initially moving with a velocity of 10 m/s. It collides with a stationary car with a mass of 2000 kg. After the collision, the first car moves with a velocity of 5 m/s. What is the final velocity of the second car?
In this case, we need to use the conservation of momentum to find the final velocity of the second car. The total initial momentum can be calculated by adding the individual momenta of the two cars:
Total initial momentum = (1000 kg * 10 m/s) + (2000 kg * 0 m/s) = 10000 kg m/s + 0 kg m/s = 10000 kg m/s
The total final momentum is also equal to the total initial momentum, due to momentum conservation. Therefore, the final velocity of the second car can be calculated using the formula:
Final velocity of the second car = Total final momentum / (mass of the second car)
Final velocity of the second car = 10000 kg m/s / 2000 kg = 5 m/s
4. A rocket with a mass of 5000 kg is moving with a velocity of 100 m/s. It ejects a fuel canister with a mass of 200 kg at a velocity of 200 m/s. What is the final velocity of the rocket?
To find the final velocity of the rocket, we need to apply the conservation of momentum. The total initial momentum is given by the sum of the individual momenta of the rocket and the fuel canister:
Total initial momentum = (5000 kg * 100 m/s) + (200 kg * 200 m/s) = 500000 kg m/s + 40000 kg m/s = 540000 kg m/s
The total final momentum is equal to the total initial momentum, so we can calculate the final velocity of the rocket using the formula:
Final velocity of the rocket = Total final momentum / (mass of the rocket)
Final velocity of the rocket = 540000 kg m/s / 5000 kg = 108 m/s
5. A 1 kg ball is moving with a velocity of 4 m/s. It collides with a wall and comes to rest. What is the impulse experienced by the ball?
The impulse experienced by the ball can be calculated by multiplying the force exerted on the ball by the time it takes to come to rest. Since the ball comes to rest, its final velocity is 0 m/s. Therefore, the change in velocity is:
Change in velocity = 0 m/s – 4 m/s = -4 m/s
The impulse experienced by the ball is equal to the change in momentum, which can be calculated by multiplying the mass of the ball with the change in velocity:
Impulse = 1 kg * -4 m/s = -4 kg m/s
These are the solutions to the worksheet questions on momentum conservation. By using the principles of momentum conservation, we can calculate the change in momentum, final velocities, and impulses experienced by objects in various scenarios. Understanding these concepts is crucial in analyzing and predicting the motion of objects in collisions and interactions.
Step-by-Step Solutions
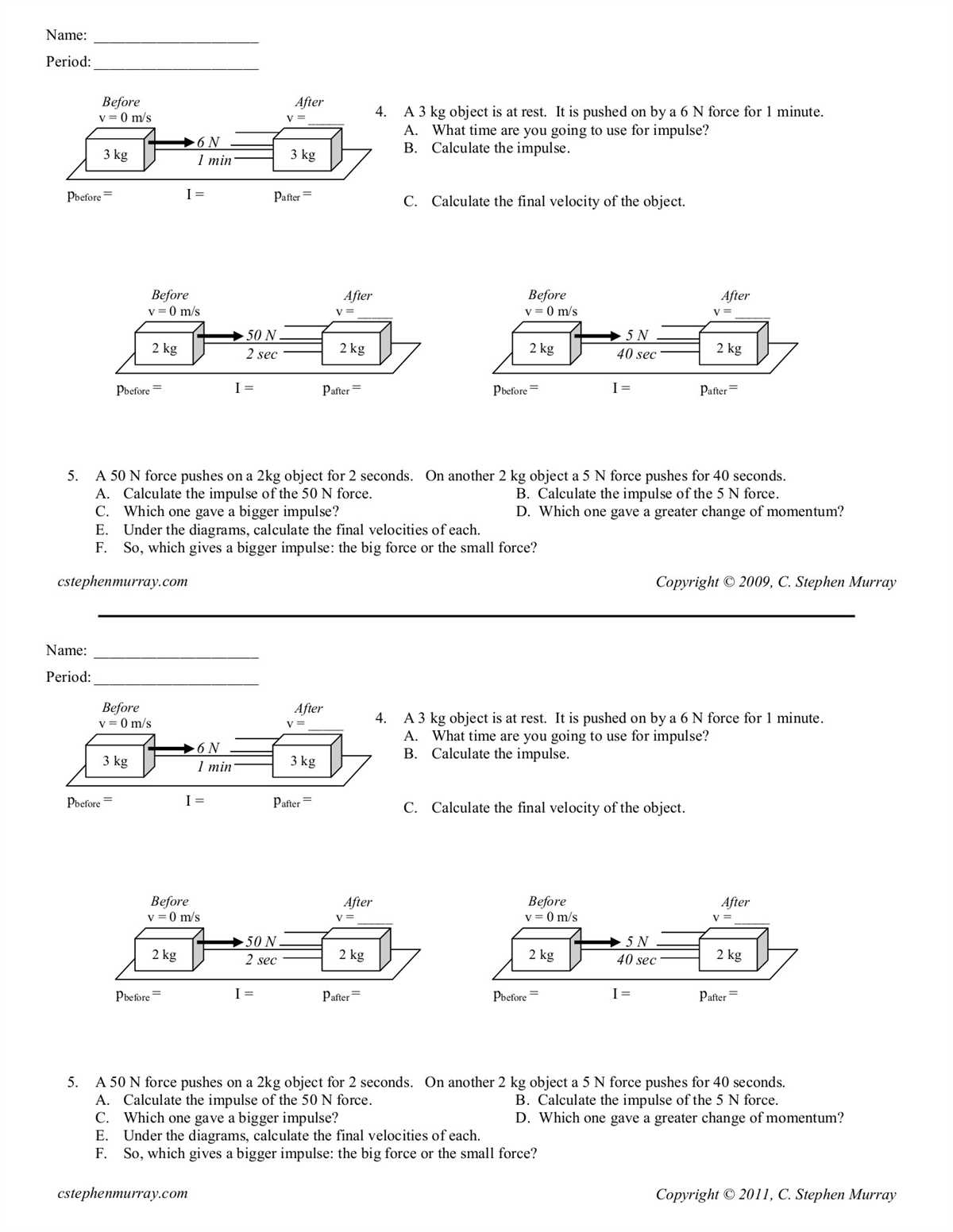
When solving momentum conservation problems, it is important to follow a step-by-step approach. This helps ensure that all necessary information is taken into account and that the correct formulas and calculations are used.
Step 1: Identify the objects involved in the collision and their initial momenta. This can be determined by considering the mass and velocity of each object before the collision.
Step 2: Determine the total initial momentum of the system. This is done by summing up the individual momenta of the objects involved.
Step 3: Analyze the collision and determine whether it is elastic or inelastic. In an elastic collision, kinetic energy is conserved, while in an inelastic collision, kinetic energy is not conserved.
Step 4: Use the principles of momentum conservation to set up an equation. This equation should state that the total initial momentum is equal to the total final momentum.
Step 5: Solve the equation for the final momenta of the objects. This can be done by isolating the unknown variables and solving for them algebraically.
Step 6: Check your solution by plugging in the calculated final momenta back into the equation from step 4. If the equation holds true, then the solution is correct.
By following these steps, you can successfully solve momentum conservation problems and find the final momenta of the objects involved in the collision. Practice and familiarity with the concepts will improve your ability to solve these types of problems efficiently and accurately.
Common Mistakes and Tips
When working on momentum conservation problems, it’s common to make a few mistakes. Here are some common mistakes to avoid and some tips to help you approach these problems effectively:
1. Ignoring external forces: One common mistake is forgetting to consider external forces acting on the system. Remember, momentum is conserved only when there are no external forces acting on the system. Take the time to identify all the forces acting on the objects involved in the collision.
2. Misinterpreting conservation of momentum: Another mistake is misinterpreting the principle of momentum conservation. Remember that momentum is a vector quantity, meaning it has both magnitude and direction. When working with momentum conservation, be sure to properly account for the direction of the momentum vectors.
3. Incorrectly applying equations: It’s important to carefully apply the equations related to momentum conservation. Double-check your algebraic manipulations and ensure you are using the correct signs and variables in your calculations. It’s also helpful to clearly define your coordinate system and choose appropriate positive and negative directions.
4. Neglecting the final state: Sometimes, students only focus on the initial state of the system and neglect the final state after the collision. Remember that momentum is conserved throughout the entire interaction, including the final state. Make sure to consider the velocities of the objects after the collision and analyze any changes in their momentum.
Here are some tips to help you approach momentum conservation problems:
- Draw clear diagrams: Start by drawing clear and labeled diagrams of the system before and after the collision. This will help you visualize the problem and identify the forces involved.
- Break the problem into stages: Sometimes, it’s helpful to break the problem into stages and analyze each stage separately. This can make the problem more manageable and prevent confusion.
- Use vector notation: When working with momentum vectors, use vector notation to clearly represent both magnitude and direction. This will help you keep track of the vectors and ensure accurate calculations.
- Check your answer: Always check your answer to ensure it makes sense. Verify that momentum is conserved and that the magnitudes and directions of the velocities are reasonable for the given situation.
By avoiding common mistakes and following these tips, you’ll be better equipped to solve momentum conservation problems effectively. Remember to practice regularly to improve your skills and understanding of this topic.