
Welcome to lesson 1 of comparing fractions and decimals! In this lesson, we will be going over the answer key for the exercises you completed in the previous lesson. This will help you understand how to compare fractions and decimals accurately and confidently. So let’s dive in and see how you did!
First, let’s start with the exercises on comparing fractions. You were given a set of fractions and asked to determine which ones are greater than, less than, or equal to each other. The answer key will show you the correct comparisons and explain the reasoning behind them.
Next, we will move on to comparing decimals. Decimals can be a bit trickier to compare, but with the right strategies, you’ll be able to master this skill. The answer key will provide you with the correct comparisons and guide you through the process of comparing decimals step by step.
By going through the answer key, you will gain a better understanding of how to compare fractions and decimals. Remember to take note of any mistakes you made and learn from them. Practice makes perfect, so keep working on these skills, and in no time, you’ll be a pro at comparing fractions and decimals!
Objective
The objective of this lesson is to teach students how to compare fractions and decimals. By the end of the lesson, students should be able to correctly compare and order fractions and decimals, understand the concept of equivalent fractions and decimals, and apply these skills to solve real-world problems.
Key phrases:
- Compare fractions and decimals
- Correctly compare and order fractions and decimals
- Understand the concept of equivalent fractions and decimals
- Apply skills to real-world problems
Throughout this lesson, students will engage in various activities and exercises to develop their understanding and proficiency in comparing fractions and decimals. They will be introduced to strategies such as finding common denominators and converting fractions to decimals to facilitate accurate comparisons. Students will also learn how to identify and use equivalent fractions and decimals to determine if two numbers are equal.
By the end of the lesson, students should be able to confidently apply their knowledge to real-world scenarios, such as comparing prices at the grocery store or determining the length of time between two events expressed as fractions and decimals. This lesson will provide a solid foundation for future lessons on working with fractions and decimals, and will equip students with valuable skills for problem-solving in mathematics and everyday life.
Understanding fractions and decimals
Fractions and decimals are two different ways to represent parts of a whole. While fractions are written as a ratio of two numbers separated by a line, decimals are written as a number with a decimal point.
Fractions: A fraction represents a part of a whole or a group and consists of a numerator and a denominator. The numerator represents the number of parts we have, while the denominator represents the total number of equal parts that make up the whole or group. For example, in the fraction 3/4, the numerator is 3 and the denominator is 4. This means we have 3 out of 4 equal parts of the whole.
Decimals: Decimals are another way to represent parts of a whole or a group. They are based on the decimal system, which uses the base 10. In a decimal number, the digit to the right of the decimal point represents tenths, the digit to the right of that represents hundredths, and so on. For example, in the decimal number 0.75, the digit 7 represents 7 tenths and the digit 5 represents 5 hundredths.
Comparing fractions and decimals is an important skill in mathematics. It allows us to understand and interpret numerical information in different formats. By learning how to convert between fractions and decimals, we can solve problems, make calculations, and make sense of real-life situations.
Example:
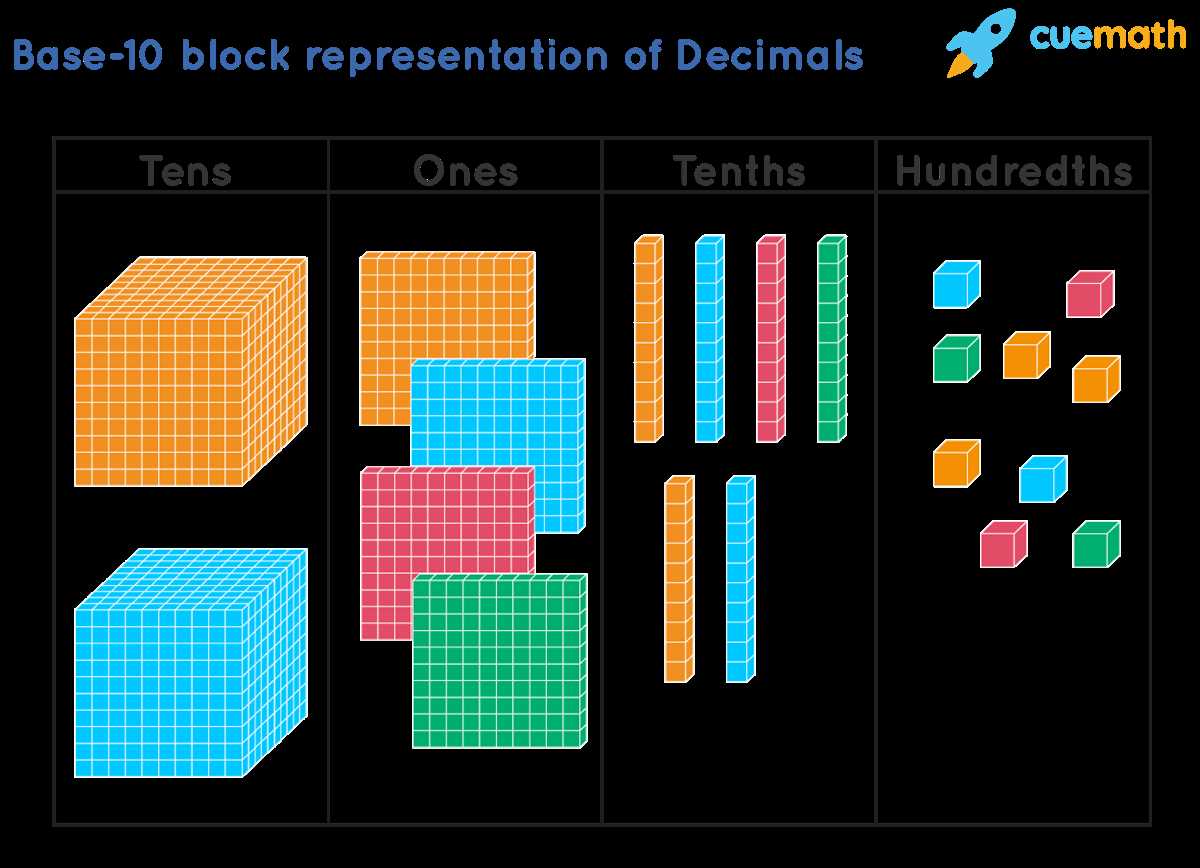
Let’s say we have a pizza that is divided into 8 equal slices. If we eat 3 slices, we can represent this as the fraction 3/8 or the decimal 0.375. Both representations convey the same information – that we have consumed 3 out of 8 slices.
Comparing fractions and decimals
Comparing fractions and decimals is an important skill in mathematics. It allows us to determine which value is greater or smaller between two numbers. Understanding how to compare fractions and decimals enables us to make accurate comparisons and solve various mathematical problems.
A fraction represents a part of a whole, while a decimal represents a number in the base-10 system. When comparing fractions and decimals, we need to convert them into the same format to easily compare them. For example, we can convert a fraction into a decimal by dividing the numerator by the denominator.
When comparing fractions and decimals, we can use different techniques such as converting fractions to decimals, finding common denominators, or using visual representations. To compare fractions, we can also look at the numerator and denominator to determine which fraction is larger or smaller. In decimals, we look at the place value of digits after the decimal point.
For example, when comparing the fractions 1/4 and 1/3, we can convert both fractions to decimals by dividing 1 by 4 and 1 by 3. The decimal form of 1/4 is 0.25 and the decimal form of 1/3 is approximately 0.33. By comparing these decimals, we can see that 0.33 is greater than 0.25. Therefore, 1/3 is greater than 1/4.
It is important to practice comparing fractions and decimals to develop a strong understanding of their relationship and to confidently solve problems in mathematical contexts.
Practice Exercises
In order to strengthen your understanding and proficiency in comparing fractions and decimals, it is essential to practice solving various exercises. This will help reinforce the concepts learned in the lesson, improve your problem-solving skills, and enhance your ability to accurately compare fractions and decimals.
Here are some practice exercises to get you started:
Exercise 1:
- Compare the fractions 3/8 and 2/5.
- Convert the decimal 0.6 into a fraction and compare it with the fraction 4/5.
- Order the following decimals from least to greatest: 0.75, 0.6, 0.9, 0.25.
Exercise 2:
| Question | Answer |
|---|---|
| Compare the fractions 1/3 and 2/5. | The fraction 2/5 is greater than the fraction 1/3. |
| Convert the decimal 0.4 into a fraction and compare it with the fraction 3/4. | The decimal 0.4 is equivalent to the fraction 2/5, which is less than the fraction 3/4. |
| Order the following decimals from least to greatest: 0.35, 0.6, 0.15, 0.05. | 0.05 < 0.15 < 0.35 < 0.6 |
Make sure to show all your work and explanations for each exercise. If you encounter any difficulties, refer back to the lesson materials and review the relevant concepts. Practice regularly to further improve your skills in comparing fractions and decimals.
Answer Key
Here is the answer key for the Compare Fractions and Decimals Lesson 1:
1. Compare the Fractions:
- A: 2/5
- B: 3/8
- C: 7/10
- D: 1/2
- E: 4/5
2. Compare the Decimals:
- A: 0.4
- B: 0.37
- C: 0.63
- D: 0.25
- E: 0.9
Summary:
In this lesson, we learned how to compare fractions and decimals. We practiced comparing fractions by finding a common denominator and then comparing the numerators. We also compared decimals by looking at the digit in the tenths or hundredths place. Overall, it is important to remember that when comparing fractions, larger denominators do not always mean a larger value, and when comparing decimals, the digit in the tenths or hundredths place determines the bigger value.