When studying systems of inequalities, it is important to have a clear understanding of the key concepts and how to solve for the answer key. This study guide aims to provide a comprehensive overview of the topic, with a focus on the answer key for solving these types of problems.
The study guide begins by explaining the basic definition of a system of inequalities and the different methods for solving them. It covers the graphical representation of inequalities and how to plot the solution set on a coordinate plane.
The study guide also includes a step-by-step explanation of how to solve systems of inequalities algebraically. It highlights the importance of identifying the variables and solving for their values, as well as determining the regions of the solution set.
In order to ensure a thorough understanding of the topic, the study guide provides numerous examples along with their corresponding answer key. These examples cover a range of difficulty levels, allowing students to practice and reinforce their understanding of the material.
By following this study guide, students will gain a solid foundation in solving systems of inequalities and be well-prepared for any related assignments or exams. The answer key provided will serve as a valuable resource for self-assessment and checking their work.
6 Study Guide and Intervention Systems of Inequalities Answer Key
In mathematics, systems of inequalities involve multiple inequalities with the same variables. These systems can be graphed on a coordinate plane to represent the region of solutions. The 6 Study Guide and Intervention Systems of Inequalities Answer Key provides solutions and explanations for problems related to this topic.
The answer key is a valuable resource for students studying systems of inequalities, as it helps them check their work and understand the steps involved in solving problems. It includes step-by-step solutions to various types of inequalities, such as linear inequalities and systems with absolute values.
The answer key also provides examples of graphing systems of inequalities on a coordinate plane. It explains how to determine the shaded region and identify the solution set. Additionally, it offers tips and strategies for solving systems of inequalities efficiently.
Key concepts covered in the 6 Study Guide and Intervention Systems of Inequalities Answer Key include:
- Graphing linear inequalities
- Solving systems of linear inequalities
- Graphing systems of linear inequalities
- Solving systems of absolute value inequalities
- Interpreting the solution set of a system of inequalities
With the help of the 6 Study Guide and Intervention Systems of Inequalities Answer Key, students can improve their understanding of this topic and enhance their problem-solving skills. It serves as a valuable tool for both teachers and students in the study of systems of inequalities.
Solving Systems of Inequalities Graphically
A system of inequalities is a set of two or more inequalities that are graphed on the same coordinate plane. Solving a system of inequalities graphically involves finding the region where the shaded areas of each inequality overlap. This region represents the solution to the system.
Graphing a system of inequalities requires understanding how to graph individual inequalities. Each inequality can be graphed by first converting it to slope-intercept form (y = mx + b) and then plotting the boundary line. The inequality symbol determines whether the line should be solid or dashed. For example, if the inequality symbol is “>=” or “<=", the line is graphed as a solid line. If the symbol is ">” or “<", the line is graphed as a dashed line.
Once the boundary lines are graphed, the next step is to shade the appropriate regions. If the inequality symbol is “>” or “>=”, the region above the line is shaded. If the inequality symbol is “<" or "<=", the region below the line is shaded. The shaded regions represent the solutions to each individual inequality.
To find the solution to the system of inequalities, identify the overlapping region of the shaded areas. This region represents the values that satisfy all of the given inequalities. In some cases, the overlapping region may be a single point or a bounded area. In other cases, the overlapping region may be an unbounded area.
Properties of Solutions to Systems of Inequalities
A system of inequalities consists of multiple inequalities that need to be satisfied simultaneously. The solution to a system of inequalities is the set of all values that make all inequalities in the system true.
There are several key properties of solutions to systems of inequalities that can help us analyze and graph them:
- Feasible Region: The feasible region is the set of all possible solutions to a system of inequalities. It is represented by a shaded region on a graph. The points within this region satisfy all the inequalities in the system.
- Graphing: To graph a system of inequalities, we plot each inequality on the same coordinate plane and shade the region that satisfies all the inequalities. The feasible region is the overlapping shaded area.
- Corner Points: The corner points of the feasible region are the points where the boundary lines of the inequalities intersect. These points are important because they represent the maximum or minimum values of the objective function in linear programming problems.
- Unbounded Solutions: In some cases, a system of inequalities may have an unbounded solution, meaning there is no maximum or minimum value. This occurs when the feasible region extends infinitely in one or more directions.
Understanding and applying these properties can help us solve and analyze systems of inequalities more effectively. By graphing the system and identifying the feasible region and its properties, we can make informed decisions and solutions in various real-life scenarios.
Methods for Solving Systems of Inequalities Algebraically
When solving systems of inequalities algebraically, there are several methods that can be used to find the solution. These methods involve manipulating the inequalities to isolate the variables and determine the possible values that satisfy both equations. Here are some common methods for solving systems of inequalities algebraically:
- Graphing: This method involves graphing both inequalities on the coordinate plane and finding the intersection of the shaded regions. The solution to the system of inequalities is the set of points that satisfy both inequalities.
- Substitution: In this method, one inequality is solved for one variable in terms of the other variables, and then substituted into the other inequality. This creates a single-variable inequality that can be solved to find the range of values for that variable. The process is repeated for the other variables until a solution is determined.
- Elimination: This method involves manipulating the inequalities so that one variable can be eliminated through addition or subtraction. This creates a single-variable inequality that can be solved to find the range of values for that variable. The process is repeated for the other variables until a solution is determined.
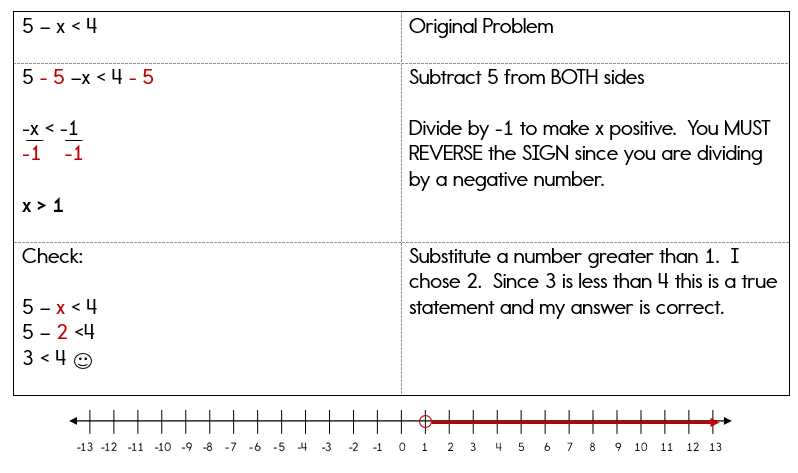
It is important to note that in algebraic methods, inequalities can be manipulated just like equations, as long as the same operation is applied to both sides of the inequality. However, it is essential to consider the direction of the inequality when performing these operations, as multiplying or dividing by a negative number will reverse the direction of the inequality.
By using these methods, systems of inequalities can be solved algebraically to find the range of values that satisfy both equations. This allows for a more precise solution and a deeper understanding of the relationship between the variables in the system.
Word Problems Involving Systems of Inequalities
Systems of inequalities are a powerful tool for solving word problems that involve multiple constraints or conditions. They allow us to represent and analyze situations where there are multiple inequalities simultaneously. In these types of problems, we are often interested in finding the range of values that satisfy all the given conditions.
One common type of word problem involving systems of inequalities is the “maximum/minimum” problem. These problems ask us to find the largest or smallest possible value of a certain quantity, subject to multiple constraints. For example, we may be asked to find the maximum possible profit for a company, given certain production and sales constraints.
To solve these types of problems, we first set up the inequalities that represent the given conditions. Each inequality represents a constraint, and the variables in the system represent the quantities we are trying to optimize. We then graph the system of inequalities on a coordinate plane, and find the region that satisfies all the conditions. The maximum or minimum value of the quantity we are interested in can be found by evaluating the function at the vertices of this region.
Another type of word problem involving systems of inequalities is the “feasibility” problem. In these problems, we are given a set of constraints and asked to determine if there exists a solution that satisfies all the conditions. For example, we may be asked if it is possible to allocate a certain amount of resources among different projects, given certain constraints on the availability of resources.
To solve feasibility problems, we set up the inequalities that represent the given conditions and graph the system on a coordinate plane. If the shaded region formed by the inequalities is non-empty, then there exists a solution that satisfies all the conditions. If the shaded region is empty, then no solution is possible.
Word problems involving systems of inequalities are common in many areas such as economics, engineering, and resource allocation. The ability to set up and solve these types of problems is an important skill for data analysis and decision making.
Graphing Linear Inequalities in Two Variables
Linear inequalities in two variables can be graphed on a coordinate plane to represent a region of possible solutions. The graph of a linear inequality in two variables is a shaded region that may include lines and/or curves. The region represents all the points that satisfy the inequality.
When graphing a linear inequality, it is important to remember the rules for shading the region. If the inequality has a greater than or less than symbol, the region is shaded with a dotted line. If the inequality has a greater than or equal to or less than or equal to symbol, the region is shaded with a solid line.
For example, consider the inequality y > 2x – 1. To graph this inequality, first plot the boundary line y = 2x – 1. Then choose a test point, such as (0,0), and substitute the coordinates into the inequality. If the inequality is true, shade the region containing the test point. If the inequality is false, shade the opposite region.
Graphing linear inequalities in two variables allows us to visually represent a range of solutions for a given situation. It provides a useful tool for analyzing relationships and making decisions based on the constraints of the inequality. Understanding how to graph these inequalities is an essential skill in algebra and can be applied to various real-world scenarios.
Solving Systems of Linear Equations and Inequalities
In mathematics, systems of linear equations and inequalities are a fundamental topic that involves finding the values of variables that satisfy multiple equations or inequalities simultaneously. These systems are often encountered in real-life scenarios, such as optimizing resources in business or solving engineering problems.
Solving systems of linear equations involves finding the values of variables that make each equation in the system true. This can be done through various methods, such as substitution, elimination, or using matrices. The goal is to find a solution that satisfies all the equations in the system.
Similarly, solving systems of linear inequalities involves finding the values of variables that satisfy multiple inequalities simultaneously. The solution to a system of inequalities is often represented as a shaded region on a graph, where all the points within the shaded region satisfy the given inequalities.
One important concept in solving systems of linear equations and inequalities is the idea of a feasible region. The feasible region is the set of all points that satisfy all the given equations or inequalities. This region can be determined graphically or algebraically.
To solve systems of inequalities, one can use techniques such as graphing, substitution, or elimination. Graphing involves plotting the inequalities on a coordinate plane and finding the overlapping shaded region that satisfies all the inequalities. Substitution and elimination methods involve manipulating the equations to solve for one variable and then substitute the value into the other equation or inequality.
Overall, solving systems of linear equations and inequalities is an important skill in mathematics and has various applications in real-life situations. Mastery of these concepts allows for efficient problem-solving and mathematical modeling in diverse fields.
Practice Problems and Answer Key
If you’re studying systems of inequalities and need some extra practice, you’ve come to the right place. Below, you’ll find a variety of practice problems that will help you solidify your understanding of this topic. Each problem is accompanied by a step-by-step solution, so you can check your work and see where you went wrong, if necessary.
1. Solve the following system of inequalities:
Problem:
- 2x + 3y ≤ 12
- x – 2y > 5
Solution:
In order to solve a system of inequalities, we need to find the region of the coordinate plane that satisfies both inequalities. We can start by graphing each inequality separately:
- For the inequality 2x + 3y ≤ 12, we can rewrite it in slope-intercept form as y ≤ -2/3x + 4. We can then graph this line and shade the region below it.
- For the inequality x – 2y > 5, we can rewrite it in slope-intercept form as y < 1/2x - 5/2. We can then graph this line and shade the region below it.
The region where the shaded areas overlap represents the solution to the system of inequalities. In this case, it is the shaded area below both lines. The solution to the system of inequalities is therefore:
- x – 2y > 5
- 2x + 3y ≤ 12
- x > 5
- y < -2/3x + 4
2. Solve the following system of inequalities:
Problem:
- 3x + y ≤ 6
- 2x – y > 4
Solution:
Using a similar process as before, we can graph each inequality and find their overlap. The solution to this system of inequalities is:
- 2x – y > 4
- 3x + y ≤ 6
- x > 2
- y ≤ -3x + 6
By practicing problems like these and checking your work with the provided solutions, you’ll become more confident in solving systems of inequalities. Remember to carefully graph each inequality and shade the appropriate regions to find the solution. Good luck!