Quadratic equations are an important topic in mathematics, and solving them can be challenging for students. However, with the help of technology and online resources, students now have access to various tools and projects that can assist them in finding answers to quadratic clue projects. Such projects often involve real-life scenarios, where students are given clues or information to solve quadratic equations. These projects not only help students practice their math skills but also enhance their critical thinking and problem-solving abilities.
One popular tool used by students to find answers to quadratic clue projects is online graphing calculators. These calculators allow students to plot the given clues on a graph and visualize the quadratic equation. By analyzing the graph, students can identify the key points and make educated guesses about the possible solutions. Another helpful resource is the Quadratic Formula, which provides a direct method for finding the roots of a quadratic equation. Students can plug in the given clues into the formula and calculate the solutions step by step.
Furthermore, there are numerous online platforms and forums where students can seek guidance and collaborate with peers on quadratic clue projects. These platforms not only provide answers to specific projects but also offer explanations and insights into the solving process. Students can share their thoughts, ask questions, and learn from others’ approaches, fostering a sense of community and collaboration in the learning process.
In conclusion, quadratic clue projects require students to think critically and apply their mathematical knowledge to real-life situations. With the help of technological tools, such as online graphing calculators and the Quadratic Formula, as well as online platforms and forums, students can find answers to these projects and enhance their problem-solving skills. These projects not only make learning quadratic equations more engaging but also promote collaboration and community among students.
Quadratic Clue Project Answers
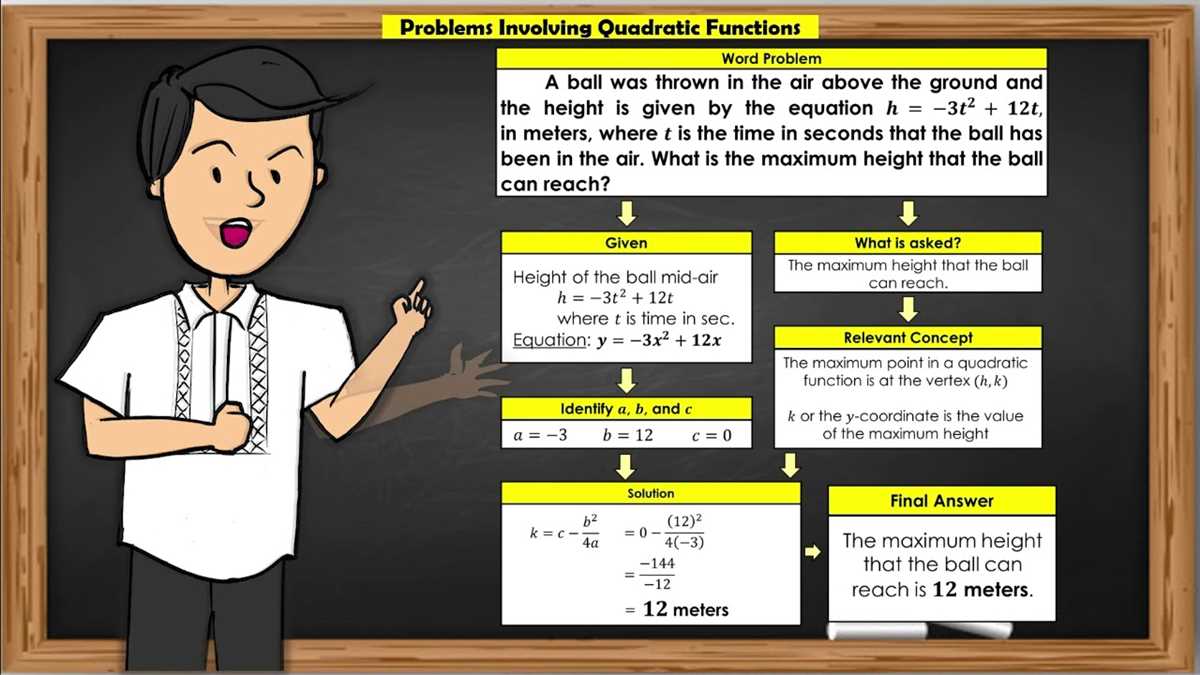
In the context of the Quadratic Clue Project, students are tasked with solving a set of clues to discover the answers to quadratic equations. This project is designed to engage students in the learning process and reinforce their understanding of quadratic functions and equations.
The clues provided in the project guide students through a series of steps, requiring them to use their knowledge of quadratic equations to find the solution. Each clue provides a piece of the puzzle, leading students closer to the final answer.
Students will need to use various techniques to solve the clues, such as factoring, completing the square, or using the quadratic formula. By applying these methods, students will be able to manipulate the equations and find the necessary values to solve each clue.
The Quadratic Clue Project not only allows students to practice their problem-solving skills, but also helps reinforce their understanding of quadratic equations and their applications. Students are able to see firsthand how quadratic equations can be used to model real-world situations and solve complex problems.
- Factoring: Students will need to factor the given quadratic equation to find its roots
- Completing the square: Students will be required to complete the square to transform the equation into vertex form
- Quadratic formula: Students may need to use the quadratic formula to find the solutions of the equation
Overall, the Quadratic Clue Project is a hands-on and engaging way for students to apply their knowledge of quadratic equations and strengthen their problem-solving abilities. It provides an opportunity for students to work independently or collaboratively, fostering critical thinking and teamwork skills.
Understanding the Quadratic Clue Project
The Quadratic Clue Project is a mathematical exercise that helps students understand the concepts and applications of quadratic equations. By solving a series of clues and puzzles, students are able to practice their problem-solving skills and reinforce their understanding of quadratic equations.
In the Quadratic Clue Project, students are given a set of clues and asked to find the values of unknown variables in a quadratic equation. They must use their knowledge of quadratic equations, including factoring, completing the square, and the quadratic formula, to solve the clues and find the solution to the puzzle.
The project is designed to engage students in a hands-on and interactive way, allowing them to apply their mathematical knowledge to real-world scenarios. By working through the clues and puzzles, students develop critical thinking skills and gain a deeper understanding of quadratic equations. The project also encourages collaboration and teamwork, as students can work together to solve the clues and share their problem-solving strategies.
The Quadratic Clue Project is a valuable tool for teachers to assess students’ understanding of quadratic equations. By observing their problem-solving process and evaluating their solutions, teachers can identify areas where students may need additional support and provide targeted instruction. The project also provides a fun and engaging way for students to learn and practice quadratic equations, making it an effective teaching tool.
Overall, the Quadratic Clue Project is an innovative and effective way to teach and reinforce the concepts of quadratic equations. It challenges students to think critically, apply their mathematical knowledge, and work collaboratively, all while having fun. Whether used as an individual assignment or a group activity, the project is a valuable tool for students to develop their problem-solving skills and deepen their understanding of quadratic equations.
How to Solve Quadratic Clue Problems
Quadratic clue problems often appear in math exams and require a systematic approach to find the solution. These types of problems involve quadratic equations, which are equations of the form ax^2 + bx + c = 0, where x represents the unknown variable and a, b, and c are constants. To solve these problems, it is important to carefully analyze the given clues and use the quadratic formula or factoring techniques.
1. Identify the given clues: Read the problem carefully and identify the relevant information. Look for keywords or phrases that indicate the presence of a quadratic equation, such as “squared,” “area,” or “vertex.”
2. Set up the equation: Once you have identified the relevant clues, write down the quadratic equation that represents the problem. Use the given information to determine the values of a, b, and c in the equation ax^2 + bx + c = 0.
3. Decide on the method: Depending on the nature of the problem, decide whether to use the quadratic formula or factoring to find the solution. Factoring is often preferred if the equation can be easily factored into two binomials, while the quadratic formula is a more general method.
4. Solve the equation: If factoring is chosen, factor the quadratic equation into two binomials and set each binomial equal to zero to find the values of x. If the quadratic formula is used, substitute the values of a, b, and c into the formula and simplify to find the solutions. Remember that quadratic equations can have zero, one, or two real solutions.
5. Check the solutions: After finding the solutions, substitute them back into the original equation to ensure they satisfy the problem’s conditions. This step is important to avoid extraneous solutions that may arise during the solving process.
By following these steps and practicing solving quadratic clue problems, you can enhance your problem-solving skills and improve your ability to tackle similar math problems in the future. Remember to approach each problem systematically and double-check your solutions to ensure accuracy.
Tips and Tricks for Finding Quadratic Clue Solutions

Quadratic clue projects can often be challenging, requiring a combination of mathematical skills, critical thinking, and problem-solving abilities. Here are some tips and tricks to help you find quadratic clue solutions more effectively:
1. Understand the Problem
Before diving into the calculations, make sure you fully understand the problem statement and the given clues. Identify the key information provided and take note of any restrictions or special conditions mentioned. This will help you set up the appropriate equations and focus on the right path towards finding a solution.
2. Utilize the Quadratic Formula
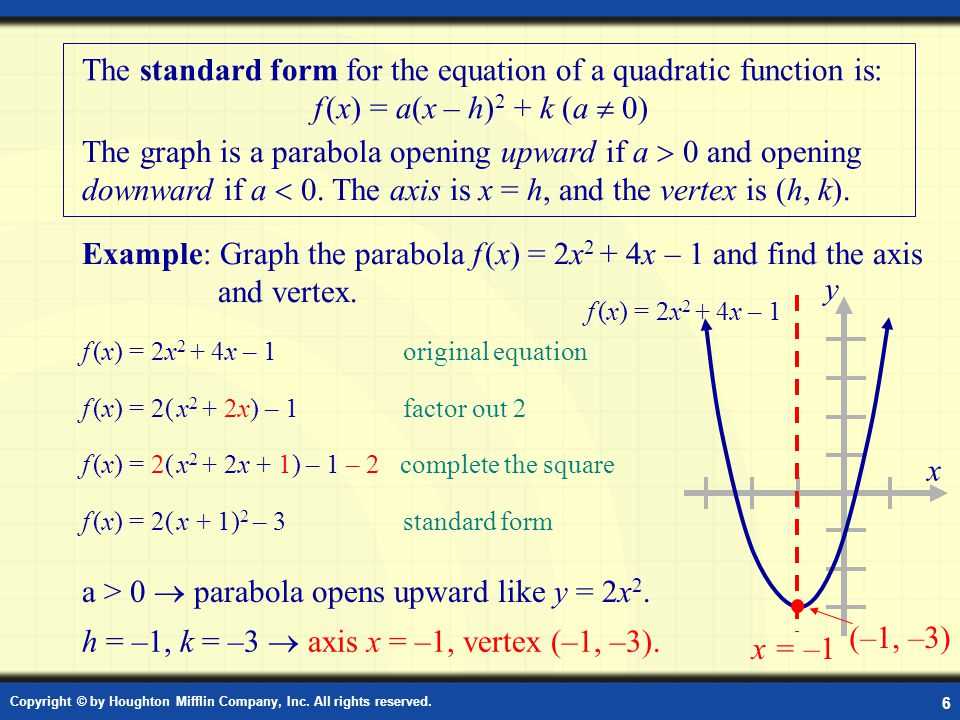
The quadratic formula is a powerful tool for solving quadratic equations. It states that for any equation in the form ax^2 + bx + c = 0, the solutions can be found using the formula:
x = (-b ± √(b^2 – 4ac)) / 2a
Make sure to correctly identify the values of a, b, and c in the given equation and substitute them into the formula to find the solutions. Keep in mind that a quadratic equation can have zero, one, or two real solutions.
3. Look for Patterns and Relationships

Quadratic clue projects often involve analyzing patterns and relationships between variables. Look for any recurring patterns or connections between different clues. Sometimes, graphing the given equations or creating a table of values can help visualize these relationships and make it easier to find the solutions.
4. Break Down the Problem
If the problem seems too complex at first, try breaking it down into smaller parts. Solve each part individually and then combine the solutions to find the final answer. Sometimes, tackling a problem step by step can make it more manageable and less overwhelming.
5. Analyze Extraneous Solutions
When solving quadratic equations, be aware of potential extraneous solutions. These are solutions that may satisfy the final equation, but not the original problem. Check each solution against the given clues to ensure they are valid. Discard any extraneous solutions that do not align with the problem’s constraints.
By following these tips and tricks, you can enhance your approach to quadratic clue projects and improve your chances of finding the correct solutions efficiently. Remember to practice and develop your problem-solving skills to become more adept at identifying and applying the appropriate techniques for each unique problem.
Common Mistakes to Avoid in the Quadratic Clue Project
When working on the Quadratic Clue Project, there are several common mistakes that students should be aware of in order to ensure accurate and successful results. These mistakes often lead to incorrect solutions and can hinder the understanding and application of quadratic equations. By avoiding these common pitfalls, students can enhance their understanding of the topic and improve their problem-solving skills.
1. Incorrect use of the quadratic formula:
One of the most common mistakes in the Quadratic Clue Project is the incorrect application of the quadratic formula. Students may forget to use the correct coefficients or fail to simplify their solutions. It is crucial to double-check the formula and plug in the correct values for a, b, and c in order to obtain accurate results. Additionally, simplifying the solutions to their simplest form is essential for the final answer.
2. Misinterpreting clue information:

Another common mistake in the project is misinterpreting the clue information given. Students may misread or misunderstand the information provided, leading to erroneous conclusions. It is important to carefully read and analyze the clues provided, making sure to understand the given context and any restrictions or conditions that may be implied. This will help ensure that the correct equations are set up and solved.
3. Incomplete or inaccurate labeling:
Properly labeling the variables and solutions is crucial in the Quadratic Clue Project. Students may omit or mislabel the variables, making it difficult to determine which values correspond to which parts of the problem. This can lead to confusion and incorrect answers. It is important to clearly label variables such as x, y, and any other relevant values, as well as labeling the final solutions to match the context of the problem.
4. Failure to check solutions:
Many students often forget to check their solutions in the Quadratic Clue Project. This can result in incorrect answers, as some solutions may not satisfy the original problem. It is important to substitute the obtained solutions back into the original equation and verify that they make sense in the given context. This step is crucial in ensuring the accuracy and validity of the solutions.
Avoiding these common mistakes in the Quadratic Clue Project will not only lead to accurate and correct answers, but it will also enhance the understanding and application of quadratic equations. By carefully applying the quadratic formula, interpreting clues accurately, labeling variables correctly, and checking solutions, students can confidently solve quadratic clue problems and strengthen their problem-solving skills in mathematics.
Exploring Real-Life Applications of Quadratic Clue Project
In the Quadratic Clue Project, students are challenged to use their knowledge of quadratic equations to solve a mystery. While this project is often used as a fun and engaging way to reinforce mathematical concepts in the classroom, it also has real-life applications that can be explored.
One real-life application of quadratic equations is in the field of physics. Quadratic equations can be used to model the motion of objects in free fall or in projectile motion. By analyzing the motion of these objects, scientists and engineers can make predictions about their trajectory and velocity. This information is crucial in fields such as aerospace engineering, where accurate calculations are needed to design and build aircraft.
Quadratic equations also have applications in the field of economics. For example, the cost and revenue functions of a business can often be modeled using quadratic equations. By finding the vertex of the quadratic function, economists can determine the optimal level of production or pricing that will maximize profits. This information is valuable for businesses looking to optimize their operations and maximize their financial performance.
In addition, quadratic equations are used in computer programming and graphics. Graphics software often uses quadratic equations to create smooth curves and shapes. By manipulating the coefficients of the quadratic equations, programmers can create visually appealing and realistic images. This application of quadratic equations highlights the intersection of mathematics and technology, showing how mathematical concepts are used in practical and creative ways.
- In physics, quadratic equations can be used to model the motion of objects in free fall or projectile motion.
- In economics, quadratic equations can be used to model cost and revenue functions and optimize business operations.
- In computer programming and graphics, quadratic equations are used to create smooth curves and shapes in graphics software.
Overall, the Quadratic Clue Project provides students with an opportunity to explore the real-life applications of quadratic equations. By solving the mystery using quadratic equations, students are able to see how these mathematical concepts are relevant in various fields and industries. This project not only reinforces their understanding of quadratic equations, but also showcases the practicality and versatility of mathematics in the real world.
Taking Your Quadratic Clue Skills to the Next Level
Now that you have learned the basics of solving quadratic equations and interpreting quadratic clues, it’s time to take your skills to the next level. With a solid foundation in quadratic clue solving, you can now apply your knowledge to more complex problems and real-world scenarios.
1. Challenge Yourself with Advanced Problems: Start by attempting more difficult quadratic clue problems. Look for clues that involve multiple variables or require you to solve for unknowns in different parts of the equation. This will help you sharpen your problem-solving skills and deepen your understanding of quadratic equations.
2. Explore Real-World Applications: Quadratic equations are not limited to abstract math problems. They have many practical applications in various fields, such as physics, engineering, and finance. Look for real-world scenarios where quadratic equations can be used to model and solve problems. This will not only help you see the relevance of quadratic clue skills but also broaden your understanding of how mathematics is applied in different contexts.
3. Collaborate and Discuss: Engage in discussions with fellow students or colleagues who are also interested in quadratic clue solving. Share your approaches, ask questions, and discuss different strategies. Collaborating with others can provide fresh perspectives and insights that can further enhance your skills.
4. Further Study: If you want to delve deeper into quadratic equations and their applications, consider studying advanced topics such as complex numbers, conic sections, or optimization. These areas will expand your understanding and enable you to tackle more complex problems in the future.
In conclusion, by continually challenging yourself, exploring real-world applications, collaborating with others, and furthering your study, you can take your quadratic clue skills to the next level. These skills not only help you excel in math but also develop problem-solving abilities that are valuable in various disciplines and real-life situations.