Understanding translations and reflections is an essential skill in mathematics. Whether you’re studying geometry or calculus, being able to accurately translate and reflect shapes and objects is vital for solving problems and understanding concepts. To reinforce your understanding of these concepts, many math teachers assign translation and reflection worksheet answers for practice.
A translation is a transformation that moves an object a certain distance while preserving its shape and orientation. In a translation, every point of the object’s original position is moved in the same direction and distance. It is important to accurately understand the direction and distance of the translation in order to correctly answer the worksheet questions.
A reflection, on the other hand, is a transformation that flips an object over a line. The line of reflection is often referred to as the “mirror line” and can be horizontal, vertical, or diagonal. Reflections create mirror images of the original object, and it is important to accurately identify the line of reflection to correctly answer the worksheet questions.
Translation and Reflection Worksheet Answers
In this article, we will provide you with the answers to a translation and reflection worksheet. This worksheet focuses on the basic concepts of translation and reflection in geometry, and tests your understanding of these concepts through a series of problems. By reviewing the answers to this worksheet, you can check your work and solidify your understanding of translation and reflection.
Translation Answers:
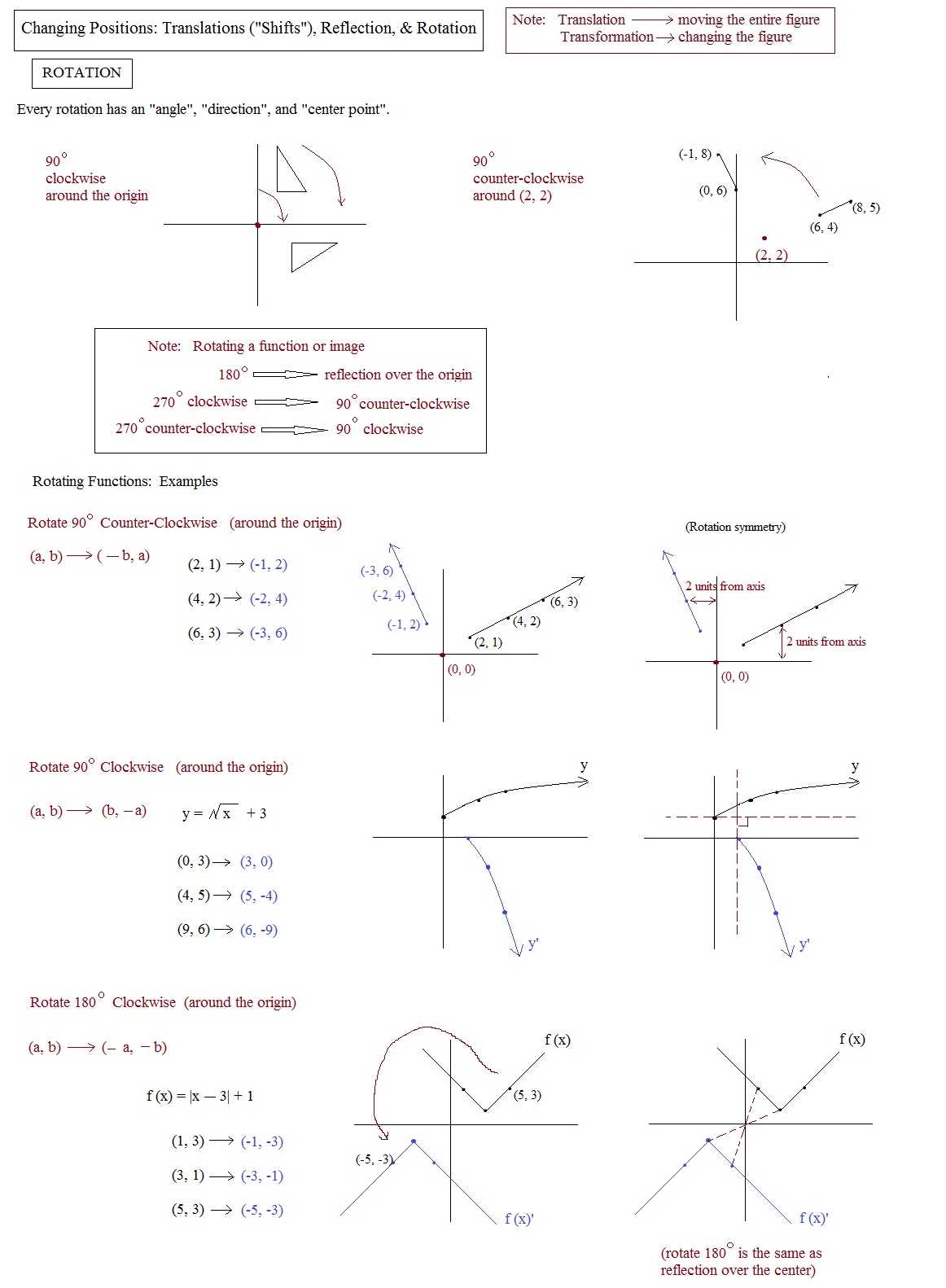
- Problem 1: The image of point A(2, 3) after a translation of 4 units to the right and 2 units down is A'(6, 1).
- Problem 2: The image of point B(-5, 7) after a translation of 3 units to the left and 5 units up is B'(-8, 12).
- Problem 3: The image of point C(0, -4) after a translation of 1 unit to the right and 3 units up is C'(1, -1).
Reflection Answers:
- Problem 1: The image of point D(3, 2) after a reflection over the x-axis is D(3, -2).
- Problem 2: The image of point E(-2, 4) after a reflection over the y-axis is E(2, 4).
- Problem 3: The image of point F(1, -5) after a reflection over the origin is F(-1, 5).
By going through these answers, you can compare your solutions and ensure that you have correctly applied the rules of translation and reflection in geometry. Understanding these concepts is essential for further studies in geometry and related fields, so it’s important to practice and master them.
Understanding Translation in Geometry
Translation is a fundamental concept in geometry that involves moving a shape or an object from one position to another without changing its shape or size. It is a type of transformation that preserves distances and angles, making it an important tool in solving geometric problems.
In translation, every point of the shape is moved the same distance and in the same direction. This movement is typically described using vector notation, where the vector represents the distance and direction of the translation. For example, if a shape is translated 3 units to the right and 2 units up, the vector notation would be (3,2).
When performing a translation, it is important to understand the properties that are preserved. The most significant property is that the shape and the size of the object remain unchanged. This means that the corresponding sides and angles of the original shape and the translated shape are congruent. Another important property is that parallel lines remain parallel after the translation.
Translations are commonly used in various areas of geometry, such as in mapping, navigation, and computer graphics. They are also used to simplify geometric problems by moving shapes to more convenient positions. By understanding the concept of translation and how it preserves certain properties, geometric problems can be approached and solved more effectively.
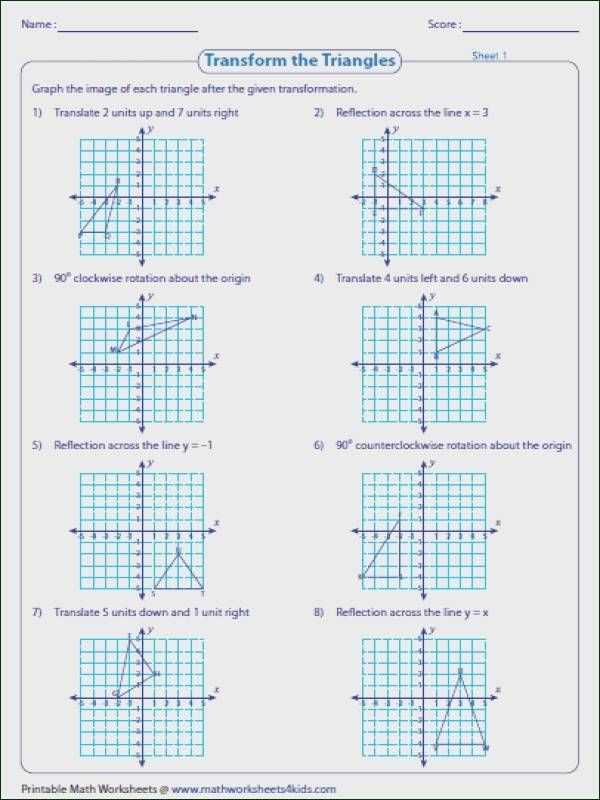
Step-by-Step Guide to Translating Figures
When it comes to translating figures, there are several steps you need to follow in order to accurately move the figure from one position to another. Whether you are translating a shape, an image, or a graph, these steps will help ensure that your translation is precise and consistent.
Step 1: Identify the figure you want to translate. Before you can begin the translation process, you need to determine which figure you want to move. This can be a shape, an image, or a graph, depending on the context. Once you have identified the figure, make sure you understand its dimensions and any specific features that need to be maintained during the translation.
Step 2: Determine the direction and distance of the translation. Next, you need to decide where you want to move the figure and how far you want to move it. This can be done by specifying the direction (up, down, left, or right) and the distance (in units) of the translation. It is important to be precise and consistent in your measurements to ensure an accurate translation.
Step 3: Apply the translation rule. Once you have determined the direction and distance of the translation, you can apply the translation rule to move the figure accordingly. The translation rule states that every point on the figure is moved the same distance in the specified direction. You can do this by adding or subtracting the same value from the x and y coordinates of each point on the figure.
Step 4: Verify the accuracy of the translation. After you have applied the translation rule, you should verify the accuracy of the translation by checking if the moved figure matches your intended position. You can do this by comparing the coordinates of the original figure and the translated figure. If they match, then the translation has been successful. If not, you may need to adjust your calculations and try again.
- Remember these steps whenever you need to translate figures. By following this step-by-step guide, you will be able to accurately move shapes, images, and graphs from one position to another.
- Practice translating different figures in order to improve your skills and confidence in applying these steps.
- Keep in mind that precision and consistency are key factors in achieving an accurate translation. Pay attention to details and double-check your calculations.
- Don’t be afraid to ask for help or seek additional resources if you are having trouble with a translation. Sometimes, a fresh perspective can make all the difference.
Common Mistakes to Avoid in Translations
Translation is an art that requires a deep understanding of both the source and target languages. Even experienced translators can make mistakes that can impact the accuracy and clarity of the translation. Here are some common mistakes to avoid:
- Lack of cultural knowledge: Translators should have a good understanding of the cultural nuances of both the source and target languages. Failure to consider cultural differences can lead to misinterpretations and misunderstandings in the translated text.
- Word-for-word translations: Translating word-for-word can result in awkward and unnatural phrasing. It is important to focus on conveying the meaning and intent of the text rather than sticking strictly to the literal translation.
- Ignoring context: Context plays a crucial role in translation. Translators should consider the overall context of the text, including the audience, purpose, and tone, to ensure the translation accurately captures the intended message.
- Overlooking idiomatic expressions: Idioms can be challenging to translate. Translators should be careful not to overlook or misinterpret idiomatic expressions, as they can significantly impact the meaning and effectiveness of the translation.
- Not proofreading: Proofreading is an essential step in the translation process. It helps identify and correct any errors, inconsistencies, or awkward phrasing in the translated text. Neglecting to proofread can result in a subpar translation.
Avoiding these common mistakes can help ensure accurate and high-quality translations. Translators should continuously strive to improve their skills and stay updated on language advancements and cultural changes to provide the best possible translations.
Exploring Reflection in Geometry
In the field of geometry, reflection is a fundamental concept that plays a crucial role in understanding the properties and transformations of shapes. Reflection is a transformation that is used to create a mirror image of an object across a line called the line of reflection. This concept can be visualized as folding a shape along the line of reflection, resulting in the two halves of the shape being perfectly aligned.
When exploring reflection in geometry, it is important to understand the characteristics and properties of the reflection of a shape. One key property of reflection is that the distance between each point and the line of reflection remains the same before and after the reflection. This means that for every point A on the original shape, there is a corresponding point A’ on the reflected shape that is equidistant from the line of reflection.
Geometric shapes can undergo reflection across different lines, including horizontal lines, vertical lines, and oblique lines. Reflection across a horizontal line results in a vertical flip of the object, while reflection across a vertical line results in a horizontal flip. Reflection across an oblique line creates a mirror image that is tilted at a certain angle.
Reflection is not only an important concept in geometry, but it also has practical applications in various fields. For example, in architecture, the concept of reflection is used to design buildings with symmetrical facades. In art, reflection is often used to create visually interesting compositions and illusions. Understanding reflection in geometry allows us to analyze and manipulate shapes in a systematic and logical manner.
Key Concepts:
- Reflection is a transformation that creates a mirror image of a shape across a line.
- The line of reflection is the line across which the shape is reflected.
- The distance between each point and the line of reflection remains the same before and after the reflection.
- Shapes can be reflected across horizontal, vertical, and oblique lines.
- Reflection has practical applications in fields such as architecture and art.
Techniques for Reflecting Figures
Reflecting figures is an important concept in geometry that involves creating a mirror image of a given figure. This can be done by using different techniques that allow us to accurately reflect the figure across a line or a point. These techniques include:
- Line Reflection: This technique involves reflecting a figure across a line called the line of reflection. To reflect a figure across a line, we simply need to draw a line parallel to the line of reflection, which is equidistant from each corresponding point of the original figure. This creates a mirror image of the original figure.
- Point Reflection: Point reflection is another technique used to reflect a figure. In this technique, the figure is reflected across a point called the center of reflection. To reflect a figure across a point, we simply need to draw lines connecting each point of the original figure to the center of reflection. The reflected figure will be created by extending these lines to the other side of the center of reflection.
- Coordinates: Reflecting figures can also be done using the coordinate system. In this technique, each point of the original figure is assigned coordinates. To reflect the figure across a line or a point, we can simply change the sign of the x-coordinate or the y-coordinate, or both, depending on the line or point of reflection. This allows us to easily determine the coordinates of the reflected figure.
These techniques for reflecting figures are essential in geometry as they help us understand the symmetrical properties of different shapes and figures. By mastering these techniques, we can accurately reflect figures and deduce important geometric properties of the original and reflected figures.
Solving Translation and Reflection Worksheet Problems – Conclusion
Mastering translation and reflection worksheet problems is essential for understanding and applying geometric transformations. By practicing these problems, students can develop their spatial reasoning skills and gain a deeper understanding of coordinates and transformations.
When solving translation problems, it’s important to remember that the coordinates of each point are shifted by a fixed distance in a given direction. To find the new coordinates, add or subtract the translation amounts provided in the problem to the original coordinates. It can be helpful to visualize the translation by drawing arrow vectors on a Coordinate Plane.
Reflection problems involve flipping an image or a figure across a line of reflection. To solve these problems, it’s crucial to identify the equation of the line of reflection and use it to find the new coordinates of the points. It can be helpful to draw the line of reflection and visualize the reflection process.
Overall, solving translation and reflection worksheet problems requires a combination of analytical and visualization skills. Students should practice these problems regularly to improve their understanding and proficiency in geometric transformations.