If you are a student studying mathematics, you are probably familiar with quadratic equations. These are equations of the form ax² + bx + c = 0, where a, b, and c are constants. Solving quadratic equations can be a tricky task, but it is an important skill to master. In this article, we will discuss the answer key for Unit 8 quadratic equations homework 3.
In Unit 8, students are typically introduced to different methods of solving quadratic equations, such as factoring, completing the square, and using the quadratic formula. Homework 3 is designed to give students practice in applying these methods to solve quadratic equations. The answer key provides the solutions to the problems given in the homework, allowing students to check their work and understand any mistakes they may have made.
By using the answer key for Unit 8 quadratic equations homework 3, students can verify whether they have correctly solved the quadratic equations. This helps them assess their understanding of the concepts and identify areas that need improvement. Additionally, the answer key can serve as a valuable resource for students who may be struggling with solving quadratic equations, as it provides step-by-step solutions that can guide them through the process.
Unit 8 Quadratic Equations Homework 3 Answer Key
Here is the answer key for Unit 8 Quadratic Equations Homework 3. This homework assignment focuses on solving quadratic equations using various methods such as factoring, completing the square, and using the quadratic formula.
1. Solve the following quadratic equations by factoring:
- Example 1: x^2 + 5x + 6 = 0
- Example 2: 2x^2 – 9x – 5 = 0
- Example 3: 4x^2 + 12x + 9 = 0
2. Solve the following quadratic equations by completing the square:
- Example 1: x^2 – 6x + 9 = 0
- Example 2: 2x^2 + 8x + 5 = 0
- Example 3: 3x^2 – 10x + 7 = 0
3. Solve the following quadratic equations using the quadratic formula:
- Example 1: x^2 + 4x – 5 = 0
- Example 2: 2x^2 + x – 3 = 0
- Example 3: 3x^2 – 7x + 2 = 0
Remember to check your solutions by substituting them back into the original equation. If the equation is satisfied, then the solution is correct. Make sure to show all work and clearly label your answers.
Explaining Quadratic Equations

A quadratic equation is a polynomial equation of the second degree. It has the form: ax^2 + bx + c = 0, where a, b, and c are coefficients, and x is the variable. The highest power of x in a quadratic equation is 2. Quadratic equations are widely used in various branches of mathematics, physics, and engineering.
Quadratic equations can have zero, one, or two real solutions. The number and nature of solutions can be determined by analyzing the discriminant, which is the value inside the square root in the quadratic formula. If the discriminant is positive, the equation has two distinct real solutions. If it is zero, the equation has one real solution. And if the discriminant is negative, the equation has no real solutions, but has two complex solutions.
There are several methods to solve quadratic equations, including factoring, completing the square, and using the quadratic formula. Factoring involves rewriting the equation as a product of two binomials. Completing the square involves adding a constant term to both sides of the equation to make it a perfect square trinomial. The quadratic formula can be used to solve any quadratic equation, regardless of whether it can be factored or completed. It provides a direct formula to find the solutions of a quadratic equation.
In summary, quadratic equations are polynomial equations of the second degree. They can have zero, one, or two real solutions, depending on the discriminant. Quadratic equations can be solved using various methods, such as factoring, completing the square, or using the quadratic formula. Understanding quadratic equations is essential in many areas of mathematics and science.
Solving Quadratic Equations Using Factoring
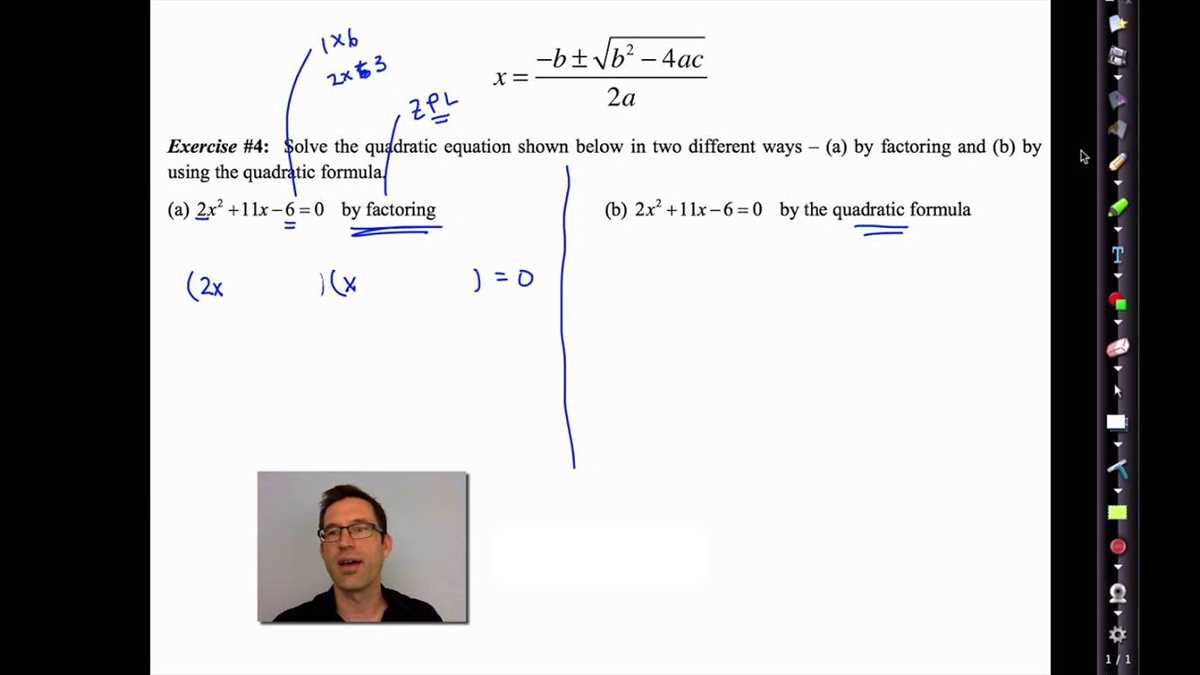
Factoring is one of the most common methods used to solve quadratic equations. It involves breaking down the quadratic equation into two binomial factors and setting each factor equal to zero to find the solutions.
When using factoring to solve a quadratic equation, the first step is to set the equation equal to zero. Then, we can look for two binomial factors that multiply together to give us the quadratic equation. To do this, we need to find the factors of the quadratic term and the constant term of the equation. Once we have identified the factors, we can set each factor equal to zero and solve for the variable to find the solutions of the quadratic equation.
- Example:
Let’s solve the quadratic equation x^2 + 5x + 6 = 0 using factoring. To factor this equation, we need to find two binomial factors that multiply to give us x^2 + 5x + 6. The factors of the quadratic term x^2 are (x)(x), and the factors of the constant term 6 are (1)(6) and (2)(3). After some trial and error, we find that the binomial factors of x^2 + 5x + 6 are (x + 2) and (x + 3). Setting each factor equal to zero, we have (x + 2) = 0 and (x + 3) = 0. Solving for x, we find the solutions x = -2 and x = -3.
Factoring is a powerful tool for solving quadratic equations, but it may not always be possible to factor an equation. In such cases, we can turn to alternative methods such as completing the square or using the quadratic formula to find the solutions.
Solving Quadratic Equations Using the Quadratic Formula
Quadratic equations are second-degree polynomial equations that have a standard form of ax^2 + bx + c = 0, where a, b, and c are constants. Solving these equations involves finding the values of x that make the equation true. One method to solve quadratic equations is by using the quadratic formula.
The quadratic formula is x = (-b ± √(b^2 – 4ac)) / 2a. This formula allows us to find the solutions or roots of a quadratic equation. By substituting the values of a, b, and c into the formula, we can calculate the two possible values of x. The ± symbol indicates that there are typically two solutions, one for the positive square root and one for the negative square root.
When using the quadratic formula, it’s important to remember the order of operations. First, we calculate the expression inside the square root, known as the discriminant, which determines the nature of the solutions. If the discriminant is positive, there are two distinct real solutions. If the discriminant is zero, there is one real solution. If the discriminant is negative, there are two complex solutions.
The quadratic formula provides a reliable method for solving quadratic equations, even in cases where factoring or completing the square is not possible or practical. By substituting the values of a, b, and c into the formula, we can effectively find the roots of any quadratic equation and solve for x.
Understanding Discriminant of Quadratic Equations
The discriminant can take on three possible values:
- If the discriminant is positive (D > 0), then the quadratic equation has two distinct real solutions. This means that the graph of the equation intersects the x-axis at two different points.
- If the discriminant is zero (D = 0), then the quadratic equation has one real solution. This means that the graph of the equation touches the x-axis at one point, which is also referred to as a double root.
- If the discriminant is negative (D < 0), then the quadratic equation has no real solutions. This means that the graph of the equation does not intersect the x-axis, and therefore, does not have any real roots.
The discriminant provides valuable information about the nature of the solutions to a quadratic equation. It helps us determine whether the equation has real solutions or not, and if it does, whether they are distinct or repeated. This information is important in various fields of mathematics and science, including algebra, geometry, physics, and engineering.
Applying Quadratic Equations in Real-Life Situations
In mathematics, quadratic equations play a critical role in modeling and solving real-life problems. These equations, which have the general form of ax^2 + bx + c = 0, can be used to find solutions in a variety of fields including physics, engineering, and economics.
One practical application of quadratic equations is in the field of projectile motion. When an object is thrown or launched into the air, its motion can be described using quadratic equations. By analyzing the initial velocity, angle of launch, and gravitational force, we can determine important information such as the maximum height reached, the time of flight, and the range of the projectile.
In engineering, quadratic equations are often used to model and optimize structures. For example, architects and engineers may use quadratic equations to determine the dimensions of an arch or a bridge in order to ensure structural stability. By considering factors such as load distribution, material strength, and architectural design, they can find the optimal dimensions that will minimize costs and maximize efficiency.
Quadratic equations also have applications in economics, particularly in the field of revenue and profit analysis. Businesses often use these equations to determine the price and quantity of a product that will maximize their profit. By considering factors such as production costs, demand elasticity, and market competition, they can find the optimal selling price and production level that will result in the highest profit.
In conclusion, quadratic equations are not just abstract mathematical concepts, but powerful tools that can be applied to solve real-life problems. Whether it’s analyzing projectile motion, designing structures, or making business decisions, understanding and applying quadratic equations can provide valuable insights and solutions in various fields.
Tips for Practicing and Mastering Quadratic Equations:

Quadratic equations can be challenging, but with the right practice and strategies, you can become proficient in solving them. Here are some tips to help you practice and master quadratic equations:
- Understand the basics: Before diving into complex quadratic equations, make sure you have a solid understanding of the basics. Familiarize yourself with the quadratic formula, discriminant, and different methods of solving quadratic equations.
- Practice regularly: Consistent practice is key to mastering quadratic equations. Set aside dedicated study time to solve various types of quadratic equations. The more you practice, the more comfortable you will become with different problem-solving strategies.
- Work through examples: Use textbooks, online resources, or practice problem sets to work through examples of quadratic equations. Start with simple equations and gradually progress to more complex ones. This will help you gain confidence and develop problem-solving skills.
- Create a study group: Collaborating with peers who are also studying quadratic equations can be beneficial. Discussing and solving problems together can help reinforce your understanding and provide different perspectives on problem-solving approaches.
- Seek help when needed: Don’t hesitate to ask for help if you’re struggling with quadratic equations. Consult your teacher, classmates, or online forums for clarification on concepts or specific problems. Getting assistance can save you time and help you grasp difficult concepts.
- Review and analyze your mistakes: When you make mistakes while solving quadratic equations, take the time to review and understand where you went wrong. Analyzing your mistakes can help you identify patterns, correct misconceptions, and improve your problem-solving skills.
- Explore real-world applications: Quadratic equations have numerous real-world applications, such as projectile motion, optimization problems, and financial calculations. Explore these applications to see how quadratic equations are used in different fields. This can make the subject more engaging and help you see the practical relevance of quadratic equations.
By following these tips, you can practice effectively and gain confidence in solving quadratic equations. Remember, consistent effort and perseverance are key to mastering any mathematical concept. Happy practicing!