
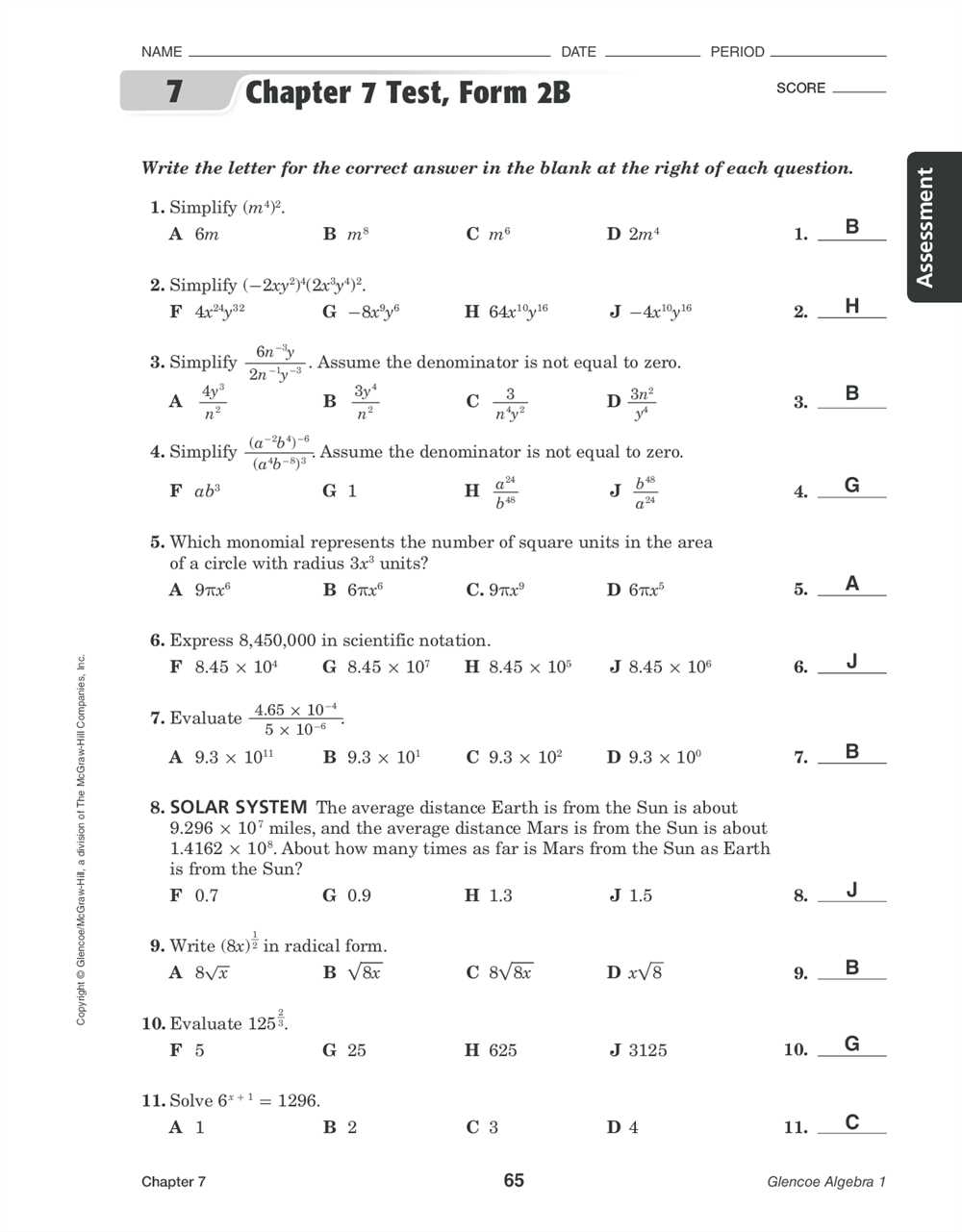
Algebra is a foundational topic in mathematics, and understanding how to solve algebraic equations is essential for success in higher level math courses. Glencoe Algebra 1 Chapter 4 provides students with a comprehensive exploration of equations and their solutions, helping to strengthen their problem-solving skills and build a solid algebraic foundation.
In this chapter, students will delve into linear equations and inequalities, learning how to manipulate and solve them using a variety of strategies. The Glencoe Algebra 1 Chapter 4 answer key serves as a valuable resource for checking their work and verifying the accuracy of their solutions.
By using the Glencoe Algebra 1 Chapter 4 answer key, students can gain a deeper understanding of the step-by-step process involved in solving equations. This key allows them to compare their own work with the correct answers, identifying any errors or misconceptions they may have. It serves as a valuable learning tool for review and reinforcement of key algebraic concepts.
With the Glencoe Algebra 1 Chapter 4 answer key, students can feel confident in their ability to solve equations and tackle more complex problems. By following the correct steps and understanding the underlying principles, they can unlock the secrets of algebraic equations and pave the way for continued success in their math journey.
Overview of Glencoe Algebra 1 Chapter 4
In Chapter 4 of Glencoe Algebra 1, students will learn about two important concepts in algebra: quadratic functions and equations, and exponential functions and equations. These topics are fundamental in understanding algebraic relationships and are building blocks for future mathematical concepts.
Quadratic Functions and Equations
A quadratic function is a function that can be written in the form y = ax^2 + bx + c, where a, b, and c are constants. In this chapter, students will explore how to graph quadratic functions, find the vertex of a parabola, and solve quadratic equations. They will also learn about different methods for factoring quadratic expressions and solving quadratic equations by using the quadratic formula.
Understanding quadratic functions and equations is crucial in various fields, such as physics, engineering, and computer science. This chapter will provide students with the necessary skills to analyze and solve real-world problems involving quadratic relationships.
Exponential Functions and Equations
An exponential function is a function of the form y = ab^x, where a and b are constants. In this chapter, students will explore exponential growth and decay, learn how to graph exponential functions, and solve exponential equations. They will also learn about the properties of exponential functions and how to use logarithmic functions to solve exponential equations.
Exponential functions play a significant role in many areas, including finance, population growth, and natural phenomena. By mastering the concepts in this chapter, students will gain the tools needed to analyze exponential relationships and make predictions based on real-world data.
Overall, Chapter 4 of Glencoe Algebra 1 provides a comprehensive overview of quadratic functions and exponential functions. By the end of this chapter, students will have a solid foundation in these important algebraic concepts, which will enable them to tackle more advanced topics in algebra and beyond.
Understanding Algebraic Expressions
Algebraic expressions are a fundamental concept in mathematics, and a key component of algebraic equations. Being able to understand and manipulate algebraic expressions is crucial for solving problems involving variables and unknown values.
At its core, an algebraic expression is a mathematical statement that combines numbers, variables, and mathematical operations such as addition, subtraction, multiplication, and division. These expressions can be as simple as a single variable or as complex as a combination of multiple variables and operations.
- Variables: Variables are symbols that represent unknown values. They are usually represented by letters, such as x or y. Variables allow us to solve equations and find specific values by assigning different values to them.
- Constants: Constants are fixed values that do not change. They are usually represented by numbers, such as 3 or 7.
- Coefficients: Coefficients are the numbers that multiply variables. They indicate how many times a variable appears in an expression. For example, in the expression 5x, 5 is the coefficient of the variable x.
- Like terms: Like terms are terms that have the same variables raised to the same powers. They can be combined using the rules of addition and subtraction. For example, in the expression 3x + 2x, the terms 3x and 2x are like terms and can be combined to give 5x.
Understanding algebraic expressions is essential for solving equations, simplifying expressions, and analyzing mathematical relationships. By mastering the basics of algebraic expressions, you will be better equipped to tackle more advanced concepts in algebra and other areas of mathematics.
Definition and Examples of Algebraic Expressions
An algebraic expression is a mathematical phrase that includes variables, numbers, and operations such as addition, subtraction, multiplication, and division. It represents a generalized rule or formula and allows us to solve problems or perform calculations in a more flexible way.
An algebraic expression consists of several components. The variables represent unknown values and are usually represented by letters, such as x or y. Numbers, also known as constants, are specific values that are known and do not change. Operations, such as addition (+), subtraction (-), multiplication (*), and division (/), are used to manipulate the variables and numbers in the expression.
For example, the expression 3x + 2y – 5 represents a linear combination of variables with coefficients. The coefficient of each variable (3 and 2) indicates the number of times the variable is multiplied. The constants (-5) remain unchanged throughout the expression. By substituting specific values for the variables, we can evaluate the expression and obtain a numerical result.
Algebraic expressions can also include exponents, which represent repeated multiplication. For instance, the expression 2x^2 – 3y^3 includes variables raised to the power of 2 and 3, respectively. These exponents indicate that the variables are multiplied by themselves a certain number of times.
In summary, algebraic expressions provide a way to represent mathematical relationships and formulas using variables, numbers, and operations. They allow us to generalize problems and solve them for various values of the variables. By understanding and manipulating algebraic expressions, we can solve complex equations, analyze patterns, and make predictions in various fields such as science, engineering, and finance.
Evaluating Algebraic Expressions

When working with algebra, it is important to be able to evaluate algebraic expressions. An algebraic expression is a mathematical statement that contains numbers, variables, and operations such as addition, subtraction, multiplication, and division. Evaluating an algebraic expression involves substituting values for the variables and then simplifying the expression using the specified operations.
In order to evaluate algebraic expressions, it is important to understand the order of operations. The order of operations is a set of rules that dictate the order in which mathematical operations should be performed. The acronym PEMDAS can help remember the order of operations: Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right).
Let’s consider an example of evaluating an algebraic expression. Suppose we have the expression 3x + 2y – 5z, and we are given that x = 2, y = 4, and z = 1. To evaluate this expression, we substitute the given values for x, y, and z into the expression:
- 3(2) + 2(4) – 5(1)
Next, we simplify the expression using the order of operations:
- 6 + 8 – 5
- 14 – 5
- 9
Therefore, when x = 2, y = 4, and z = 1, the value of the expression 3x + 2y – 5z is 9.
Evaluating algebraic expressions is a fundamental skill in algebra. It allows us to find the value of an expression for specific values of the variables. By understanding the order of operations and following a systematic approach, we can simplify complex expressions and solve algebraic problems.
Simplifying Algebraic Expressions

In algebra, simplifying expressions is the process of reducing a mathematical expression to its simplest form by combining like terms and applying the rules of arithmetic. This simplification helps to make the expression easier to work with and understand.
One common strategy for simplifying algebraic expressions is to combine like terms. Like terms are terms that have the same variable raised to the same power. For example, in the expression 3x + 5 + 2x, the terms 3x and 2x are like terms because they both have the variable x raised to the first power. To simplify this expression, we can combine these like terms to get 5x + 5.
Another strategy for simplifying algebraic expressions is to use the distributive property. This property allows us to multiply a term outside of parentheses by each term inside the parentheses. For example, in the expression 2(3x + 4), we can distribute the 2 to get 6x + 8. This makes the expression simpler by removing the parentheses.
Additionally, we can simplify algebraic expressions by combining like terms and then applying the rules of arithmetic. This involves performing operations such as addition, subtraction, multiplication, and division on the terms of the expression. By simplifying expressions in this way, we can often find the value of the expression or solve equations involving the expression.
In conclusion, simplifying algebraic expressions is an important skill in algebra. By combining like terms, applying the distributive property, and using arithmetic operations, we can simplify expressions and make them easier to work with. This simplification helps us to better understand and solve problems involving algebraic expressions.
Solving Equations
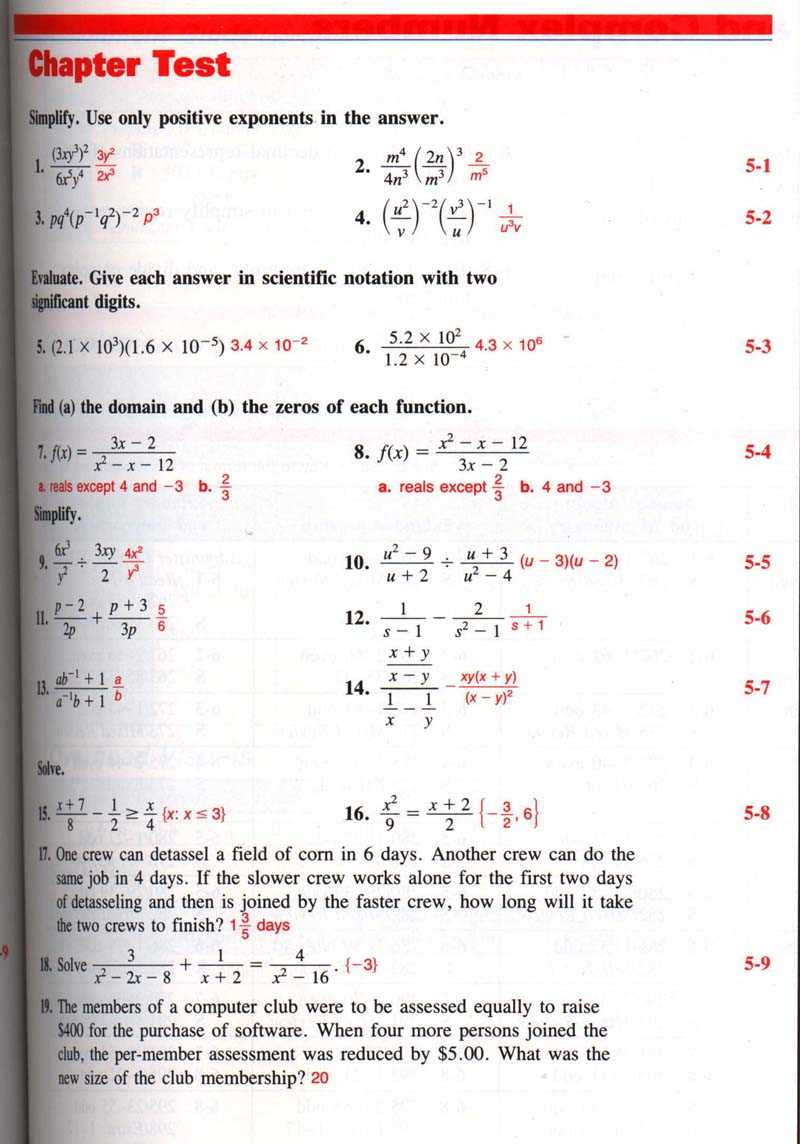
Solving equations is an essential skill in algebra. It is the process of finding the value of an unknown variable in an equation. By using various operations such as addition, subtraction, multiplication, and division, we can isolate the variable and determine its value.
One common method for solving equations is to start by simplifying both sides of the equation. This involves combining like terms and using the distributive property if necessary. Then, we can perform the same operation on both sides to isolate the variable. For example, if we have the equation 3x + 5 = 17, we can begin by subtracting 5 from both sides to get 3x = 12. Then, we can divide both sides by 3 to find that x = 4.
Sometimes, solving equations involves using inverse operations. For instance, if we have the equation 2x/3 = 4, we can multiply both sides by 3/2 to cancel out the fraction and find that x = 6. In other cases, we may need to use more than one step to solve an equation. This could involve combining like terms, distributing, and then isolating the variable.
- Solving equations requires understanding the properties of operations and how to apply them.
- Equations can have one solution, no solution, or infinitely many solutions.
- It is important to check the solution once we find it to ensure it is valid.
In conclusion, solving equations is a fundamental skill in algebra that allows us to find the value of an unknown variable. By using various operations and applying the properties of equality, we can manipulate equations to isolate the variable and determine its value. It is crucial to understand the steps involved in solving equations and to check the solution for validity.
Understanding Equations
Equations are fundamental tools in algebra that allow us to describe relationships between variables. They are used to solve problems involving unknown quantities and discover new information. By understanding equations, we can analyze and manipulate mathematical expressions to find solutions and make predictions.
Linear equations are equations in which the variable has an exponent of one and is raised to the power of one. They can be represented by a straight line on a graph, and their solutions often represent the intersection points of different lines. For example, the equation 2x + 3 = 7 can be solved to find that x = 2.
Quadratic equations are equations in which the variable is raised to the power of two. They can be represented by a parabola on a graph, and their solutions often represent the x-values where the parabola intersects the x-axis. For example, the equation x^2 + 4x – 5 = 0 can be solved to find that x = -5 or x = 1.
Equations can be solved using various methods, such as factoring, completing the square, or using the quadratic formula. It is important to understand the properties of equations, such as the distributive property and the law of exponents, to simplify and manipulate them effectively.
By understanding equations, we can solve real-world problems involving rates, distances, areas, and more. Equations are not only a mathematical concept; they are a powerful tool for problem-solving and critical thinking.
The Importance of Balancing Equations in Chemistry
In chemistry, balancing equations is an essential skill for understanding chemical reactions. Chemical equations describe the reactants and products involved in a reaction, and balancing them ensures that the number of atoms is conserved.
Chemical equations consist of chemical symbols and coefficients, with coefficients representing the number of atoms or molecules involved. Balancing equations involves adjusting the coefficients to ensure that the number of atoms of each element is the same on both sides of the equation.
For example, in the reaction 2H2 + O2 → 2H2O, the equation is unbalanced because there are four hydrogen atoms on the left side and only two on the right side. By adding a coefficient of 2 in front of H2O, the equation becomes balanced: 2H2 + O2 → 4H2O.
Understanding how to balance chemical equations is crucial for calculating the quantities of substances involved in a reaction and predicting the products obtained. It is a fundamental skill in chemistry that allows scientists to make accurate predictions and perform experiments.