
In this section, we explore the essential principles that form the core of mathematical reasoning in spatial calculations. Understanding these concepts is crucial for approaching challenges effectively. Mastery of the basic structures allows for successful problem-solving in a variety of contexts. Through practice, one can develop a clear approach to tackling mathematical problems efficiently.
Basic Principles in Spatial Relationships
To solve mathematical puzzles involving shapes and measurements, grasping the fundamental relationships between objects is essential. These relationships define how figures interact with each other, how their properties influence one another, and how they can be manipulated to reach desired results.
Angles and Their Properties
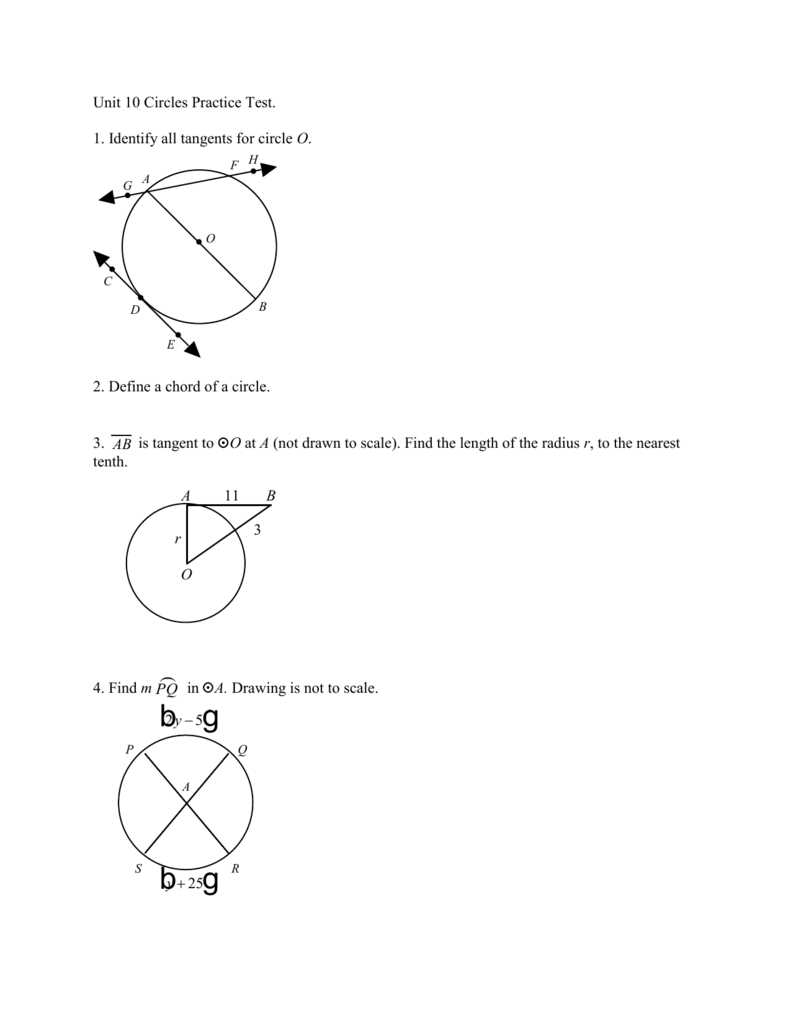
Understanding angles is key to many problems. The different types of angles, including acute, right, obtuse, and reflex, offer distinct insights into problem-solving. Correctly identifying and using these angles in calculations is a vital step in any related challenge.
Perimeter and Area Calculations
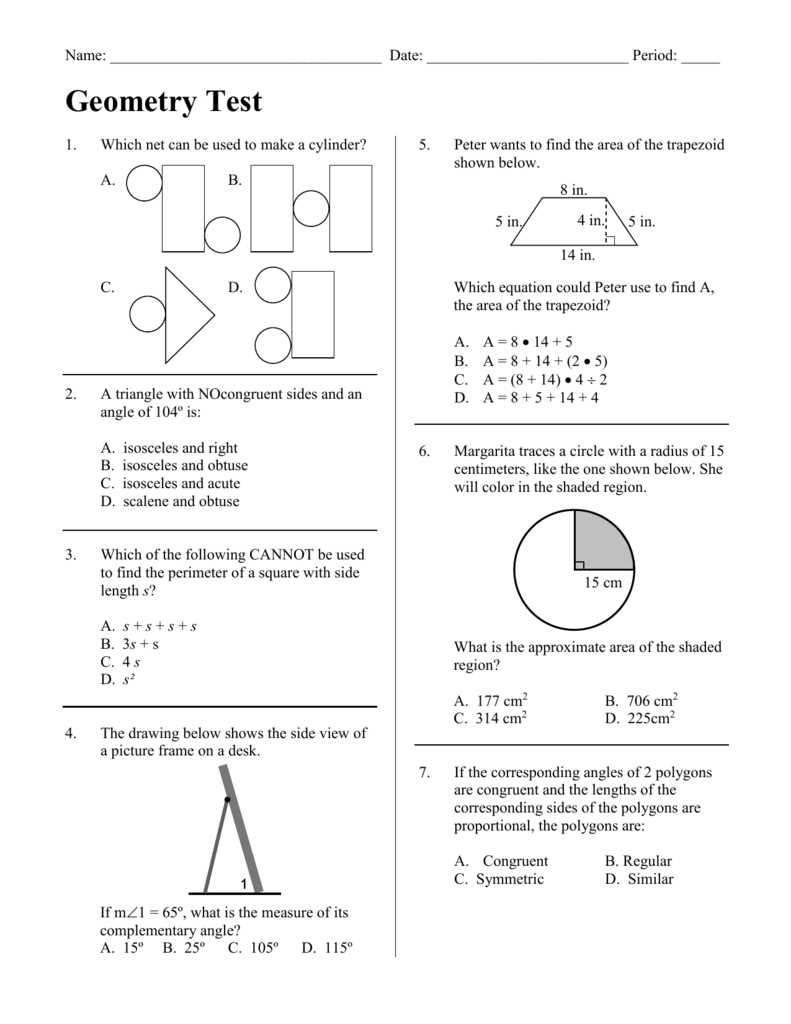
Perimeter and area are fundamental concepts when working with shapes. Calculating the perimeter gives insight into the boundary of an object, while area focuses on the space inside. Knowing the formulas for common shapes, such as rectangles and circles, is a powerful tool in solving many related problems.
Problem-Solving Techniques
There are various strategies to approach complex scenarios. One of the most effective methods is to break down larger problems into smaller, more manageable parts. Working step by step helps to minimize mistakes and ensures a clearer path to the solution.
Visualization and Diagram Drawing
Drawing diagrams or creating visual models can provide a clearer perspective of the problem. This simple method often reveals relationships between elements that may not be immediately obvious in the written form.
Practice and Repetition
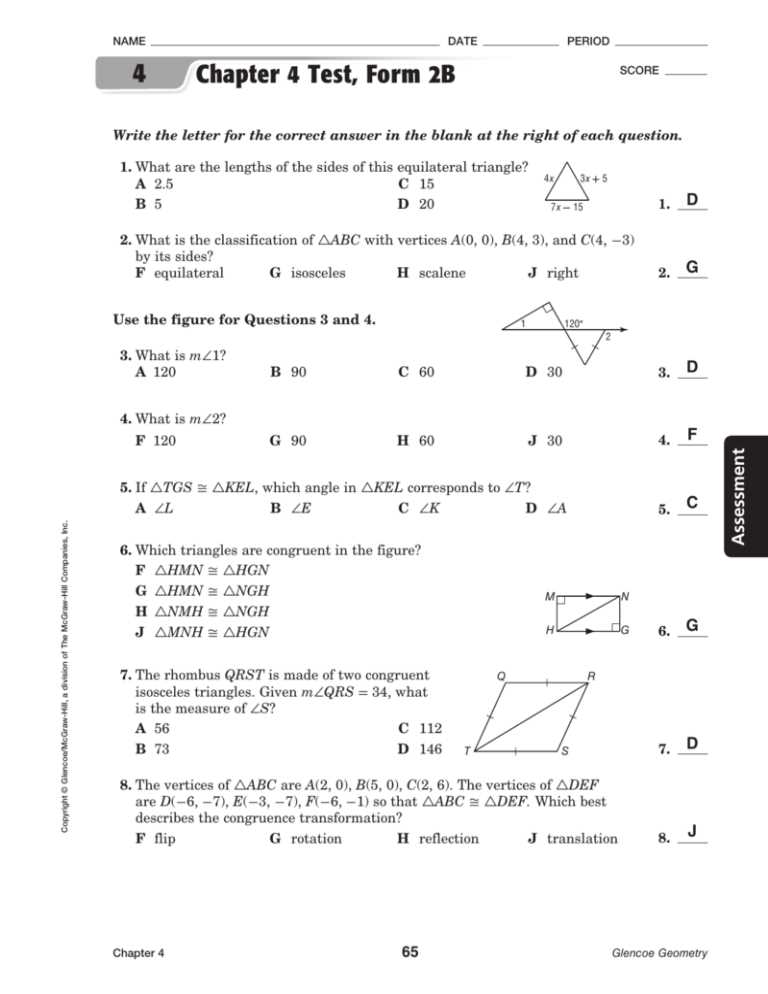
Continual practice is key to building confidence. By solving a range of problems, learners can reinforce their understanding and develop quicker, more accurate solutions over time.
Recommended Exercises for Improvement
- Work on problems involving angle calculations to improve accuracy.
- Practice perimeter and area calculations using a variety of shapes.
- Revisit challenging problems to find alternative methods of solving them.
By consistently engaging with exercises and applying the learned principles, anyone can master the key concepts that underpin this area of study.
Preparation Guide for Spatial Problem Solving
Key Ideas in Mathematical Structures
Crucial Theorems for Academic Achievement
Common Pitfalls in Spatial Assessments
Understanding Reasoning and Logical Frameworks
Approaches to Solving Spatial Challenges
Practice Problems for Mastery in Mathematics
Successfully tackling mathematical challenges requires a solid grasp of core principles and methods. With the right approach, anyone can master these concepts and apply them confidently. This section covers essential strategies, common mistakes, and effective problem-solving techniques that will help ensure success in academic assessments involving spatial reasoning.
To begin, understanding the core ideas is the first step. These include the relationships between various shapes, angles, and measurements, and how they are used to build solutions. By mastering these core concepts, students can approach any problem systematically and accurately.
One of the most important steps is recognizing the vital theorems that guide spatial reasoning. These theorems provide the foundational rules necessary for making logical conclusions and solving problems efficiently. Understanding them is essential for achieving success in any academic scenario.
It’s also important to be aware of the common errors that often arise in these types of assessments. Misunderstandings about the properties of shapes, incorrect calculations, and failure to apply theorems correctly are frequent pitfalls. Recognizing and avoiding these mistakes will save time and lead to more accurate results.
Another essential skill is developing a clear understanding of logical reasoning. Being able to follow a step-by-step process, make connections between ideas, and justify each decision is key to success in mathematical challenges. By developing logical frameworks, students can approach even the most difficult problems with confidence.
Problem-solving techniques play a major role in how efficiently solutions are reached. Breaking down complex problems into smaller, manageable tasks allows for more straightforward solutions. Practice with a variety of problems helps reinforce these skills, making the process quicker and more intuitive over time.
Finally, continual practice with exercises is vital for mastery. By working through numerous problems, students can strengthen their understanding of key concepts, recognize patterns, and improve their problem-solving skills. Consistent practice is the best way to ensure readiness for any academic challenges involving spatial reasoning.