Welcome to the answer key for Chapter 2: Measurements and Calculations. In this chapter, we will dive into the world of measurements and calculations, which are fundamental skills for any scientific discipline. Understanding how to measure and calculate accurately is crucial for conducting experiments, analyzing data, and drawing meaningful conclusions. In this answer key, you will find the solutions to the practice problems and exercises at the end of each section, helping you to reinforce your understanding of the material covered in this chapter.
In the first section, we will explore the basic concepts of measurements, including units, prefixes, and significant figures. Measurements are the foundation of scientific investigations, and understanding how to express them properly is essential for clear communication. We will also delve into the world of significant figures, which represent the precision of a measurement and help us avoid overestimating the accuracy of our calculations.
The second section focuses on the different types of calculations commonly used in scientific experiments. We will cover basic mathematical operations, such as addition, subtraction, multiplication, and division, and discuss how to apply them correctly using units and significant figures. Additionally, we will explore more complex calculations, such as solving proportions and converting between different units of measurement. By mastering these calculations, you will be equipped with the necessary tools to analyze and interpret experimental data accurately.
In conclusion, this answer key provides a comprehensive resource for reviewing and evaluating your understanding of Chapter 2: Measurements and Calculations. By practicing the problems and exercises included here, you will solidify your knowledge of the key concepts and ensure that you are on the right track to success in your scientific studies. So, let’s dive in and unlock the answers to your burning questions!
Chapter 2 Measurements and Calculations Answer Key
Measurements and calculations are essential components of chemistry and other scientific disciplines. In Chapter 2, you will find the answer key to the various questions and problems related to measurements and calculations. These answers will help you understand the concepts and techniques involved in solving problems in this field.
The chapter starts with a discussion on the importance of accurate measurements in science. It covers different units of measurement, such as the metric system, and provides examples on how to convert between different units. The answer key will guide you through these conversions and show you how to perform them correctly.
Furthermore, the answer key includes solutions to various calculation problems in the chapter. This includes problems related to significant figures, scientific notation, and dimensional analysis. You will learn how to determine the number of significant figures in a given measurement, how to perform calculations using numbers in scientific notation, and how to use dimensional analysis to solve complex problems.
The answer key also provides explanations for the different types of errors that can occur during measurements and calculations. It covers topics such as systematic errors, random errors, and how to minimize these errors to obtain accurate results. Understanding these errors is crucial for conducting precise experiments and obtaining reliable data.
In conclusion, the answer key to Chapter 2 Measurements and Calculations will help you master the concepts and techniques needed for accurate measurements and calculations in chemistry and other scientific disciplines. It will provide you with the necessary guidance to solve problems and understand the key principles involved in this field. Make sure to use the answer key as a reference and practice answering the questions and solving the problems on your own to improve your skills.
The Importance of Measurements in Science
Measurements play a crucial role in scientific research and understanding. Whether it’s in the fields of physics, chemistry, biology, or any other scientific discipline, accurate measurements are essential for gathering data, making observations, and drawing conclusions. In fact, measurements are the foundation on which scientific knowledge is built.
Accurate and precise measurements help scientists quantify and evaluate phenomena, allowing them to analyze and compare results. Without precise measurements, it would be difficult to determine if a particular variable or factor is significant or if it’s just a random occurrence. For example, in a chemical reaction, precise measurements of reactant quantities can help determine the exact stoichiometry of the reaction and predict the product yield.
Standard units of measurement, such as the metric system, provide a common language for scientists around the world. Consistent use of standard units ensures that measurements can be easily understood, replicated, and communicated. For instance, when conducting experiments, scientists need to record and report their findings using standardized units, such as grams, meters, or seconds, to ensure that their results can be accurately compared and interpreted by others.
Measurements also act as a basis for calculations in scientific equations, formulas, and theories. Many scientific laws, such as Newton’s laws of motion or Einstein’s theory of relativity, rely on accurate measurements for their formulation and verification. Without precise measurements, it would be impossible to accurately calculate and predict the behavior of objects and systems in the natural world.
Furthermore, measurements enable researchers to test hypotheses and validate theories. By taking measurements and gathering data, scientists can compare their results to predicted values or expected trends. If the measurements deviate significantly from the predicted values, it may indicate flaws in the theories or the need for further investigation. This iterative process of measurement, analysis, and refinement is crucial for advancing scientific knowledge and understanding.
In summary, measurements form the backbone of scientific research and discovery. They provide a means of quantifying and evaluating phenomena, establish a common language for communication and comparison, enable calculations and predictions, and facilitate the testing and validation of hypotheses and theories.
Units of Measurement and Conversion Factors
The field of measurement plays a crucial role in the scientific world, providing a framework for documenting and communicating precise quantities. In order to ensure accurate and consistent measurements, scientists rely on standardized units of measurement. These units are developed and maintained by international scientific organizations, such as the International System of Units (SI). By adhering to these standardized units, scientists can effectively communicate their findings and maintain consistency across different disciplines.
One of the fundamental concepts in measurement is conversion factors. Conversion factors allow for the conversion between different units of measurement, enabling scientists to express quantities in a variety of formats. Conversion factors are based on the relationships between different units, such as the number of inches in a foot or the number of grams in a kilogram.
For example:
- 1 inch = 2.54 centimeters
- 1 kilogram = 1000 grams
By using these conversion factors, scientists can easily convert measurements from one unit to another. This is particularly important when working with data or conducting experiments that involve multiple units of measurement. Conversion factors provide a bridge between different units, allowing scientists to seamlessly manipulate and analyze data in a meaningful way.
It is important to note that when using conversion factors, it is essential to maintain the correct relationships between units. This ensures that the converted measurements are accurate and reliable. Additionally, it is crucial to use appropriate significant figures when converting between units, as this preserves the precision of the measurement.
In summary, units of measurement and conversion factors are essential tools in the scientific world. They enable scientists to communicate precise quantities and convert between different units. By understanding and using these concepts, scientists can ensure accurate measurements and meaningful data analysis.
SI Units and Prefixes

The International System of Units (SI) is a metric system of measurement used in science, industry, and everyday life. It provides a standardized set of units for measuring length, mass, time, temperature, and many other physical quantities. The SI units are based on the metric system, which uses powers of 10 to define the relationship between different units.
One key feature of the SI system is the use of prefixes to denote multiples or fractions of a unit. These prefixes, such as kilo, mega, milli, micro, and nano, can be added to the base unit to indicate larger or smaller quantities. For example, a kilometer is equal to 1000 meters, while a milligram is equal to 0.001 grams.
The table below provides a list of some common SI prefixes and their corresponding factors:
| Prefix | Symbol | Factor |
|---|---|---|
| kilo- | k | 10^3 |
| mega- | M | 10^6 |
| milli- | m | 10^-3 |
| micro- | μ | 10^-6 |
| nano- | n | 10^-9 |
These prefixes can be combined with any SI unit to denote larger or smaller quantities. For example, a kilogram (kg) is equal to 1000 grams (g), and a megahertz (MHz) is equal to one million hertz (Hz).
The use of SI units and prefixes allows for easy conversion between different units and simplifies calculations. It provides a common language for scientists and engineers around the world to communicate and collaborate.
Using Measurement Tools and Techniques
Accurate measurements are essential in many fields, including science, engineering, and construction. To ensure accuracy, professionals use a variety of measurement tools and techniques. These tools allow for precise and consistent measurements, enabling accurate calculations and analysis.
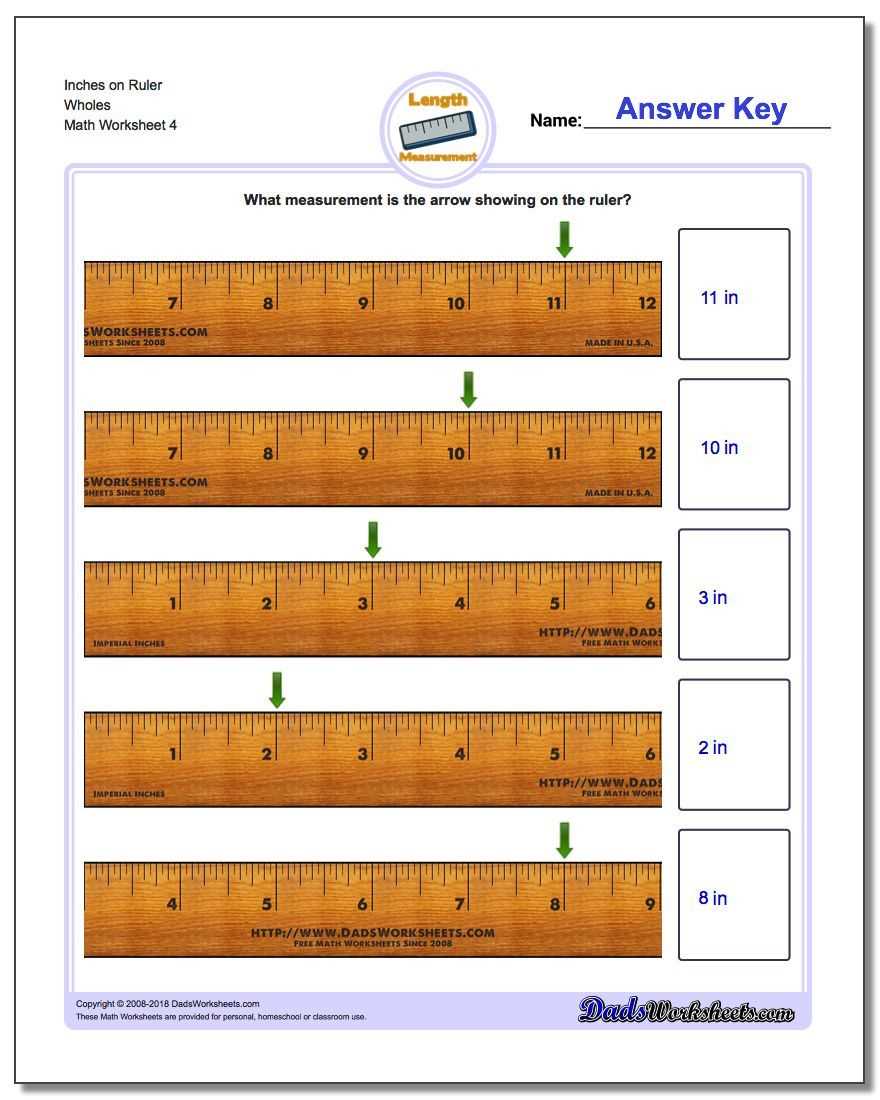
One commonly used measurement tool is the ruler or tape measure. A ruler is typically used for shorter distances, while a tape measure can be extended to measure longer lengths. These tools have markings or increments that allow for precise measurements in inches, centimeters, or millimeters. When using a ruler or tape measure, it is important to ensure it is aligned correctly and zeroed at the starting point to obtain accurate measurements.
For more complex measurements, professionals often turn to instruments such as calipers and micrometers. These tools provide incredibly precise measurements, down to a fraction of a millimeter. Calipers are typically used for outside measurements, while micrometers are used for internal measurements. They are commonly used in fields such as mechanical engineering, machining, and quality control.
In addition to physical measurement tools, there are also various techniques and methods for measuring. One such technique is the use of dimensional analysis, where physical quantities are expressed in terms of basic dimensions such as length, mass, and time. This approach allows for conversions between different units and simplifies complex calculations.
Overall, the use of measurement tools and techniques is vital for accurate and reliable measurements in various industries. Whether using rulers and tape measures for everyday measurements or advanced instruments like calipers and micrometers for precise engineering requirements, these tools enable professionals to obtain accurate data and make informed decisions based on measurements.
Significant Figures and Scientific Notation
Significant figures are a way to express the precision of a measurement or calculation. They represent the digits in a number that are known with certainty, plus one digit that is estimated or uncertain. The number of significant figures in a value is determined by the measuring device or the precision of the calculation.
In scientific notation, a number is expressed as the product of a coefficient and a power of 10. The coefficient is a number between 1 and 10 (inclusive), and the power of 10 represents the number of times the decimal point is moved to the right or left. Scientific notation is especially useful when working with very large or very small numbers, as it simplifies calculations and allows for easier comparison and representation of data.
When performing calculations with significant figures and scientific notation, it is important to follow certain rules to maintain the accuracy and precision of the final result. For addition and subtraction, the result should be rounded to the least precise decimal place. For multiplication and division, the result should be rounded to the same number of significant figures as the measurement with the fewest significant figures.
Understanding significant figures and scientific notation allows scientists and researchers to accurately represent and communicate data. It helps prevent errors and ensures the reliability of experimental results. By using these tools, scientists can maintain the integrity of their work and contribute to the advancement of knowledge in their respective fields.
Accuracy, Precision, and Error Analysis
In scientific measurements, it is essential to understand the concepts of accuracy, precision, and error analysis. Accuracy refers to the proximity of a measurement to the true or accepted value. In other words, an accurate measurement is one that is close to the actual value being measured. This can be determined by comparing the measurement to a known standard or by conducting multiple measurements and calculating the average. Accuracy is crucial in scientific research as it ensures the reliability and validity of the results.
Precision, on the other hand, refers to the consistency or reproducibility of a measurement. It is a measure of how close multiple measurements of the same quantity are to each other. High precision means that the measurements are tightly clustered, indicating low variability. Precision can be determined by calculating the standard deviation or the range of the measurements. Precision is important in scientific experiments because it allows for the detection of small changes or differences in the data.
Error analysis is the process of evaluating and quantifying the errors associated with a measurement. It involves identifying and understanding the sources of error and determining their impact on the overall measurement. Errors can be categorized as systematic or random. Systematic errors are consistent and predictable, resulting from flaws in the measurement apparatus or technique. Random errors, on the other hand, are unpredictable and occur randomly, leading to variability in the measurements. Error analysis helps to improve the accuracy and precision of measurements by identifying and minimizing the impact of errors.
To ensure accurate and precise measurements, scientists employ various techniques and tools, such as calibration, statistical analysis, and experimental controls. Calibration involves adjusting or verifying the accuracy of the measurement apparatus using known standards. Statistical analysis allows for the quantification of errors and the determination of confidence intervals. Experimental controls help to minimize the impact of external factors and ensure the precision of the measurements.
In conclusion, accuracy, precision, and error analysis are crucial concepts in scientific measurements. Accurate and precise measurements are essential for reliable and valid scientific research. Error analysis helps to identify and minimize errors, improving the accuracy and precision of measurements. By understanding and applying these concepts, scientists can ensure the quality and integrity of their data.