
Strengthening your understanding of basic math concepts is essential for mastering higher levels of study. Developing problem-solving skills and familiarizing yourself with various types of questions will build a solid foundation for future success. Regularly working on exercises and reviewing solutions will help reinforce learning and improve overall performance.
Key Concepts to Focus On
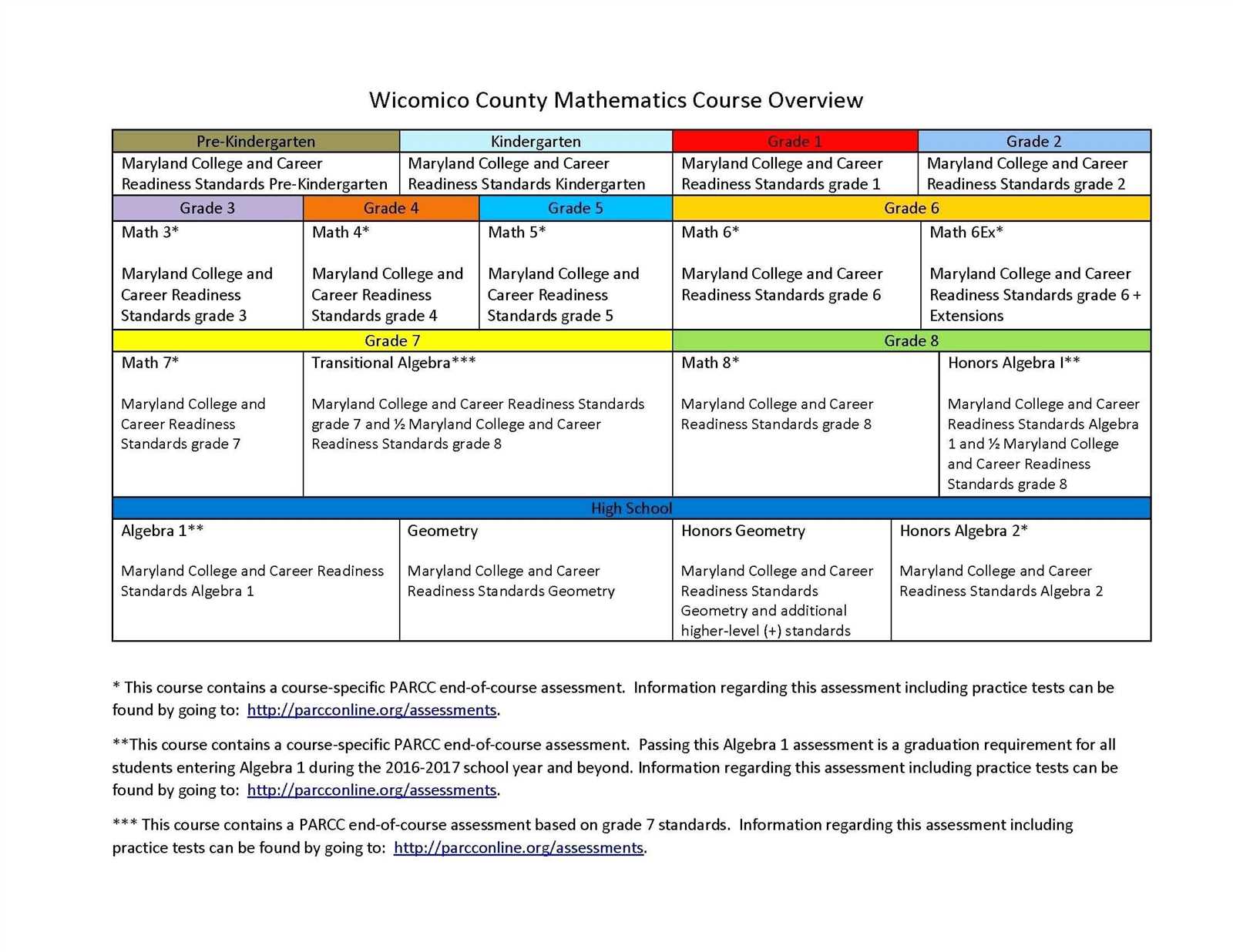
Focusing on core ideas is crucial for grasping complex problems. Some of the main topics include:
- Equation solving: Mastering the art of balancing equations with unknown variables.
- Operations with numbers: Understanding how to perform basic operations accurately.
- Patterns and sequences: Identifying numerical patterns and predicting future terms.
Approaching Complex Problems
When faced with a challenging question, it is important to break it down step by step. Here are some tips:
- Read the problem carefully and highlight the key information.
- Write down what you already know and what needs to be solved.
- Apply the correct methods based on the concepts you have studied.
How to Improve Your Problem-Solving Skills
Continuous practice is vital for improvement. Here are some strategies to enhance your abilities:
- Start with simpler problems to gradually build your confidence.
- Seek out different variations of similar problems to strengthen your understanding.
- Review your work to learn from mistakes and identify areas for growth.
Why Working Through Exercises Matters
Engaging with real-world examples and challenging questions helps deepen your understanding of concepts. The more you expose yourself to different types of problems, the better prepared you will be for future studies. Regular practice also builds your ability to solve problems under time pressure, a valuable skill in many areas of life.
Mathematical Problem Solving: Key Strategies for Success
Improving your ability to solve mathematical challenges requires a systematic approach. By focusing on fundamental ideas, developing effective strategies, and learning from mistakes, you can significantly enhance your problem-solving skills. This section covers the essential techniques that will help you tackle various problems with confidence and efficiency.
Understanding Essential Mathematical Concepts
Mastering the core concepts is crucial for progressing in any mathematical field. Key areas include solving for unknowns, understanding number relationships, and recognizing patterns. A solid grasp of these ideas forms the foundation for approaching more complex questions with ease.
Proven Strategies for Effective Preparation
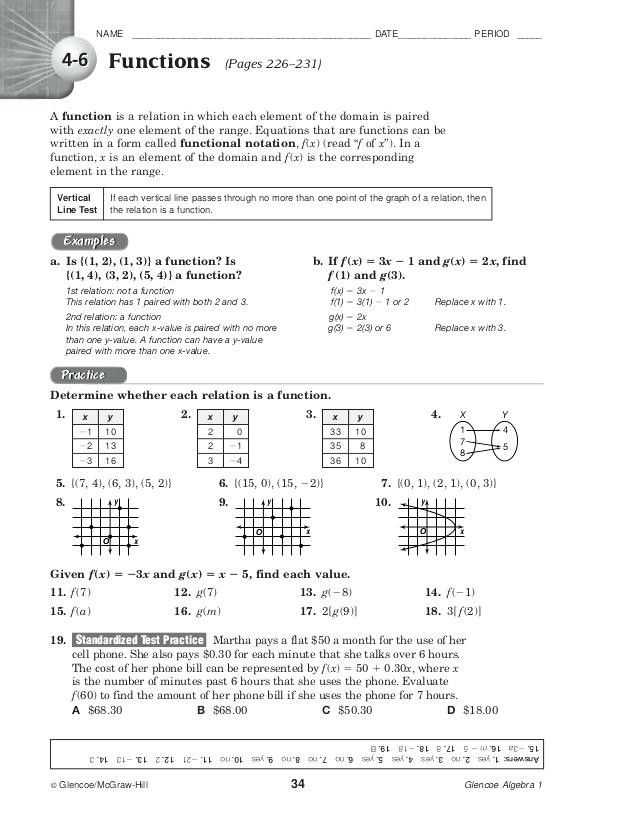
Preparation is the key to success. Focus on working through different types of problems to familiarize yourself with the concepts. Develop a study plan that includes reviewing solutions to identify where improvements can be made. Regular exposure to new questions will keep your skills sharp.
By practicing with real problems, you gain confidence and improve your ability to solve complex scenarios. Repetition helps reinforce concepts and enhances your mental agility, making it easier to work under pressure and in time-sensitive situations.
Common Pitfalls and How to Avoid Them
Many learners make common mistakes that can be avoided with careful attention. These include overlooking important details, misapplying formulas, and rushing through problems. Always take the time to double-check your work and follow the correct steps. Learning from your errors ensures continuous improvement.
Benefits of Engaging with Real-World Problems

Solving authentic challenges that reflect real-life applications can improve your problem-solving ability. Working through such exercises exposes you to different problem types and enhances your adaptability. The more varied your practice, the better you will perform when faced with similar tasks in the future.