
The answer key for the 1-2 Modeling Quantities exercise provides a comprehensive guide to help students understand and solve the problems given in the exercise. This answer key is an essential resource for teachers and students alike, as it provides step-by-step solutions and explanations for each problem.
Modeling quantities involves representing and analyzing real-life situations using mathematical models. By using mathematical models, students can understand and solve complex problems related to quantities, such as rates of change, percentages, and proportions.
The answer key for the 1-2 Modeling Quantities exercise covers various topics, including linear and exponential growth, unit conversions, data analysis, and problem-solving strategies. Students can refer to this answer key to check their answers, understand the steps involved in solving each problem, and identify any mistakes they may have made.
Furthermore, the answer key includes helpful tips and suggestions for problem-solving, allowing students to strengthen their mathematical thinking and reasoning skills. It also provides additional practice problems that reinforce the concepts covered in the exercise, ensuring a thorough understanding of modeling quantities.
2 Modeling Quantities Answer Key
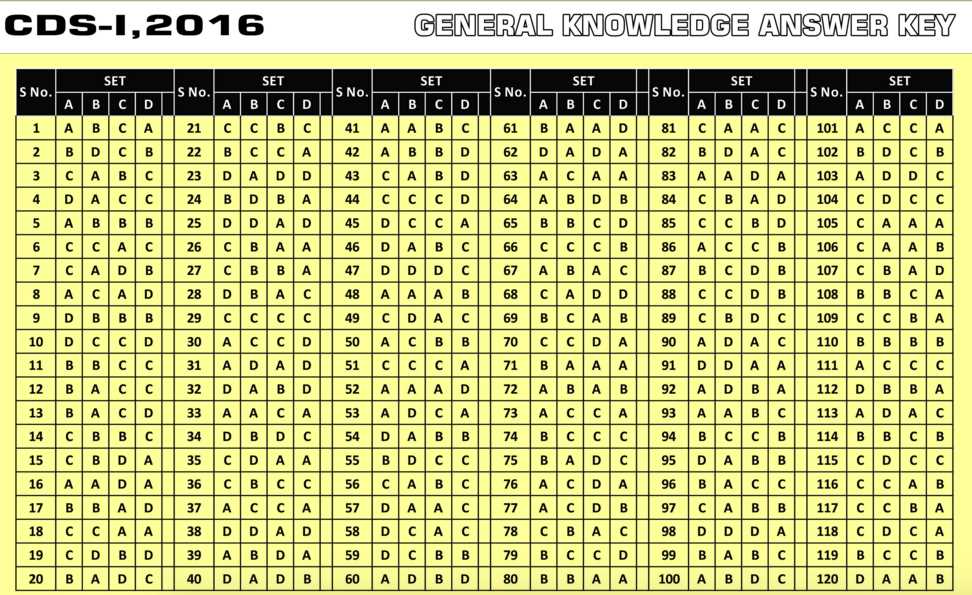
In the world of mathematics, modeling quantities is an essential skill. It helps us understand and analyze real-life situations by representing them in mathematical terms. The answer key to modeling quantities provides a valuable resource for students and teachers alike. It offers a comprehensive guide to solving problems and understanding the concepts behind them.
The answer key serves as a reference point for students to check their work and ensure that they are on the right track. It provides step-by-step solutions to the questions, allowing students to see where they went wrong and learn from their mistakes. The modeling quantities answer key also gives teachers a tool to assess their students’ understanding and progress.
Key Concepts:
- Conversion Factors: The answer key demonstrates the use of conversion factors, which are ratios that help convert one unit of measure to another. It shows how to set up and solve equations using these conversion factors to model quantities accurately.
- Variables and Formulas: The answer key showcases the importance of variables and formulas in modeling quantities. It provides examples of how to identify and use variables to represent unknown quantities and demonstrates the use of formulas to calculate desired values.
- Graphs and Tables: The answer key shows how to use graphs and tables to model quantities visually. It explains how to interpret data from these visual representations and use them to solve problems.
The modeling quantities answer key is a valuable tool that aids in learning and understanding mathematical concepts. It helps students build a solid foundation in modeling quantities and equips them with the skills needed to tackle complex real-world scenarios. By using and referring to the answer key, students can gain confidence in their problem-solving abilities and develop a deeper understanding of mathematical principles.
Understanding Modeling Quantities
Modeling quantities is an essential part of understanding and solving real-world problems. Quantities, or values, are often represented by variables and equations in mathematical modeling. By using mathematical models, we can analyze and predict different scenarios, such as population growth, economic trends, or physical phenomena.
When modeling quantities, it is crucial to define the variables and their relationships accurately. Each variable represents a specific value or attribute of the system we are studying. For example, in a population growth model, we may use variables for the initial population size, the growth rate, and the time elapsed. By expressing the relationships between these variables through equations, we can simulate and predict population growth over time.
In order to create an effective mathematical model for quantities, we must consider the following:
- The units of measurement for each variable: Using consistent units ensures that our model is accurate and meaningful. Different quantities may be measured in units such as meters, kilograms, gallons, or dollars.
- The assumptions and limitations of the model: Modeling quantities often requires simplifications and assumptions. It is important to acknowledge the limitations of the model and understand how they may affect the accuracy of the results.
- The validity and reliability of the data used: Models are only as good as the data they are based on. It is crucial to use reliable and relevant data to build and validate our models.
- The interpretation and communication of the results: Modeling quantities is not only about finding solutions but also about interpreting and communicating the results effectively. We must be able to explain the implications of our findings to others and make informed decisions based on the model’s predictions.
Overall, modeling quantities allows us to gain insights into complex systems and make informed decisions. It is a powerful tool that helps us understand and solve real-world problems, from predicting the spread of diseases to optimizing resources in business and engineering.
The Importance of Answer Key in Modeling Quantities
In the field of mathematics, modeling quantities is an essential skill that allows us to understand and predict real-world phenomena. Whether it’s analyzing population growth, predicting sales trends, or determining the trajectory of a projectile, modeling quantities provides valuable insights into the world around us. However, it is equally important to have an answer key that accurately represents the solutions to these models.
An answer key serves as a benchmark for evaluating the accuracy and correctness of our modeling efforts. It provides a reference point against which we can compare our own solutions, allowing us to identify and correct any errors or misconceptions we may have. Without an answer key, it becomes difficult to determine the validity of our models and the reliability of our predictions.
One of the key benefits of having an answer key is the ability to check our work and verify the solutions. It allows us to assess our understanding of the concepts and techniques used in modeling quantities. Through comparing our answers with those provided in the answer key, we can identify areas of improvement and strengthen our mathematical skills.
An answer key also plays a crucial role in the teaching and learning process. It provides educators with a valuable tool for assessing students’ understanding and progress. By using the answer key to grade assignments, teachers can identify areas where students are struggling and tailor their instruction accordingly.
Furthermore, an answer key can serve as a self-study resource for students. It allows them to practice solving problems and check their answers independently, helping them to develop their problem-solving skills and gain confidence in their abilities.
In summary, the answer key is an indispensable tool in modeling quantities, providing a reference point for evaluating the accuracy of our solutions and promoting a deeper understanding of mathematical concepts. Whether used by educators or students, having an answer key enhances the learning experience and enables us to make more informed decisions based on our models.
Key Concepts in Modeling Quantities
In the study of mathematical modeling, there are several key concepts that are crucial to understand. These concepts form the foundation for effectively representing and analyzing quantities in various real-world scenarios. By grasping these concepts, individuals can develop the skills necessary for making accurate predictions, solving problems, and making informed decisions.
1. Variables: A variable is a symbol that represents an unknown quantity. It is used to represent a changing or unknown value in a mathematical model. By assigning a variable to a quantity, we can express relationships and patterns in a mathematical form.
2. Functions: Functions are mathematical expressions that relate one variable to another. They describe how one quantity depends on another. By understanding the relationship between variables, we can create function models to represent various real-world phenomena.
3. Equations: Equations are mathematical statements that state the equality of two expressions. They can be used to represent relationships between quantities and solve for unknown values. Equations are essential in modeling quantities as they allow us to relate different variables and understand their interactions.
4. Graphs: Graphs are visual representations of mathematical relationships. They provide a way to analyze and interpret data by representing quantities on a coordinate plane. Graphs allow us to see patterns, trends, and relationships between variables, making them valuable tools in modeling quantities.
5. Units of Measurement: Units of measurement are essential in modeling quantities as they provide a standard for comparing and quantifying different quantities. By using consistent units, we can accurately represent and analyze quantities in mathematical models.
By understanding and applying these key concepts, individuals can develop a strong foundation in modeling quantities. These concepts provide the necessary tools for representing real-world phenomena mathematically, making predictions, and solving problems in various fields such as science, engineering, economics, and more.
Solving Problems Using Modeling Quantities
One of the key skills in mathematics is the ability to solve problems by modeling quantities. This involves taking a real-world situation and representing it mathematically in order to find a solution. By using mathematical models, we can analyze and understand complex situations, make predictions, and make informed decisions.
When solving problems using modeling quantities, it is essential to identify the relevant variables and their relationships. These variables can represent different aspects of the problem, such as time, distance, volume, or cost. By assigning mathematical symbols or equations to these variables, we can create a model that describes the problem.
For example, imagine you are planning a road trip and want to calculate the total cost of gas. You know that the price of gas per gallon is $2.50 and your car’s gas mileage is 30 miles per gallon. By using the equation cost = price per gallon * number of gallons, you can model the cost of gas as a function of the distance you plan to travel. This model allows you to calculate the cost based on different scenarios and make decisions accordingly.
In addition to creating the initial model, solving problems using modeling quantities often involves manipulating the equations to solve for unknown variables. This requires a strong understanding of algebraic principles and problem-solving techniques. By rearranging equations, substituting values, and simplifying expressions, we can find answers to our questions and verify the validity of our models.
In summary, solving problems using modeling quantities is a fundamental skill in mathematics. It allows us to represent real-world situations in a mathematical framework and find solutions through analysis and manipulation of equations. By mastering this skill, we can approach a wide range of problems with confidence and make informed decisions based on quantitative analysis.
Tips for Using the Answer Key in Modeling Quantities
When working on modeling quantities problems, it is important to use the answer key effectively to ensure accurate results. Here are some tips to help you make the most of the answer key:
- Review the provided answer key: Before you start solving the problem, take a moment to carefully read through the answer key. Understand the format and the expected solution, as it will help guide you in your approach.
- Break down the problem: Complex modeling quantity problems can often be overwhelming. Break them down into smaller, more manageable parts. Refer to the answer key to identify the key steps or strategies needed to solve each part.
- Compare your solution: Once you have solved the problem, compare your solution with the one provided in the answer key. Look for any discrepancies or errors. If your answer differs, review your work to identify where you may have made a mistake.
- Understand the reasoning: Besides the final answer, pay attention to the reasoning or explanation provided in the answer key. This will help you understand the conceptual aspects of the problem and improve your overall understanding of modeling quantities.
- Learn from your mistakes: If you find that your solution differs from the answer key, don’t get discouraged. Mistakes happen, and they provide valuable learning opportunities. Take the time to analyze your error and understand where you went wrong.
By following these tips, you can effectively use the answer key in modeling quantities to improve your problem-solving skills and deepen your understanding of the concepts involved.
Common Mistakes to Avoid in Modeling Quantities
When it comes to modeling quantities, there are several common mistakes that people often make. These errors can significantly impact the accuracy and reliability of the model, leading to incorrect predictions and analysis. It is essential to be aware of these mistakes and take steps to avoid them.
1. Oversimplifying the Model
One common mistake is oversimplifying the model by ignoring certain factors or assuming linear relationships. It is essential to consider all relevant variables and their potential non-linear relationships to accurately model quantities. Failing to do so can lead to inaccurate predictions and flawed analysis.
2. Incorrect Assumptions

Another common mistake is making incorrect assumptions about the underlying data and its behavior. It is crucial to gather and analyze sufficient data to validate the assumptions made in the model. Ignoring important factors or making unsupported assumptions can lead to biased results and inaccurate predictions.
3. Inadequate Data Quality
Modeling quantities requires high-quality data. Insufficient or unreliable data can lead to inaccurate models and flawed predictions. It is crucial to ensure that the data used in the model is complete, accurate, and representative of the phenomenon being modeled. Using insufficient or biased data can undermine the validity of the model and its predictions.
4. Lack of Validation
Model validation is an essential step in the modeling process. It involves comparing the model’s predictions with observed data to assess its accuracy and reliability. Failing to validate the model can lead to overfitting or underfitting, where the model either fits the training data too closely or does not capture the underlying patterns. Validation helps ensure that the model is robust and can be trusted for future predictions.
5. Ignoring Uncertainty
Modeling quantities involves dealing with uncertainty. Failing to account for uncertainty can lead to misleading results and unreliable predictions. It is essential to incorporate measures of uncertainty into the model and communicate them effectively to stakeholders. Ignoring uncertainty can lead to incorrect decisions and potential financial or operational risks.
Avoiding these common mistakes can greatly improve the accuracy and reliability of models used to represent quantities. By being mindful of oversimplification, incorrect assumptions, data quality, model validation, and uncertainty, practitioners can develop more robust and useful models for predicting and analyzing quantities.