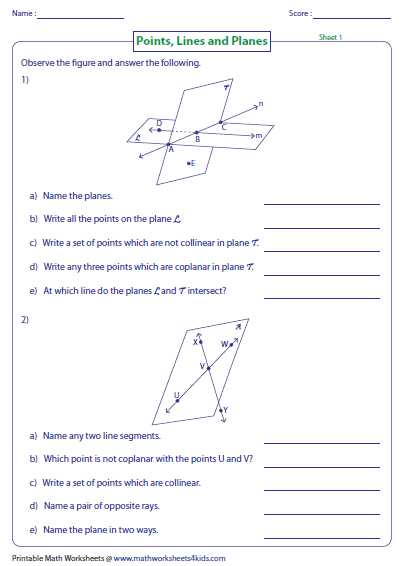
In mathematics, understanding line segments and distance is a fundamental skill. It serves as the basis for more complex concepts such as geometry and measurement. In this article, we will explore the answers to the skills practice exercises related to line segments and distance.
The exercises in the skills practice focused on various aspects of line segments and distance, including determining lengths of line segments, finding midpoints, and applying the distance formula. By going through the answers, readers will gain a better understanding of these concepts and their applications.
One of the main skills tested in the practice exercises is the ability to find the length of a line segment. This involves measuring the distance between two points on a line. The answers to these exercises will provide step-by-step explanations on how to calculate the length using the distance formula or by employing the Pythagorean theorem.
Moreover, the skills practice also includes exercises on finding the midpoint of a line segment. The midpoint is a point that divides the line segment into two equal halves. The answers will demonstrate the process of finding the coordinates of the midpoint using the given endpoints.
By examining the answers to the skills practice line segments and distance, readers will enhance their problem-solving abilities and deepen their understanding of these fundamental mathematical concepts. Mastery of these skills is crucial for success in higher-level mathematics courses and various real-world applications.
2 Skills Practice Line Segments and Distance Answers

In this exercise, we will be exploring line segments and distance. Line segments are portions of a line that are bounded by two endpoints. The distance between two points can be calculated using the Pythagorean theorem. This exercise will provide answers to the practice questions related to line segments and distance.
To find the distance between two points (x1, y1) and (x2, y2), we can use the formula:
Distance = √[(x2 – x1)^2 + (y2 – y1)^2]
Let’s look at an example:
- Point A is located at (-2, 3).
- Point B is located at (4, -1).
To find the distance between point A and point B, we can substitute the values into the formula:
Distance = √[(4 – (-2))^2 + (-1 – 3)^2] = √[6^2 + (-4)^2] = √[36 + 16] = √52 ≈ 7.21
So, the distance between point A and point B is approximately 7.21 units. This distance represents the length of the line segment connecting the two points.
Section 1: Understanding Line Segments
A line segment is a part of a line that has two endpoints. It is a straight path that connects these two points, and has a definite length. In geometry, line segments are represented by a short line with a bar above it, indicating the two endpoints.
When studying line segments, it is important to understand their basic properties and how to measure their lengths. To measure the length of a line segment, you can use a ruler or a measuring tape. Start at one endpoint and align the 0 mark with it, then read the measurement at the other endpoint. This will give you the length of the line segment in the unit of measurement being used.
Line segments can be categorized based on their lengths. If a line segment has the same length as another line segment, they are considered congruent. On the other hand, if two line segments have different lengths, they are categorized as unequal or non-congruent. It is also possible for line segments to have zero length, which means they are coincident and occupy the same position on a line.
Key Terms:
- Line segment: a part of a line with two endpoints.
- Endpoint: the point at which a line segment starts or ends.
- Congruent: line segments with the same length.
- Non-congruent: line segments with different lengths.
- Coincident: line segments with zero length that occupy the same position on a line.
Understanding line segments is fundamental in geometry as they form the building blocks for more complex geometric shapes. Their properties and measurements are essential in solving problems involving lengths, angles, and shapes. By grasping the concepts and terminology associated with line segments, students can develop a solid foundation in geometry and apply their understanding to various mathematical and real-world situations.
Section 2: Identifying Endpoints of a Line Segment
In geometry, a line segment is a part of a line that is bounded by two distinct endpoints. Identifying these endpoints is crucial for accurately representing and working with line segments. By determining the beginning and ending points of a line segment, we can accurately measure its length and manipulate it in various geometric operations.
To identify the endpoints of a line segment, we can use different methods depending on the given information. One common approach is to look for any labeled points on the line segment, as these are likely to be the endpoints. Another method is to evaluate the given coordinates or measurements of the line segment to determine where it starts and where it ends.
Example:
Given a line segment AB, we can determine its endpoints by examining the information provided. If point A is labeled on the line segment and point B is not, we can conclude that A is one endpoint and B is the other. Alternatively, if the coordinates of A and B are given, we can compare their values to determine which point represents the starting and ending points of the line segment.
Identifying the endpoints of a line segment allows us to accurately represent and manipulate geometric figures. Whether it is for measuring lengths, constructing other shapes, or solving mathematical problems, knowing the endpoints of a line segment is fundamental in geometry.
Section 3: Finding the Length of a Line Segment
In geometry, finding the length of a line segment is an essential skill. The length of a line segment can be calculated using various methods, but one of the most common approaches is using the distance formula. This formula utilizes the coordinates of the endpoints of the line segment to determine its length. The distance formula is derived from the Pythagorean theorem, which states that in a right triangle, the sum of the squares of the lengths of the two shorter sides is equal to the square of the length of the hypotenuse.
To apply the distance formula, the first step is to identify the coordinates of the endpoints of the line segment. Let’s consider an example where the endpoints are (x1, y1) and (x2, y2). Once the coordinates are known, the distance formula is simply:
d = √[(x2 – x1)^2 + (y2 – y1)^2]
This formula calculates the distance between the two endpoints of the line segment. By substituting the values of the coordinates into the formula, the length of the line segment can be determined. It is important to note that the result will always be a positive value, as distance is a scalar quantity and cannot be negative.
Finding the length of a line segment is a fundamental skill in geometry and has various practical applications. It is used in various fields such as architecture, engineering, and physics to measure distances and sizes accurately. By understanding and practicing the distance formula, individuals can enhance their spatial reasoning and problem-solving abilities.
Section 4: Applying the Distance Formula
The distance formula is a fundamental tool in geometry that allows us to find the distance between two points in a coordinate plane. It is derived from the Pythagorean theorem and can be applied to various real-life scenarios. In this section, we will explore how to use the distance formula to solve practical problems.
Example 1:
Suppose we have two points A(2, 3) and B(5, -1) in a coordinate plane. To find the distance between these two points, we can use the distance formula:
d = sqrt((x2 – x1)^2 + (y2 – y1)^2)
Substituting the values of the coordinates from points A and B, we have:
d = sqrt((5 – 2)^2 + (-1 – 3)^2)
d = sqrt(3^2 + (-4)^2)
d = sqrt(9 + 16)
d = sqrt(25)
d = 5
Thus, the distance between points A(2, 3) and B(5, -1) is 5 units.
Example 2:
Consider a scenario where a person is planning to walk from their house to a grocery store. The person’s house is located at point H(3, 6) and the grocery store is located at point G(9, 2). To find the distance the person needs to walk, we again use the distance formula:
d = sqrt((x2 – x1)^2 + (y2 – y1)^2)
Substituting the values of the coordinates from points H and G, we have:
d = sqrt((9 – 3)^2 + (2 – 6)^2)
d = sqrt(6^2 + (-4)^2)
d = sqrt(36 + 16)
d = sqrt(52)
d ≈ 7.211
The person needs to walk approximately 7.211 units from their house to the grocery store.
By applying the distance formula, we can accurately calculate the distance between two points in a coordinate plane. This mathematical tool has wide applications in various fields, including navigation, surveying, and engineering.
Solving Problems Involving Line Segments
In this section, we will explore different techniques for solving problems involving line segments. Line segments are one-dimensional figures that consist of two endpoints and all the points between them. They can be horizontal, vertical, or diagonal, and can intersect with other line segments or other shapes.
One important concept when working with line segments is distance. The distance between two points on a line segment is the length of the segment that connects them. We can calculate the distance using the Pythagorean theorem or by subtracting the coordinates of the endpoints. This concept is essential when calculating the perimeter of a shape or finding the length of a line segment.
In order to solve problems involving line segments, we need to have a clear understanding of the given information and what needs to be found. We can then use different mathematical strategies, such as algebraic equations or geometric properties, to solve the problem.
For example, if we are given the lengths of two line segments and we need to find the length of a third segment that forms a right triangle with the other two, we can use the Pythagorean theorem to solve for the missing length. On the other hand, if we are given the coordinates of two endpoints and we need to find the equation of the line segment, we can use the slope-intercept form or the point-slope form.
It is important to practice different problems involving line segments to strengthen our problem-solving skills and develop a deeper understanding of the concepts. By applying mathematical principles and using logical reasoning, we can successfully solve problems and find solutions in various real-life situations.
Section 6: Understanding Distance on a Coordinate Plane

In this section, we will explore the concept of distance on a coordinate plane. A coordinate plane is a two-dimensional grid that consists of a horizontal x-axis and a vertical y-axis. Each point on the plane is identified by its coordinates, which are represented as (x, y). To calculate the distance between two points on the coordinate plane, we can use the distance formula, which is derived from the Pythagorean theorem.
The distance formula states that the distance between two points (x1, y1) and (x2, y2) can be found by taking the square root of the sum of the squared differences of their x-coordinates and y-coordinates. Mathematically, this can be expressed as:
d = √((x2 – x1)² + (y2 – y1)²)
To illustrate this concept, let’s consider an example. Suppose we have two points A and B on the coordinate plane, with coordinates A(3, 4) and B(7, 8). To find the distance between these two points, we can substitute the given coordinates into the distance formula:
d = √((7 – 3)² + (8 – 4)²)
d = √(4² + 4²)
d = √(16 + 16)
d = √32
d ≈ 5.66
Therefore, the distance between points A and B is approximately 5.66 units on the coordinate plane.
This understanding of distance on a coordinate plane is crucial in various mathematical applications, such as calculating the length of line segments, determining the shortest distance between two points, and solving problems involving geometric shapes.
Section 7: Finding the Midpoint of a Line Segment
When working with line segments, it is often useful to find the midpoint. The midpoint is the point on the line segment that is equidistant from both endpoints. It is located exactly halfway between the two endpoints.
To find the midpoint of a line segment, you can use the midpoint formula. The formula states that the x-coordinate of the midpoint is the average of the x-coordinates of the endpoints, and the y-coordinate of the midpoint is the average of the y-coordinates of the endpoints. This can be represented as:
Midpoint: ( (x₁ + x₂)/2 , (y₁ + y₂)/2 )
For example, if we have a line segment with endpoints at (1, 4) and (7, 10), we can find the midpoint by averaging the x-coordinates and the y-coordinates:
| Endpoint | x-coordinate | y-coordinate |
|---|---|---|
| (1, 4) | 1 | 4 |
| (7, 10) | 7 | 10 |
| Midpoint | (1 + 7)/2 = 4 | (4 + 10)/2 = 7 |
Therefore, the midpoint of the line segment with endpoints (1, 4) and (7, 10) is (4, 7).
Finding the midpoint of a line segment is important in various mathematical and geometric applications. It can be used to determine the center point of a circle, divide a line segment into equal parts, or find the midpoints of multiple line segments to create a geometric figure.
Section 8: Applying Midpoints to Real-Life Scenarios

Understanding midpoints and their applications can be incredibly valuable in real-life scenarios. Whether you’re designing a floor plan, determining the optimal placement for furniture, or even calculating the shortest distance between two points, knowledge of midpoints and line segments can help you solve these problems efficiently.
One common application of midpoints is in the field of architecture and interior design. For example, when designing a room layout, it’s important to consider the placement of furniture to create a visually appealing and functional space. By identifying the midpoint of a wall or an area, designers can ensure that furniture pieces are placed symmetrically and create a balanced composition.
In addition to interior design, midpoints are also relevant in urban planning and transportation. City planners often need to calculate the shortest distance between two locations, such as determining the most efficient route for a new road or evaluating the accessibility of public transportation. By finding the midpoint of a line segment connecting these locations, planners can estimate the optimal location for infrastructure development.
Furthermore, midpoints can be applied in the field of surveying and mapmaking. Surveyors may need to measure the distance between two points on a map, such as determining the length of a property boundary. By accurately identifying the midpoint of the line segment connecting these points, surveyors can easily divide the distance and calculate the precise measurements.
In conclusion, understanding midpoints and their applications is crucial in various real-life scenarios involving design, planning, and measurement. Professionals in architecture, interior design, urban planning, and surveying can greatly benefit from this knowledge, as it allows them to make informed decisions and solve problems effectively.