
Arithmetic sequences and series are important topics in mathematics that involve the study of patterns and progressions. It is crucial for students to practice these concepts to develop a deep understanding and mastery of the subject. This article provides 10 practice exercises with detailed answers to help students strengthen their skills in arithmetic sequences and series.
Each practice exercise focuses on a different aspect of arithmetic sequences and series, including finding the common difference, identifying the nth term, determining the sum of a series, and solving word problems related to arithmetic sequences. By covering a range of topics, these exercises offer a comprehensive approach to mastering arithmetic sequences and series.
The answer key provided for each practice exercise allows students to check their work and identify any mistakes they may have made. It also serves as a guide for understanding the process and logic behind each solution. This step-by-step approach ensures that students not only obtain the correct answers but also learn the necessary techniques and strategies for solving similar problems in the future.
Practicing arithmetic sequences and series regularly is essential for students to build confidence and fluency in these mathematical concepts. By utilizing the provided answer key, students can enhance their problem-solving skills and deepen their understanding of arithmetic sequences and series. With consistent practice and the guidance of detailed solutions, students can excel in their mathematics studies and achieve success in related fields.
2 Practice Arithmetic Sequences and Series Answer Key: Master the Concepts
Arithmetic sequences and series are fundamental concepts in mathematics, and mastering them is essential for success in higher-level math courses. The 2 practice arithmetic sequences and series answer key provides students with a comprehensive understanding of these concepts and allows them to check their work and correct any mistakes.
The answer key includes detailed solutions to each practice problem, explaining the reasoning and steps involved in finding the correct answers. This helps students develop a deep understanding of the underlying principles and techniques used in arithmetic sequences and series.
Key topics covered in the answer key:
- Finding the common difference in an arithmetic sequence
- Calculating the nth term of an arithmetic sequence
- Determining the sum of an arithmetic series
- Identifying arithmetic sequences and series in real-world scenarios
By mastering these concepts, students can apply their knowledge to solve a variety of mathematical problems and improve their overall problem-solving skills. The answer key also serves as a valuable tool for self-assessment, allowing students to identify areas of weakness and focus their studies accordingly.
Furthermore, the answer key provides an opportunity for students to practice their mathematical reasoning and critical thinking skills. By comparing their own solutions to the correct answers, students can analyze their thought processes and identify areas for improvement.
Overall, the 2 practice arithmetic sequences and series answer key is an invaluable resource for students seeking to strengthen their understanding of arithmetic sequences and series. By mastering these fundamental concepts, students can build a solid foundation for future math studies and enhance their problem-solving abilities.
Arithmetic Sequences: Understanding the Basics
An arithmetic sequence is a sequence of numbers in which the difference between any two consecutive terms is constant. It is a fundamental concept in mathematics, often used in various real-world applications such as finance, physics, and computer science. Understanding the basics of arithmetic sequences is essential for solving problems and analyzing patterns.
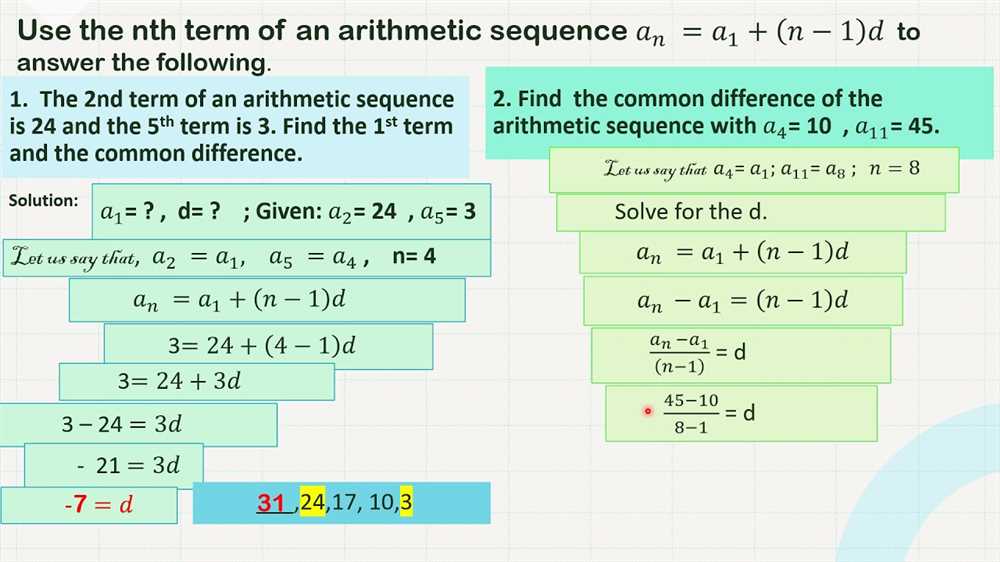
In an arithmetic sequence, each term can be obtained by adding or subtracting the common difference to the previous term. The first term is denoted as a1, the common difference as d, and the n-th term as an.
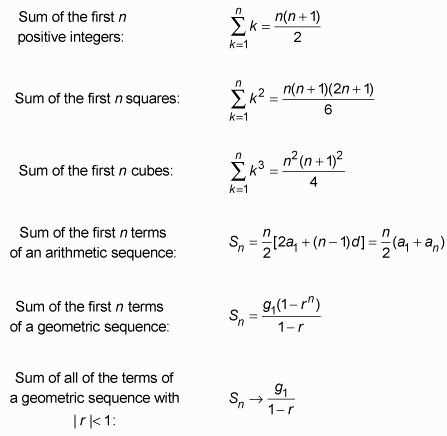
To find the n-th term of an arithmetic sequence, we can use the formula an = a1 + (n-1)d. This formula allows us to find any term in the sequence by plugging in the values of the first term, the common difference, and the position of the term.
We can also find the sum of a certain number of terms in an arithmetic sequence, often referred to as the arithmetic series. The formula for the sum of the first n terms of an arithmetic series is Sn = (n/2)(a1 + an), where Sn represents the sum and an is the n-th term of the sequence.
Arithmetic sequences and series play a crucial role in various mathematical concepts, including calculus, algebra, and number theory. By understanding the basics of arithmetic sequences, we can analyze patterns, predict future terms, and solve a wide range of mathematical problems.
Solving Arithmetic Sequences: Step-by-Step Guide
In mathematics, an arithmetic sequence is a sequence of numbers where the difference between consecutive terms is constant. Solving arithmetic sequences involves finding the next term or any term in the sequence based on this constant difference.
To solve an arithmetic sequence, follow these step-by-step instructions:
- Identify the initial term: Look for the given starting number in the sequence. This will be denoted as a1.
- Determine the common difference: Calculate the difference between any two consecutive terms in the sequence. This will be denoted as d.
- Write down the formula: Use the formula for the n-th term of an arithmetic sequence, which is given by an = a1 + (n-1)d. It represents the relationship between the term number, initial term, and common difference.
- Substitute the values: Input the known values into the formula. For example, if you are trying to find the 5th term, substitute n = 5, a1 for the initial term, and d for the common difference.
- Solve for the unknown term: Simplify the equation and solve for the unknown term using basic arithmetic operations.
By following these steps, you can easily solve any arithmetic sequence and find the missing term or determine any term in the sequence based on the given information.
Practice Problems: Arithmetic Sequences
In mathematics, an arithmetic sequence is a sequence of numbers in which the difference between consecutive terms is constant. This type of sequence is often encountered in various real-life situations, such as calculating regular payments or predicting future values.
When dealing with arithmetic sequences, it is essential to understand the key concepts and formulas associated with them. One important formula is the explicit formula for finding the nth term of an arithmetic sequence. The formula is given by:
an = a1 + (n – 1)d
where an represents the nth term, a1 is the first term, n is the position of the term in the sequence, and d is the common difference.
To strengthen your understanding of arithmetic sequences, it is crucial to practice solving various problems. Here are some practice problems to help you master the topic:
- Find the 10th term of an arithmetic sequence with a first term of 2 and a common difference of 3.
- Determine the common difference of an arithmetic sequence if the 5th term is 20 and the 10th term is 35.
- Calculate the sum of the first 15 terms of an arithmetic sequence with a first term of 4 and a common difference of 2.
- Given the first term of an arithmetic sequence is 7 and the 6th term is 25, find the common difference and the 10th term of the sequence.
By practicing these types of problems, you will become more comfortable with arithmetic sequences and be able to solve more complex problems involving them.
Arithmetic Series: Definition and Formulas
An arithmetic series is a sequence of numbers in which the difference between any two consecutive terms is constant. In other words, each term in the series is obtained by adding a fixed amount to the previous term. The fixed amount is known as the common difference, denoted by d.
The formula to find the nth term of an arithmetic sequence is given by:
an = a1 + (n – 1)d
where an represents the nth term, a1 is the first term, n is the position of the term in the sequence, and d is the common difference.
The formula to find the sum of the first n terms of an arithmetic series, also known as the arithmetic series formula, is given by:
Sn = (n/2)(a1 + an)
where Sn represents the sum of the first n terms, a1 is the first term, an is the nth term, and n is the number of terms in the series.
By using these formulas, you can easily calculate the nth term or the sum of the first n terms of an arithmetic series. These concepts are important in various areas of mathematics and real-life applications, such as finance, economics, and physics.
Finding the Sum of Arithmetic Series: A Detailed Approach
Arithmetic series are a sequence of numbers in which the difference between consecutive terms remains constant. To find the sum of an arithmetic series, there are several key steps that must be followed.
Step 1: Identify the first term (a1), the common difference (d), and the number of terms (n). The first term is the starting number of the sequence, the common difference is the constant value by which each term increases or decreases, and the number of terms represents how many terms are in the series.
Step 2: Use the formula for the nth term of an arithmetic series to find the last term (an). The formula is: an = a1 + (n-1)d. By plugging in the values of a1, d, and n, the last term can be calculated.
Step 3: Use the formula for the sum of an arithmetic series to find the total sum (Sn). The formula is: Sn = ((a1 + an) / 2) * n. By plugging in the values of a1, an, and n, the sum of the arithmetic series can be calculated.
To illustrate these steps, let’s consider an example. Given the arithmetic series with a1 = 1, d = 4, and n = 5, we can calculate the last term using the formula an = a1 + (n-1)d = 1 + (5-1)4 = 17. Then, plugging in the values of a1, an, and n into the sum formula, we get Sn = ((1 + 17) / 2) * 5 = 45.
In conclusion, finding the sum of an arithmetic series involves identifying the first term, the common difference, and the number of terms, using the formula for the last term, and then using the formula for the sum of the series. This approach allows for a systematic and detailed calculation of the sum of any arithmetic series.
Practice Problems: Arithmetic Series
Arithmetic series is a sequence of numbers in which the difference between consecutive terms is constant. It is important to understand and be able to solve arithmetic series problems, as they frequently appear in mathematical calculations and real-life situations. Here are some practice problems to help you improve your skills in this area.
Problem 1:
Find the sum of the arithmetic series: 6, 12, 18, 24, … , 102.
To find the sum of an arithmetic series, we need to know the first term (a), the common difference (d), and the number of terms (n). In this problem, a = 6, d = 6, and n = 17 (102 divided by 6). Using the formula for the sum of an arithmetic series, S = n/2 * (2a + (n-1)d), we can calculate the sum:
S = 17/2 * (2*6 + (17-1)*6) = 17/2 * (12 + 16*6) = 17/2 * (12 + 96) = 17/2 * 108 = 918.
Problem 2:
Find the 10th term of the arithmetic series: 3, 6, 9, 12, …
To find the nth term of an arithmetic series, we can use the formula: nth term = a + (n-1)d. In this problem, a = 3, d = 3, and n = 10. Plugging in the values, we get:
10th term = 3 + (10-1)*3 = 3 + 9*3 = 3 + 27 = 30.
Problem 3:
Find the common difference of an arithmetic series if the sum of the first 8 terms is 120 and the first term is 4.
To find the common difference, we need to use the formula for the sum of an arithmetic series and solve for d. In this problem, a = 4, S = 120, and n = 8. Plugging in these values, we have:
120 = 8/2 * (2*4 + (8-1)d) = 4 * (8 + 7d) = 32 + 28d.
Subtracting 32 from both sides, we get:
88 = 28d.
Dividing both sides by 28, we find that the common difference is 3.
- Practice problems like these can help you solidify your understanding of arithmetic series and improve your problem-solving skills.
- Remember to always identify the first term, common difference, and number of terms before attempting to solve an arithmetic series problem.
- Using the formulas for the sum of an arithmetic series and the nth term, you can easily find the desired values.
By regularly practicing arithmetic series problems, you will become more comfortable with this concept and more confident in your mathematical abilities.
Answer Key: 10 2 Practice Arithmetic Sequences and Series
In the 10 2 practice on arithmetic sequences and series, students were given a set of problems to solve in order to practice their understanding of these concepts. The answer key provides the correct answers to these problems, allowing students to check their work and identify any mistakes they may have made. This answer key serves as a valuable resource for both students and teachers, as it helps to reinforce the concepts learned in class and provides an opportunity for self-assessment.
The answer key includes step-by-step solutions for each problem, making it easier for students to follow along and understand the correct method of solving. It also provides explanations for any key steps or formulas used, allowing students to deepen their understanding of the material. The answer key may also include additional notes or tips to help students with common mistakes or challenging concepts.
The 10 2 practice on arithmetic sequences and series covers various topics, such as finding the common difference, determining the nth term, and calculating the sum of a series. The answer key provides solutions to problems that require these skills, ensuring that students can practice and master each concept covered. It is important for students to carefully review the answer key and understand the correct solutions in order to improve their problem-solving skills and perform well on future assessments.
Overall, the answer key for the 10 2 practice on arithmetic sequences and series is an invaluable tool for students to assess their understanding and improve their skills. It provides correct solutions, step-by-step explanations, and additional tips or notes, allowing students to learn from their mistakes and reinforce their knowledge. By using the answer key effectively, students can enhance their understanding of arithmetic sequences and series and achieve success in their math studies.


