
Geometry is a branch of mathematics that deals with the properties and relationships of shapes, lines, angles, and dimensions in space. It is a fundamental subject that is widely studied in schools, colleges, and universities. One of the key aspects of learning geometry is practicing different types of problems and exercises to strengthen understanding and problem-solving skills.
In this article, we will explore the answers to the 11.1 practice questions in geometry. These practice exercises are designed to help students reinforce their knowledge of various geometry concepts, such as angles, polygons, and circles.
By providing answers to these practice problems, students can check their work, identify any mistakes or misunderstandings, and gain confidence in their geometry skills. It is important to note that these answers should be used as a reference, and it is recommended to attempt the exercises on your own before checking the solutions.
What is 11 1 Practice A Geometry?
11 1 Practice A Geometry is a practice assignment or worksheet designed to help students reinforce their understanding of geometry concepts and skills. It is typically used in a classroom setting, where students can work individually or in groups to complete the practice questions and exercises.
This practice assignment focuses on geometry, which is a branch of mathematics that deals with the properties and relationships of points, lines, angles, shapes, and solids. In 11 1 Practice A Geometry, students can expect to encounter questions that require them to identify and classify geometric shapes, solve problems involving angles and triangles, and apply geometric theorems and formulas.
The purpose of 11 1 Practice A Geometry is to provide students with an opportunity to apply their knowledge and skills in a practical context. By working through the practice assignment, students can develop their problem-solving abilities, deepen their understanding of geometry concepts, and improve their overall performance in the subject.
To complete 11 1 Practice A Geometry successfully, students should have a solid foundation in basic geometry concepts, such as angles, lines, and shapes. They should also be familiar with geometric formulas and theorems, as well as be able to apply them to solve problems.
Overall, 11 1 Practice A Geometry serves as a valuable tool for students to practice and strengthen their geometry skills. It helps them build confidence in their abilities and prepares them for more challenging geometry tasks and assessments.
Importance of Practicing Geometry
Geometry is a fundamental branch of mathematics that deals with the properties, measurement, and relationships of shapes and figures. It provides the foundation for understanding spatial concepts and is crucial in various fields such as architecture, engineering, computer graphics, and even everyday life. Practicing geometry helps develop critical thinking skills, spatial reasoning abilities, and problem-solving techniques.
One of the main reasons why practicing geometry is important is its practical application in real-life situations. For example, understanding angles and measurements can help in home improvement projects, such as calculating the right angles for cutting tiles or building furniture. Additionally, geometry is essential in fields like architecture and engineering, where precise measurements and calculations are necessary for constructing buildings and structures.
Apart from its practicality, practicing geometry enhances logical thinking and problem-solving abilities. Through geometry, students learn how to approach complex problems by breaking them down into smaller, more manageable parts. They develop the ability to analyze relationships between different elements and apply logical reasoning to arrive at solutions. These skills are not only valuable in mathematics but also in other subjects and real-life situations.
Another benefit of practicing geometry is its contribution to spatial reasoning. By studying geometry, students develop the ability to visualize and manipulate objects in three-dimensional space. This spatial awareness is beneficial in various fields, including engineering, architecture, and design, where visualization and spatial reasoning skills are essential. Moreover, geometry helps improve one’s spatial memory, perceptual abilities, and overall understanding of the physical world.
In conclusion, practicing geometry is of great importance due to its practical application in real-life, its contribution to logical thinking and problem-solving skills, and its enhancement of spatial reasoning abilities. Whether you are pursuing a career in a math-related field or simply want to improve your cognitive skills, dedicating time to practicing geometry can bring numerous benefits and help you develop a deeper understanding of the world around you.
Understanding the Basics of 11 1 Practice A Geometry Answers
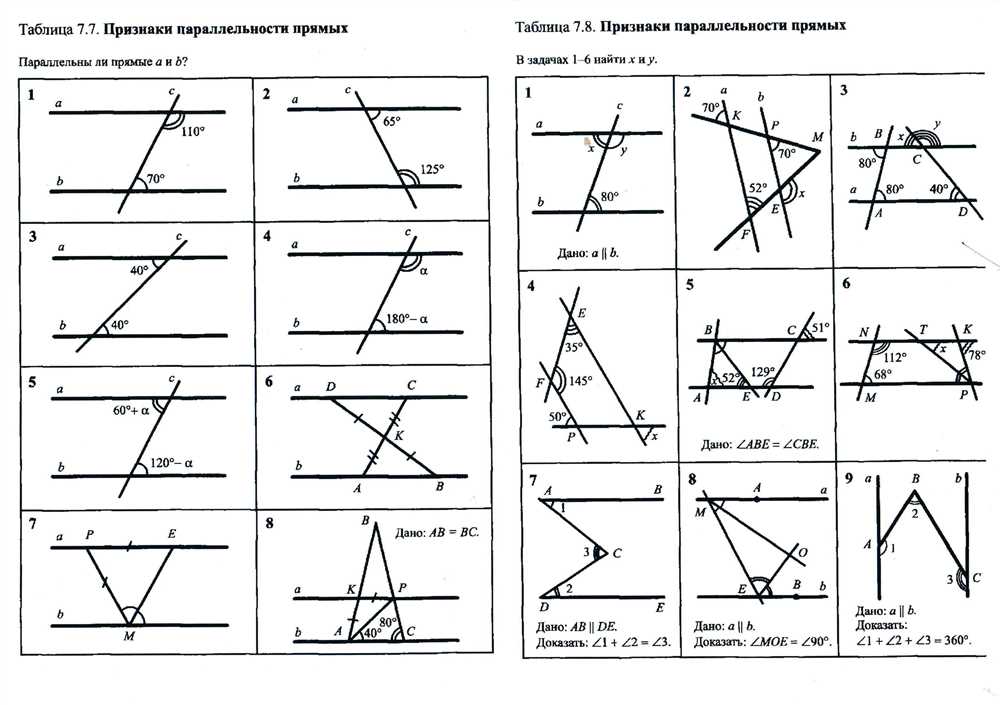
When it comes to geometry, practice is key to mastering the subject. One common practice exercise is 11 1 Practice A Geometry, which focuses on various skills and concepts related to angles, parallel lines, and transversals. In order to successfully solve these practice problems, it is essential to understand the basics of geometry and have a solid grasp of key definitions and theorems.
One important concept covered in 11 1 Practice A Geometry is the relationship between parallel lines and transversals. A transversal is a line that intersects two or more other lines. In this practice, students are often asked to identify corresponding angles, alternate interior angles, alternate exterior angles, and consecutive interior angles formed by a transversal and parallel lines. It is crucial to remember the properties and relationships between these angles in order to accurately determine their measures.
To solve the problems in 11 1 Practice A Geometry, it is also important to have a strong understanding of basic angle relationships. This includes knowing the properties of vertical angles, linear pairs, and supplementary angles. Identifying these angle relationships allows students to set up and solve equations to find missing angle measures.
Additionally, a solid grasp of the definitions and properties of different types of angles, such as acute, obtuse, and right angles, is necessary to accurately answer the questions in this practice. Students must be able to identify and classify angles based on their measures in order to use this information to solve for missing angle measures or determine angle relationships.
In conclusion, understanding the basics of geometry and having a solid grasp of key definitions, theorems, and angle relationships is crucial when working on 11 1 Practice A Geometry. By practicing these concepts and being familiar with the various angle relationships and properties, students can confidently solve the practice problems and improve their overall understanding of geometry.
Key Concepts in Geometry
In geometry, there are several key concepts that are essential to understanding the subject. These concepts provide the foundation for studying and analyzing shapes, figures, and spatial relationships. Some of these key concepts include points, lines, and planes.
Points: A point is a fundamental building block in geometry. It has no size, shape, or dimension, but it represents a specific location in space. Points can be labeled using capital letters, such as A, B, or C.
Lines: A line is a straight path that extends infinitely in both directions. It is made up of an infinite number of points. In geometry, lines are represented by lowercase letters or by any two points on the line, such as line AB or line CD. There are different types of lines, such as parallel lines, perpendicular lines, and intersecting lines.
Planes: A plane is a flat, two-dimensional surface that extends infinitely in all directions. It is defined by at least three non-collinear points or by a letter name, such as plane ABC. A plane can also be described as a flat surface that has length and width but no thickness.
Angles: Angles are formed when two lines or line segments intersect. They are measured in degrees and are labeled using three points, with the vertex point in the middle. Angles can be classified as acute, right, obtuse, straight, or reflex, depending on their measure.
Congruence: Congruence refers to objects that have the same shape and size. In geometry, congruent figures have the same angle measurements and side lengths. They can be rotated, reflected, or translated without changing their shape or size.
Symmetry: Symmetry is a property of figures that have a reflective or rotational symmetry. A figure has a reflective symmetry if it can be folded along a line so that the two halves match exactly. It has rotational symmetry if it can be rotated around a point and still match its original position.
Similarity: Similarity refers to objects that have the same shape but different sizes. In geometry, similar figures have the same shape, but their side lengths are proportional. They can be resized by a scale factor without changing their shape.
These key concepts in geometry provide the foundation for further exploration and understanding of more complex concepts and theorems. They are essential for analyzing and solving problems involving shapes, figures, and spatial relationships.
How to Solve Geometry Problems
Geometry is a branch of mathematics that deals with the study of shapes, sizes, and properties of different figures in a plane or in space. Solving geometry problems requires a systematic approach and a strong understanding of geometric concepts. Here are some steps to help you solve geometry problems:
1. Understand the problem:
The first step in solving any geometry problem is to carefully read and understand the problem statement. Identify the given information and the specific question or requests in the problem. This will help you plan your strategy for finding the solution.
2. Draw a diagram:

Geometry problems often involve visualizing the given information in the form of a diagram. Take the time to draw an accurate and clear diagram representing the given information and any additional information you might need. Label the diagram with the given values and any necessary variables.
3. Apply geometric principles:
Use your knowledge of geometric principles and formulas to analyze the problem and determine which concepts are relevant. Apply the appropriate formulas or theorems to find the solution. Make sure to show all your steps and calculations clearly.
4. Check your solution:
Once you have found a solution, it is important to check your work for accuracy. Double-check your calculations and make sure your solution makes sense in the context of the problem. Verify that your answer satisfies any given conditions or constraints.
By following these steps and practicing regularly, you can develop your skills in solving geometry problems. Remember to always think logically and apply the appropriate geometric principles to arrive at an accurate solution.
Exploring Common Mistakes in 11 1 Practice A Geometry Answers
Geometry can be a challenging subject, and it is not uncommon for students to make mistakes when solving problems. In the 11 1 Practice A Geometry workbook, there are several common mistakes that students often make. By identifying and understanding these mistakes, students can improve their problem-solving skills and avoid repeating them in the future.
One common mistake in the 11 1 Practice A Geometry answers is incorrectly applying the properties of angles. Students may confuse the different types of angles, such as supplementary or complementary angles, and use the wrong formulas or theorems to solve the problem. It is important to carefully read and understand the given information about the angles in the problem before applying any formulas or theorems.
Another common mistake is misidentifying or mislabeling geometric shapes. This can lead to incorrect calculations and answers. For example, students may incorrectly identify a triangle as an isosceles triangle when it is actually an equilateral triangle, or mistakenly label the sides or angles of a shape. It is crucial to accurately identify and label the geometric shapes in order to apply the correct formulas and theorems.
Furthermore, students often make mistakes in their calculations and computations. They may make arithmetic errors, such as adding or subtracting incorrectly, or make errors in multiplication or division. These mistakes can greatly impact the final answer and may lead to incorrect solutions. It is important to double-check all calculations and computations to ensure accuracy.
In conclusion, exploring common mistakes in 11 1 Practice A Geometry answers can help students improve their problem-solving skills and avoid repeated errors. By understanding the common mistakes related to angles, geometric shapes, and calculations, students can become more diligent in their approach to solving geometry problems and achieve better results.
Common Errors to Avoid in Geometry
Geometry can be a challenging subject, but it becomes even more difficult when students make common errors in their problem-solving. By being aware of these mistakes, students can avoid them and improve their understanding of geometry concepts. Here are some common errors to watch out for:
1. Misinterpreting the given information:
One common error is misinterpreting the information given in a problem. It’s important to carefully read the problem and understand what is being asked. Students should pay attention to details and ensure they are using the correct measurements, angles, or shapes provided in the problem.
2. Making incorrect assumptions:

Another common mistake is making incorrect assumptions about the problem. Students may assume that certain angles or shapes are congruent when they are not. It’s crucial to base solutions on facts and properties that are explicitly stated or proven, rather than making assumptions based on intuition.
3. Using incorrect formulas:
Using the wrong formulas is a common error that can lead to incorrect solutions. Students should make sure they are using the appropriate formulas for the specific problem they are solving. It’s helpful to review and memorize the relevant formulas, and understand when and how to apply them correctly.
4. Not showing sufficient work:
In geometry, it’s essential to show all the steps and calculations involved in solving a problem. Skipping steps or not showing sufficient work can make it difficult for others to understand the solution and can also lead to careless errors. Students should take the time to write out their reasoning and show each step clearly.
5. Overlooking the principles of congruence and similarity:

Geometry relies heavily on the principles of congruence and similarity. Students may overlook these principles and make mistakes in their problem-solving. It’s important to understand the properties of congruent and similar figures and use them appropriately when solving geometry problems.
By being aware of these common errors and actively working to avoid them, students can improve their accuracy and confidence in geometry. It’s essential to practice problem-solving regularly and seek clarification whenever there is confusion. With time and practice, students can become proficient in geometry and excel in their studies.