
In the study of functions, it is crucial to understand the basic building blocks known as parent functions. These fundamental functions serve as a foundation for more complex functions and allow us to explore the transformations that occur when manipulating different variables.
The 2 7 Parent Functions and Transformations Answer Key provides comprehensive insight into these foundational functions and their associated transformations. This answer key serves as a valuable resource for students and educators alike, providing a clear and concise explanation of each parent function and their corresponding graphs.
By examining the answer key, learners can deepen their understanding of functions such as linear, quadratic, cubic, square root, absolute value, and exponential functions. Each function is accompanied by a detailed explanation of its properties, including domain, range, intercepts, and symmetry. Additionally, the answer key elucidates how each function can be transformed through translations and dilations, enabling students to visualize these transformations graphically.
With the 2 7 Parent Functions and Transformations Answer Key, students can grasp the essential concepts of functions and build a strong foundation for further exploration in calculus and other branches of mathematics. This answer key is an invaluable tool that promotes conceptual understanding, problem-solving skills, and mathematical proficiency.
Overview of Parent Functions in Algebra

The study of algebra involves understanding various functions and their transformations. These functions serve as foundational building blocks, known as parent functions, that can be altered and manipulated to create different mathematical models. By understanding the characteristics and properties of parent functions, students can gain a solid foundation for studying more complex functions and equations.
There are several key parent functions that are commonly studied in algebra. These include linear functions, quadratic functions, cubic functions, absolute value functions, square root functions, and exponential functions. Each of these functions has a unique shape and set of characteristics that make them useful in different mathematical contexts.
Linear functions have a constant rate of change and form a straight line when graphed. They can be represented by the equation y = mx + b, where m is the slope and b is the y-intercept.
Quadratic functions have a parabolic shape and can be represented by the equation y = ax^2 + bx + c. The vertex of the parabola represents the minimum or maximum point of the function.
Cubic functions have a shape similar to a “S” curve and can be represented by the equation y = ax^3 + bx^2 + cx + d. They have both positive and negative slopes and can have multiple turning points.
Absolute value functions have a v-shaped graph and are represented by the equation y = |x|. They are used to represent situations where the distance from a point to a reference point is important.
Square root functions have a graph that starts at the origin and curves to the right. They are represented by the equation y = √x and are commonly used to model situations where the rate of growth is proportional to the square root of the input.
Exponential functions have a graph that increases or decreases rapidly. They are represented by the equation y = a^x, where a is a positive constant. Exponential functions are commonly used to model situations involving growth or decay.
By studying these parent functions and their transformations, students can gain a deeper understanding of how functions behave and how to manipulate them to solve various mathematical problems. This knowledge is fundamental in many areas of mathematics and can be applied to real-world scenarios as well.
Definition of Parent Functions
The parent functions are the basic, most fundamental functions that serve as the building blocks for more complex functions. These functions are usually simple and can be represented by a simple equation or graph. They define the basic shape and characteristics of functions and can be transformed through various operations to create new functions.
There are several common parent functions that are widely used in mathematics. These include the linear function, quadratic function, cubic function, square root function, absolute value function, and exponential function. Each of these parent functions has its own unique characteristics and can be represented by a specific equation and graph.
- The linear function, represented by the equation f(x) = ax + b, where a and b are constants, represents a straight line with a constant slope.
- The quadratic function, represented by the equation f(x) = ax^2 + bx + c, where a, b, and c are constants, represents a parabola.
- The cubic function, represented by the equation f(x) = ax^3 + bx^2 + cx + d, where a, b, c, and d are constants, represents a curve with more steepness than a parabola.
- The square root function, represented by the equation f(x) = sqrt(x), represents the inverse operation of squaring a number.
- The absolute value function, represented by the equation f(x) = |x|, represents the distance of a number from zero on a number line.
- The exponential function, represented by the equation f(x) = a^x, where a is a constant, represents exponential growth or decay.
These parent functions serve as a starting point for understanding more complex functions. By studying their properties and understanding how they can be transformed, mathematicians can analyze and solve a wide range of mathematical problems.
Common Parent Functions
Parent functions are the basic, unaltered functions that serve as the foundation for all other functions. They are often used as a starting point for understanding more complex functions and transformations. There are several common parent functions that are frequently studied in mathematics.
1. Linear Function

The linear function, also known as the identity function, is represented by the equation y = x. This function creates a straight line with a slope of 1. It is a basic building block for understanding more complicated functions, as it represents a direct relationship between input and output values.
2. Quadratic Function
The quadratic function is represented by the equation y = x^2. It creates a parabolic curve that opens upwards or downwards, depending on the coefficient of x^2. This function is commonly used to model relationships with a strong positive or negative correlation.
3. Absolute Value Function
The absolute value function is represented by the equation y = |x|. It produces a V-shaped graph that is symmetrical about the y-axis. This function is often used to represent distances or magnitudes, as it always returns a positive value regardless of the input.
4. Square Root Function
The square root function is represented by the equation y = √x. It creates a curve that starts at the origin and increases as x becomes larger. This function is commonly used to represent relationships with a non-linear growth pattern.
These are just a few examples of common parent functions. Understanding their properties and graphing techniques is essential for studying more complex functions and their transformations.
Characteristics of Parent Functions
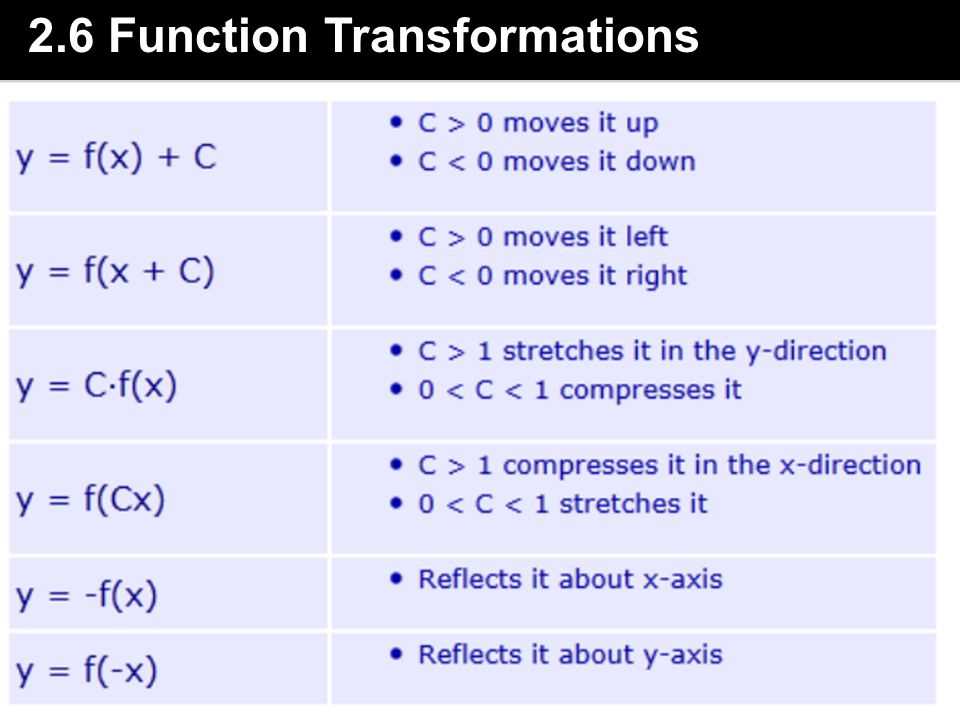
In mathematics, a parent function is a basic function from which other functions in a family of functions are derived through transformations. Understanding the characteristics of parent functions is crucial in graphing and analyzing functions, as it provides a foundation for understanding how different transformations affect the shape and behavior of graphs.
Here are some important characteristics of common parent functions:
Linear Function:
- A linear function, represented by the equation y = mx + b, is a straight line with a constant slope (m) and a y-intercept (b).
- The slope determines whether the line increases or decreases in a positive or negative direction.
- The y-intercept is the point where the line intersects the y-axis.
Quadratic Function:

- A quadratic function, represented by the equation y = ax^2 + bx + c, is a parabola.
- The coefficient “a” determines the direction and width of the parabola (positive “a” opens upwards, negative “a” opens downwards).
- The vertex represents the minimum or maximum point of the parabola.
- The axis of symmetry is a vertical line passing through the vertex.
Absolute Value Function:
- An absolute value function, represented by the equation y = |x|, is a V-shaped graph.
- The graph is symmetric with respect to the y-axis.
- The vertex of the graph is the point (0, 0).
Square Root Function:
- A square root function, represented by the equation y = √x, is a graph that starts at the point (0, 0) and increases as x increases.
- The domain of the square root function is the set of non-negative real numbers, as the square root of a negative number is undefined in the real number system.
- The range of the function is the set of non-negative real numbers.
These are just a few examples of parent functions that serve as foundational building blocks for more complex functions. Understanding their characteristics and how they transform is essential in analyzing and graphing various functions.
Transformation of Parent Functions
Parent functions are a set of basic functions that serve as building blocks for more complex functions. These functions include linear, quadratic, cubic, absolute value, square root, exponential, and logarithmic functions. Each parent function has a unique shape and set of characteristics.
Transformations of parent functions involve changing the shape, position, and size of the original function. These transformations can be accomplished through a series of operations such as translations, reflections, stretches, and compressions.
- Translations: Translations involve shifting the graph of a function horizontally or vertically. Horizontal translations are achieved by adding or subtracting a value to the x-coordinate of each point on the graph, while vertical translations involve adding or subtracting a value to the y-coordinate.
- Reflections: Reflections involve flipping the graph of a function over a specific axis. For example, a reflection over the x-axis changes the sign of the y- coordinate of each point on the graph.
- Stretches and Compressions: Stretches and compressions involve changing the size of the graph of a function. A stretch stretches the graph vertically or horizontally, while a compression compresses it. These changes are achieved by multiplying or dividing the x or y-coordinates of each point on the graph by a scaling factor.
By applying various combinations of translations, reflections, stretches, and compressions, we can create a wide range of functions with different shapes and characteristics. Understanding how to transform parent functions is essential in graphing and analyzing functions in mathematics and other fields.
Definition of Function Transformation
A function transformation is a change or manipulation applied to a basic parent function (such as a linear, quadratic, or exponential function) that alters its shape, position, or orientation on a coordinate plane. These transformations can be achieved by modifying the equation of the parent function, resulting in a new graph.
There are several types of function transformations, including translations, reflections, dilations, and compressions.
- Translations: A translation is a horizontal or vertical shift of the parent function. It moves the entire graph a certain distance in a specific direction. A horizontal translation involves adding or subtracting a value to the x-coordinate, while a vertical translation involves adding or subtracting a value to the y-coordinate.
- Reflections: A reflection is a flip of the parent function over a line. It can be a reflection over the x-axis, y-axis, or any other line. A reflection over the x-axis negates the y-coordinate, while a reflection over the y-axis negates the x-coordinate.
- Dilations: A dilation is a stretching or shrinking of the parent function. It involves multiplying the x and/or y-coordinate by a scale factor. If the scale factor is greater than 1, the graph will be stretched, and if it is between 0 and 1, the graph will be shrunk.
- Compressions: A compression is similar to a dilation, but it involves dividing the x and/or y-coordinate by a scale factor. This results in a graph that appears more cramped or compressed.
By applying these transformations, we can modify the behavior and appearance of a parent function to create a new function that fits our specific needs or requirements. Understanding and manipulating function transformations is essential in various fields, such as mathematics, physics, computer science, and engineering.
Types of Transformations
Transformations are changes that can be applied to a parent function to create a new graph. There are four main types of transformations: translations, reflections, stretches/compressions, and vertical shifts. Each type of transformation changes the position, orientation, or size of the graph in different ways.
1. Translations
A translation is a transformation that slides the graph of a function horizontally or vertically without changing its shape or size. A horizontal translation is also known as a shift. It moves the graph left or right along the x-axis. A positive value moves the graph to the right, while a negative value moves it to the left. A vertical translation shifts the graph up or down along the y-axis. A positive value moves the graph up, while a negative value moves it down.
2. Reflections
A reflection is a transformation that flips the graph of a function across a line. The line of reflection can be the x-axis, the y-axis, or any other horizontal or vertical line. A reflection across the x-axis changes the sign of the y-coordinates, while a reflection across the y-axis changes the sign of the x-coordinates. A reflection across a line other than the axes alters both the x-coordinates and y-coordinates.
3. Stretches/Compressions
A stretch or compression is a transformation that changes the size of the graph of a function. A stretch is equivalent to “stretching” the graph vertically or horizontally, while a compression is equivalent to “compressing” the graph vertically or horizontally. A stretch or compression affects both the x-coordinates and y-coordinates of the graph. A stretch/compression in the x-direction stretches/compresses the graph horizontally, while a stretch/compression in the y-direction stretches/compresses the graph vertically.
4. Vertical Shifts
A vertical shift is a transformation that moves the entire graph of a function up or down along the y-axis. It does not change the shape or orientation of the graph. A positive value for the vertical shift moves the graph up, while a negative value moves it down.
By applying these types of transformations to a parent function, we can create graphs with different characteristics and behaviors. Understanding the different types of transformations is essential for analyzing and manipulating functions in mathematics and other fields.