
Calculus 1 is the introductory course in calculus, and one of the fundamental concepts that students learn in this course is limits. Limits play a crucial role in understanding the behavior of functions and are used to define important concepts such as continuity and differentiability.
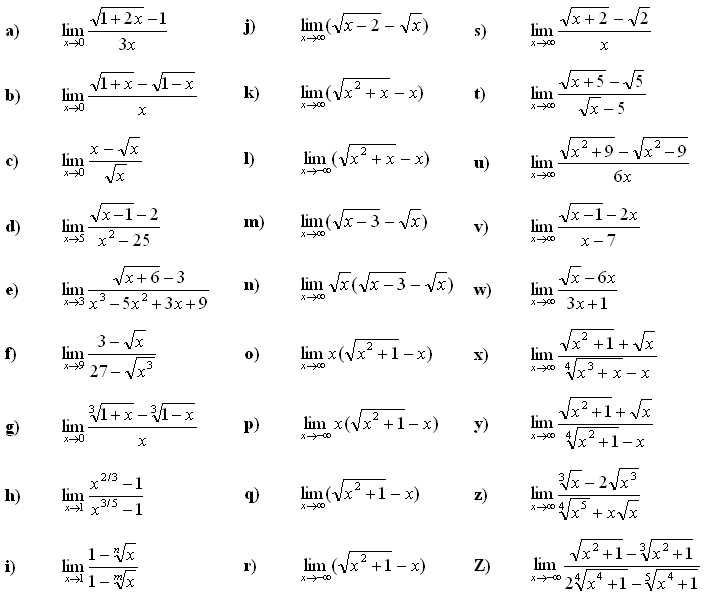
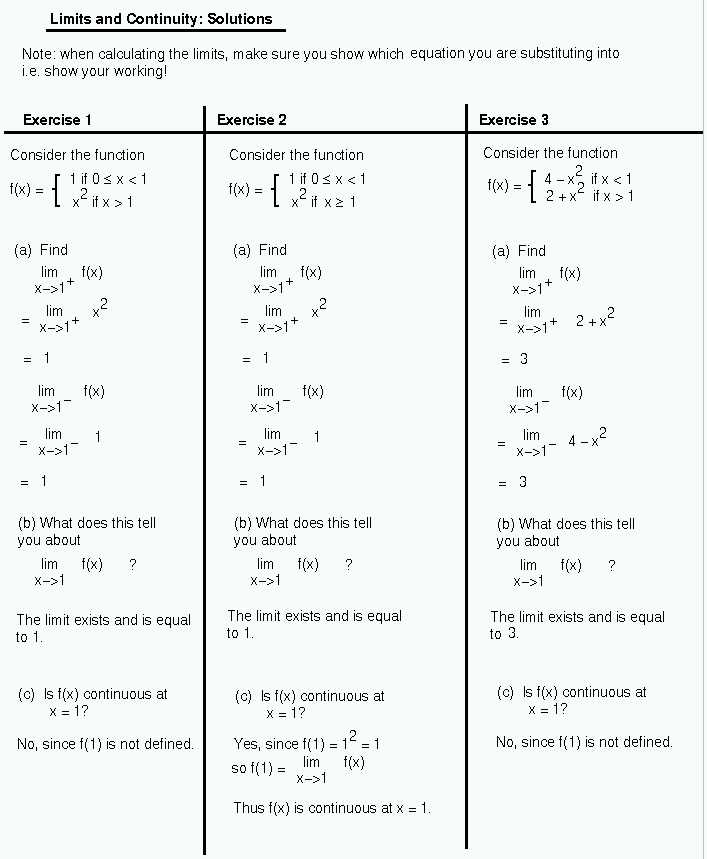
When studying limits, students often practice solving problems and exercises to strengthen their understanding and application of the concept. One such resource is a calculus 1 worksheet on limits, which provides a set of problems for students to solve and check their solutions.
In this article, we will explore the answers to the calculus 1 worksheet on limits. By going through the solutions step by step, students can gain a deeper insight into the process of evaluating limits and understand the reasoning behind each answer.
Understanding limits is crucial for success in calculus and other advanced mathematical topics. By mastering the concepts and techniques covered in this worksheet, students will be equipped with the necessary skills to tackle more complex problems in calculus and beyond.
Understanding the concept of limits in calculus
The concept of limits is fundamental in calculus and is used to describe the behavior of a function as it approaches a certain point. In calculus, a limit represents the value that a function approaches as its input approaches a certain value. It allows us to analyze the behavior of a function near a particular point and determine its properties such as continuity or differentiability.
When studying limits, it is essential to understand the concept of approaching a point from both sides. A function may approach a different value from the left (negative side) and the right (positive side). If the function approaches the same value from both sides, we say that the limit exists. However, if the function approaches different values from the left and right, the limit does not exist.
One of the ways to evaluate limits is by direct substitution, where we can substitute the value the function is approaching into the expression and calculate the function’s value at that point. However, there are cases when direct substitution does not work, such as when the function is not defined at that point or when the expression is indeterminate.
In such cases, we can use algebraic manipulation or special limit properties to evaluate the limit. Some common properties that can be used to evaluate limits include the limit laws, which involve the sum, difference, product, and quotient of functions. Additionally, the squeeze theorem and the Intermediate Value Theorem can be applied in certain situations to find the limit of a function.
Understanding the concept of limits is essential in calculus as it forms the foundation for many important topics, such as derivatives and integrals. It allows us to analyze the behavior of functions and make predictions about their values. By studying limits, we gain insights into the behavior of functions and their properties, which are crucial in various fields such as physics, engineering, economics, and more.
Exploring the properties of limits
The concept of limits plays a fundamental role in calculus. It allows us to study the behavior of a function as its input values approach a certain point. By investigating the different properties of limits, we can gain a deeper understanding of the behavior of functions and make more accurate calculations.
1. Limit laws: There are several important laws that govern the behavior of limits. These laws include the sum, difference, product, and quotient rules, which allow us to calculate limits of functions that involve basic operations. Additionally, there are laws for limits of compositions and limits of exponential and logarithmic functions. Understanding and applying these laws is crucial when working with limits.
2. One-sided limits: The concept of limits can be extended to include one-sided limits. A one-sided limit focuses on the behavior of a function as the input values approach a certain point from either the left or the right. One-sided limits can provide valuable information about the behavior of a function, particularly at points where the function is undefined or has a discontinuity.
3. Continuity: Limits also have a close connection to the concept of continuity. A function is said to be continuous at a point if its limit exists at that point and is equal to the value of the function at that point. Continuity is an important property that allows us to study functions analytically and make predictions about their behavior in different scenarios.
- 4. Intermediate value theorem:
The intermediate value theorem is a powerful result that is based on the properties of limits. It states that if a continuous function takes on two different values at two points a and b, then it must take on every value between those two points at some point within the interval [a, b]. This theorem is often used to prove the existence of solutions to equations or to demonstrate certain properties of continuous functions.
In conclusion, exploring the properties of limits allows us to analyze the behavior of functions in a systematic and precise manner. By understanding the limit laws, one-sided limits, continuity, and the intermediate value theorem, we can make accurate predictions about the behavior of functions and solve complex mathematical problems.
Solving basic limit problems
When working with limits, it is important to understand how to solve basic limit problems. These problems involve finding the value that a function approaches as its input approaches a certain value. By determining the behavior of the function as the input approaches the given value, we can find the limit.
One common method for solving basic limit problems is to plug in the value that the input approaches directly into the function. This can help determine if the function approaches a finite value, goes to infinity, or has no limit.
Another technique for solving limits is to simplify the function algebraically. This can involve factoring, combining like terms, or using properties of exponents and logarithms. Once the function is simplified, one can then evaluate the limit.
Furthermore, some limit problems may require the use of specific limit theorems. These theorems include the limit of a sum, difference, or product of functions, the limit of a quotient of functions, and the limit of a composition of functions. By applying these theorems, the limit can be found more easily.
In conclusion, solving basic limit problems involves plugging in the value, simplifying algebraically, and applying limit theorems when necessary. Understanding these techniques can help in determining the behavior of a function as the input approaches a specific value.
Evaluating limits algebraically
When it comes to evaluating limits algebraically, there are various techniques that can be used depending on the problem at hand. By understanding the properties of limits, it is possible to simplify and manipulate algebraic expressions to find their limits.
One such technique is direct substitution, which involves substituting the value at which the limit is being evaluated into the function and simplifying. For example, if we have the limit of f(x) as x approaches a, we can simply substitute a into the function f(x) and evaluate the resulting expression.
An example of this technique would be evaluating the limit of (x^2 – 9) / (x – 3) as x approaches 3. By substituting 3 into the function, we get (3^2 – 9) / (3 – 3) = (9 – 9) / 0, which is an indeterminate form. In this case, we would need to employ additional techniques to evaluate the limit.
Another technique is factoring, where we factor the numerator and denominator of the function to see if any common factors can be canceled out. This can help simplify the expression and make it easier to evaluate the limit. For example, if we have the limit of (x^2 – 4) / (x – 2) as x approaches 2, we can factor the numerator as (x – 2)(x + 2) and cancel out the common factors of (x – 2), resulting in the limit of (x + 2) as x approaches 2, which is simply 4.
In some cases, we may need to use more advanced techniques such as L’Hopital’s rule, which allows us to evaluate limits involving indeterminate forms (such as 0/0 or ∞/∞) by taking the derivative of the numerator and denominator repeatedly until we reach a determinate form. This can be particularly useful when dealing with complex functions.
Overall, evaluating limits algebraically requires a good understanding of algebraic manipulation and the properties of limits. By utilizing various techniques such as direct substitution, factoring, and L’Hopital’s rule, it is possible to solve a wide range of limit problems and determine the behavior of functions as they approach certain values.
Using limit laws to simplify calculations

In calculus, limit laws are powerful tools that help simplify and evaluate complex limit calculations. These laws enable us to break down complicated expressions and analyze the behavior of functions as they approach certain values. By understanding and applying limit laws, we can simplify calculations and make them more manageable.
One important limit law is the sum law, which states that the limit of the sum of two functions is equal to the sum of their limits. This law allows us to break down a complicated expression into simpler parts and evaluate each part separately. For example, if we have the limit of (f(x) + g(x)) as x approaches a, we can evaluate the limits of f(x) and g(x) individually and then add the results together.
Another useful limit law is the product law, which states that the limit of the product of two functions is equal to the product of their limits. Similar to the sum law, this law allows us to simplify expressions by evaluating each part separately and then multiplying the results. It is particularly helpful when dealing with functions that involve multiplication or division.
The quotient law is another important limit law, stating that the limit of the quotient of two functions is equal to the quotient of their limits, provided the limit of the denominator is not zero. This law is useful when dealing with rational functions and helps simplify calculations involving division. By applying the quotient law, we can break down complex expressions and evaluate them more easily.
These are just a few examples of the limit laws that can be used to simplify calculations in calculus. By understanding and applying these laws, we can make complex limit calculations more manageable and obtain accurate results. Limit laws are essential tools for anyone studying calculus and understanding their applications can greatly enhance one’s ability to solve problems and analyze functions.
Solving limit problems involving indeterminate forms
When calculating limits, it is common to come across indeterminate forms, which are expressions that do not have a clear value. These include forms such as 0/0, ∞/∞, 0*∞, and ∞-∞. Solving limit problems involving indeterminate forms requires special techniques to determine the actual value of the limit.
One approach to solving these problems is through algebraic manipulation. By factoring, simplifying, or applying limit laws, it is often possible to convert the indeterminate form into a form that can be evaluated. For example, by multiplying the numerator and denominator by a conjugate or rationalizing the expression, it is possible to simplify the indeterminate form and find a limit that can be calculated.
Another technique for solving limit problems involving indeterminate forms is through the use of L’Hospital’s Rule. This rule states that if the limit of the quotient of two functions approaches an indeterminate form, then the limit of the quotient of their derivatives will yield the same result. By repeatedly taking derivatives and evaluating the limit, it is possible to find the actual value of the limit.
It is important to note that not all limit problems involving indeterminate forms can be solved using algebraic manipulation or L’Hospital’s Rule. In some cases, additional techniques such as Taylor series expansions, trigonometric identities, or special limit theorems need to be applied. It is crucial to analyze the specific problem and choose the appropriate method to solve the limit.
In conclusion, solving limit problems involving indeterminate forms often requires creative thinking and the application of various techniques. Whether through algebraic manipulation, L’Hospital’s Rule, or other advanced methods, it is possible to determine the actual value of the limit and evaluate the function at that point.
Applying limits to analyze the behavior of functions

When studying calculus, one of the fundamental concepts is the limit. A limit is a mathematical tool that allows us to understand how a function behaves as it approaches a certain input value. By applying limits, we can analyze various aspects of a function, such as continuity, differentiability, and the location of vertical asymptotes.
Continuity: By taking the limit of a function as the input approaches a specific value, we can determine if the function is continuous at that point. If the limit exists and is equal to the value of the function at that point, then the function is continuous. If the limit does not exist or is not equal to the value of the function, then the function is not continuous at that point.
Differentiability: The concept of limits also helps us analyze the differentiability of a function. If the limit of the difference quotient (the rate of change of the function) exists as the input approaches a specific value, then the function is differentiable at that point. If the limit does not exist or is not unique, then the function is not differentiable at that point.
Vertical asymptotes: Limits are also useful in determining the presence of vertical asymptotes in a function. By taking the limit of the function as the input approaches a certain value (usually either positive or negative infinity), we can determine if the function approaches a specific value or if it tends towards infinity or negative infinity. If the limit exists and is finite, then there is no vertical asymptote. If the limit approaches infinity or negative infinity, then there is a vertical asymptote at that value.
In conclusion, applying limits to analyze the behavior of functions is an essential tool in calculus. It allows us to determine the continuity, differentiability, and presence of vertical asymptotes of a function. By understanding these concepts, we can gain a deeper insight into the behavior and properties of functions in calculus.