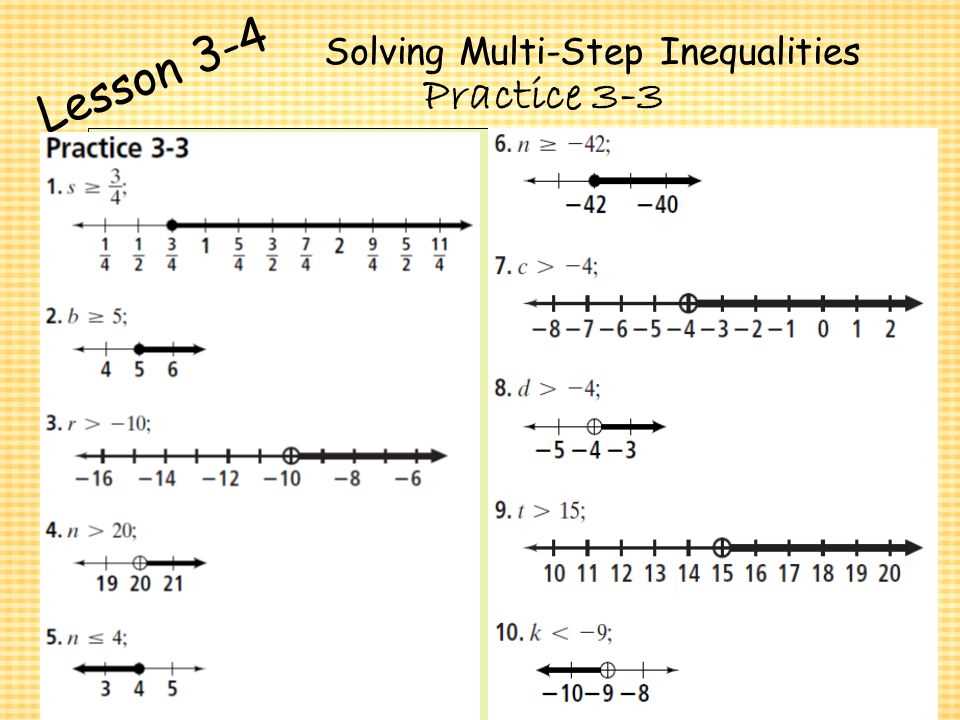
Systems of inequalities are a valuable tool in mathematics for representing multiple constraints or conditions. They involve multiple inequalities and allow us to find a range of possible solutions that satisfy all of these conditions simultaneously. In this article, we will explore 3 3 practice systems of inequalities form K and provide an answer key to help you understand and solve these problems.
The 3 3 practice systems of inequalities form K is a set of three inequalities that need to be solved simultaneously. These inequalities can involve variables and constants, and the goal is to find the range of values that satisfy all three conditions. By solving these systems of inequalities, we can explore and understand the relationships between multiple constraints or conditions.
Using the answer key for the 3 3 practice systems of inequalities form K, you will be able to check your solutions and verify if you have correctly solved the system of inequalities. This answer key will provide you with the correct range of values that satisfy all three conditions and help you gain confidence in your problem-solving skills.
By practicing and mastering the 3 3 practice systems of inequalities form K, you will develop a strong understanding of how to solve and interpret systems of inequalities. This skill is valuable in various mathematical disciplines and real-life scenarios where multiple constraints need to be taken into account. So, let’s dive in and explore the fascinating world of systems of inequalities!
What is a practice system of inequalities form K?
A practice system of inequalities form K refers to a specific type of mathematical exercise that involves solving a system of inequalities using the format given in form K. This format typically includes a set of equations with inequality symbols such as less than (<), greater than (>), less than or equal to (≤), or greater than or equal to (≥).
In a practice system of inequalities form K, the goal is to find the solution set that satisfies all the given inequalities simultaneously. This solution set is represented by the region on a coordinate plane where all the inequalities overlap, indicating the values that satisfy all the conditions.
Students are often required to graph the inequalities on a coordinate plane to visually represent the solution set. They can then use various techniques, such as shading regions or testing points, to determine the values that satisfy the system of inequalities.
Practicing with systems of inequalities form K helps students develop their skills in solving and graphing multiple inequalities simultaneously. This topic is important in various fields, such as economics, where inequalities are used to represent constraints and boundaries in decision-making processes.
Example:
Solve the following system of inequalities using form K:
- x + y < 5
- 2x – y ≥ 0
Graphing the first inequality, x + y < 5, we draw a dotted line with a downward slope passing through the point (0, 5). Shading the region below this line represents the values that satisfy the inequality.
Graphing the second inequality, 2x – y ≥ 0, we draw a solid line with an upward slope passing through the point (0, 0). Shading the region above this line represents the values that satisfy the inequality.
The overlapping shaded region of both inequalities represents the solution set, indicating the values that satisfy both conditions.
Why are practice systems of inequalities important in mathematics?

The study of practice systems of inequalities is crucial in mathematics because it helps students develop their problem-solving skills and apply mathematical concepts to real-life situations. Inequalities are mathematical expressions that compare two values or quantities, and they play a fundamental role in various fields such as economics, physics, and engineering.
By practicing systems of inequalities, students learn how to analyze and interpret different scenarios, make connections between mathematical representations and real-world contexts, and make informed decisions based on mathematical constraints. This type of problem-solving skill is essential in today’s data-driven society, where decision-making often requires a quantitative analysis of multiple variables.
Moreover, practice systems of inequalities allow students to understand the concept of solutions and solution sets. In mathematics, a solution set represents all the possible values that satisfy a given inequality system. By solving systems of inequalities, students learn how to identify and represent solution sets graphically or algebraically, and they develop a deeper understanding of the interplay between equations and inequalities.
Furthermore, practice systems of inequalities help students develop logical reasoning skills and enhance their ability to analyze complex situations. Inequality systems often involve multiple constraints and variables, requiring students to think critically and apply mathematical principles to identify feasible solutions. This type of problem-solving skill is not only essential in mathematics but also applicable to various disciplines and real-life scenarios.
In conclusion, practice systems of inequalities are important in mathematics because they help students develop problem-solving skills, apply mathematical concepts to real-life situations, understand solution sets, and enhance logical reasoning abilities. By mastering this topic, students build a strong foundation for further mathematical studies and develop skills that are valuable in various fields.
Key components of a practice system of inequalities form K
A practice system of inequalities form K consists of several key components that are essential for solving and graphing inequalities. These components help to understand and visualize the relationship between variables and represent them on a graph.
Inequality symbols: The system of inequalities uses symbols such as <, >, ≤, and ≥ to compare the values of variables. These symbols indicate whether the values are less than, greater than, less than or equal to, or greater than or equal to.
Variable constraints: Each inequality in the system has its own set of constraints for the variables involved. These constraints define the range of possible values for each variable and limit the solutions to the inequalities.
Graphing tools: Graphing is an important component of a practice system of inequalities form K. It allows for a visual representation of the inequalities and their solutions. Graphing tools such as a coordinate plane and graph paper are used to plot the inequalities and shade the regions that satisfy the constraints.
Solution set: The solution set is the set of all possible values that satisfy all the inequalities in the system. It is represented by shading the appropriate region on the graph or by listing the values that satisfy all the constraints.
Algorithmic approach: Solving a system of inequalities form K requires an algorithmic approach, which involves systematically analyzing each inequality and its constraints, graphing the inequalities, and determining the overlapping shaded regions to find the solution set.
These key components are crucial for successfully solving and graphing a system of inequalities form K. They provide a structured approach to understanding and visualizing the relationships between variables and finding the solutions to the inequalities. By utilizing these components effectively, one can accurately represent and interpret the solutions to the system of inequalities.
Common challenges when solving practice systems of inequalities
When solving practice systems of inequalities, there are several common challenges that students may encounter. These challenges can make the process of finding a solution more difficult and may require additional steps or strategies. Here are some of the common challenges:
- Complex graphs: One challenge is dealing with complex graphs when graphing the system of inequalities. Sometimes the graphs may have multiple lines or curves that intersect or overlap, making it difficult to determine the exact solution region.
- Identifying the solution region: Another challenge is identifying the solution region from the graph. Students might struggle with identifying the shaded region that represents the solution to the system of inequalities, especially if the graph is complex or contains multiple overlapping regions.
- Interpreting inequalities: Understanding the meaning of the inequalities in the context of the problem can also pose a challenge. Sometimes students may misinterpret the inequality signs and incorrectly represent the constraints in the system.
- Using the correct method: There are different methods for solving systems of inequalities, such as graphing, substitution, or elimination. Choosing the most appropriate method for a given problem can be challenging, especially if the problem does not provide clear instructions on which method to use.
- Dealing with infinite solutions or no solution: In some cases, the system of inequalities may have infinite solutions or no solution at all. Determining and interpreting these cases correctly can be challenging for students who are not familiar with these concepts.
Overall, solving practice systems of inequalities requires a solid understanding of graphing, interpreting inequalities, and selecting the appropriate method. Students may need to practice these skills and strategies to overcome the common challenges and successfully find the solution to a system of inequalities.
Tips and tricks for solving practice systems of inequalities efficiently
When it comes to solving practice systems of inequalities, efficiency is key. Here are some tips and tricks to help you solve these problems quickly and accurately:
- Identify the graph: Before diving into the problem, it’s important to visualize the inequality graph. This can help you understand the constraints and boundaries presented by the system.
- Combine like terms: Simplify the inequalities by combining like terms. This can help you eliminate unnecessary variables and make the equations easier to work with.
- Choose a method: There are different methods to solve systems of inequalities, such as graphing, substitution, or elimination. Choose the method that best suits the given problem.
- Use intervals: To represent the solution set on a number line, use intervals. Determine the points where the inequalities intersect or overlap, and represent these regions with intervals.
- Check your solution: After solving the system, always check your solution. Plug in the values back into the original inequalities to verify if they satisfy all the given constraints.
By following these tips and tricks, you can approach practice systems of inequalities with confidence. Remember to practice regularly to improve your skills and speed in solving these types of problems.
Examples of Practice Systems of Inequalities and Their Solutions
In the study of systems of inequalities, students often encounter a variety of examples that help them understand the concept and practice solving them. Here are a few examples of practice systems of inequalities and their solutions:
Example 1:
Consider the system of inequalities:
- x + y ≤ 5
- 2x – y < 2
To solve this system, we can graph each inequality on the coordinate plane and find the overlapping region. The solution to the system is the set of points that satisfy both inequalities simultaneously. In this case, the solution is the triangular region below the line x + y = 5 and above the line 2x – y = 2.
Example 2:
Consider the system of inequalities:
- x > 0
- y < 4
- x + y > 3
To solve this system, we can again graph each inequality on the coordinate plane. However, this time we notice that there is no overlap between the regions defined by the inequalities. Therefore, there is no solution to this system of inequalities. The empty set represents the solution.
Example 3:
Consider the system of inequalities:
- x + 2y ≤ 8
- x – 2y ≤ 4
To solve this system, we can choose a test point, such as (0, 0), and substitute it into each inequality. If the test point satisfies both inequalities, then it is included in the solution. In this case, (0, 0) satisfies both inequalities, so it is included in the solution. The solution is the shaded region below the line x + 2y = 8 and below the line x – 2y = 4.
These examples illustrate the different ways in which systems of inequalities can be solved and the variety of solutions that can arise. By practicing these types of problems, students can develop a deeper understanding of how inequalities work and enhance their problem-solving skills.
Checking if your practice systems of inequalities solutions are correct is an important step in the learning process. It helps you identify any mistakes or errors in your work and allows you to understand the concepts more accurately. Here are some steps you can follow to check if your solutions are correct:
1. Substitute the values back into the original inequalities
One way to check your solutions is by substituting the values back into the original inequalities. If the solution satisfies all the inequalities, then it is correct. For example, if the solution to the system of inequalities is (x = 3, y = 2), substitute these values into each inequality and check if both sides of the inequality are true. If they are, then your solution is correct.
2. Graph the solution on a coordinate plane
Another way to check your solution is by graphing it on a coordinate plane. Plot the points that satisfy all the inequalities and shade the region that represents the solution. Compare your graph with the original inequalities to see if they match. If they do, then your solution is correct. This method is especially useful when dealing with systems of linear inequalities.
3. Solve the system of inequalities algebraically
If you have a system of two linear inequalities, you can solve it algebraically to check your solution. This involves manipulating the inequalities to isolate the variables and then determining the range of values that satisfy both inequalities. If your solution matches the one obtained algebraically, then it is correct.
By following these steps, you can ensure that your practice systems of inequalities solutions are correct and gain a better understanding of the topic. Remember to double-check your work and seek help if you’re unsure about any step or concept.