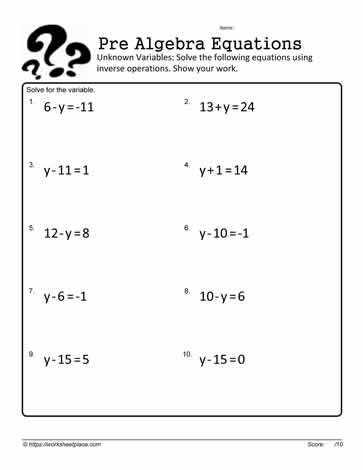
Are you struggling with solving systems of equations with three variables? Look no further! In this article, we will provide you with five systems of equations worksheets and their answers to help you practice and master this topic.
Solving systems of equations with three variables can be challenging, but with the right practice, you can become a pro! Each worksheet in this collection focuses on a different set of equations and provides step-by-step solutions to guide you through the process.
Whether you are a student preparing for a test or an educator looking for resources to share with your students, these worksheets will be invaluable. They cover a wide range of difficulty levels, ensuring there is something for everyone to enhance their understanding of systems of equations.
Don’t let complex systems of equations intimidate you! Take advantage of these worksheets and their answers to build your confidence and improve your problem-solving skills. With consistent practice, you’ll soon be able to tackle even the most challenging systems of equations with ease.
So, what are you waiting for? Dive into these 3 5 systems with three variables worksheet answers and start honing your skills today!
What is a system of equations with three variables?
A system of equations with three variables consists of a set of equations that are interconnected and involve three unknown quantities. These equations can be linear or nonlinear, and the goal is to find the values of the variables that satisfy all the equations simultaneously. Solving a system of three-variable equations requires finding the values that make all equations true.
The equations in a system with three variables can represent real-life situations or mathematical problems. They can be used to model and solve problems in various fields such as physics, chemistry, economics, and engineering. By solving these systems, we can obtain solutions that describe meaningful relationships between the variables and provide insight into the problem at hand.
Systems of three-variable equations can be solved using various methods, such as substitution, elimination, or matrix algebra. These methods involve manipulating the equations to reduce the system into an equivalent one with fewer variables. The final step is to solve for the remaining variables and find their values.
To check if a solution is valid, we substitute the found values back into the original equations and verify that they satisfy all of them. If they do, then the solution is correct, and it represents the intersection point or points of the equations in the system. If there is no solution or the equations are inconsistent, then the system is considered to be inconsistent or dependent.
In summary, a system of equations with three variables is a set of interconnected equations with three unknowns. Solving these systems allows us to find the values of the variables that make all equations true and provides valuable information about the problem being modeled.
Importance of solving systems with three variables
The ability to solve systems of equations with three variables is an important skill in mathematics and real-world problem-solving. These systems arise in various fields such as engineering, physics, economics, and computer science. By solving these systems, we can find the values of the variables that satisfy all the equations simultaneously, providing valuable insights and solutions.
One key reason why solving systems with three variables is important is that it allows us to model and analyze more complex situations. In many real-world problems, there are multiple variables that interact with each other, and understanding their relationships is crucial. By setting up a system of equations with three variables, we can capture these relationships and represent the problem mathematically. Solving the system then provides us with a solution that can be utilized to make predictions and decisions.
The ability to solve systems with three variables also helps in the process of linear optimization. In optimization problems, we aim to maximize or minimize a certain objective function while satisfying a set of constraints. Often, these constraints involve multiple variables, and finding the optimal solution requires solving a system of equations with three variables. By solving such systems, we can identify the optimal values of the variables that lead to the best possible outcome.
Furthermore, solving systems with three variables enhances our problem-solving skills and mathematical reasoning. It requires us to analyze and manipulate multiple equations simultaneously, applying various algebraic techniques such as elimination, substitution, and matrix methods. These skills are valuable not only in mathematics but also in other areas of life, where understanding and solving complex problems is crucial.
In conclusion, the importance of solving systems with three variables extends beyond the realm of mathematics. It enables us to model complex situations, make predictions, optimize outcomes, and enhance our problem-solving skills. Mastering this skill is essential for anyone seeking to excel in mathematics and other disciplines that rely on quantitative analysis and critical thinking.
Solving systems of equations with three variables

Solving systems of equations with three variables can be more complex and challenging compared to systems with two variables. However, by using various methods and techniques, it is possible to find the solutions for these types of systems.
One common method for solving systems of equations with three variables is the method of substitution. This involves solving one equation for one variable and substituting that expression into the other equations. By eliminating one variable at a time, the system can be simplified to a system of two equations with two variables, which can be solved using methods like substitution or elimination.
Another method for solving these systems is the method of elimination. This involves adding or subtracting equations in such a way that one variable is eliminated, leaving a system of two equations with two variables. Then, this reduced system can be solved using the methods mentioned above.
In some cases, it may be necessary to use the method of matrices and determinants, known as Cramer’s Rule, to solve systems of equations with three variables. This method involves representing the coefficients of the variables in a matrix form and calculating determinants to find the values of the variables.
Overall, solving systems of equations with three variables requires a combination of algebraic manipulation, logical thinking, and understanding of different solution methods. Practice and familiarity with these methods will improve problem-solving skills in this area of mathematics.
Method 1: Substitution
The method of substitution is a technique used to solve systems of equations with three variables. It involves solving one equation for one variable and substituting that value into the other equations to find the values of the other variables.
Here’s how the method works:
- Start by selecting two equations from the system.
- Solve one equation for one variable. Choose a variable that is easy to isolate.
- Substitute the value of the variable obtained in step 2 into the other equation.
- Solve the resulting equation for the remaining variable.
- Substitute the values of the variables obtained in steps 2 and 4 back into one of the original equations to find the value of the third variable.
Let’s look at an example to better understand the process. Suppose we have the following system of equations:
Equation 1: 2x + 3y – z = 10
Equation 2: x + y + 2z = 5
Equation 3: 3x – 2y + z = -3
Step 1: We can choose Equation 2 and Equation 3.
Step 2: Let’s solve Equation 2 for x. We have x = 5 – y – 2z.
Step 3: Substitute the value of x from step 2 into Equation 3:
3(5 – y – 2z) – 2y + z = -3
Step 4: Simplify the equation and solve for y:
15 – 3y – 6z – 2y + z = -3
-5y – 5z = -18
y + z = 18/5
Step 5: Substitute the values of y and z into Equation 1:
2x + 3(18/5) – z = 10
Step 6: Simplify the equation and solve for x:
2x + 54/5 – z = 10
2x – z = 16/5
x = (16/5) + z/2
Using this method of substitution, we can find the values of x, y, and z that satisfy the given system of equations.
Method 2: Elimination
In solving systems of linear equations with three variables, another method commonly used is the method of elimination. This method involves manipulating the equations to eliminate one variable at a time, ultimately resulting in a system of two equations with two variables that can be easily solved.
To begin with, it is important to ensure that the equations are arranged in a standard form, where the variables are aligned vertically. This can be achieved by rearranging the equations if necessary. Once in standard form, the elimination method can be applied.
The first step in the elimination method is to choose two equations from the system and identify a variable to eliminate. This is usually done by looking for a coefficient of one variable that can be made equal in magnitude but with opposite signs in the two equations. By adding or subtracting the equations, the chosen variable will be eliminated.
Next, the resulting equation after elimination is obtained. This equation will involve the remaining two variables. Repeat the previous steps, choosing different pairs of equations and eliminating different variables until only two variables remain in the system.
Finally, solve the system of two equations with two variables using any preferred method, such as substitution or elimination. Once the values of the two variables are found, substitute them back into one of the original equations to solve for the third variable.
The method of elimination can be an effective approach for solving systems of linear equations with three variables, as it breaks down the problem into more manageable steps. However, it requires careful manipulation and organization of the equations to ensure accurate results.
Method 3: Matrix method
Another method for solving systems of equations with three variables is the matrix method. This method involves representing the system of equations as a matrix equation and then performing row operations to solve for the variables.
To use the matrix method, we start by writing the coefficients of the variables and the constants in the system of equations as a matrix. We then perform row operations to simplify the matrix and eliminate variables.
Let’s consider an example to understand the matrix method. Suppose we have the following system of equations:
2x + 3y – z = 10
4x – y + 2z = 4
x + 2y + 3z = 6
Using the matrix method, we can write the system of equations as the following matrix equation:
| 2 | 3 | -1 | 10 |
| 4 | -1 | 2 | 4 |
| 1 | 2 | 3 | 6 |
From here, we can perform row operations to simplify the matrix and eliminate variables. The goal is to transform the matrix into row echelon form or reduced row echelon form. Once the matrix is in this form, we can easily solve for the variables.
The matrix method provides a systematic approach to solving systems of equations with three variables. It allows us to organize the coefficients and constants into a matrix, making it easier to perform row operations and find the solution. Practice with solving systems of equations using the matrix method can help solidify the understanding of linear algebra concepts and improve problem-solving skills.
Practice Exercises
Practice exercises are an essential part of mastering the concepts and techniques required to solve 3×5 systems with three variables. These exercises enable students to apply their knowledge and skills in a variety of problem-solving scenarios, helping them to develop fluency and proficiency in the subject.
Exercise 1:
Solve the following system of equations using the elimination method:
- 2x + 3y – z = 4
- x – 2y + z = 3
- 3x – 5y + 2z = 7
Exercise 2:
Use substitution to solve the system of equations:
- x + y + z = 6
- x – 2y – z = 1
- 2x + y + 3z = 10
Exercise 3:
Apply the Gauss-Jordan elimination method to solve the following system of equations:
- x + y – z = 2
- 2x – 3y + z = -4
- 3x + 2y – 2z = 5
Exercise 4:
Solve the system of equations using the matrix method:
- 3x + 2y – z = 5
- 2x – 4y + 3z = -1
- x + y – 2z = 7
Exercise 5:
Practice solving 3×5 systems with three variables by solving the following system of equations:
- 2x + 3y – z = 1
- 4x – 2y + 3z = 5
- x – 5y – z = 2
These exercises provide students with the opportunity to refine their problem-solving skills and strengthen their understanding of solving 3×5 systems with three variables. By practicing different methods of solving such systems, students can develop a solid foundation in algebra and enhance their ability to tackle more complex mathematical problems.
Example problem 1: Solve the system using substitution
In this example problem, we will solve a system of equations using the method of substitution. The system consists of three equations with three variables.
Let’s consider the following system:
- Equation 1: 2x + 3y – z = 7
- Equation 2: 3x – 4y + 2z = -1
- Equation 3: x + y + z = 3
To solve this system using substitution, we will solve one equation for one variable and substitute that expression into the other equations.
Let’s start by solving Equation 3 for the variable x. We isolate x by subtracting y and z from both sides:
| x = 3 – y – z |
Now we substitute this expression for x in Equation 1 and Equation 2:
- Equation 1: 2(3 – y – z) + 3y – z = 7
- Equation 2: 3(3 – y – z) – 4y + 2z = -1
We simplify these equations:
- Equation 1: 6 – 2y – 2z + 3y – z = 7
- Equation 2: 9 – 3y – 3z – 4y + 2z = -1
We combine like terms:
- Equation 1: y – 3z = 1
- Equation 2: -7y – z = -10
Now we have a system of two equations with two variables. We can solve this system using any method we prefer, such as substitution or elimination.
That’s how we solve a system of equations using the method of substitution. It involves isolating one variable and substituting its expression in the other equations to simplify the system.