Geometry is a fascinating subject that deals with the study of shapes, sizes, dimensions, and properties of objects in the physical world. It plays a crucial role in various fields such as architecture, engineering, and art. To excel in geometry, it is essential to practice and strengthen the foundational knowledge and skills.
One effective way to reinforce geometry concepts is through the 5 5 Practice A Geometry textbook exercises. These exercises focus on five key areas: angle relationships, parallel and perpendicular lines, congruent triangles, quadrilaterals, and similar figures. By solving these problems, students can sharpen their analytical thinking, problem-solving abilities, and geometric intuition.
The answers to the exercises in 5 5 Practice A Geometry provide students with an invaluable tool to check their work and ensure their understanding of the material. With the help of these answers, students can identify any mistakes they may have made and learn from them. Moreover, having access to the correct answers allows students to develop a deeper understanding of the underlying principles and concepts in geometry.
By regularly practicing with the 5 5 Practice A Geometry answers, students can build their confidence and proficiency in the subject. They will become more adept at identifying geometric patterns, applying theorems and formulas, and solving geometric problems accurately and efficiently. This practice will not only benefit their performance in geometry but also lay a solid foundation for advanced mathematical concepts in the future.
In conclusion, the 5 5 Practice A Geometry answers are an essential resource for any student looking to master the basics of geometry. By using these answers to check their work and learn from their mistakes, students can strengthen their foundational knowledge, problem-solving skills, and geometric intuition. Regular practice with these exercises will undoubtedly lead to improved performance and a deeper understanding of the fascinating world of geometry.
Why is it important to practice geometry?
Geometry is not just a subject that is studied in school, but it is also an essential part of our everyday lives. From calculating the area of a room to understanding the shapes and angles in architectural designs, geometry plays a crucial role in various fields. Therefore, practicing geometry is important for several reasons.
1. Developing critical thinking skills: Geometry requires logical reasoning and problem-solving skills. Through practicing geometry, students learn how to analyze shapes, patterns, and spatial relationships, which helps develop their critical thinking abilities. These problem-solving skills can be applied to real-life situations, enabling individuals to make informed decisions and solve complex problems.
2. Enhancing spatial awareness: Geometry involves understanding and manipulating various shapes and their dimensions. By practicing geometry, individuals develop spatial awareness, which is crucial in fields such as architecture, engineering, and design. Being able to visualize and comprehend spatial relationships enhances creativity and allows for the creation of innovative designs and solutions.
3. Strengthening mathematical foundation: Geometry is an integral part of mathematics, and by practicing geometry, students reinforce their understanding of mathematical concepts and principles. It helps them solidify their knowledge of concepts such as angles, shapes, symmetry, and measurement. A strong mathematical foundation is essential for success in higher-level math courses and various careers that require mathematical skills.
4. Preparing for standardized tests: Geometry is a prominent topic in standardized tests such as the SAT and ACT. By practicing geometry, students become familiar with different types of geometric problems and develop the necessary skills to solve them accurately and efficiently. This preparation can significantly improve their performance on these tests and increase their chances of admission to colleges and universities.
5. Understanding the world around us: Geometry is all around us, from the design of buildings to the arrangement of objects in our environment. By practicing geometry, individuals gain a deeper understanding of the world around them and recognize the geometric principles at play. This knowledge allows them to appreciate the beauty and complexity of their surroundings and apply geometric concepts in their daily lives.
In conclusion, practicing geometry is essential for the development of critical thinking skills, enhancing spatial awareness, strengthening mathematical foundations, preparing for standardized tests, and understanding the world around us. It is a subject that goes beyond the confines of the classroom and plays a significant role in various aspects of our lives.
Understanding the basics of geometry
Geometry is a branch of mathematics that deals with the study of shapes, sizes, and properties of figures and spaces. It is a fundamental subject that is widely used in various fields, including architecture, engineering, and design. By understanding the basics of geometry, one can gain valuable insights into the relationships between different objects and develop problem-solving skills.
In geometry, there are several key concepts that form the foundation of the subject. One such concept is points, which are basic building blocks and have no size or shape. These points can be connected to form line segments, which are defined by two endpoints. Line segments can be extended to form lines, which are infinitely long in both directions.
Another important concept in geometry is angles, which are formed when two lines intersect. Angles can be measured in degrees and are classified based on their size:
- Acute angles are less than 90 degrees.
- Right angles are exactly 90 degrees.
- Obtuse angles are more than 90 degrees but less than 180 degrees.
- Straight angles are exactly 180 degrees.
Understanding angles is crucial in geometry as they play a vital role in determining the relationships between lines and shapes.
Shapes are another important aspect of geometry, with some of the common shapes including:
- Triangles, which have three sides and three angles.
- Squares, which have four equal sides and four right angles.
- Rectangles, which have four sides and four right angles, but opposite sides are not necessarily equal.
- Circles, which have a curved boundary and are defined by a fixed center point.
Understanding the properties and characteristics of these shapes is essential in solving geometric problems and analyzing real-world scenarios.
Overall, having a solid grasp on the basics of geometry is crucial for further exploration of the subject and its applications in various fields. By understanding points, lines, angles, and shapes, individuals can develop the necessary skills to solve complex geometric problems and gain a deeper understanding of the world around them.
Five basic concepts of geometry
In the study of geometry, there are five key concepts that form the foundation of this mathematical discipline. These concepts are essential for understanding the relationships between shapes, sizes, and angles, and are applied in various geometric calculations.
1. Point:
A point is the most basic concept in geometry. It is represented by a dot and has no size or dimension. Points are used to define positions in space and serve as the building blocks for constructing other geometric figures.
2. Line:
A line is a collection of points that extends indefinitely in both directions. It is represented by a straight path with no thickness or width. Lines can be straight or curved, and they are important for defining the boundaries and intersections of various shapes.
3. Plane:
A plane is a two-dimensional flat surface that extends infinitely in all directions. It has no thickness and is represented by a shape that looks like a tabletop. Planes are used to define the surfaces of different geometric figures, and they are crucial for understanding the properties of shapes in three-dimensional space.
4. Angle:
An angle is formed by two rays with a common endpoint, called the vertex. It represents the amount of rotation between the two rays and is measured in degrees or radians. Angles are used to measure the amount of turn or deviation between two lines or surfaces, and they play a significant role in geometric constructions and calculations.
5. Shape:
A shape is a closed figure made up of lines or curves. It can be two-dimensional, like a square or a circle, or three-dimensional, like a cube or a sphere. Shapes are classified based on their properties, such as the number of sides, angles, or symmetry. They are fundamental in geometry as they provide the basis for understanding the properties and relationships between different figures.
These five basic concepts of geometry form the bedrock of the subject and are essential for understanding and applying its principles in various mathematical and practical contexts.
How to solve geometry problems using the 5 5 practice?
The 5 5 practice in geometry is a method used to solve geometry problems by breaking them down into smaller steps and applying specific strategies to each step. This practice is particularly effective for problems that involve angles, lines, shapes, and measurements. By following the 5 5 practice, you can approach geometry problems systematically and find the correct solutions.
Step 1: Understand the problem. Carefully read the problem and identify the given information, what needs to be calculated, and any additional conditions or constraints. This step is crucial to ensure that you have a clear understanding of the problem before proceeding.
Step 2: Draw a diagram. Visualize the problem by drawing a clear and accurate diagram. Label the given information and any additional variables or measurements that need to be determined. The diagram acts as a visual representation of the problem, making it easier to analyze and solve.
Step 3: Identify and apply relevant formulas or theorems. Review your knowledge of geometric formulas and theorems and identify the ones that apply to the problem at hand. This step involves applying the appropriate formulas or theorems to the given information and using them to calculate the unknowns.
Step 4: Solve step-by-step. Break down the problem into smaller components or steps, and solve each step sequentially. This may involve finding the measurements of angles or sides, calculating areas or volumes, or determining relationships between different elements of the geometry problem.
Step 5: Check your answer. Once you have completed the calculations and obtained a solution, check your answer to ensure its accuracy. Review the problem statement and verify that your solution satisfies all the given conditions and constraints. Double-check your calculations and retrace your steps if necessary.
In summary, the 5 5 practice is a systematic approach to solving geometry problems. By following these steps of understanding the problem, drawing a diagram, applying relevant formulas, solving step-by-step, and checking your answer, you can effectively solve a wide range of geometry problems.
Improve problem-solving skills
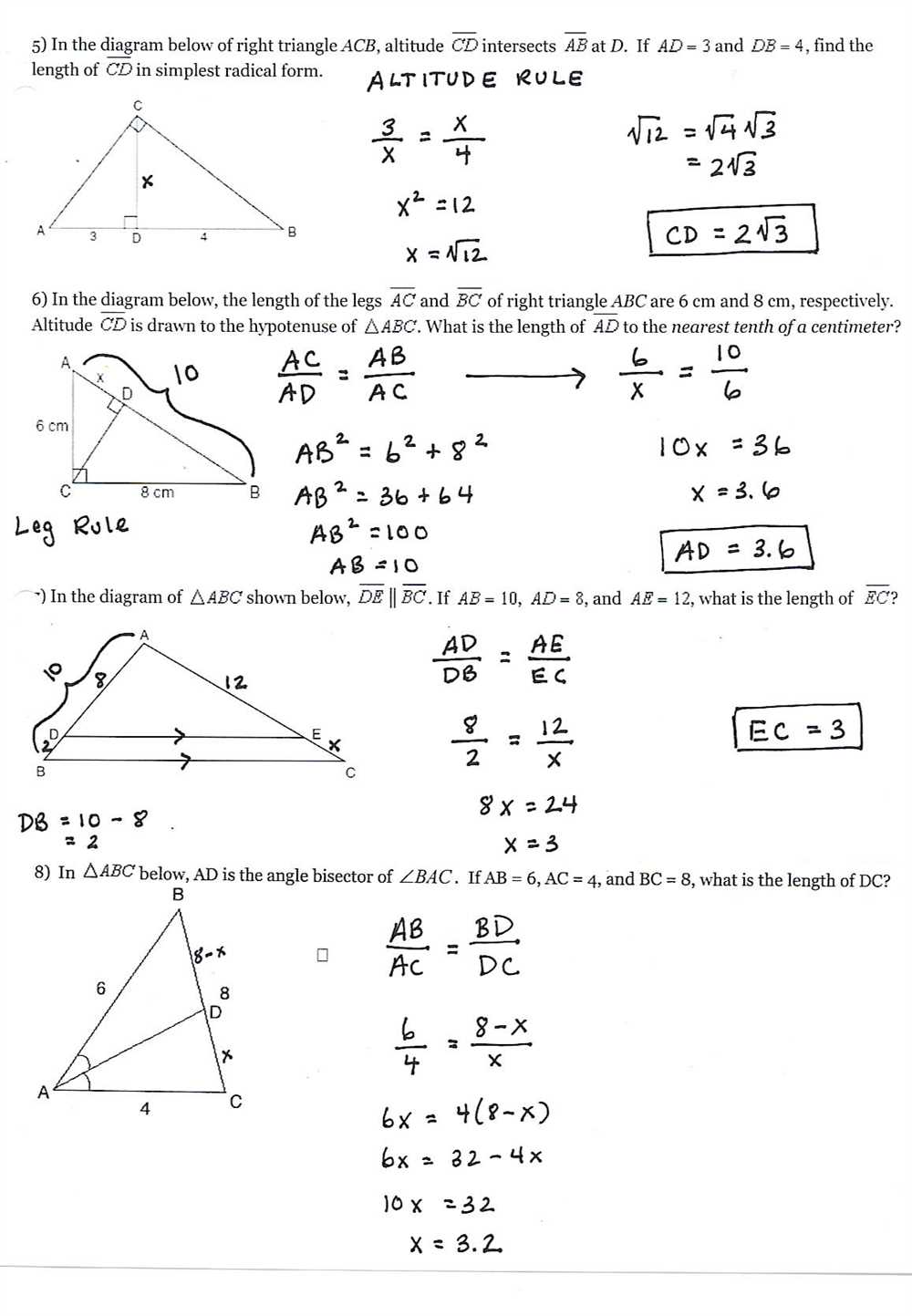
Problem-solving skills are essential in many areas of life, including academics, work, and personal situations. Whether you are solving a math problem or trying to find a solution to a conflict, the ability to think critically and approach problems from different angles is crucial.
One way to improve problem-solving skills is through practice. By regularly engaging in problem-solving activities, you can develop your ability to analyze situations, identify potential solutions, and make informed decisions. This can be done through activities such as solving logic puzzles, playing strategy games, or completing math problems.
Another method to enhance problem-solving skills is by seeking out challenges and taking on new and unfamiliar tasks. By stepping out of your comfort zone and tackling difficult problems, you can sharpen your ability to think creatively and adapt to different situations. This could involve volunteering for a challenging project at work, enrolling in a class outside of your area of expertise, or participating in a problem-solving competition.
In addition to practice and challenging yourself, developing strong problem-solving skills also requires effective communication and collaboration. Being able to work well with others, listen to different perspectives, and incorporate feedback can greatly enhance your ability to find innovative solutions. Engaging in group problem-solving activities, such as team projects or brainstorming sessions, can help you develop these important skills.
In conclusion, improving problem-solving skills is a valuable endeavor that can benefit various areas of life. By practicing regularly, seeking out challenges, and enhancing communication and collaboration abilities, you can become a more effective problem solver. This skill set is not only useful for academic and work settings, but also for personal growth and navigating the complexities of everyday life.
Enhance spatial reasoning abilities
Spatial reasoning is an essential cognitive skill that allows individuals to visualize and manipulate objects in their mind. It involves the ability to think in three dimensions and mentally manipulate spatial information, which is crucial for understanding and solving problems in various fields, including geometry, architecture, engineering, and navigation.
Enhancing spatial reasoning abilities can have numerous benefits in academic and professional settings. It helps individuals develop a better understanding of geometry concepts and visualize complex shapes and structures. It also improves problem-solving skills, as spatial reasoning enables individuals to analyze and find solutions to spatial puzzles and challenges more effectively.
- Improved performance in STEM fields: Spatial reasoning is particularly important in science, technology, engineering, and mathematics (STEM) fields. By enhancing spatial reasoning abilities, individuals can excel in subjects such as physics, computer science, and engineering, as these fields often require the ability to visualize and manipulate complex three-dimensional objects and concepts.
- Enhanced creativity and innovation: Spatial reasoning skills are closely linked to creative thinking and innovation. By developing spatial reasoning abilities, individuals can brainstorm and generate innovative ideas, visualize the potential outcomes of their ideas, and design and create new inventions and solutions.
- Improved navigation skills: Spatial reasoning is also vital for navigation and wayfinding. It helps individuals develop a mental map of their surroundings, navigate through unfamiliar environments, and determine the shortest or most efficient routes to their destinations.
- Better performance in visual arts: Spatial reasoning plays a significant role in visual arts, such as painting, sculpture, and architecture. By honing spatial reasoning abilities, individuals can better understand composition, perspective, and spatial relationships, enabling them to create more visually appealing and impactful pieces of art.
Overall, enhancing spatial reasoning abilities can have a profound impact on various aspects of life, from academic and professional success to everyday problem-solving and creativity. By engaging in activities and exercises that promote spatial reasoning, individuals can sharpen this cognitive skill and unlock their full potential in various domains.