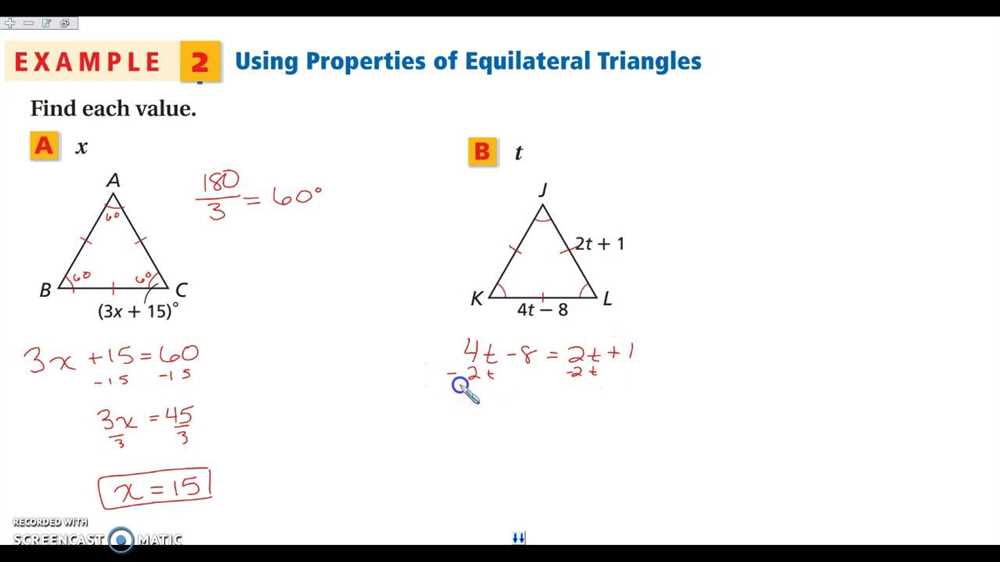
In mathematics, triangles are fundamental geometric shapes that are studied extensively. Two special types of triangles are isosceles triangles and equilateral triangles. Understanding their properties and relationships is crucial in solving various mathematical problems. In this article, we will explore the answer key to the 7 2 Isosceles and Equilateral Triangles worksheet, providing clear explanations and examples to help you grasp these concepts.
An isosceles triangle is a triangle that has two sides of equal length. This means that two angles opposite those sides are also equal. By knowing this, we can apply specific properties and theorems to solve problems involving isosceles triangles. The answer key to the 7 2 Isosceles and Equilateral Triangles worksheet will walk you through various problems, illustrating how to identify and use these properties effectively.
On the other hand, an equilateral triangle is a triangle that has all three sides and angles equal in measure. Equilateral triangles have unique properties, such as all angles being 60 degrees and all sides being congruent. By understanding these properties, we can solve problems related to equilateral triangles confidently. The answer key to the 7 2 Isosceles and Equilateral Triangles worksheet will provide step-by-step explanations and examples, helping you master these concepts effectively.
By practicing with the 7 2 Isosceles and Equilateral Triangles worksheet and referring to the provided answer key, you will enhance your understanding of these triangle types and be better equipped to tackle more complex mathematical problems. Geometry plays an essential role in numerous fields, from architecture to engineering, and mastering concepts related to triangles is a stepping stone to success in these areas. Take your time, grasp the concepts, and enjoy the process of learning and applying geometry in practical contexts.
What are isosceles and equilateral triangles?
An isosceles triangle is a type of triangle that has two sides of equal length. This means that two of its angles are also equal. The third angle, called the base angle, is different from the other two angles. In an isosceles triangle, the two equal sides are opposite to the two equal angles. The base angle is opposite to the base side.
An equilateral triangle is a type of triangle that has all three sides of equal length. This means that all three angles in an equilateral triangle are also equal. Each angle in an equilateral triangle measures 60 degrees. In an equilateral triangle, each side is opposite to each angle and each angle is equal to each side.
Both isosceles and equilateral triangles have symmetry. An isosceles triangle has a line of symmetry along the perpendicular bisector of the base, meaning that if you fold the triangle along this line, the two halves will match exactly. An equilateral triangle has three lines of symmetry, passing through each vertex and the midpoint of the opposite side.
In geometry, isosceles and equilateral triangles are important because they have unique properties and can be used to solve various mathematical problems. They are often used in constructions and proofs, as well as in real-life applications such as architecture and engineering.
- In an isosceles triangle, the angles opposite to the equal sides are also equal.
- In an equilateral triangle, all three angles are equal to 60 degrees.
- The sum of the angles in a triangle is always 180 degrees.
- An isosceles triangle can be classified as acute, obtuse, or right depending on the measure of its angles.
- An equilateral triangle is always classified as acute because all three angles are less than 90 degrees.
Properties of Isosceles Triangles
An isosceles triangle is a triangle that has two sides of equal length. This property leads to several unique properties and relationships within the triangle.
Base Angles: In an isosceles triangle, the angles opposite the two equal sides are called the base angles. The base angles are always congruent, which means they have the same measure. This can be proved using the fact that the angles of a triangle add up to 180 degrees.
Median: The median of an isosceles triangle is a line segment that connects the midpoint of the base to the vertex opposite the base. In an isosceles triangle, the median is also an altitude, perpendicular bisector, and angle bisector. This means that it divides the base into two equal segments, forms right angles with the base, and divides the vertex angle into two congruent angles.
Height: The height of an isosceles triangle is the perpendicular distance from the base to the vertex opposite the base. Since the median is also an altitude, the height of an isosceles triangle can be found by dividing the length of the base by two and using the Pythagorean theorem to find the height.
Isosceles Triangle Theorem: The Isosceles Triangle Theorem states that if two sides of a triangle are congruent, then the angles opposite those sides are congruent. This theorem can be used to prove other properties and relationships within isosceles triangles.
Overall, the properties of isosceles triangles are interconnected and provide a foundation for understanding and solving problems involving these specific types of triangles.
Base angles of an isosceles triangle
An isosceles triangle is a triangle that has two sides of equal length. One of the key properties of an isosceles triangle is that its base angles are congruent, meaning they have the same measure. This is true regardless of the size or shape of the triangle.
When you have an isosceles triangle, you can identify the base angles by looking at the two sides that are of equal length. These two sides are called the legs of the triangle, and the third side is called the base. The base angles are the angles formed by the legs and the base.
For example, let’s consider an isosceles triangle with side lengths of 7, 7, and 10 units. In this case, the two sides of length 7 are the legs, and the side of length 10 is the base. The base angles of this triangle will be congruent, so we can say that both base angles are x degrees.
To find the measure of the base angles in an isosceles triangle, you can use the formula: base angle = (180 – top angle) / 2. The top angle is the angle formed by the two legs of the triangle, opposite the base. By finding the measure of the top angle and substituting it into the formula, you can determine the measure of the base angles.
Equal sides of an isosceles triangle
An isosceles triangle is a triangle that has two sides of equal length. These two sides are called the legs of the triangle, while the third side is called the base. In an isosceles triangle, the angles opposite the legs are also equal. This means that if two sides of a triangle are equal in length, the angles opposite those sides are also equal.
When working with isosceles triangles, it is important to remember these key properties. For example, if we know that two sides of a triangle are equal, we can determine that the angles opposite those sides are also equal. This can be useful in solving problems involving isosceles triangles, as it allows us to find missing angles or sides.
Example: Let’s say we have an isosceles triangle with two sides of length 5 units each and a base of length 8 units. We can use the fact that the angles opposite the equal sides are equal to find the measure of the angles. By dividing the triangle into two congruent right triangles, we can use trigonometry to find the angle measures.
To find the measure of the angles, we can use the sine function. For one of the right triangles, we have sin(x) = 5/8, where x is the measure of the angle opposite the base. Solving for x, we find that x ≈ 38.66 degrees. Therefore, the angles opposite the legs in the isosceles triangle are approximately 38.66 degrees each.
Knowing the properties of isosceles triangles and how to use them can greatly simplify problem-solving in geometry. By recognizing the equal sides and their corresponding angles, we can efficiently find missing measurements and solve various geometric problems. This knowledge is essential for understanding and working with isosceles triangles.
Properties of equilateral triangles
An equilateral triangle is a special type of triangle that has three congruent sides and three congruent angles. These triangles have many interesting properties that make them unique and important in geometry.
Equal sides: One of the defining characteristics of an equilateral triangle is that all three sides are of equal length. This means that if we know the length of one side, we automatically know the lengths of the other two sides. This property allows us to easily calculate various measurements and angles within the triangle.
Equal angles: Another notable property of equilateral triangles is that all three angles are equal and measure 60 degrees each. This symmetry makes equilateral triangles visually appealing and often used in design and art. Moreover, the sum of all angles in an equilateral triangle is always 180 degrees.
Center of symmetry: The center of an equilateral triangle is a point that is equidistant from all three vertices. This point is known as the circumcenter and is also the center of symmetry for the triangle. It is possible to draw a circle that passes through all three vertices of an equilateral triangle, with the center of the circle coinciding with the circumcenter of the triangle.
Perpendicular bisectors: The perpendicular bisectors of the sides of an equilateral triangle intersect at a single point, which is the circumcenter of the triangle. In other words, if we draw a line segment that starts from a vertex and bisects the opposite side perpendicularly, all three of these lines will intersect at the same point.
Regular polygon: Due to their symmetrical nature, equilateral triangles are considered regular polygons. A regular polygon is a polygon with all sides and angles congruent. Equilateral triangles are the simplest regular polygon and serve as the building blocks for other regular polygons, such as squares, hexagons, and so on.
In conclusion, equilateral triangles possess several unique properties that distinguish them from other triangles. Their equal sides and angles, center of symmetry, intersecting perpendicular bisectors, and classification as regular polygons make them fascinating objects to study in geometry.
All sides are equal in an equilateral triangle
An equilateral triangle is a special type of triangle where all three sides are of equal length. This means that the lengths of the sides are the same and they are all congruent. In mathematical terms, if we denote the length of one side as “s”, then the lengths of the other two sides are also “s”.
This property of an equilateral triangle can be easily observed by measuring the lengths of its sides using a ruler. If all three sides have the same measurement, then it is an equilateral triangle. The fact that the lengths of the sides are equal also means that all three angles in an equilateral triangle are equal to 60 degrees.
An equilateral triangle can be characterized by its symmetry. Since all sides and angles are equal, the triangle has rotational symmetry of order 3. This means that if the triangle is rotated by an angle of 120 degrees clockwise or counterclockwise, it will still look the same. This symmetry property makes equilateral triangles aesthetically pleasing and often used in art and design.
In addition to the equal side lengths, equilateral triangles also have other interesting properties. For example, the altitude, or the perpendicular line segment from one vertex to the opposite side, bisects the opposite side and is also the median and angle bisector. The incenter, or the point where the angle bisectors intersect, is also the centroid and circumcenter of the triangle.
All angles are equal in an equilateral triangle
An equilateral triangle is a special type of triangle where all three sides and all three angles are equal. This means that each angle in an equilateral triangle measures 60 degrees.
The fact that all angles are equal in an equilateral triangle can be proven using various methods. One way to prove this is by considering the properties of isosceles and equilateral triangles. An isosceles triangle has two equal sides and two equal angles, which means that the base angles of an isosceles triangle are equal. In an equilateral triangle, all three sides are equal, so all three angles must also be equal.
The equality of angles in an equilateral triangle is also evident when considering the sum of the angles in a triangle. The sum of all angles in any triangle is always 180 degrees. Since an equilateral triangle has three equal angles, each angle measures 60 degrees. Therefore, the sum of the angles in an equilateral triangle is 180 degrees, satisfying the property of all triangles.
In summary, all angles in an equilateral triangle are equal and measure 60 degrees. This property can be proven through the properties of isosceles and equilateral triangles, as well as the sum of angles in a triangle.
Relationship between isosceles and equilateral triangles

Isosceles and equilateral triangles are both types of triangles that have some interesting relationships. An isosceles triangle is a triangle with two equal sides, while an equilateral triangle is a triangle with three equal sides.
One relationship between these two types of triangles is that every equilateral triangle is also an isosceles triangle. This is because in an equilateral triangle, all three sides are equal, so any two sides can be considered as the equal sides of an isosceles triangle.
Another relationship between isosceles and equilateral triangles is in terms of their angles. In an isosceles triangle, the angles opposite the equal sides are also equal. In an equilateral triangle, not only are all three sides equal, but all three angles are also equal, measuring 60 degrees.
Furthermore, both types of triangles have interesting properties when it comes to their heights and areas. In an isosceles triangle, the height, or the perpendicular distance from the base to the opposite vertex, can be calculated using the Pythagorean theorem. In an equilateral triangle, the height can be found by using the formula (sqrt(3)/2) times the length of one side. Additionally, both types of triangles have a unique relationship between their area and their side lengths, with formulas that can be used to calculate their areas.
In conclusion, isosceles and equilateral triangles have several relationships in terms of their sides, angles, heights, and areas. Understanding these relationships can help in solving problems and proving geometric theorems involving these types of triangles.