Algebra 1 is a fundamental course that lays the groundwork for more advanced mathematical concepts. Despite its importance, mastering algebra can be a challenging task for many students. One way to ensure a solid understanding of the subject is by regularly assessing knowledge through tests and quizzes. However, when it comes to evaluating performance, having access to the answer key plays a crucial role in the learning process.
The Algebra 1 functions test answer key serves as a valuable tool to help students navigate through complex problems and verify their solutions. By having access to the answer key, students can gain immediate feedback on their performance, identify areas for improvement, and reinforce their understanding of algebraic concepts.
Furthermore, the answer key allows students to analyze their mistakes and learn from them. By comparing their solutions with the correct answers, students can identify recurring errors and misconceptions, enabling them to make the necessary adjustments in their approach to problem-solving. This process fosters a deeper understanding of algebra and enhances critical thinking skills.
In addition, the answer key can provide invaluable support for teachers. With access to the answer key, teachers can efficiently assess student understanding, provide targeted feedback, and track progress over time. It also allows for the identification of common difficulties or misconceptions among students, enabling instructors to adjust their teaching strategies accordingly.
What is Algebra 1?

Algebra 1 is a branch of mathematics that deals with symbols and the manipulation of these symbols to represent relationships between numbers and quantities. It is a fundamental concept in mathematics and serves as a building block for more advanced math courses. In Algebra 1, students learn about various types of functions, equations, and inequalities.
Functions: One of the core topics in Algebra 1 is functions. A function is a relation between a set of inputs (called the domain) and a set of outputs (called the range), where each input has exactly one output. Students learn how to represent functions using equations, tables, and graphs, and how to perform operations on functions such as addition, subtraction, multiplication, and division.
Equations and Inequalities: Another important aspect of Algebra 1 is equations and inequalities. Students learn how to solve linear equations and inequalities, quadratic equations, and systems of equations. They also learn how to graph equations and inequalities on the coordinate plane and analyze their solutions.
Problem-solving skills: Algebra 1 helps students develop critical thinking and problem-solving skills. They learn how to analyze a problem, identify relevant information, and apply algebraic concepts to find a solution. These skills are not only important in mathematics but also in various real-life applications such as economics, physics, and engineering.
Overall, Algebra 1 serves as a foundation for higher-level mathematics and provides students with essential tools for understanding and solving mathematical problems. It helps them develop logical reasoning and analytical skills that are crucial for success in future math courses and practical life situations.
Overview of Algebra 1
Algebra 1 is the foundation of all higher-level mathematics courses and plays a crucial role in developing logical thinking and problem-solving skills. This branch of mathematics deals with the study of mathematical symbols and the rules for manipulating those symbols to solve equations and represent relationships between variables.
Key Concepts:
In Algebra 1, students are introduced to a variety of key concepts that form the building blocks for more advanced math courses. These concepts include:
- Variables: Algebra uses letters, called variables, to represent unknown quantities or numbers in equations. This allows for the generalization of problem-solving techniques and the ability to solve equations with different values.
- Equations: Equations are mathematical statements that express the equality of two expressions. Algebra 1 focuses on solving linear equations, which involve variables raised to the first power.
- Functions: Functions are an essential part of algebra and describe relationships between input and output values. Students learn to graph and analyze linear and quadratic functions, as well as understand concepts like domain and range.
- Inequalities: Inequalities are mathematical statements that express relationships where one quantity is greater or less than another. Students learn to solve and graph linear and quadratic inequalities.
- Systems of Equations: A system of equations involves multiple equations that need to be solved simultaneously. Students learn techniques to solve systems of linear equations using methods like substitution or elimination.
- Exponents and Radicals: Exponents and radicals are used to represent repeated multiplication and roots. Students learn properties and rules for simplifying expressions with exponents and radicals.
Mastering the concepts learned in Algebra 1 is crucial as they form the foundation for higher-level math courses like Algebra 2, Geometry, and Calculus. They also have real-world applications in fields like science, engineering, and economics.
Importance of Algebra 1 functions test
Algebra 1 functions test is crucial for students in their mathematical journey as it assesses their understanding of fundamental concepts and skills related to functions. Functions play a significant role in various branches of mathematics, science, and engineering, making it essential for students to grasp these concepts early on.
First and foremost, the Algebra 1 functions test assesses students’ ability to understand and analyze relationships between variables. By learning about functions, students develop skills to calculate and interpret rates of change, solve problems involving real-life situations, and make predictions based on given data. These skills are invaluable in fields such as economics, physics, and computer science, where analyzing patterns and predicting outcomes are essential.
The test also evaluates students’ proficiency in manipulating and solving equations involving functions. This knowledge is fundamental in higher-level mathematics courses, where functions become more complex and intertwined. In addition, a solid foundation in algebraic function manipulation helps students in their general problem-solving skills, as they learn to approach problems systematically, analyze different scenarios, and apply logical reasoning to find the correct solutions.
Furthermore, the Algebra 1 functions test provides an opportunity for students to demonstrate their understanding and mastery of mathematical concepts. By performing well on the test, students not only prove their comprehension of functions but also build confidence and motivation to tackle more advanced mathematical topics. Additionally, the test results can be used by educators to identify areas of weakness and adjust their instruction accordingly, ensuring that students receive targeted support and intervention when needed.
In conclusion, the Algebra 1 functions test is an essential component of students’ mathematical education. It assesses their understanding of functions, which are fundamental in various academic and professional fields. A strong grasp of functions enables students to analyze relationships, make predictions, and solve problems across disciplines. By performing well on the test, students demonstrate their proficiency and build a solid foundation for future mathematical endeavors.
Sample Algebra 1 functions test questions
In Algebra 1, functions are an important concept to understand. They represent relationships between inputs and outputs, and can be represented mathematically in various ways. To assess your understanding of functions, here are some sample test questions:
Question 1:
Given the function f(x) = 2x + 5, find f(3).
Answer: To find f(3), substitute x = 3 into the function: f(3) = 2(3) + 5 = 6 + 5 = 11. Therefore, f(3) = 11.
Question 2:
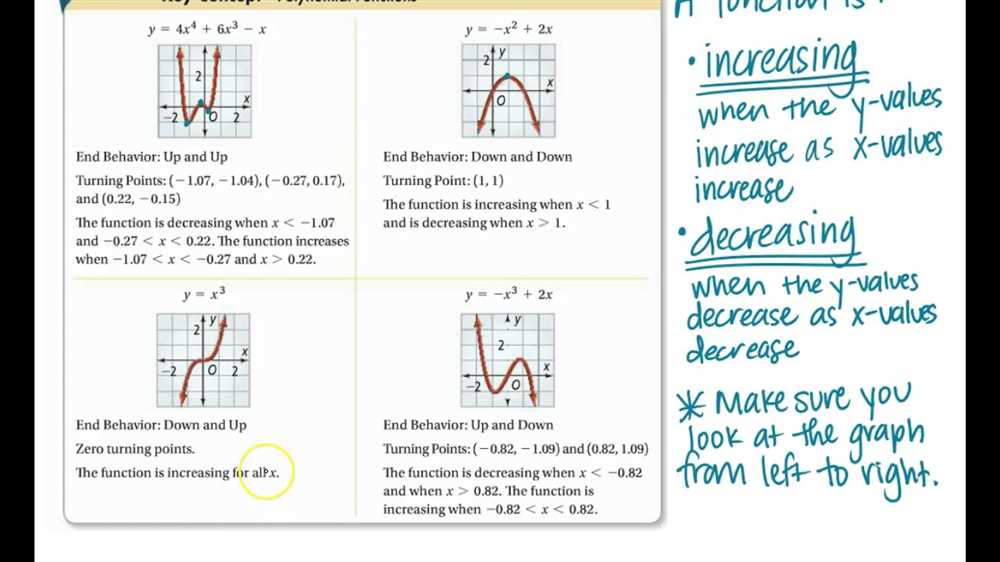
Identify the domain and range of the function g(x) = x^2.
Answer: The domain of the function g(x) = x^2 is all real numbers, because any value of x can be squared. The range of the function is all non-negative real numbers, because squaring a real number always results in a non-negative value.
Question 3:
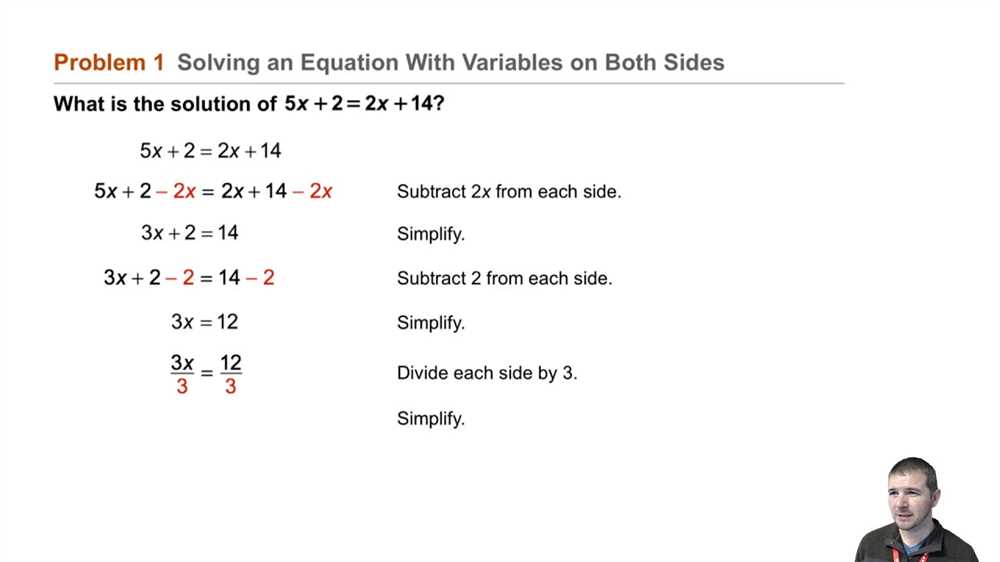
Solve the equation 3x + 7 = 16 for x.
Answer: To solve the equation 3x + 7 = 16, we need to isolate x. Subtract 7 from both sides: 3x + 7 – 7 = 16 – 7, which simplifies to 3x = 9. Then, divide both sides by 3: 3x/3 = 9/3, which gives x = 3. Therefore, the solution to the equation is x = 3.
These are just a few examples of the types of questions you may encounter on an Algebra 1 functions test. Make sure to practice solving equations, evaluating functions, and identifying domain and range to prepare for similar questions.
Question 1
f(-3/2) = 2(-3/2) – 5
Simplifying the expression, we get:
- f(-3/2) = -3 – 5
- f(-3/2) = -8
Therefore, when x = -3/2, the value of f(x) is -8.
Question 2
In question 2 of the Algebra 1 functions test, students were asked to solve a system of equations. The system of equations was given as:
y = 3x – 2
y = -2x + 5
To solve the system of equations, students were required to find the values of x and y that satisfy both equations. This can be done by either substitution or elimination method. Substitution involves solving one equation for one variable and substituting it into the other equation. Elimination method involves adding or subtracting the equations to eliminate one variable and then solving for the remaining variable.
To solve this specific system of equations, substitution method can be used. We can solve the first equation for y:
y = 3x – 2
Now, we can substitute this expression for y into the second equation:
3x – 2 = -2x + 5
Next, we solve for x by rearranging the equation:
3x + 2x = 5 + 2
5x = 7
x = 7/5
Now that we have the value of x, we can substitute it back into the first equation to find the value of y:
y = 3(7/5) – 2
y = 3(7/5) – (10/5)
y = 21/5 – 10/5
y = 11/5
Therefore, the solution to the system of equations is x = 7/5 and y = 11/5.
Answer key for question 1
Question 1: Solve the following equation for x: 3x + 5 = 17
Answer: To solve this equation, we need to isolate x on one side of the equation. First, we can subtract 5 from both sides:
3x + 5 – 5 = 17 – 5
3x = 12
Next, we can divide both sides of the equation by 3 to solve for x:
3x/3 = 12/3
x = 4
Therefore, the solution to the equation 3x + 5 = 17 is x = 4.
Answer key for question 2
The question 2 of the Algebra 1 function test asks students to solve a given equation for a specific variable. The equation provided is:
2x + 5y = 10
To solve this equation for x, one must isolate the variable by moving the other terms to the other side of the equation. Here are the steps to solve the equation:
- Subtract 5y from both sides of the equation: 2x = 10 – 5y
- Divide both sides of the equation by 2 to isolate x: x = (10 – 5y) / 2
Therefore, the answer key for question 2 is x = (10 – 5y) / 2. This equation represents the solution for x when the value of y is given.
Tips for preparing for Algebra 1 functions test
Preparing for an Algebra 1 functions test can seem intimidating, but with the right approach and strategies, you can feel more confident and prepared. Here are some tips to help you prepare effectively:
1. Review the key concepts
Start by reviewing the key concepts and definitions related to functions. Understand the basic properties of functions, such as domain, range, and functions notation. Make sure you can confidently solve equations and inequalities involving functions.
2. Practice solving function problems

The best way to improve your problem-solving skills is through practice. Solve a variety of function problems, including finding the slope of a line, graphing linear and quadratic functions, and analyzing function transformations. Familiarize yourself with different types of functions and their graphs.
3. Understand function notation
Function notation is crucial in Algebra 1 functions. Make sure you understand how to interpret and use function notation correctly. Practice translating verbal descriptions into algebraic expressions and vice versa.
4. Memorize key formulas
There are certain formulas and relationships that you need to know for the functions test. Memorize formulas for slope, midpoint, and distance between two points. Also, review the quadratic formula and its applications.
5. Seek help if needed
If you’re struggling with any concepts or problems, don’t hesitate to seek help. Ask your teacher or classmates for clarification. Consider working with a tutor or joining a study group to get additional support and guidance.
By following these tips and putting in consistent effort, you can effectively prepare for your Algebra 1 functions test. Remember to manage your time well, stay organized, and practice regularly. Good luck!