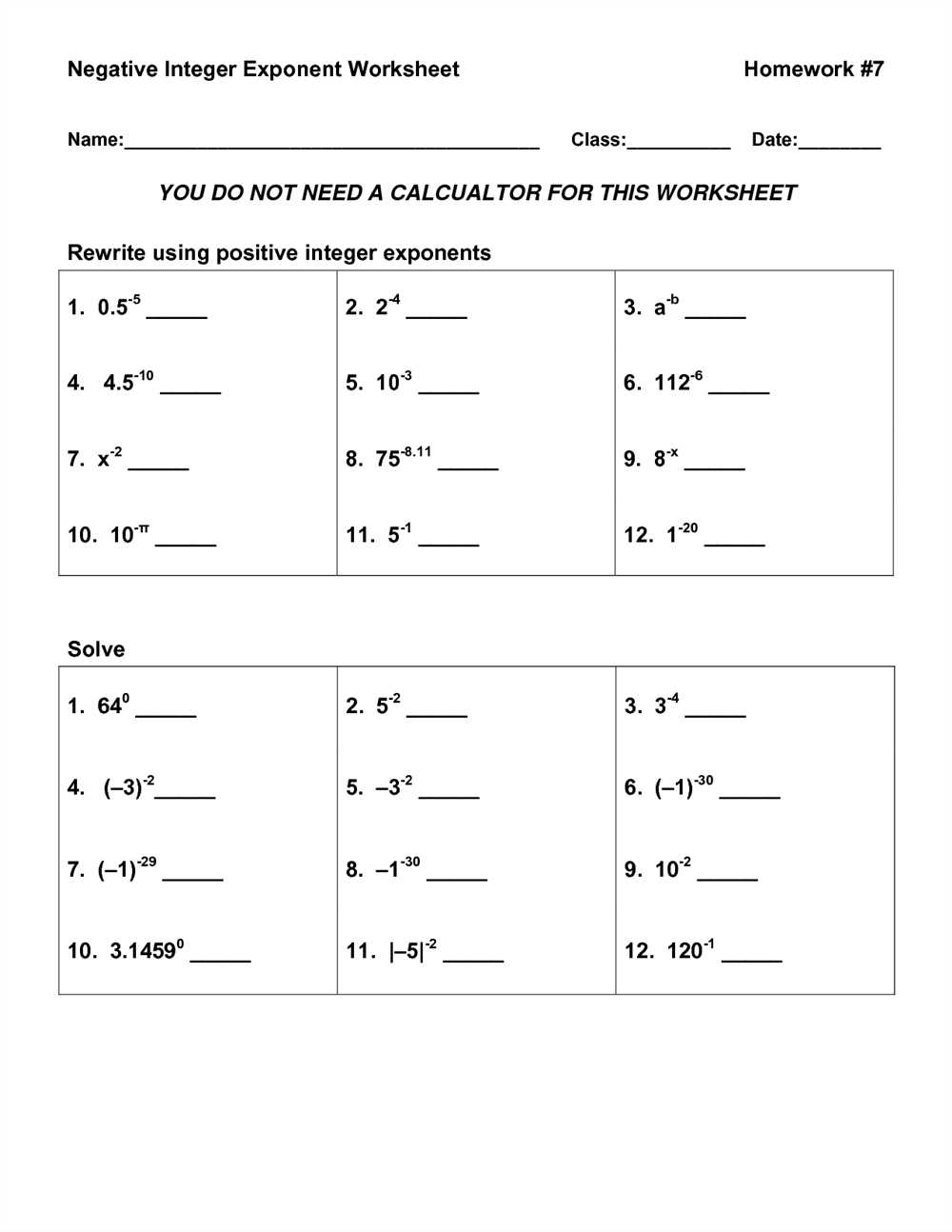
Exponents are an important concept in mathematics that allow us to express and manipulate numbers in a more efficient way. They are often used in equations and formulas, and understanding how to work with exponents is crucial for success in many areas of math and science.
The “I Love Exponents” worksheet is a practice exercise that provides students with a series of problems to solve involving exponents. This answer key will help you check your work and ensure that you are on the right track.
By using this answer key, you can quickly verify if you have correctly simplified expressions with exponents, correctly evaluated exponents, and correctly solved problems involving operations with exponents. It is a valuable tool for self-assessment and for teachers to assess their students’ understanding of exponents.
Remember, when working with exponents, it is important to understand the rules and properties that govern their manipulation. This answer key will not only provide you with the correct answers but also serve as a guide to understanding the techniques and steps involved in solving problems with exponents.
Answer Key: I Love Exponents Worksheet Answers

In the world of mathematics, exponents play a crucial role in solving complex equations. The “I Love Exponents” worksheet aims to test students’ understanding of exponents and how they can be applied in various mathematical operations. Let’s take a closer look at the answer key for this worksheet to better comprehend the concepts involved.
Question 1: Evaluate the expression 23.
- Answer: 8
Question 2: Simplify the expression 52 + 32.
- Answer: 34
Question 3: What is the value of 60?
- Answer: 1
Question 4: Solve the equation x4 = 16 for x.
- Answer: x = 2 or x = -2
Question 5: Simplify the expression (23)2.
- Answer: 64
Question 6: Evaluate the expression (-3)2.
- Answer: 9
Question 7: Simplify the expression 42 ÷ 23.
- Answer: 8
Question 8: Solve the equation 2x = 16 for x.
- Answer: x = 4
Question 9: Simplify the expression 51 + 30 – 22.
- Answer: 4
Question 10: Evaluate the expression (23)2 ÷ 24.
- Answer: 2
This answer key provides the correct solutions to the various exponent-related questions in the “I Love Exponents” worksheet. By understanding and mastering the rules and properties of exponents, students can perform accurate calculations and solve equations efficiently.
The Importance of Understanding Exponents
Exponents are a fundamental concept in mathematics, and understanding them is crucial for success in various areas of study, including algebra, calculus, and physics. Exponents represent repeated multiplication or division of a number by itself, and they play a central role in simplifying numerical expressions and solving equations.
Exponents enhance computational efficiency: One of the key benefits of understanding exponents is that they allow for efficient calculations. By using exponents, we can represent large numbers or repeated operations in a concise and manageable way. For example, instead of writing out 2 × 2 × 2 × 2 × 2, we can simply write 2^5. This makes calculations faster and easier, especially when dealing with complex equations or large numbers.
Exponents enable exponential growth and decay: Exponents also play a crucial role in understanding exponential growth and decay. Many real-world phenomena, such as population growth, compound interest, and radioactive decay, can be modeled using exponential functions. Being able to work with exponents allows us to analyze and predict the behavior of these systems, providing valuable insights in fields like finance, biology, and physics.
Exponents connect various branches of mathematics: Understanding exponents helps establish connections between different areas of mathematics. Exponents are extensively used in algebra, where they are central to polynomial equations, factoring, and solving systems of equations. Furthermore, exponents are essential in calculus, as they are used to represent rates of change and exponential functions. Having a solid grasp of exponents allows students to navigate these advanced mathematical concepts with ease.
Overall, understanding exponents is essential for building a strong foundation in mathematics. They provide a powerful tool for efficient calculations, enable the study of exponential growth and decay, and establish connections between different areas of mathematics. By mastering exponents, students can unlock a multitude of problem-solving techniques and enhance their understanding of the world around them.
Step-by-Step Solutions to I Love Exponents Worksheet
Exponents are an important concept in mathematics that represent repeated multiplication of a number by itself. The I Love Exponents Worksheet is designed to help students practice their understanding of exponents and apply them in various problems. This step-by-step solution guide will explain how to solve each problem on the worksheet.
1. What is 3 raised to the power of 4?
To find 3 raised to the power of 4, we need to multiply 3 by itself four times. So, 34 = 3 x 3 x 3 x 3 = 81.
2. Simplify 23 + 52.
First, we calculate 23 = 2 x 2 x 2 = 8. Then, we calculate 52 = 5 x 5 = 25. Finally, we add the two results together: 8 + 25 = 33.
3. Evaluate (42)3.
To solve this problem, we need to raise 4 to the power of 2 and then raise the result to the power of 3. First, 42 = 4 x 4 = 16. Then, (42)3 = 163 = 16 x 16 x 16 = 4096.
4. Simplify 60 + 15.
Any number raised to the power of 0 is equal to 1. So, 60 = 1. Also, any number raised to the power of 1 is equal to itself. Therefore, 15 = 1. Finally, 60 + 15 = 1 + 1 = 2.
5. Evaluate (24)0.
Raising any number (except 0) to the power of 0 is always equal to 1. So, (24)0 = 1.
By following these step-by-step solutions, students can effectively complete the I Love Exponents Worksheet and deepen their understanding of exponents.
Common Mistakes to Avoid
In the study of exponents, it is important to be aware of common mistakes that students often make. By understanding these mistakes, you can avoid them and improve your understanding of this mathematical concept.
Mistake #1: Forgetting to apply the exponent to all parts of the expression. One common error is forgetting to raise all parts of the expression to the given exponent. For example, if you have (2x)^2, you must square both the 2 and the x to obtain the correct answer.
Mistake #2: Misapplying the rules of exponents. Another mistake often made is misapplying the rules of exponents. It is important to review these rules and understand when they apply. For instance, when dividing two exponents with the same base, you subtract the exponents.
Mistake #3: Incorrectly simplifying expressions. Many students make mistakes when simplifying expressions with exponents. It is crucial to carefully simplify each term and combine like terms correctly. Pay attention to the signs and powers of the variables.
Mistake #4: Confusing negative exponents with fractions. Negative exponents and fractions are often confused. Remember that a negative exponent indicates that the base should be inverted and raised to the positive exponent.
Mistake #5: Using wrong properties of exponents. Finally, be cautious of using incorrect properties of exponents. It is essential to use the correct properties when simplifying or solving equations involving exponents, such as the power rule and product rule.
By being aware of these common mistakes and practicing regularly, you can improve your skills in working with exponents and avoid errors that can hinder your understanding and performance in this mathematical area.
Additional Practice Problems
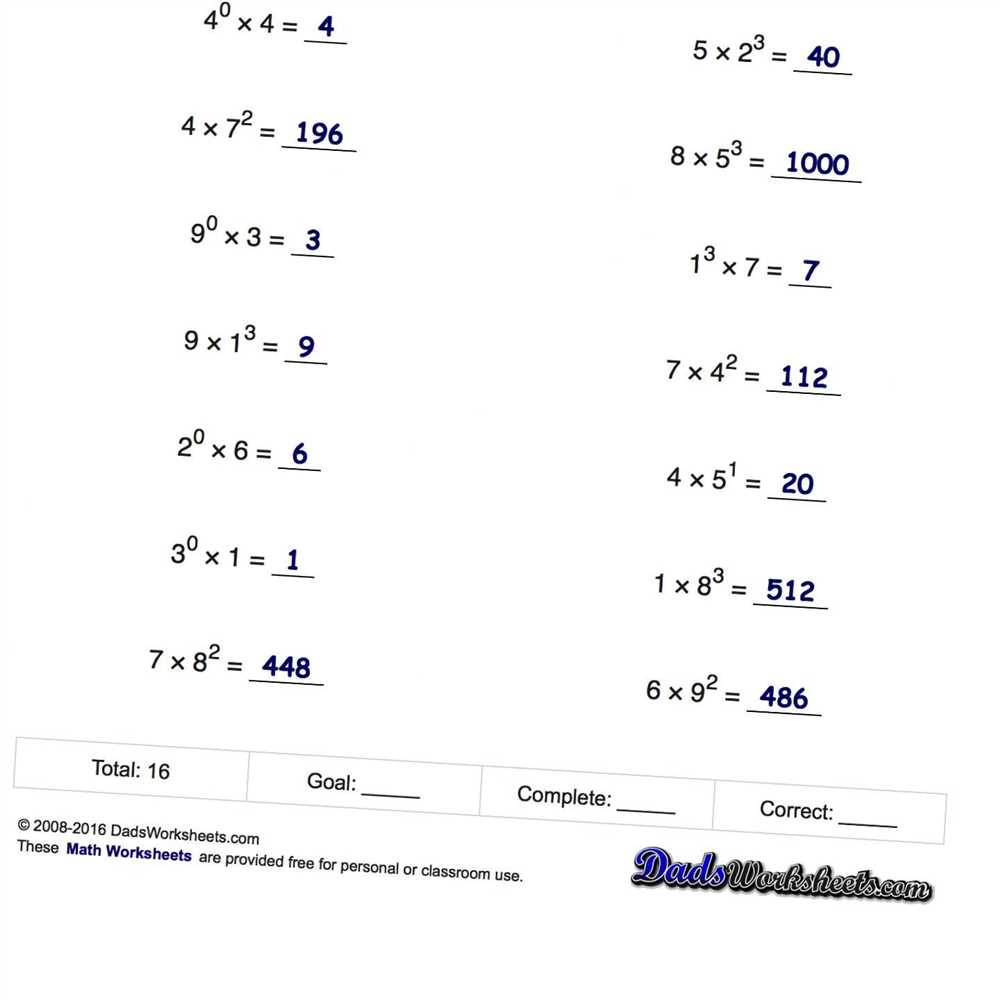
Looking for some extra practice with exponents? Here are a few additional problems to help reinforce your understanding of this important concept.
Problem 1:
Simplify the expression: 23 + 42
To solve this problem, we first evaluate the exponents: 23 = 2 x 2 x 2 = 8 and 42 = 4 x 4 = 16. Then, we add the results together: 8 + 16 = 24. Therefore, the simplified expression is 24.
Problem 2:
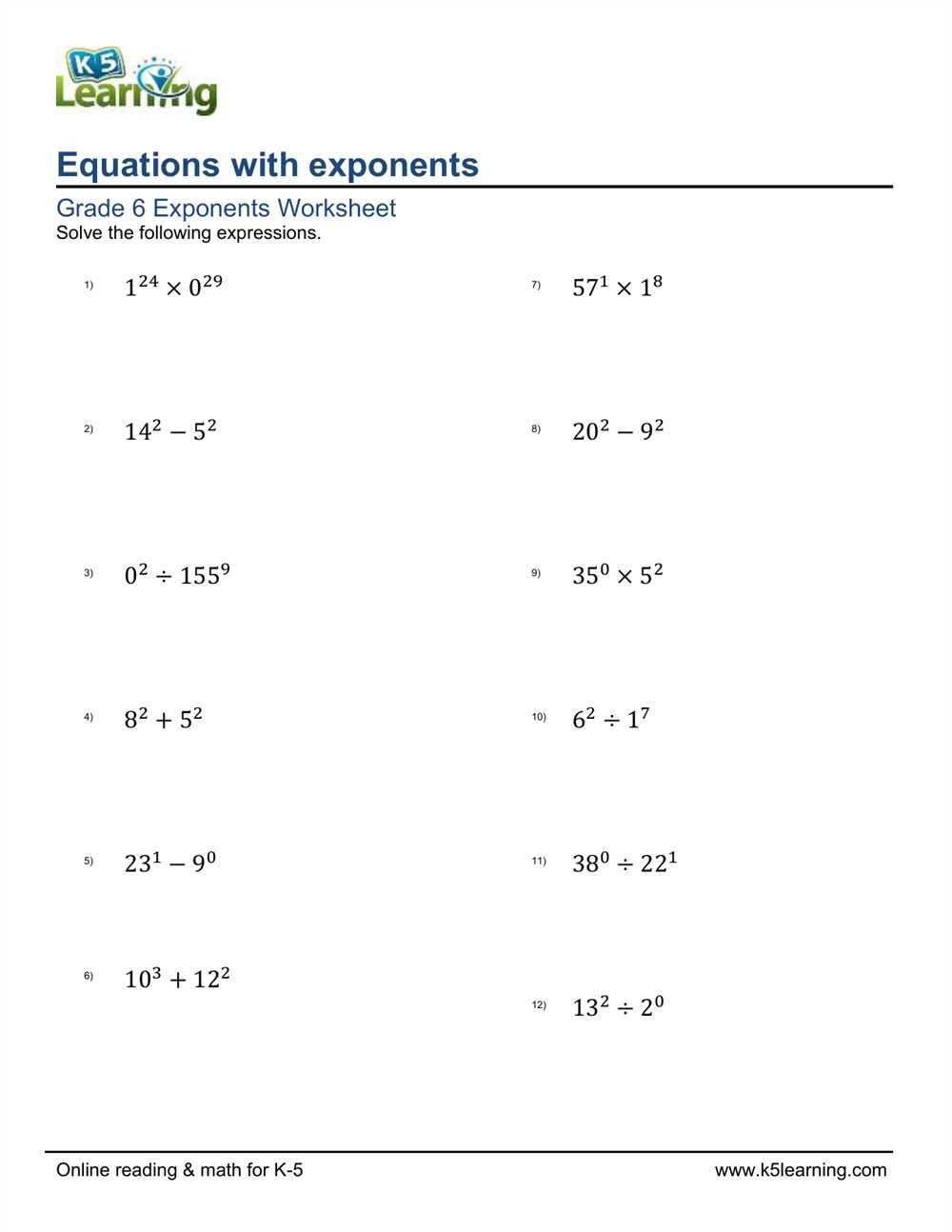
Calculate the value of the expression: (52)3
To simplify this expression, we need to multiply the exponents: (52)3 = 52 x 3 = 56. Therefore, the value of the expression is 56.
Problem 3:
Simplify the expression: 24 – 32
To solve this problem, we first evaluate the exponents: 24 = 2 x 2 x 2 x 2 = 16 and 32 = 3 x 3 = 9. Then, we subtract the results: 16 – 9 = 7. Therefore, the simplified expression is 7.
Problem 4:
Calculate the value of the expression: (42)2
To simplify this expression, we need to multiply the exponents: (42)2 = 42 x 2 = 44. Therefore, the value of the expression is 44.
Remember, practicing with these problems will help solidify your understanding of exponents and allow you to confidently solve more complex equations involving exponents in the future.
Exploring Real-Life Applications of Exponents
Exponents play a crucial role in various real-life scenarios where quantities grow or decrease at exponential rates. Understanding and applying exponents can empower individuals to analyze and predict outcomes in fields such as finance, biology, physics, and computer science.
Finance: Exponents are frequently used in financial calculations, such as compound interest. For instance, when calculating the growth of an investment, the formula A = P(1 + r/n)^(nt) is used, where A represents the final amount, P is the principal investment, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the number of years. By using exponents, individuals can quickly determine the future value of their investments and make informed financial decisions.
Biology: In biology, exponents are utilized to model population growth. The exponential growth model is often applied to study the growth of bacteria or the spread of diseases. By understanding the exponential growth equation, N = N₀ * e^(rt), where N represents the population at a given time, N₀ is the initial population, e is Euler’s number (approximately 2.71828), r is the growth rate, and t is the time, scientists can estimate population sizes and predict the behavior of biological systems.
Physics: In physics, exponents are frequently encountered when dealing with radioactive decay. The half-life of a radioactive substance is the time it takes for half of the substance to decay. The exponential decay equation, N = N₀ * 0.5^(t/h), describes this process, where N represents the remaining amount, N₀ is the initial amount, t is the time elapsed, and h is the half-life. By utilizing exponents, physicists can accurately determine the decay rate of radioactive substances and make calculations for various applications, including radiocarbon dating.
Computer Science: Exponents are also fundamental in computer science and the study of algorithms. Efficient algorithms often use techniques involving exponents to optimize search, sort, and matrix multiplication operations. By analyzing and understanding the behavior of algorithms with exponents, computer scientists can develop faster and more efficient programs.
Overall, exponents provide a valuable tool for understanding and making predictions in a wide range of real-life applications. Whether it’s managing finances, predicting population growth, studying radioactive decay, or optimizing computer algorithms, the understanding of exponents can greatly enhance decision-making and problem-solving abilities.
Tips and Tricks for Working with Exponents
Exponents are a fundamental concept in mathematics, and understanding how to work with them is crucial for solving equations and simplifying expressions. Here are some tips and tricks to help you navigate the world of exponents:
1. Understanding the Basic Rules

- When multiplying numbers with the same base, add the exponents.
- When dividing numbers with the same base, subtract the exponents.
- When raising a number to a power, multiply the exponents.
- Any number raised to the power of zero equals 1.
- Any number raised to the power of one equals itself.
2. Simplifying Expressions
Simplifying expressions with exponents can often make them easier to work with. Some strategies to simplify expressions include:
- Using the rules of exponents to combine like terms and reduce the number of variables in the expression.
- Factoring out common factors and simplifying the remaining terms.
- Applying the distributive property to simplify expressions with multiple terms.
- Using the power of a power rule to simplify expressions with multiple exponents.
3. Strategies for Problem Solving
When solving problems involving exponents, it can be helpful to:
- Identify the base and the exponent in the equation.
- Look for patterns or relationships between the given numbers or variables.
- Apply the appropriate rule of exponents to simplify the equation.
- Use algebraic manipulation to isolate the variable and find its value.
- Check your solution by substituting the value back into the original equation.
By understanding the basic rules of exponents, simplifying expressions, and employing effective problem-solving strategies, you’ll gain confidence in working with exponents and be better equipped to tackle more complex mathematical problems.