
Understanding the concepts of area and circumference is crucial in the field of mathematics and geometry. These two measurements play a significant role in calculating the size and dimensions of various shapes and objects. In this article, we will provide an answer key to help you solve problems related to the area and circumference of different geometric figures.
Calculating the area of a shape involves determining the amount of space inside that particular shape. It is typically measured in square units, such as square inches or square centimeters. To find the area of a rectangle, multiply its length by its width. Similarly, the formula for calculating the area of a triangle is 1/2 times the base multiplied by the height.
The circumference of a shape refers to the distance around its outer boundary. It is an essential measurement when dealing with circular objects. The formula for finding the circumference of a circle is 2 times pi (π) times the radius. Pi is an irrational number approximately equal to 3.14 or 22/7, while the radius is the distance from the center of the circle to any point on its circumference.
By having access to this answer key, you will be able to check your solutions and verify the correctness of your calculations. Whether you are a student studying geometry or a professional working with shapes and measurements, this answer key will serve as a valuable resource in solving problems related to area and circumference. So let’s dive in and explore the world of mathematics and geometry together!
What is the area formula for a circle?
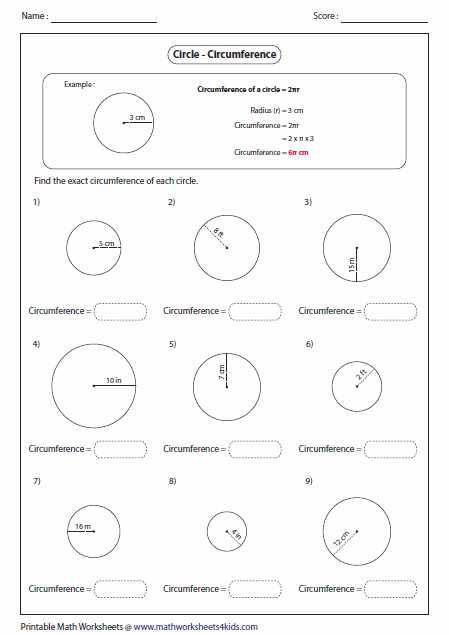
A circle is a geometric shape that is defined as a set of points on a plane that are equidistant from a fixed center point. The area of a circle is the measure of the surface enclosed by its boundary. The formula to calculate the area of a circle is:
Area = π * r2
Where π (pi) is a mathematical constant approximately equal to 3.14159, and r is the radius of the circle. The radius is the distance from the center of the circle to any point along its boundary.
This formula can be derived using calculus or by dividing the circle into infinitesimally small sectors and then adding up their areas. However, for practical purposes, the formula is often used to quickly calculate the area of a circle in real-world applications.
The area of a circle is always expressed in square units, such as square inches, square centimeters, or square meters, depending on the unit of measurement used for the radius. It is important to note that the area represents the amount of space inside the circle and does not include the boundary itself.
How to calculate the circumference of a circle?
In order to calculate the circumference of a circle, you need to know the radius or the diameter of the circle. The circumference, represented by the symbol “C”, is the distance around the circle. It is found by multiplying the diameter of the circle by the mathematical constant pi (π), which is approximately 3.14159. Therefore, the formula to calculate the circumference of a circle is:
C = 2πr or C = πd
Where:
- C represents the circumference
- π (pi) is a mathematical constant approximately equal to 3.14159
- r represents the radius of the circle
- d represents the diameter of the circle
If you have the radius of the circle, you can simply plug it into the formula C = 2πr. If you have the diameter instead, you can use the formula C = πd. By substituting the appropriate value and performing the necessary calculations, you will be able to determine the circumference of the circle.
It is important to note that the circumference is always greater than the diameter of the circle. In fact, the circumference is exactly pi times the diameter. This relationship is true for all circles, regardless of their size.
Calculating the circumference of a circle is a fundamental mathematical concept that has practical applications in various fields, such as engineering, architecture, and physics. Knowing the circumference of a circle can help professionals determine the length of curved objects or plan the layout of circular structures. It is also a key component in formulas for calculating other circle-related measurements, such as the area of the circle.
Understanding the relationship between area and circumference
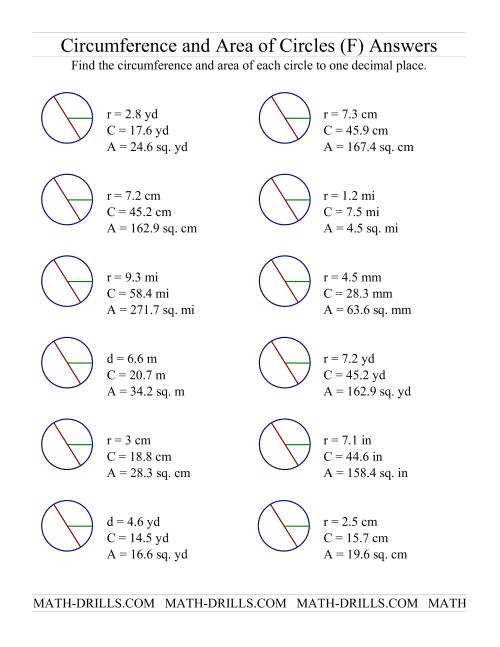
The relationship between the area and circumference of a shape, especially a circle, is an important concept in geometry. It helps us understand the connections and dependencies between these two measurements.
The area of a shape refers to the amount of space enclosed by its boundaries. In the case of a circle, the area can be calculated using the formula A = πr^2, where A represents the area and r represents the radius of the circle. This formula indicates that the area is directly proportional to the square of the radius. In other words, increasing the radius of a circle will result in a greater area.
- Closer Look at Circumference and Diameter
The circumference of a shape, on the other hand, refers to the length of its boundary. For a circle, the circumference is given by the formula C = 2πr, where C represents the circumference and r represents the radius. This formula shows that the circumference is directly proportional to the radius. Therefore, increasing the radius of a circle will result in a greater circumference.
The relationship between the area and circumference of a circle can be further understood by examining the diameter. The diameter is a straight line passing through the center of the circle, and it is exactly twice the length of the radius. This means that if we double the radius, we also double the diameter. Consequently, the area and circumference will be quadrupled because the area is influenced by the square of the radius, while the circumference is directly proportional to the radius.
- The Concept of Pi
Another key aspect of understanding the relationship between the area and circumference of a circle is the concept of π (pi). Pi is an irrational number approximately equal to 3.14159 and is used as a constant in the formulas mentioned earlier. Pi represents the ratio between the circumference and the diameter of a circle. It is a fundamental factor that relates the measurements of a circle with its area and circumference.
In conclusion, understanding the relationship between the area and circumference of a shape, particularly a circle, involves knowing the formulas for calculating these measurements and recognizing their dependencies on the radius and diameter. Additionally, comprehending the concept of pi as a constant factor further enhances our understanding of this relationship. These concepts and formulas are essential for solving problems related to the area and circumference of circles and other geometric shapes.
Why is π used in the formulas for circle?
The value of π, approximately 3.14159, is used in the formulas for circles because it is a mathematical constant that represents the relationship between a circle’s circumference and its diameter. π is an irrational number, which means its decimal representation goes on forever without repeating, making it an ideal constant for circle calculations.
In the formulas for a circle’s area and circumference, π is used to establish the inherent relationship between the two measurements. The diameter of a circle is equal to twice the radius, and the circumference of a circle is equal to π times the diameter. This relationship allows mathematicians and engineers to easily calculate the circumference of a circle when given its diameter or radius, or vice versa.
The use of π in circle formulas dates back thousands of years, with the concept first being introduced by ancient civilizations such as the Egyptians and Babylonians. The ancient Greeks, particularly the mathematician Archimedes, made significant contributions to understanding the properties of circles and the value of π. Throughout history, mathematicians have continued to refine the value of π using various methods, but it remains an irrational number with infinite decimal places.
π is a fundamental constant in mathematics and is used not only in circle calculations but also in many other areas of mathematics and science. Its precise value is vital for accurate calculations and measurements involving circles, such as determining the area of a circular field, the volume of a cylinder, or the trajectory of a satellite in orbit around a planet.
In conclusion, π is used in the formulas for circles because it represents the relationship between a circle’s circumference and its diameter. It is a fundamental constant in mathematics and has been used for centuries to calculate various properties of circles and other related geometric shapes.
Key concepts for solving area and circumference problems
Solving area and circumference problems requires a good understanding of key concepts related to these geometrical measurements. Here are some important concepts to keep in mind when approaching such problems:
Circles
- Radius: The distance from the center of a circle to any point on its circumference.
- Diameter: The distance across a circle, passing through its center, and equal to twice the radius.
- Circumference: The perimeter of a circle, or the distance around its outer boundary, given by the formula C=2πr, where r is the radius.
Area of a circle
The area of a circle is the measure of the interior of the circle and can be calculated using the formula:
A = πr2
where A represents the area and r represents the radius.
General approach to solving area and circumference problems
When faced with a problem involving area or circumference, it is important to carefully read the problem statement and identify the given information and what is being asked for. Next, apply the appropriate formulas and solve the equation or expression to find the desired result.
It is also helpful to sketch a diagram or visualize the problem to better understand the geometric configuration and the relationships between the different components. Pay attention to units of measurement and make sure to convert them if necessary to ensure consistency.
Practice and familiarity with these key concepts and formulas will enable you to confidently solve area and circumference problems and apply them to real-life situations.
Common mistakes to avoid when calculating area and circumference
When calculating the area and circumference of shapes, it is important to be aware of certain common mistakes that can easily be made. By avoiding these mistakes, you can ensure accurate and reliable calculations.
1. Forgetting to use the correct formulas: One of the most common mistakes when calculating area and circumference is using the wrong formulas. Each shape has its own specific formula, so it is crucial to double-check and use the correct one. Whether it is the formula for the area of a circle or the circumference of a rectangle, make sure you are using the right formula for the shape you are working with.
2. Misinterpreting measurements: Another common mistake is misinterpreting measurements. It is important to carefully read and understand the given measurements before applying them to the formulas. Whether it is the radius of a circle or the length of a side of a square, be sure to correctly identify and use the appropriate measurement in your calculations.
3. Rounding errors: Rounding errors can also lead to inaccurate calculations. When rounding measurements or intermediate results, it is important to follow the correct rounding rules and be aware of the precision required. Failure to do so can lead to significant errors in the final calculations of area and circumference.
4. Forgetting to convert units: If the measurements are given in different units, it is essential to convert them to a common unit before performing calculations. Failure to convert units can result in incorrect calculations and unreliable results. Always double-check and ensure that all measurements are in the same unit before proceeding with calculations.
5. Not using parentheses correctly: When performing calculations involving multiple operations, it is crucial to use parentheses correctly to ensure the correct order of operations. Incorrect use of parentheses can lead to errors in the final result of area and circumference. Always remember to carefully place parentheses where needed and follow the correct order of operations.
By avoiding these common mistakes, you can ensure accurate and reliable calculations of area and circumference for various shapes. Double-checking formulas, correctly interpreting measurements, being mindful of rounding errors, converting units, and using parentheses correctly are all essential steps to achieve accurate results.
Step-by-step examples: calculating the area and circumference of circles
Calculating the area and circumference of circles is a fundamental skill in geometry. To find the area of a circle, you can use the formula A = πr^2, where A represents the area and r represents the radius of the circle. The circumference, on the other hand, can be calculated using the formula C = 2πr, where C represents the circumference.
Let’s take an example to understand the process step by step. Suppose we have a circle with a radius of 3 units. To find the area, we substitute the value of the radius into the formula A = πr^2. Therefore, A = π(3^2) = π(9) = 9π square units. So, the area of the circle is 9π square units.
Next, let’s find the circumference of the same circle. We use the formula C = 2πr and substitute the value of the radius. Therefore, C = 2π(3) = 6π units. So, the circumference of the circle is 6π units.
In another example, let’s consider a circle with a diameter of 10 units. To find the radius, we divide the diameter by 2, which gives us a radius of 5 units. Using the formula A = πr^2, we can calculate the area as A = π(5^2) = π(25) = 25π square units. Thus, the area of the circle is 25π square units.
Similarly, to find the circumference using the formula C = 2πr, we substitute the radius value of 5 units. Therefore, C = 2π(5) = 10π units. So, the circumference of the circle is 10π units.
These step-by-step examples illustrate how to calculate the area and circumference of circles. Remember to use the correct formulas and units when solving problems involving circles to ensure accurate results.