Are you looking for the answers to the Unit 4 Review in the Big 10 textbook? Well, you’ve come to the right place! In this article, we will provide you with the answers to all the questions in the Unit 4 Review section.
Unit 4 focuses on various topics, such as grammar, vocabulary, and reading comprehension. It challenges students to apply their knowledge and skills in different contexts. The review section at the end of the unit helps students consolidate what they have learned and assess their understanding.
In this article, we will provide comprehensive answers to all the questions in the Unit 4 Review. Whether you are a student preparing for an upcoming test or a teacher looking for a resource to check your students’ answers, this article will be a valuable reference for you.
So, let’s dive into the Unit 4 Review answers and make sure you have a solid understanding of the topics covered in this unit. Whether it’s identifying correct verb tenses, selecting the appropriate vocabulary words, or comprehending a passage, we’ve got you covered!
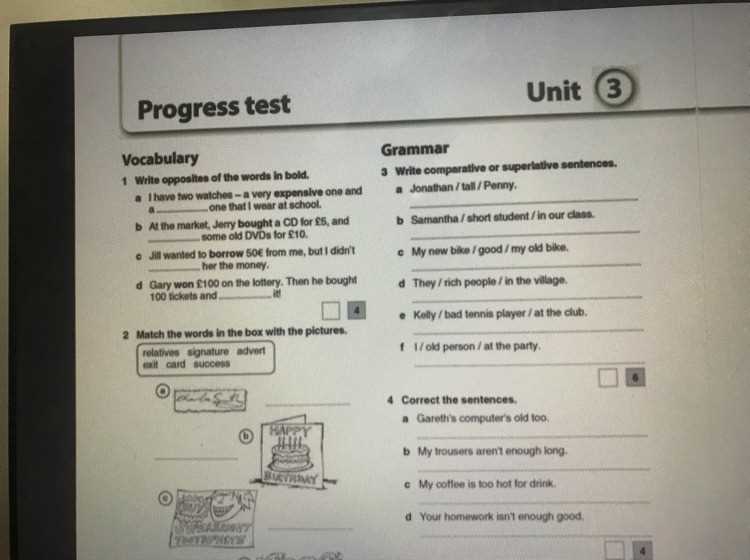
Big 10 Unit 4 Review Answers

As we review Unit 4 of the Big 10 curriculum, we will focus on the key concepts and provide the corresponding answers to the review questions. Unit 4 covers various topics, including algebraic expressions, equations, inequalities, functions, and graphing.
Expressions and Equations: The first part of Unit 4 focuses on understanding and simplifying algebraic expressions. Students learn how to combine like terms, solve equations with one variable, and solve literal equations. They also practice simplifying expressions with exponents and evaluating expressions by substituting values for variables.
Inequalities: The second part of Unit 4 delves into solving and graphing inequalities. Students learn how to solve inequalities with one variable, including both compound and absolute value inequalities. They also study graphing linear inequalities on a number line and coordinate plane.
Functions and Graphing: The final part of Unit 4 covers functions and graphing. Students learn about the concept of a function and the relationship between inputs and outputs. They also practice graphing linear equations and inequalities, as well as identifying key features of a graph, such as the x-intercept, y-intercept, and slope.
In conclusion, Unit 4 of the Big 10 curriculum provides a comprehensive overview of algebraic expressions, equations, inequalities, functions, and graphing. By reviewing the key concepts and corresponding answers, students can solidify their understanding and master these fundamental mathematical skills.
Answering Key Questions
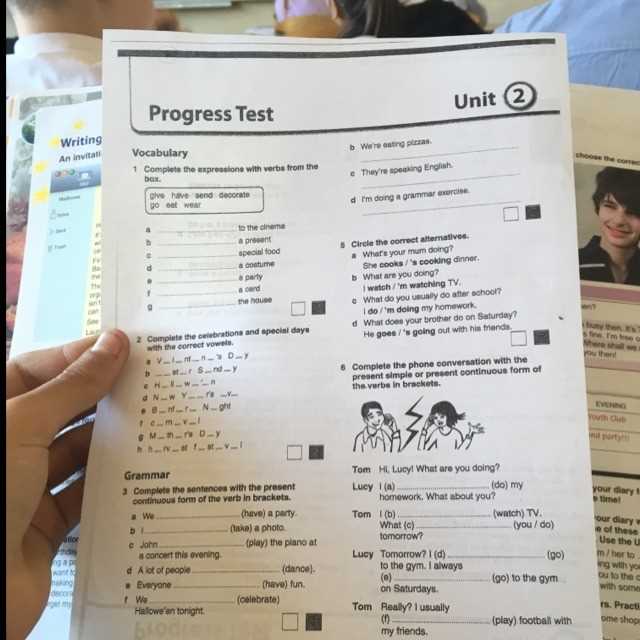
When it comes to answering key questions, it is essential to have a clear understanding of the topic at hand. Key questions often serve as the foundation for further exploration and analysis, allowing us to gain deeper insights and develop well-rounded perspectives. Whether it is in a classroom setting, a research project, or a business context, answering key questions helps to drive intellectual growth and problem-solving.
One important aspect of answering key questions is formulating the right questions to begin with. Asking clear and concise questions sets the stage for a focused inquiry and helps guide the research or discussion in the right direction. By identifying the key aspects of the topic and thinking critically about what specific information is needed, we can shape our questions to target the most important areas of investigation.
Another crucial element of answering key questions is gathering accurate and reliable information. With the vast amount of information available today, it is essential to critically evaluate sources and select those that provide trustworthy and relevant data. By utilizing credible sources and conducting thorough research, we can ensure that our answers are based on solid evidence and logical reasoning.
Once we have gathered the necessary information, it is important to analyze and interpret the data effectively. By employing critical thinking skills and applying relevant frameworks or theories, we can make sense of the information and draw meaningful conclusions. This analytical process allows us to go beyond simple facts and figures and develop a more comprehensive understanding of the topic.
In conclusion, answering key questions requires a combination of critical thinking, research skills, and the ability to effectively communicate our findings. By formulating focused and relevant questions, gathering accurate information, and analyzing the data, we can provide well-informed answers that contribute to our understanding of the topic at hand. Whether it is in academic, professional, or personal contexts, the ability to answer key questions is a valuable skill that fosters intellectual growth and problem-solving abilities.
Understanding Key Concepts
When studying any subject or topic, it is essential to have a clear understanding of the key concepts that form its foundation. Key concepts are the fundamental ideas or principles that underpin a particular subject area and help to provide structure and coherence to the learning process. By mastering these key concepts, students can develop a deeper understanding of the subject matter and effectively apply their knowledge to real-world situations.
Identifying key concepts: Before delving into the details of a subject, it is important to identify the key concepts that are central to understanding it. These concepts often serve as the building blocks upon which more complex ideas are formed. To identify key concepts, students should pay attention to recurring themes, core principles, and foundational theories or models within the subject area.
Understanding the interrelationships: Key concepts are rarely isolated entities; instead, they are interconnected and interdependent. As such, one must strive to understand the interrelationships between different key concepts to gain a holistic understanding of the subject. Recognizing how different concepts relate to and influence one another can help students develop a more comprehensive understanding of the topic.
Applying knowledge: Understanding key concepts goes beyond mere memorization and regurgitation of information. It involves the ability to apply knowledge and principles to new situations and scenarios. By grasping the fundamental concepts of a subject, students can transfer their understanding to real-world problems, make connections between different topics, and think critically to solve complex issues.
Continual learning and revision: Key concepts are often foundational and may require continual learning and revision to fully comprehend. As students progress in their studies, they may discover new connections and nuances that deepen their understanding of these concepts. Regular review and revision are therefore crucial to ensure a solid grasp of the key concepts and their applications.
Overall, understanding key concepts is essential for successful learning and knowledge application. By mastering these foundational principles, students can develop a deeper understanding of a subject area and apply their knowledge effectively in various contexts.
Exploring Vocabulary

When it comes to language learning, one of the key aspects is expanding your vocabulary. Having a strong vocabulary allows you to communicate more effectively and express yourself with precision. So, let’s dive deep into the world of vocabulary and explore strategies to enhance your language skills.
Contextual Learning: Learning new words in context is essential for better retention. Rather than memorizing word lists, try to learn new words through reading or listening to authentic materials. This way, you can understand how words are used in different contexts and remember them more effectively.
Word Families: English is a language rich with word families. By learning the root word and its derivatives, you can expand your vocabulary quickly. For example, if you learn the word “communicate,” you can also learn related words like “communication,” “communicative,” and “communicator.”
Word Association: Creating associations or connections between new words and existing vocabulary can help you remember them better. For instance, if you come across the word “exquisite,” you can associate it with the word “delicate” or “elegant.”
Mind Maps: Mind mapping is an effective technique for organizing and connecting words visually. By creating a mind map, you can see the relationships between words, which aids in better understanding and memory retention.
Frequency Lists: Another useful strategy is to focus on high-frequency words. Learning the most commonly used words in the language can significantly improve your comprehension and communication skills. Online resources provide frequency lists that you can use as a guide.
In conclusion, exploring vocabulary is an exciting journey that requires dedication and practice. By incorporating these strategies into your language learning routine, you can enhance your vocabulary and become more proficient in English.
Analyzing Sample Problems
When it comes to studying for exams or reviewing material, analyzing sample problems is an effective strategy to strengthen your understanding and prepare yourself for success. By carefully dissecting the components of a problem, you can identify the key concepts, formulas, and steps required to solve it. This analytical approach helps to solidify your knowledge and build problem-solving skills.
Identify the key information: Before attempting to solve a sample problem, it is crucial to identify the key information provided. This includes any given values, equations, or specific conditions mentioned in the problem. Highlight or underline these details to keep track of them as you analyze the problem further.
Break down the problem: Next, break down the problem into smaller parts or steps. By dividing the problem into manageable components, you can better understand the logic behind each step and how they connect to form the solution. This breakdown also allows you to identify any potential shortcuts or alternative approaches that could simplify the problem.
Apply relevant concepts and formulas: Once the problem is broken down, apply the relevant concepts and formulas you have learned to each step. Compare the problem with similar examples or practice questions you have encountered before to ensure you are using the correct principles. This step will strengthen your understanding of the concept being tested and familiarize yourself with the application of formulas.
Evaluate your solution: After solving the problem, evaluate your solution and compare it to the expected outcome or any given information provided in the problem. Pay attention to any errors or mistakes you may have made and understand the reasoning behind the correct solution. This evaluation phase allows you to identify areas where you need improvement and provides an opportunity for further practice.
Practice, practice, practice: Analyzing sample problems should be an ongoing process throughout your study sessions. Continuously practice with different types of problems to reinforce your understanding and develop confidence in your problem-solving abilities. The more you practice, the more comfortable and proficient you will become in tackling different types of problems in your exams.
Practicing Real-Life Scenarios
In order to be prepared for real-life situations, it is crucial to practice various scenarios and learn how to effectively handle them. This is particularly important in the context of Big 10 unit 4 review answers, as it allows students to apply their knowledge in a practical way. By engaging in real-life scenarios, students can develop their critical thinking skills, problem-solving abilities, and decision-making capabilities.
Role-playing: One effective way to practice real-life scenarios is through role-playing. Students can take on different roles and simulate situations that they may encounter in the future. For example, they can act as a customer trying to resolve a complaint, a manager handling a difficult employee, or a team member dealing with a challenging task. By playing different roles, students can gain valuable insights into the complexities of real-life situations and learn how to navigate them effectively.
- Case studies:
Another useful practice method is analyzing and discussing case studies. Case studies provide students with realistic scenarios and allow them to analyze the situation, identify the problem, and propose possible solutions. By examining case studies, students can practice their analytical skills and think critically about the different factors that might be at play. It also encourages collaboration and discussion among students, as they can share their perspectives and learn from each other’s insights.
In conclusion, practicing real-life scenarios is essential for students to develop their skills and prepare for the challenges they may face in the future. It allows them to apply their knowledge in a practical context, develop critical thinking abilities, and learn how to effectively handle various situations. By engaging in role-playing exercises and analyzing case studies, students can gain valuable experience and build their confidence in dealing with real-life scenarios.
Applying Formulas and Equations

When solving mathematical problems, one of the most important skills to have is the ability to apply formulas and equations. These tools allow us to express relationships between different variables and manipulate them to find the desired solution.
Formulas can be found in various fields of mathematics and science, such as geometry, physics, and chemistry. They provide a concise representation of a mathematical relationship and can be used to solve problems involving measurements, quantities, and other variables.
For example, in geometry, the Pythagorean theorem is a well-known formula that relates the lengths of the sides of a right triangle. By using this formula, we can find the length of an unknown side given the lengths of the other two sides.
Equations, on the other hand, are mathematical statements that assert the equality of two expressions. They often involve unknown variables and provide a way to solve for those variables. Equations can be solved by performing various operations, such as addition, subtraction, multiplication, and division, to isolate the unknown variable.
In summary, applying formulas and equations is an essential skill in mathematics and science. They allow us to express relationships between variables and solve problems efficiently. By understanding and utilizing these tools, we can tackle a wide range of mathematical problems and find solutions to complex real-world situations.