In Chapter 10 of geometry, circles are introduced as a new concept to students. Circles are defined as a set of points that are equidistant from a fixed point called the center. This chapter focuses on understanding the properties and relationships of circles, as well as solving problems involving them. To navigate through this chapter successfully, it is essential to have a clear understanding of key terms and formulas related to circles.
One crucial aspect covered in this chapter is the identification of different parts of a circle, such as the radius, diameter, chord, and circumference. The radius is the distance between the center of the circle and any point on its circumference, while the diameter is the distance across the circle passing through the center. A chord is a line segment that connects two points on the circumference of a circle, and the circumference is the boundary of the circle.
Understanding the relationships between these parts is essential for solving various problems related to circles. By using the formulas provided in this chapter, students can determine the length of a chord by applying the Pythagorean theorem or calculate the circumference of a circle using the formula C = 2πr, where r is the radius. These equations, along with other concepts like arc length, area of a sector, and central angles, will be thoroughly explained in the answer key for Chapter 10 circles.
Chapter 10 Circles Answer Key: Understanding the Basics

In Chapter 10 of our mathematics textbook, we delve into the fascinating world of circles. Circles are among the most fundamental geometric shapes, and understanding their properties and relationships is crucial in many areas of mathematics and real-world applications. In this chapter, we explore the basics of circles and provide an answer key to help you grasp the key concepts and solve problems effectively.
One of the fundamental aspects of circles is their definition. A circle is a closed curve consisting of all points in a plane that are equidistant from a fixed point called the center. This definition sets the foundation for understanding the various components and properties of circles. Key terms and properties such as radius, diameter, chord, secant, and tangent are explored in this chapter, and the answer key provides clear explanations and examples to help you comprehend their meanings and relationships.
The answer key explores the following key concepts:
- Calculating the circumference and area of a circle using the formulas πd and πr^2
- Understanding the relationships between various types of angles formed by chords, secants, and tangents
- Identifying and utilizing properties of tangent lines and circles
- Solving problems involving arc lengths and sector areas
The answer key in this chapter serves as a valuable resource to solidify your understanding of the basic concepts and principles related to circles. By mastering these fundamentals, you will be equipped to tackle more advanced topics in geometry and apply your knowledge in various practical scenarios. Whether you are preparing for a test or simply seeking to deepen your understanding of circles, this answer key will assist you in mastering the basics.
Exploring the Parts of a Circle: Radius, Diameter, and Circumference
A circle is a two-dimensional geometric shape that is perfectly round and consists of all points equidistant from a central point. Understanding the different parts of a circle is essential in geometry and can help solve various mathematical problems.
Radius: The radius of a circle is the distance from the center of the circle to any point on its circumference. It is denoted by the letter ‘r’ and is always exactly half the length of the diameter. The radius is used to calculate the area and circumference of a circle.
Diameter: The diameter of a circle is a straight line that passes through the center and connects two points on the circumference. It is the longest chord in a circle and is denoted by the letter ‘d’. The diameter is always twice the length of the radius. The diameter is often used to calculate the circumference and area of a circle.
Circumference: The circumference of a circle is the distance around its outer edge. It is calculated using the formula C = τr or C = πd, where ‘C’ represents the circumference, ‘r’ represents the radius, and ‘d’ represents the diameter. The circumference is an important measurement when it comes to determining the size and length of a circular object.
Understanding the radius, diameter, and circumference of a circle helps in various real-life applications, such as calculating the size of circular objects, constructing circular structures, and determining the curvature of objects in math and science.
- The radius is the distance from the center of the circle to any point on its circumference.
- The diameter is a straight line that passes through the center and connects two points on the circumference.
- The circumference is the distance around the outer edge of the circle.
Calculating Circle Area: Formulas and Examples
In geometry, the area of a circle is a key measurement that can be used to solve various problems and equations. To calculate the area of a circle, we can use the formula A = πr^2, where A represents the area and r represents the radius of the circle. The constant π (pi) is approximately equal to 3.14159 and is a fundamental mathematical constant.
Let’s explore a couple of examples to understand how to calculate the area of a circle using this formula.
Example 1:
Consider a circle with a radius of 5 units. To find the area, we can substitute the radius into the formula:
A = πr^2
A = 3.14159 * 5^2
A = 3.14159 * 25
A ≈ 78.54 square units
Therefore, the area of the circle with a radius of 5 units is approximately 78.54 square units.
Example 2:
Let’s take another circle with a radius of 7.5 units. Using the same formula, we can calculate its area:
A = πr^2
A = 3.14159 * 7.5^2
A = 3.14159 * 56.25
A ≈ 176.71 square units
Hence, the area of the circle with a radius of 7.5 units is approximately 176.71 square units.
Remember, when calculating the area of a circle, it is crucial to ensure that the radius measurement is accurate. Using the correct formula, such as A = πr^2, and substituting in the appropriate values, we can determine the area of any circle.
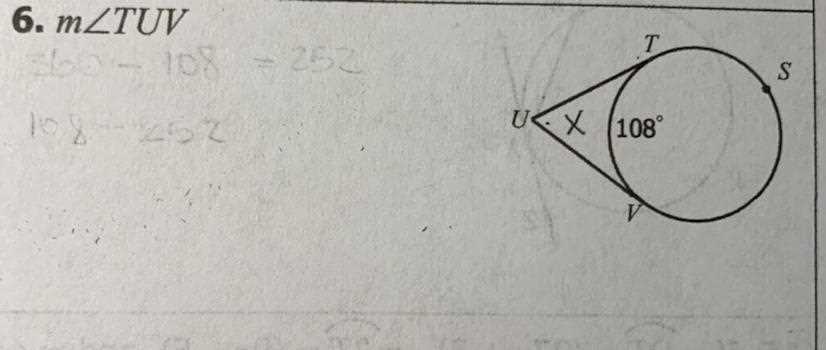
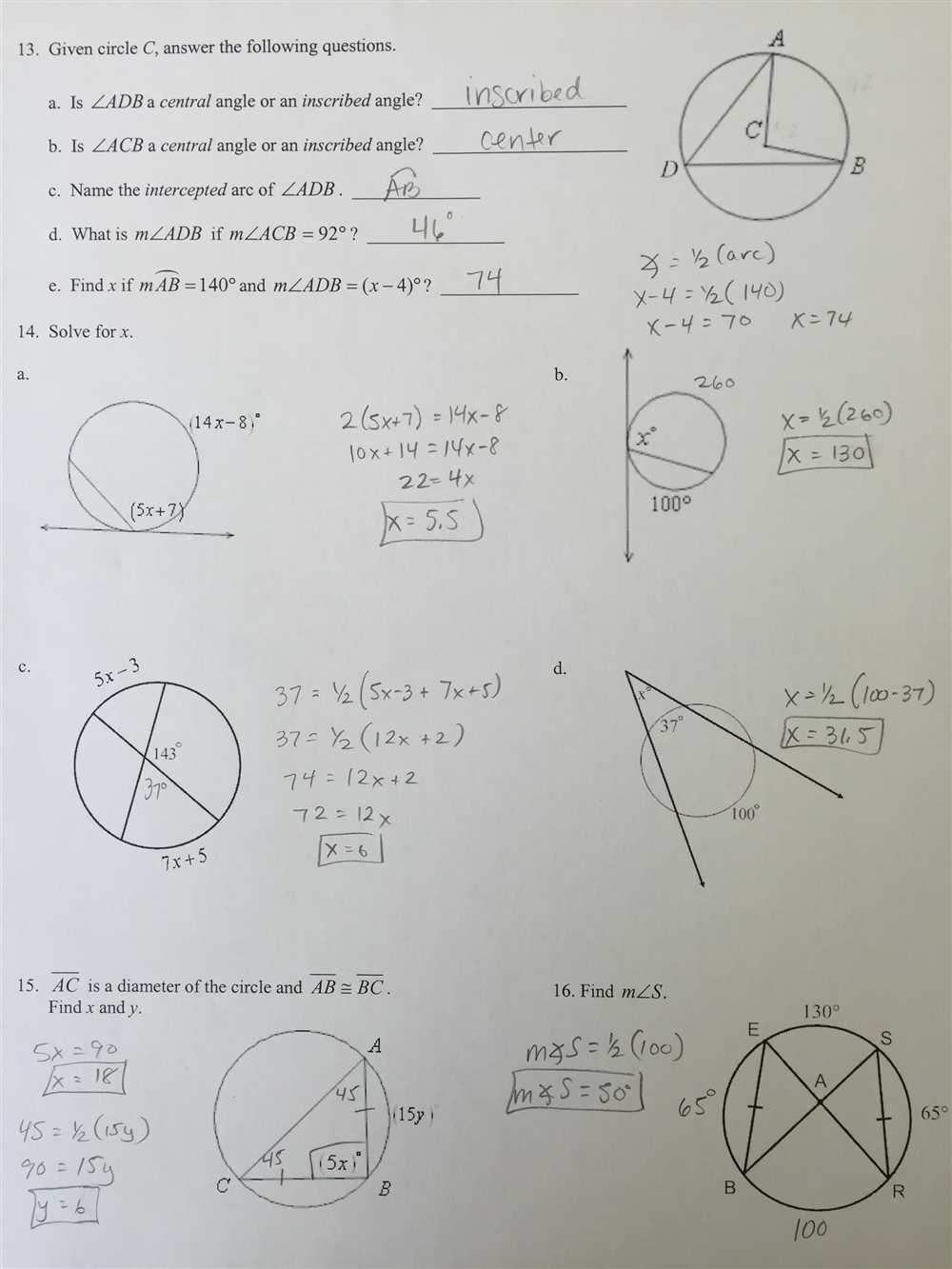
Understanding Circle Arcs and Angles: Central, Inscribed, and Intercepted
Introduction
Circle arcs and angles play an important role in geometry and are used to describe various properties and relationships within circles. In this article, we will explore three types of arcs and angles: central angles, inscribed angles, and intercepted arcs.
Central Angles
A central angle is an angle whose vertex is at the center of a circle and whose sides pass through any two points on the circumference of the circle. The measure of a central angle is equal to the measure of the arc it intercepts. In other words, if we have a central angle of 60 degrees, the corresponding arc it intercepts will also have a measure of 60 degrees. Central angles are named based on the endpoints of the arc they intercept.
Inscribed Angles
An inscribed angle is an angle whose vertex is on the circumference of a circle and whose sides pass through two other points on the circumference. The measure of an inscribed angle is half the measure of the arc it intercepts. For example, if we have an inscribed angle of 30 degrees, the corresponding arc it intercepts will have a measure of 60 degrees. Inscribed angles are also named based on the endpoints of the arc they intercept.
Intercepted Arcs
An intercepted arc is the portion of the circumference of a circle that is enclosed by an inscribed angle or a central angle. The measure of an intercepted arc is equal to the measure of the angle that intercepts it. For example, if we have an intercepted arc with a measure of 120 degrees, the corresponding angle that intercepts it will also have a measure of 120 degrees. Intercepted arcs are named based on the endpoints of the angle that intercepts them.
In conclusion, understanding circle arcs and angles, including central angles, inscribed angles, and intercepted arcs, is crucial for solving problems involving circles in geometry. These concepts help us describe and analyze the relationships between angles and arcs within a circle, providing a foundation for further exploration in this area of mathematics.
Solving Problems with Tangents and Secants: Tangent-Secant and Secant-Secant Intersections
When working with circles, it is often necessary to determine the points where tangents or secants intersect. These intersections can provide important information about the angles and lengths within the circle. Solving problems involving tangent-secant and secant-secant intersections requires an understanding of the properties of these lines and how they relate to the circle.
When a tangent and a secant intersect, it is important to remember that the point of intersection lies on the circle. This means that the distance from the center of the circle to the point of intersection is equal to the radius of the circle. This property can be used to solve problems involving the lengths of segments or the measurement of angles.
When dealing with secant-secant intersections, it is important to remember that the product of the lengths of the segments of one secant is equal to the product of the lengths of the segments of the other secant. This property, known as the power of a point theorem, can be used to solve problems involving the lengths of segments within the circle.
In addition to these properties, it is also important to understand how to use the relationships between angles formed by tangents and secants. These relationships can be used to solve problems involving the measurement of angles within the circle or the angles formed between tangents and secants.
By applying these properties and relationships, it is possible to solve a wide range of problems involving tangent-secant and secant-secant intersections. Whether it is finding the length of a segment, determining the measure of an angle, or identifying the point of intersection, understanding these concepts is essential for success in solving these types of problems.
Applying Circle Theorems: Inscribed Angles, Inscribed Polygons, and Chords

The study of circle theorems involves the examination of various relationships and properties within circles. One important concept is that of inscribed angles. An inscribed angle is an angle formed by two chords of a circle that have a common endpoint. The measure of an inscribed angle is half the measure of its intercepted arc. This theorem, known as the inscribed angle theorem, is crucial in solving problems involving circles.
Another key aspect of circle theorems is the relationship between inscribed polygons and the circles containing them. An inscribed polygon is a polygon whose vertices all lie on a circle. One important property of inscribed polygons is that the measures of their interior angles are determined by the number of sides. For example, in a regular hexagon inscribed in a circle, each interior angle measures 120 degrees. Understanding these relationships allows mathematicians to calculate various angles and lengths within inscribed polygons.
Chords are another fundamental element of circle theorems. A chord is a line segment that connects two points on a circle. One important theorem involving chords is the chord-chord angle theorem, which states that the measure of the angle formed by two intersecting chords is half the sum of the measures of the arcs intercepted by the angle. This theorem is used to solve problems involving intersecting chords, such as finding the measure of an angle formed by two intersecting chords.
- The inscribed angle theorem
- The relationship between inscribed polygons and circles
- The chord-chord angle theorem
Overall, understanding and applying these circle theorems allow mathematicians to solve complex problems involving angles, arcs, and lengths within circles and inscribed polygons. These theorems provide valuable tools for analyzing and dissecting geometric shapes and can be applied in a wide range of mathematical and real-world contexts.
Solving Real-world Problems with Circles: Examples and Step-by-step Solutions
Circles are a fundamental shape in geometry and can be found in various real-world situations. From calculating the area of a circular garden to determining the circumference of a round object, understanding how to solve problems involving circles is an essential skill.
One real-world problem that can be solved using circle principles is measuring the amount of fencing required for a circular dog run. Let’s say we have a circular dog run with a radius of 10 feet. To determine the amount of fencing needed, we can use the formula for the circumference of a circle, which is C = 2πr. Plugging in the given radius, we get C = 2π(10) = 20π feet of fencing needed. It’s important to note that if a decimal approximation is required, we can use the approximation of π as 3.14.
Another real-world problem involving circles is finding the length of a circular race track. For example, let’s say we have a circular race track with a radius of 50 meters. To find the length of the track, we can again use the formula for the circumference of a circle. Plugging in the given radius, we get C = 2π(50) = 100π meters. Using the approximation of π as 3.14, we can calculate that the length of the track is approximately 314 meters.
Solving real-world problems with circles involves understanding the formulas and principles related to circles and applying them in a step-by-step manner. By practicing these skills, we can become proficient in solving various problems involving circles and enhance our overall mathematical problem-solving abilities.
Chapter 10 Circles Answer Key: Recap and Review Questions
In Chapter 10 of our mathematics textbook, we explored the concept of circles and learned various properties related to them. As a review, let’s summarize some key points and go through a set of questions to solidify our understanding.
A circle is a perfectly round shape consisting of all points in a plane that are equidistant from a fixed center point. The distance from the center of the circle to any point on its circumference is called the radius, denoted by the letter “r.” The distance across the circle through its center is called the diameter, denoted by the letter “d.” The diameter is always twice the length of the radius.
Recap Questions:
- What is a circle?
- What is the definition of radius?
- What is the definition of diameter?
The circumference of a circle is the perimeter or distance around it. The formula to find the circumference is C = 2πr, where π (pi) is a mathematical constant approximately equal to 3.14159. The area of a circle is the amount of space enclosed by its circumference. The formula to find the area is A = πr^2.
In addition to these formulas, we also learned about central angles, arc length, and sector area in circles. A central angle is an angle whose vertex is at the center of the circle, and its rays extend to any point on the circumference. The measure of a central angle is equal to the arc length it intercepts divided by the radius of the circle.
Review Questions:
- What is the formula to find the circumference of a circle?
- What is the formula to find the area of a circle?
- What is a central angle?
- How do you find the measure of a central angle?
By understanding and applying these concepts, we can solve various problems involving circles, such as finding the length of an arc, the area of a sector, or the distance between two points on a circle’s circumference. It is important to practice these skills to build a strong foundation in geometry and be able to solve more complex problems in the future.