
Geometry can be a challenging subject, with its intricate shapes, angles, and formulas. However, with enough practice and preparation, you can master the concepts and excel in your geometry class. One effective way to gauge your understanding and identify areas for improvement is by taking practice tests.
A Chapter 7 practice test in geometry is specifically designed to assess your knowledge and skills in the topics covered in Chapter 7 of your geometry textbook. This chapter often focuses on concepts such as triangles, quadrilaterals, and the properties of polygons. By engaging with a practice test, you can familiarize yourself with the types of questions you may encounter on an actual exam and build confidence in your ability to tackle them.
Practicing with a Chapter 7 practice test allows you to assess your current level of understanding and identify any gaps in your knowledge. By analyzing the questions you answer incorrectly, you can pinpoint the specific areas where you need to focus your studying. This targeted approach enables you to make the most efficient use of your study time and ensure that you are well-prepared for any upcoming geometry assessments or exams.
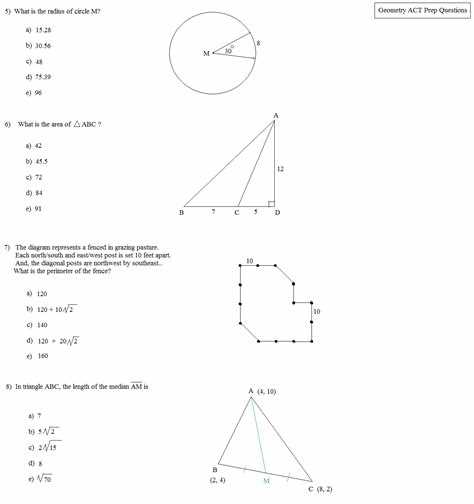
Chapter 7 Practice Test Geometry
In Chapter 7 of Geometry, students learn about various concepts related to triangles. To assess their understanding of these concepts, a practice test is often given. The Chapter 7 Practice Test in Geometry includes questions that cover topics such as triangle congruence, triangle similarity, the Pythagorean Theorem, and the special right triangles.
The practice test is designed to help students review and reinforce what they have learned in Chapter 7. It allows them to apply the principles and formulas they have learned to solve different types of problems related to triangles. By attempting this practice test, students can identify areas where they may need additional practice or clarification before the actual test.
The test consists of multiple-choice questions, as well as problems that require students to show their work and provide explanations. This format helps in evaluating not only the correctness of the answers but also the understanding and reasoning behind them. Students are encouraged to show their work and provide clear explanations to receive credit for their responses.
Overall, the Chapter 7 Practice Test in Geometry serves as a valuable tool for students to assess their knowledge and understanding of the concepts related to triangles. It helps them prepare for the actual test and identify areas where they may need additional support. By reviewing their performance on the practice test, students can focus their study efforts on the topics that require more attention, ensuring a better grasp of the material.
Solving Triangle Problems
When it comes to solving triangle problems in geometry, there are several key concepts and formulas that can be applied. Understanding these concepts and knowing how to use the appropriate formulas can help you solve various triangle problems efficiently.
One of the basic concepts in triangle problems is the Pythagorean theorem, which states that in a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides. This theorem can be used to find missing side lengths or to determine if a triangle is a right triangle.
To solve for missing angles in a triangle, you can use the trigonometric ratios sine, cosine, and tangent. These ratios relate the angles of a right triangle to the ratios of the lengths of its sides. By knowing the lengths of two sides in a triangle, you can use these ratios to find the measures of the angles.
Another important concept in solving triangle problems is the Law of Sines and the Law of Cosines. The Law of Sines states that the ratio of the length of a side to the sine of its opposite angle is constant for all sides and angles in a triangle. The Law of Cosines, on the other hand, relates the lengths of the sides of a triangle to the cosine of one of its angles.
In addition to these concepts, there are also various properties and theorems that can be applied to solve triangle problems. These include the Angle-Side-Angle (ASA), Side-Angle-Side (SAS), and Side-Side-Side (SSS) congruence criteria, as well as the Triangle Inequality Theorem.
By understanding these concepts and formulas and applying them appropriately, you can successfully solve a wide range of triangle problems in geometry.
Calculating Interior and Exterior Angles
Understanding how to calculate interior and exterior angles is crucial in geometry. Interior angles are the angles that are formed inside a polygon, while exterior angles are the angles that are formed outside the polygon.
One way to calculate the measure of interior angles in a regular polygon is to use the formula (n – 2) * 180° / n, where n represents the number of sides in the polygon. For example, if we have a regular pentagon with 5 sides, we can substitute n = 5 into the formula to find that each interior angle measures (5 – 2) * 180° / 5 = 108°.
To calculate the measure of exterior angles in a regular polygon, we can use the formula 360° / n, where n represents the number of sides in the polygon. Using the example of a regular pentagon again, we can substitute n = 5 into the formula to find that each exterior angle measures 360° / 5 = 72°.
It is important to note that the sum of the measures of the interior angles of a polygon always adds up to (n – 2) * 180°, while the sum of the measures of the exterior angles always adds up to 360°. These formulas and concepts are fundamental in geometry and allow us to solve various problems related to angles in polygons.
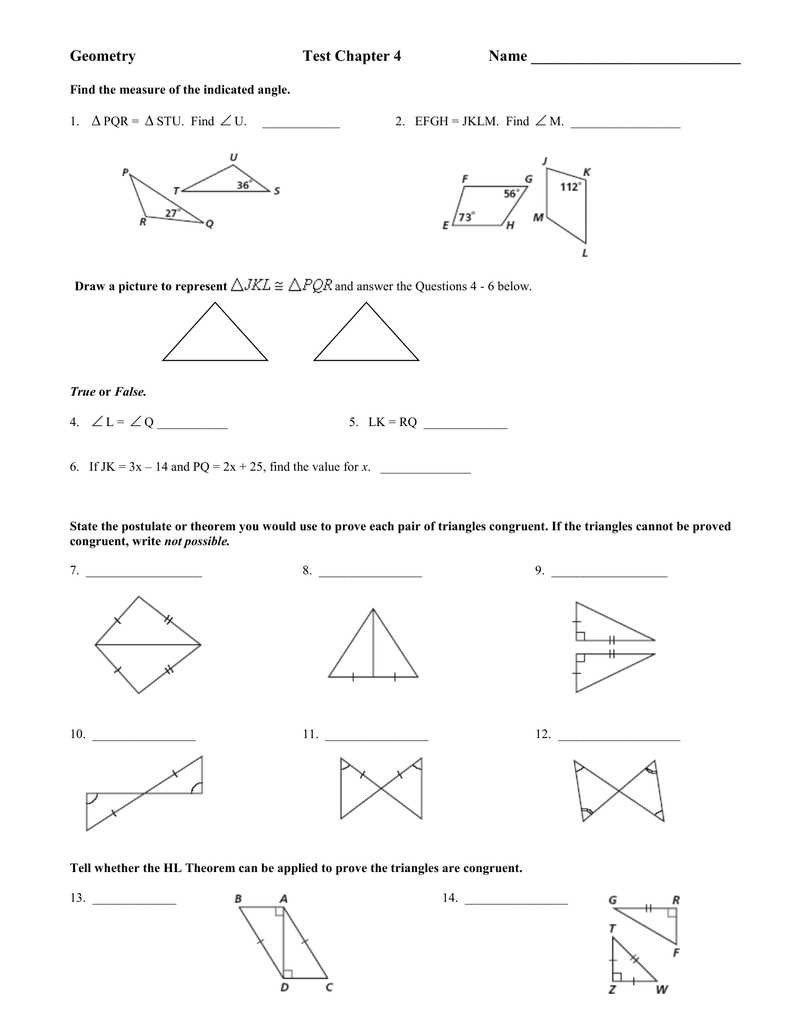
Determining Congruent Triangles
In geometry, congruent triangles are triangles that have the same shape and size. To determine whether two triangles are congruent, several criteria can be used, including side-side-side (SSS), side-angle-side (SAS), angle-side-angle (ASA), angle-angle-side (AAS), and hypotenuse-leg (HL).
The side-side-side (SSS) criterion states that if all three sides of one triangle are congruent to the corresponding sides of another triangle, then the triangles are congruent. This means that all three pairs of corresponding sides must have the same length.
The side-angle-side (SAS) criterion states that if two sides and the included angle of one triangle are congruent to the corresponding parts of another triangle, then the triangles are congruent. This means that two pairs of corresponding sides must have the same length, and the included angles must have the same measure.
The angle-side-angle (ASA) criterion states that if two angles and the included side of one triangle are congruent to the corresponding parts of another triangle, then the triangles are congruent. This means that two pairs of corresponding angles must have the same measure, and the included side must have the same length.
The angle-angle-side (AAS) criterion states that if two angles and a non-included side of one triangle are congruent to the corresponding parts of another triangle, then the triangles are congruent. This means that two pairs of corresponding angles must have the same measure, and a pair of corresponding sides must have the same length.
The hypotenuse-leg (HL) criterion is specifically used for right triangles. It states that if the hypotenuse and one leg of one right triangle are congruent to the hypotenuse and one leg of another right triangle, then the triangles are congruent.
By applying these criteria, it is possible to determine whether two triangles are congruent and establish corresponding congruent parts.
Applying the Pythagorean Theorem
The Pythagorean Theorem is a fundamental concept in geometry that allows us to find the length of one side of a right triangle when we know the lengths of the other two sides. The theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
To apply the Pythagorean Theorem, we first identify the right triangle and label the sides. The side opposite the right angle is the hypotenuse, which is typically labeled as ‘c’. The other two sides are called the legs, which are labeled as ‘a’ and ‘b’.
Once we have identified the sides of the triangle, we can use the Pythagorean Theorem to find the length of the unknown side. We square the lengths of the legs, add them together, and then take the square root of the sum to find the length of the hypotenuse or one of the legs.
For example, let’s say we have a right triangle with a leg of length 3 and a hypotenuse of length 5. We can use the Pythagorean Theorem to find the length of the other leg. The square of the length of the hypotenuse is 25, and the square of the length of the known leg is 9. If we subtract 9 from 25, we get 16. Taking the square root of 16 gives us 4, so the length of the other leg is 4.
Using Similarity to Solve Problems
The concept of similarity in geometry is a powerful tool that can be used to solve a variety of problems. Similar figures have the same shape but may have different sizes. By understanding the properties of similar figures, we can use ratios and proportions to find missing lengths, areas, and volumes.
One application of similarity is in enlargements and reductions. If two figures are similar, we can use the ratio of their corresponding sides to determine the scale factor of the enlargement or reduction. For example, if we have a triangle with sides of length 3, 4, and 5, and we want to enlarge it by a scale factor of 2, we can simply multiply each side by 2 to get a new triangle with sides of length 6, 8, and 10.
Similarity can also be used to find missing measurements in geometric figures. For example, if we have two triangles that are similar, and we know the lengths of some of the sides, we can set up a proportion and solve for the unknown side length. By using the properties of similar triangles, we can also find missing angles and determine if two figures are similar or not.
In summary, similarity is a useful concept in geometry that allows us to solve a variety of problems. By understanding the properties of similar figures, we can use ratios and proportions to find missing measurements, determine scale factors, and determine if two figures are similar. Using similarity as a problem-solving tool can help us explore and understand the relationships between different geometric figures.
Measuring Area and Volume
When it comes to geometry, measuring the area and volume of different shapes and objects is an important skill to have. By calculating these measurements, we can better understand the spatial relationships between different objects and make informed decisions about design and construction.
To measure area, we need to find the amount of space enclosed by a two-dimensional shape. This can be done by multiplying the length of one side by the length of the adjacent side. For example, the area of a rectangle can be found by multiplying its length and width. Similarly, the area of a triangle can be found by multiplying its base and height and dividing the result by 2. By using these formulas, we can accurately measure the area of various polygons and irregular shapes.
To measure volume, we need to find the amount of space enclosed by a three-dimensional object. This can be done by multiplying the area of the base by the height of the object. For example, the volume of a rectangular prism can be found by multiplying the area of its base (length times width) by its height. Similarly, the volume of a cylinder can be found by multiplying the area of its base (π times the square of the radius) by its height. By using these formulas, we can accurately measure the volume of various solids and containers.
Key phrases:
- Measuring area and volume
- Spatial relationships
- Two-dimensional shape
- Rectangular prism
- Cylinder
Identifying and Classifying Polygons
Polygons are shapes that are made up of straight lines and enclosed by a series of interconnected sides. They are two-dimensional and have different characteristics that can help us identify and classify them.
When identifying polygons, it is important to look at their properties such as the number of sides and angles. Let’s start with some common polygons:
- Triangle: A polygon with three sides and three angles.
- Quadrilateral: A polygon with four sides and four angles.
- Pentagon: A polygon with five sides and five angles.
- Hexagon: A polygon with six sides and six angles.
- Heptagon: A polygon with seven sides and seven angles.
- Octagon: A polygon with eight sides and eight angles.
- Nonagon: A polygon with nine sides and nine angles.
- Decagon: A polygon with ten sides and ten angles.
Once we have identified the number of sides and angles of a polygon, we can classify it further based on its properties. Here are some classifications:
- Regular Polygon: A polygon with all sides and angles that are equal.
- Irregular Polygon: A polygon with sides and angles that are not equal.
- Convex Polygon: A polygon with all interior angles less than 180 degrees, where no side extends beyond the figure.
- Concave Polygon: A polygon with at least one interior angle greater than 180 degrees, where at least one side extends beyond the figure.
By understanding the properties and characteristics of polygons, we can effectively identify and classify them, which is crucial in geometry and other mathematical applications.
Understanding Circles and their Properties
Circles are one of the most fundamental shapes in geometry and have a variety of unique properties. Understanding these properties is crucial in solving problems and working with circles in different contexts.
Radius: The radius of a circle is the distance from the center of the circle to any point on its circumference. It is denoted by the symbol ‘r’.
Diameter: The diameter of a circle is twice the length of its radius. It is the longest chord that can be drawn in a circle and passes through the center. The diameter is denoted by the symbol ‘d’.
Circumference: The circumference of a circle is the distance around its outer boundary. It can be calculated using the formula C = 2πr, where ‘C’ represents the circumference and ‘π’ represents the mathematical constant pi (approximately 3.14).
Area: The area of a circle is the measure of the region enclosed by its circumference. It can be calculated using the formula A = πr^2, where ‘A’ represents the area.
Tangent: A tangent to a circle is a line that intersects the circle at exactly one point. The point of intersection is called the point of tangency. A line tangent to a circle is perpendicular to the radius at the point of tangency.
Chord: A chord is a line segment that connects two points on the circumference of a circle. It may or may not pass through the center of the circle.
Understanding these basic properties of circles is essential for solving problems involving circles in geometry. Whether calculating the radius, diameter, circumference, or area, or analyzing tangents and chords, a solid understanding of circle properties is crucial in mastering this geometric shape.