
In physics, collisions between objects can be described and analyzed using the principles of conservation of momentum. This concept states that the total momentum of a closed system remains constant before and after a collision, assuming no external forces are acting on the system. Momentum is a vector quantity, calculated by multiplying an object’s mass and velocity, and its conservation allows us to predict the motion of objects involved in collisions.
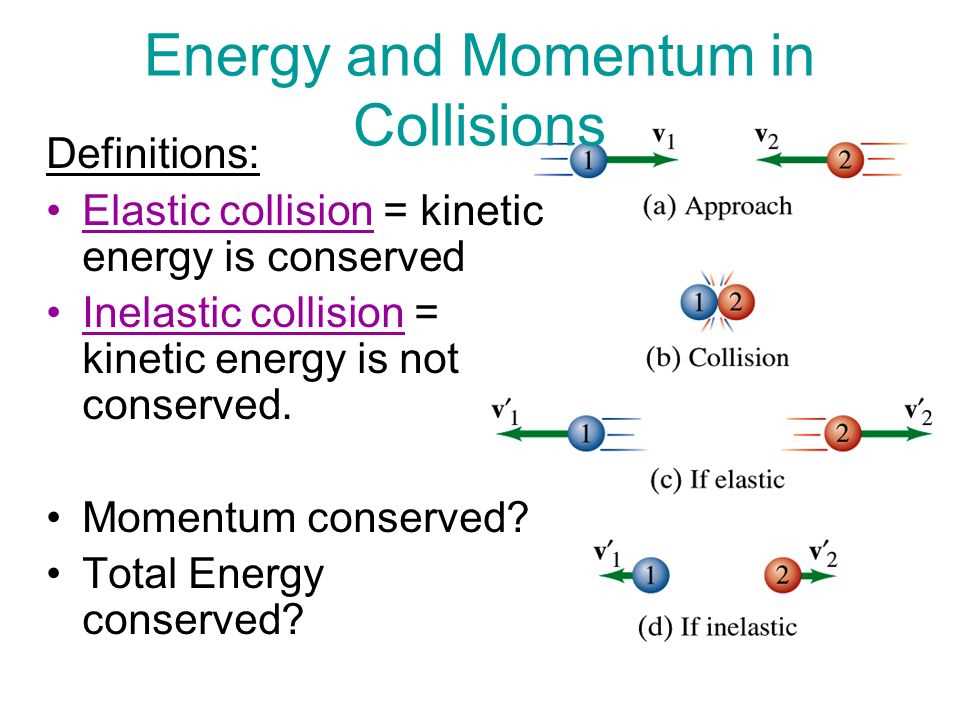
Collisions can be classified into two types: elastic and inelastic. In an elastic collision, kinetic energy and momentum are conserved, meaning that the total kinetic energy before the collision is equal to the total kinetic energy after the collision. In an inelastic collision, only momentum is conserved, and some kinetic energy is transformed into other forms of energy, such as heat or sound.
To solve collisions problems, we can use the conservation of momentum equation, which states that the initial momentum of the system is equal to the final momentum of the system. By setting up equations and solving for unknowns, we can determine the velocities or masses of objects involved in the collision.
Example:
Consider a collision between two objects, object A and object B. Object A has a mass of 2 kg and an initial velocity of 4 m/s, while object B has a mass of 3 kg and an initial velocity of -2 m/s. If the collision is elastic, what are the final velocities of both objects?
To solve this problem, we can set up the conservation of momentum equation:
(mass A * initial velocity A) + (mass B * initial velocity B) = (mass A * final velocity A) + (mass B * final velocity B)
Plugging in the values, we get:
(2 kg * 4 m/s) + (3 kg * -2 m/s) = (2 kg * final velocity A) + (3 kg * final velocity B)
Simplifying the equation, we obtain:
8 kg m/s – 6 kg m/s = 2 kg * final velocity A + 3 kg * final velocity B
By rearranging terms and solving for the final velocities, we find that the final velocity of object A is 0.33 m/s and the final velocity of object B is -0.33 m/s. Therefore, the objects move in opposite directions after the collision.
Overview of Collisions and Conservation of Momentum
Collisions occur when two objects come into contact and interact with each other. The study of collisions involves analyzing the momentum, which is the product of an object’s mass and velocity. The conservation of momentum principle states that in a closed system, the total momentum before a collision is equal to the total momentum after the collision.
There are two types of collisions: elastic and inelastic. In an elastic collision, both the momentum and kinetic energy are conserved. This means that when two objects collide, they bounce off each other without any loss of energy. On the other hand, in an inelastic collision, the kinetic energy is not conserved. Some of the energy is transformed into other forms, such as heat or sound.
When analyzing collisions, it is important to consider different factors, such as the masses and velocities of the objects involved. The momentum of an object can be calculated using the formula p = m * v, where p is the momentum, m is the mass, and v is the velocity. Using this formula, the momentum before and after a collision can be determined.
Collisions and the conservation of momentum have practical applications in various fields, such as physics, engineering, and sports. Understanding how momentum is conserved allows for the design of safer cars, the improvement of sports equipment, and the understanding of particle interactions in particle accelerators. By studying collisions and momentum, scientists and engineers are able to analyze and predict the outcome of different scenarios involving moving objects.
Importance of Worksheet Answers in Learning
Worksheet answers play a crucial role in the learning process as they provide students with a way to check their understanding and progress. They serve as a valuable tool for self-assessment and help students identify areas where they may need additional practice or clarification. By comparing their answers to the correct solutions, students can gain insights into their strengths and weaknesses, enabling them to focus on areas that require improvement.
Worksheet answers also foster independent learning and problem-solving skills. When students attempt to solve problems on their own before referring to the answers, they develop critical thinking skills and learn to approach questions from different angles. This process of trial and error enhances their problem-solving abilities, allowing them to tackle more complex tasks in the future.
Moreover, worksheet answers provide immediate feedback to students, allowing them to address misunderstandings or misconceptions right away. They prevent misconceptions from solidifying and help students correct their mistakes before they become ingrained. Timely feedback is essential for effective learning, as it ensures that students stay on track and can make necessary adjustments in their understanding.
Worksheet answers also serve as a reference guide for students, particularly when reviewing for exams or revisiting previous topics. Instead of relying solely on their memory, students can refer to the answers for guidance and remind themselves of the correct approach or solution. This helps reinforce learning and deepen understanding, as students can revisit concepts with greater confidence.
In summary, worksheet answers are key to enhancing the learning experience for students. They encourage self-assessment, foster problem-solving skills, provide immediate feedback, and serve as a valuable reference guide. By utilizing worksheet answers effectively, students can optimize their learning and achieve better academic outcomes.
Understanding Momentum and Impulse
Momentum and impulse are important concepts in physics that help us understand the behavior of objects in motion. Momentum refers to the quantity of motion an object possesses, while impulse is the change in momentum of an object. Both momentum and impulse are vector quantities, meaning they have both magnitude and direction.
When two objects collide, the total momentum of the system is conserved. This is known as the law of conservation of momentum. The momentum before the collision is equal to the momentum after the collision. This principle can be observed in various real-world scenarios, such as car crashes or billiard balls colliding on a pool table.
The equation for momentum is given by the product of an object’s mass and its velocity. Mathematically, momentum = mass × velocity. Impulse, on the other hand, is defined as the product of the force applied to an object and the time over which the force is applied. This can be represented by the equation impulse = force × time.
To solve problems involving momentum and impulse, it is important to carefully consider the direction of motion and the forces acting on the objects. It is also helpful to understand the principle of conservation of momentum and how it applies to collisions. By analyzing the changes in momentum and applying the relevant equations, we can calculate the velocities and forces involved in a collision.
In conclusion, momentum and impulse are fundamental concepts in physics that help us understand the behavior of objects in motion. They play a crucial role in analyzing collisions and determining the resulting changes in velocity and force. By studying these concepts and applying the relevant equations, we can gain a deeper understanding of the physical world around us.
Definition and Calculation of Momentum
Momentum is a fundamental concept in physics that describes the motion of objects. It is defined as the product of an object’s mass and its velocity. In mathematical terms, momentum (p) can be calculated using the equation:
p = m * v
Where m represents the mass of the object and v represents its velocity. The unit of momentum is kilogram meter per second (kg*m/s).
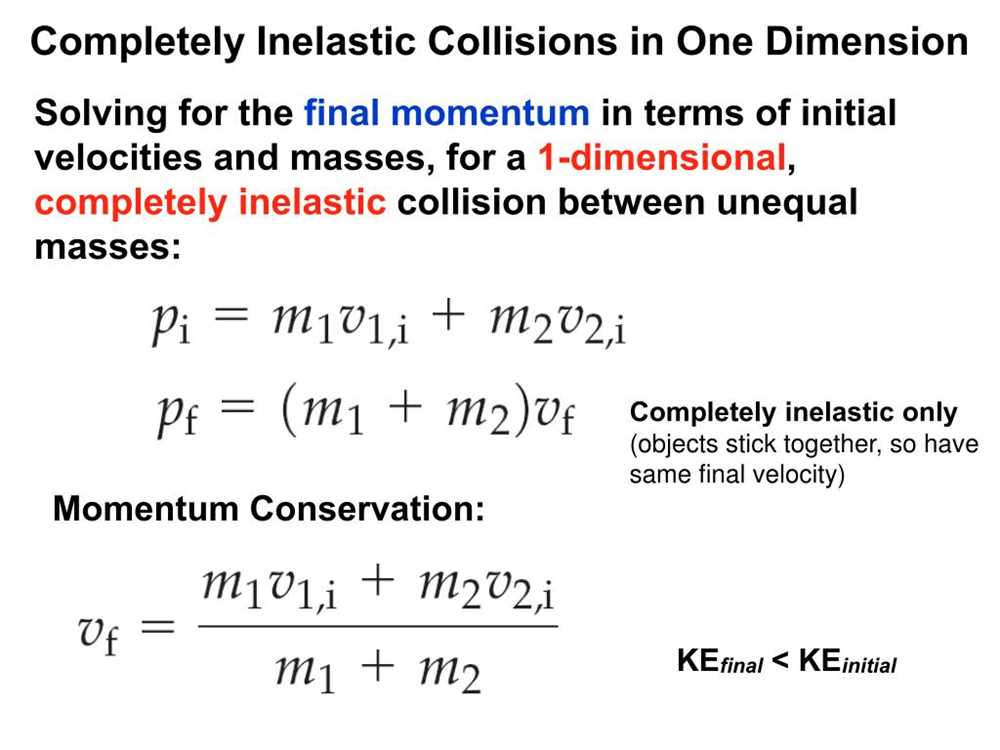
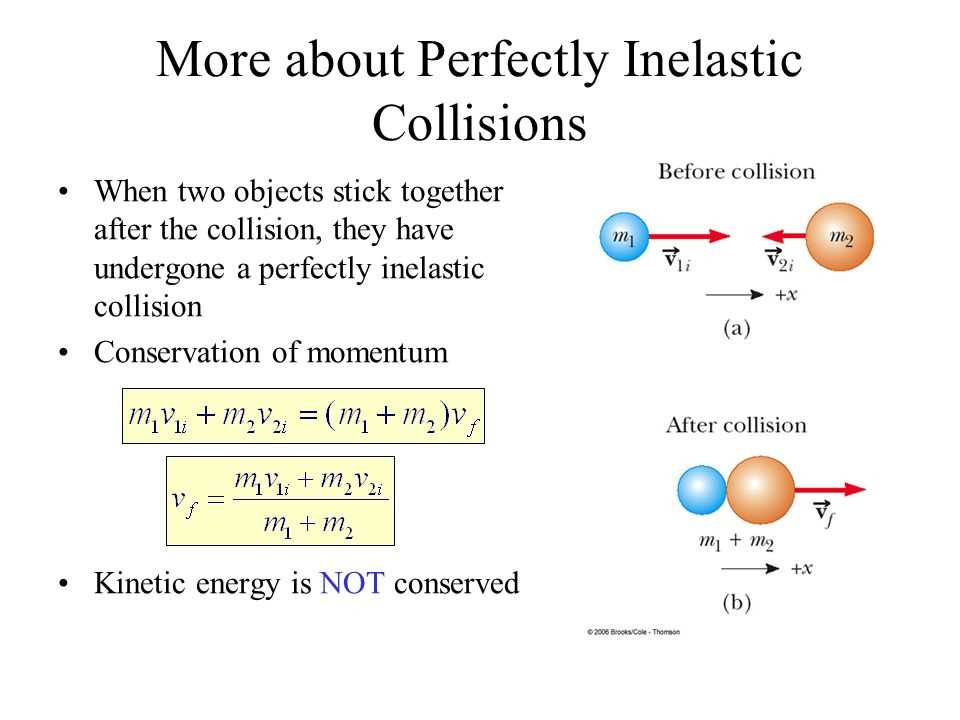
When two objects collide, the total momentum before the collision is equal to the total momentum after the collision. This principle is known as the conservation of momentum. It means that the momentum is conserved in a closed system, where no external forces act on the objects. By applying the principle of conservation of momentum, we can calculate the final velocities of the objects after collision.
There are two types of collisions: elastic and inelastic. In an elastic collision, kinetic energy is conserved, and the objects bounce off each other without any loss in energy. In an inelastic collision, kinetic energy is not conserved, and the objects may stick together or deform upon collision.
To calculate the final velocities after a collision, we can use the principle of conservation of momentum by setting up equations based on the initial momentum and the final momentum of the objects involved. By solving these equations, we can determine the final velocities of the objects.
Impulse and its Relationship to Momentum
Momentum is a fundamental concept in physics that quantifies the motion of an object. It is defined as the product of an object’s mass and its velocity. Momentum is a vector quantity, meaning it has both magnitude and direction.
When a force is applied to an object for a certain amount of time, it results in a change in momentum. This change in momentum is known as impulse. Impulse is defined as the product of the force applied to an object and the duration of time over which the force is applied. Mathematically, impulse can be calculated using the equation:
Impulse = Force × Time
This equation shows that impulse is directly proportional to force and the duration of time for which the force is applied. It also highlights the fact that impulse is a vector quantity, as force and time are both vector quantities. The unit for impulse is newton-second (Ns) or kilogram-meter per second (kg·m/s).
Impulse is closely related to the concept of momentum. According to Newton’s second law of motion, the rate of change of an object’s momentum is equal to the force applied to it. This can be expressed mathematically as:
Impulse = Change in Momentum
This equation demonstrates that impulse and the change in momentum are equal in magnitude and direction. This relationship is important in understanding the effects of interactions between objects in a collision or an explosive event. In these situations, the impulse experienced by an object before and after the interaction can be used to determine the resulting change in momentum.
The conservation of momentum principle states that the total momentum of a system remains constant if no external forces act on it. This principle applies to isolated systems, where the total momentum before an interaction is equal to the total momentum after the interaction. By understanding the relationship between impulse and momentum, we can better analyze and predict the outcomes of various collisions and explosive events.
Types of Collisions
In the study of collisions, there are two main types: elastic collisions and inelastic collisions. In an elastic collision, the total kinetic energy of the system is conserved. This means that the objects involved in the collision bounce off each other and continue moving with the same total energy as before the collision. In an inelastic collision, the total kinetic energy of the system is not conserved. Some of the energy is lost to other forms, such as heat or sound.
Elastic Collisions: In an elastic collision, the objects involved do not experience any deformation or permanent damage. The total momentum and total kinetic energy of the system are conserved. This means that the sum of the masses and velocities of the objects before the collision is equal to the sum after the collision. Elastic collisions are commonly observed in scenarios such as billiards or pool games, where the balls bounce off each other without any loss of energy.
Inelastic Collisions: In an inelastic collision, the objects involved experience deformation or stick together. The total momentum of the system is conserved, but the total kinetic energy is not. Some of the kinetic energy is transferred to other forms, such as heat or sound. Inelastic collisions are commonly observed in scenarios such as car accidents, where the vehicles come to a stop after the collision and some of the kinetic energy is dissipated as heat or sound.
It is important to understand the different types of collisions and how momentum and energy are conserved in each case. This knowledge allows scientists and engineers to analyze and predict the outcomes of collisions, which is crucial in various fields such as physics, engineering, and accident reconstruction.
Elastic Collisions
Elastic collisions are a type of collision where kinetic energy is conserved. In an elastic collision, the total kinetic energy before the collision is equal to the total kinetic energy after the collision. This means that the objects involved in the collision bounce off each other without any loss of energy. Elastic collisions are characterized by objects rebounding with the same speed and direction as before the collision.
During an elastic collision, both momentum and kinetic energy are conserved. The conservation of momentum states that the total momentum of the system before the collision is equal to the total momentum after the collision. This means that the total mass of the objects multiplied by their velocities before the collision is equal to the total mass of the objects multiplied by their velocities after the collision.
In elastic collisions, the objects involved do not undergo any deformation or permanent damage. This is because the forces exerted during the collision are repulsive forces that push the objects away from each other. The collision occurs over a very short period of time, causing an exchange of momentum, but without any loss of kinetic energy.
Example:
A ball of mass 0.5 kg is moving towards another ball at rest with a mass of 1 kg. The initial velocity of the first ball is 5 m/s and the initial velocity of the second ball is 0 m/s. After the collision, the first ball rebounds with a velocity of 4 m/s. To find the final velocity of the second ball, we can use the conservation of momentum and kinetic energy equations.
Conservation of momentum:
Initial momentum = final momentum
(0.5 kg)(5 m/s) + (1 kg)(0 m/s) = (0.5 kg)(4 m/s) + (1 kg)(vf)
2.5 kg·m/s = 2 kg·m/s + 1 kg·vf
0.5 kg·vf = 0.5 kg·m/s
vf = 1 m/s
Thus, the final velocity of the second ball is 1 m/s. This example demonstrates the principle of elastic collisions where the total momentum and kinetic energy are conserved.