
Conversion factors play a crucial role in scientific calculations, providing a way to convert between different units and measurements. In various scientific disciplines, such as chemistry and physics, accurate and precise calculations are essential for understanding and predicting phenomena. This lab report discusses the importance of conversion factors in solving problems and provides answers to a series of conversion-related questions.
When faced with a problem that involves different units, conversion factors allow scientists to bridge the gap between these units and ensure accurate calculations. By using conversion factors, it becomes possible to convert quantities from one unit to another, making it easier to compare and analyze data. This lab report explores how to identify and use conversion factors effectively, as well as how to apply them in various scientific scenarios.
Furthermore, this report presents detailed answers to a range of questions related to conversion factors. These questions challenge students to apply their understanding of conversion factors and solve complex problems. By examining the answers provided, readers can gain a deeper understanding of the principles behind conversion factors and how they are applied in practice.
In conclusion, conversion factors are essential tools in scientific calculations, enabling scientists to convert between different units and measurements. This lab report serves as a comprehensive guide to understanding and applying conversion factors, providing answers to a series of questions that test students’ knowledge in this area. By mastering the concepts discussed in this report, students will be better equipped to tackle a wide range of scientific problems that require the use of conversion factors.
Conversion Factors in Calculations Lab Report Answers
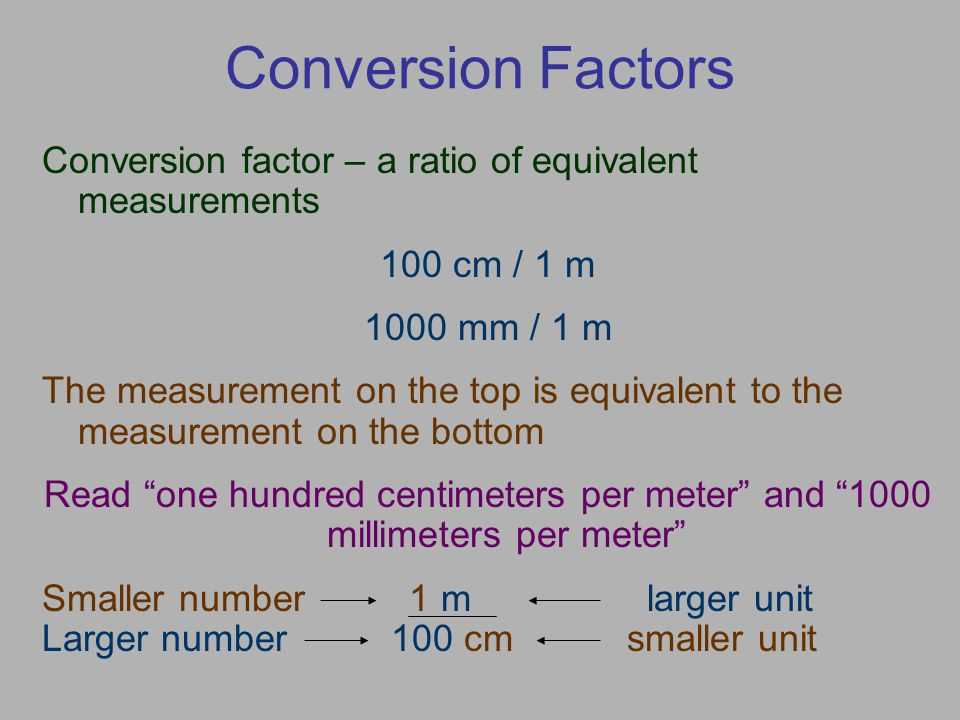
When conducting experiments or solving problems in the field of science, it is important to accurately convert between different units of measurement. Conversion factors play a crucial role in these calculations, as they provide a way to convert from one unit to another. In our lab report, we encountered various questions that required us to use conversion factors to find the desired answers.
One question in the lab report asked us to convert a measurement from inches to centimeters. To do this, we used the conversion factor of 2.54 centimeters per inch. By multiplying the measurement in inches by this conversion factor, we were able to easily convert it to centimeters.
Another question in the lab report involved converting a mass from grams to kilograms. We knew that there are 1000 grams in a kilogram, so we used this conversion factor to find the answer. By dividing the mass in grams by 1000, we obtained the equivalent mass in kilograms.
- Conversion factor: A numerical ratio that relates one unit of measurement to another
- Inches to centimeters: Multiply inches by 2.54 to get centimeters
- Grams to kilograms: Divide grams by 1000 to get kilograms
It is essential to use the correct conversion factor and pay attention to units when solving calculation problems. A conversion factor acts as a multiplier or divisor to ensure that the units cancel out correctly and the final answer is in the desired unit. By understanding and effectively utilizing conversion factors, we can confidently solve problems and accurately report our findings in scientific experiments.
Definition of Conversion Factors
Conversion factors are mathematical expressions that are used to convert one unit of measurement to another. They provide a way to relate different units of the same physical quantity and are commonly used in scientific calculations and measurements. A conversion factor is a ratio of two different units, where the numerator represents the value in the original unit and the denominator represents the value in the desired unit.
In scientific calculations, conversion factors are essential in converting measurements from one unit to another. For example, when measuring distances, you may need to convert centimeters to meters or inches to feet. Conversion factors allow you to easily make these conversions by multiplying or dividing the measurement by the appropriate ratio.
Example: To convert 10 centimeters to meters, you would use the conversion factor 1 meter = 100 centimeters. By multiplying 10 centimeters by the conversion factor, you can determine that 10 centimeters is equal to 0.1 meters.
Conversion factors are derived from known relationships between units and are based on standardized values. They provide a standardized way to express measurements and ensure consistency and accuracy in scientific calculations. In addition to converting between different units within the same system (e.g., metric system), conversion factors can also be used to convert measurements between different systems (e.g., metric to imperial).
It is important to use conversion factors correctly and ensure that you have the correct ratio of units. The appropriate conversion factor will depend on the specific units being converted and the relationship between them. Understanding conversion factors and their applications is essential in performing accurate and reliable scientific calculations.
The Importance of Conversion Factors in Calculations
Conversion factors play a crucial role in calculations across various fields of science and engineering. They are essential tools that enable us to convert between different units of measurement, ensuring accurate and meaningful results. Without conversion factors, it would be virtually impossible to compare or combine measurements made in different systems or units.
One of the main reasons conversion factors are so important is the need for standardized units of measurement. In scientific research and engineering applications, it is essential to have a consistent and uniform system for expressing physical quantities. Conversion factors provide a bridge between different systems of units, allowing scientists and engineers to easily convert measurements from one system to another.
For example:
- A scientist conducting research in the United States may need to convert temperature measurements from Fahrenheit to Celsius to communicate their findings with researchers using the metric system.
- Engineers designing a bridge may need to convert measurements of distance and weight from imperial units to SI units to comply with international standards and codes.
Conversion factors are also critical in practical applications where accurate measurements and calculations are necessary for daily operations. Industries such as manufacturing, chemistry, and medicine rely on conversion factors to ensure product quality, safety, and efficiency. From determining dosage amounts for medications to calculating ingredient ratios in a recipe, conversion factors are an integral part of countless processes.
In conclusion, conversion factors are indispensable tools for scientists, engineers, and various industries. They allow for the seamless conversion of measurements between different systems and units, ensuring standardization and accuracy in calculations. Without conversion factors, the ability to compare, combine, and interpret measurements would be severely hindered, impacting both scientific research and everyday applications.
Commonly Used Conversion Factors
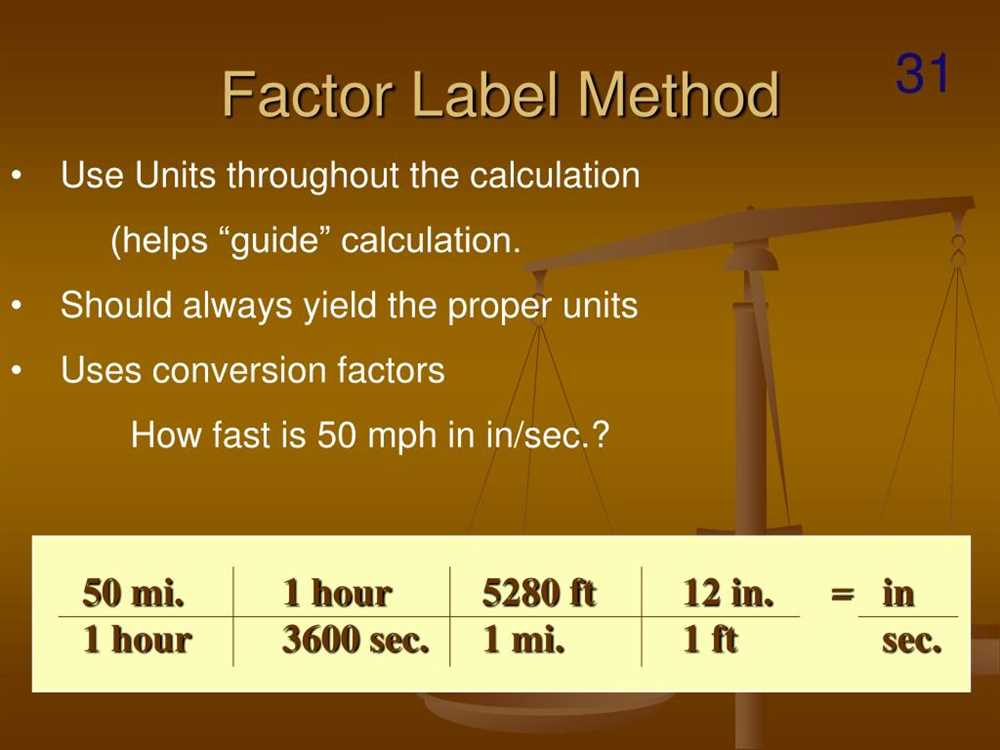
In scientific calculations and laboratory experiments, it is often necessary to convert units from one system to another. This is done using conversion factors, which are ratios that relate two different units of measurement. Understanding and using commonly used conversion factors is essential in accurately analyzing and interpreting experimental data.
Length:
- The conversion factor between centimeters (cm) and inches (in) is 2.54 cm = 1 in.
- To convert meters (m) to feet (ft), the conversion factor is 1 m = 3.281 ft.
- For kilometers (km) to miles (mi), the conversion factor is 1.609 km = 1 mi.
Mass:
- When converting grams (g) to ounces (oz), the conversion factor is 28.35 g = 1 oz.
- For kilograms (kg) to pounds (lb), the conversion factor is 2.205 kg = 1 lb.
Volume:
- The conversion factor between liters (L) and milliliters (mL) is 1 L = 1000 mL.
- To convert gallons (gal) to liters (L), the conversion factor is 1 gal = 3.785 L.
Temperature:
- To convert Celsius (°C) to Fahrenheit (°F), the conversion formula is °F = (°C × 9/5) + 32.
- For Kelvin (K) to Celsius (°C), the conversion formula is °C = K – 273.15.
These are just a few examples of commonly used conversion factors. It is important to always check the accuracy and reliability of the conversion factors being used, as slight variations and rounding errors can affect the results of scientific calculations. Additionally, it is crucial to understand the underlying principles and concepts behind the conversion factors in order to apply them correctly in different scenarios.
Conversion Factors in Metric System
The metric system is an internationally recognized decimal-based system of measurement that is widely used in science, industry, and everyday life. It provides a consistent and standardized way to express measurements in units that are easily converted from one to another. One of the key aspects of the metric system is the use of conversion factors, which allow for easy conversion between different units of measurement within the system.
A conversion factor is a ratio or fraction that expresses the relationship between two different units of measurement. It is often derived from the equivalence of two different measurements. For example, there are 1000 millimeters in one meter, so the conversion factor between millimeters and meters is 1/1000 or 0.001. To convert a measurement from millimeters to meters, you simply multiply the measurement by the conversion factor.
Conversion factors in the metric system can be applied to various types of measurements, including length, mass, volume, and temperature. For length, commonly used units in the metric system include millimeters, centimeters, meters, and kilometers. When converting between these units, the conversion factors are based on powers of 10. For example, to convert from centimeters to meters, you divide the measurement by 100, as there are 100 centimeters in one meter.
Conversion factors are also used in calculations involving mass, where the standard unit of measurement is the gram. For example, to convert grams to kilograms, the conversion factor is 0.001, as there are 1000 grams in one kilogram. Similarly, for volume, the standard unit is the liter, and the conversion factor between liters and milliliters is 1000. Finally, for temperature, the Celsius scale is commonly used in the metric system, and conversion factors are used to convert between Celsius and Kelvin.
In conclusion, conversion factors are an essential part of the metric system, allowing for easy and precise conversion between different units of measurement. They play a crucial role in scientific experiments, engineering calculations, and everyday applications, ensuring accuracy and consistency in measurements.
Conversion Factors in Imperial System
The Imperial system of measurement, commonly used in the United States, is based on a set of conversion factors that allow for the conversion between different units of measurement. These conversion factors are essential for accurate and efficient calculations in various fields, including science, engineering, and everyday life.
One of the most commonly used conversion factors in the Imperial system is the conversion between inches and feet. There are 12 inches in a foot, so the conversion factor is 12. This means that to convert inches to feet, you need to divide the number of inches by 12, and to convert feet to inches, you need to multiply the number of feet by 12.
Another important conversion factor in the Imperial system is the one between ounces and pounds. There are 16 ounces in a pound, so the conversion factor is 16. To convert ounces to pounds, you divide the number of ounces by 16, and to convert pounds to ounces, you multiply the number of pounds by 16.
Conversion factors are not limited to simple ratios like those mentioned above. They can also involve complex formulas or relationships between different units of measurement. For example, the conversion factor between Fahrenheit and Celsius temperature scales involves both addition and multiplication. It is calculated using the formula: °C = (°F – 32) * 5/9, where °C represents degrees Celsius and °F represents degrees Fahrenheit.
In conclusion, conversion factors play a crucial role in the Imperial system of measurement. They allow for easy and accurate conversion between different units of measurement, enabling efficient calculations and a better understanding of quantities in everyday life and various scientific fields.
Conversion Factors in Scientific Notation
The use of conversion factors is an essential part of scientific notation. Scientific notation is a way to express very large or very small numbers in a more concise and convenient form. It is especially useful in scientific calculations where precision is important. Conversion factors are used to convert between different units or quantities, allowing us to express the same value in different ways.
Conversion factors are ratios that relate two different units or quantities. They are often derived from known relationships or definitions. The conversion factor can be thought of as a mathematical tool that allows us to multiply or divide a given value to change its units. For example, to convert a value from meters to centimeters, we can use the conversion factor 1 meter = 100 centimeters. So, if we have a length of 2 meters, we can multiply it by the conversion factor to get 200 centimeters.
Conversion factors are particularly useful when dealing with scientific calculations that involve multiple units and measurements. They provide a way to convert between different systems of units, such as the metric and imperial systems, and allow scientists to easily compare and communicate their findings.
To effectively use conversion factors, it is important to be familiar with the units and their relationships. This includes understanding the prefixes used in scientific notation, such as kilo- (10^3), milli- (10^-3), and micro- (10^-6). It is also important to know the basic conversion factors for common units, such as length, time, mass, and volume. These conversion factors can be found in conversion tables or derived from known relationships.
Example:

Let’s say we want to convert a distance of 5 kilometers to meters. We can use the conversion factor 1 kilometer = 1000 meters. By multiplying 5 kilometers by the conversion factor, we get 5000 meters. Similarly, if we want to convert 100 milliliters to liters, we can use the conversion factor 1 liter = 1000 milliliters. By dividing 100 milliliters by the conversion factor, we get 0.1 liters.
In conclusion, conversion factors in scientific notation are essential tools for converting between different units or quantities. They allow scientists to express values in a more convenient form and facilitate accurate and precise calculations. Understanding the relationships between different units and being familiar with basic conversion factors is crucial for using conversion factors effectively in scientific calculations.