If you’re studying mathematics or have a keen interest in geometry, you’ve probably come across the concept of circles. Circles are a fundamental shape that can be found everywhere in our everyday lives, from the wheels on our cars to the plates we eat off. To fully understand circles, it is important to dive deeper into their properties, one of which is their equation. In this article, we will explore the equation of a circle worksheet answer key, providing you with a comprehensive guide to solving circle-related problems.
The equation of a circle might seem daunting at first, but it can be broken down into a few key components. In essence, the equation of a circle defines the relationship between the coordinates of a point on the circle and the circle’s center. This equation is typically written in the form (x – h)^2 + (y – k)^2 = r^2, where (h, k) represents the coordinates of the center of the circle, and r represents the radius.
Solving problems involving the equation of a circle often requires an understanding of basic algebraic techniques, such as expanding brackets and manipulating equations. The worksheet answer key provides step-by-step solutions to these problems, allowing you to practice and master these skills. By working through various exercises, you will gain confidence in your ability to solve circle-related problems and develop a deeper understanding of the mathematical principles behind circles.
Understanding the Formula for the Equation of a Circle
In mathematics, a circle is a two-dimensional geometric shape that consists of all the points in a plane that are equidistant from a fixed center point. The equation of a circle is a mathematical representation that describes the relationship between the coordinates of the points on the circle and its center.
The formula for the equation of a circle is (x – h)^2 + (y – k)^2 = r^2, where (h, k) represents the coordinates of the center of the circle, and r represents the radius. This formula allows us to graphically represent and analyze circles on a coordinate plane.
The equation can be understood by breaking down its components. The term (x – h)^2 represents the horizontal distance between any point (x, y) on the circle and the x-coordinate of the center of the circle, h. Similarly, the term (y – k)^2 represents the vertical distance between the point (x, y) and the y-coordinate of the center of the circle, k. Both terms squared together give us the total squared distance between the point and the center of the circle.
The sum of the squared distances, (x – h)^2 + (y – k)^2, should be equal to r^2, which represents the square of the radius. This means that the sum of the squared distances from any point on the circle to its center is always equal to the square of the radius.
How to derive the equation of a circle from given information
Deriving the equation of a circle from given information involves using the center and radius of the circle. By knowing the coordinates of the center and the length of the radius, we can create an equation that represents all the points on the circle.
To derive the equation, we can use the distance formula, which states that the distance between two points (x1, y1) and (x2, y2) is given by the formula:
d = √((x2-x1)^2 + (y2-y1)^2)
In the case of a circle, the distance between any point on the circle and the center is equal to the radius. So, if we have a point (x, y) on the circle and the center of the circle is (h, k), we can express the distance between the two points as:
sqrt((x-h)^2 + (y-k)^2) = r
By squaring both sides of the equation, we can eliminate the square root:
(x-h)^2 + (y-k)^2 = r^2
This equation represents all the points (x, y) that lie on the circle with center (h, k) and radius r. It is the equation of the circle in standard form.
In conclusion, by using the distance formula and the properties of a circle, we can derive the equation of a circle from given information such as the center and radius. The resulting equation represents all the points on the circle. It is a useful tool in analyzing and solving problems related to circles in mathematics and other fields.
Exploring the properties of the equation of a circle
One important property of the equation of a circle is that it provides information about the distance of any point on the circle from its center. By substituting specific values for x and y into the equation, we can determine if a point lies on the circle or not. If the point satisfies the equation, it lies on the circle, and if it does not satisfy the equation, it does not lie on the circle.
Another property of the equation of a circle is that it can be used to find the equation of a tangent line to the circle at a given point. By calculating the slope of the tangent line using the derivative of the equation, we can write an equation in the form y = mx + b, where m is the slope and b is the y-intercept.
The equation of a circle also allows us to determine important geometric features such as the diameter, circumference, and area of the circle. By knowing the radius, we can easily calculate these values using well-known formulas. For example, the diameter of a circle is twice the radius, the circumference is equal to 2π times the radius, and the area is equal to π times the square of the radius.
Overall, the equation of a circle is a powerful tool that provides information about the geometry and properties of circles. By understanding its properties and knowing how to manipulate and solve equations, we can analyze and solve a wide range of geometric problems.
Solving problems using the equation of a circle
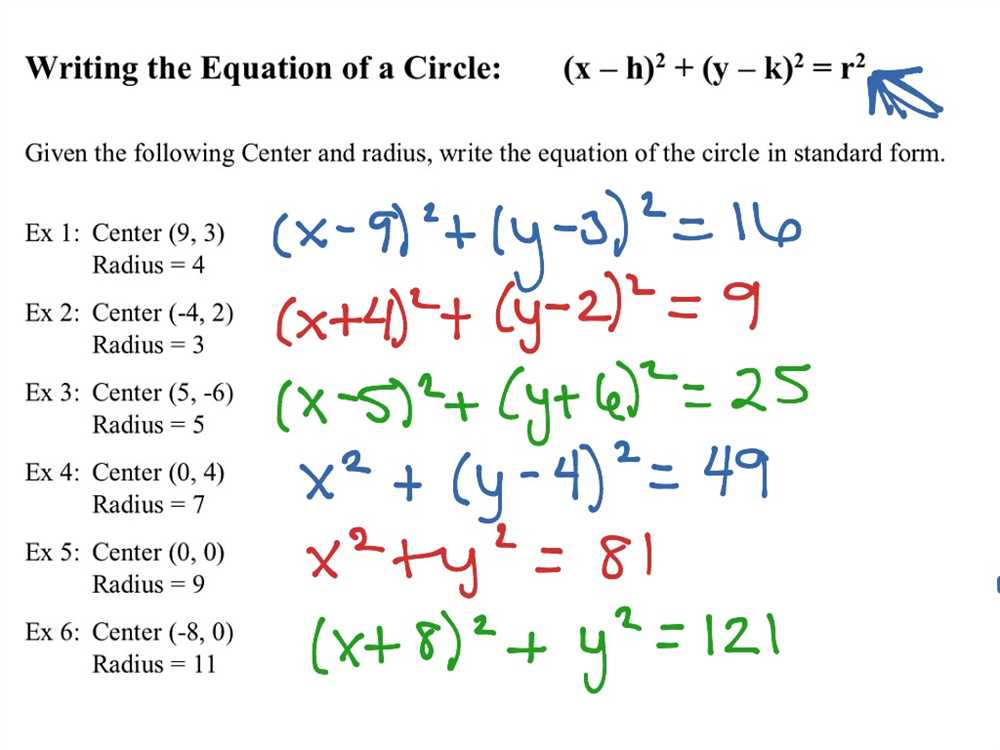
One common problem that can be solved using the equation of a circle is finding the center and radius of a given circle. To do this, you can compare the given equation with the standard form of the equation of a circle and identify the values of h, k, and r. These values represent the coordinates of the center and the radius of the circle.
Another problem that can be solved using the equation of a circle is determining if a point lies on the circle. By substituting the coordinates of the point into the equation of the circle and simplifying the expression, you can determine if the point satisfies the equation. If the result is true, then the point lies on the circle; otherwise, it does not.
The equation of a circle can also be used to solve problems involving the intersection of circles. By looking at the equations of two circles, you can compare their centers and radii to determine if they intersect, overlap, or do not intersect at all. This information can be useful in solving problems related to geometry, engineering, and physics.
Application of the equation of a circle in real-life scenarios
The equation of a circle is a mathematical tool that is used to describe the shape and properties of circles. It consists of the coordinates of the center of the circle and the radius. While it may seem like an abstract concept, the equation of a circle has numerous applications in real-life scenarios.
One application of the equation of a circle is in the field of engineering. Engineers use the equation to calculate the dimensions and parameters of circular structures such as bridges, pipelines, and tunnels. By knowing the center and radius of a circle, engineers can determine the correct dimensions for these structures, ensuring that they are safe and function properly.
Another application of the equation of a circle is in the field of navigation. GPS systems, for example, use circles to determine the location of a device. The GPS receiver calculates its position based on the distances to multiple satellites. These distances can be represented as circles with the satellite as the center and the distance as the radius. By intersecting these circles, the GPS system can pinpoint the location of the receiver.
In summary, the equation of a circle is a versatile mathematical tool that has various applications in real-life scenarios. From engineering to navigation, this equation is used to determine the dimensions, parameters, and locations of circular structures and objects. Its applications extend beyond the realm of mathematics and into practical everyday situations.
Tips and tricks for mastering the equation of a circle
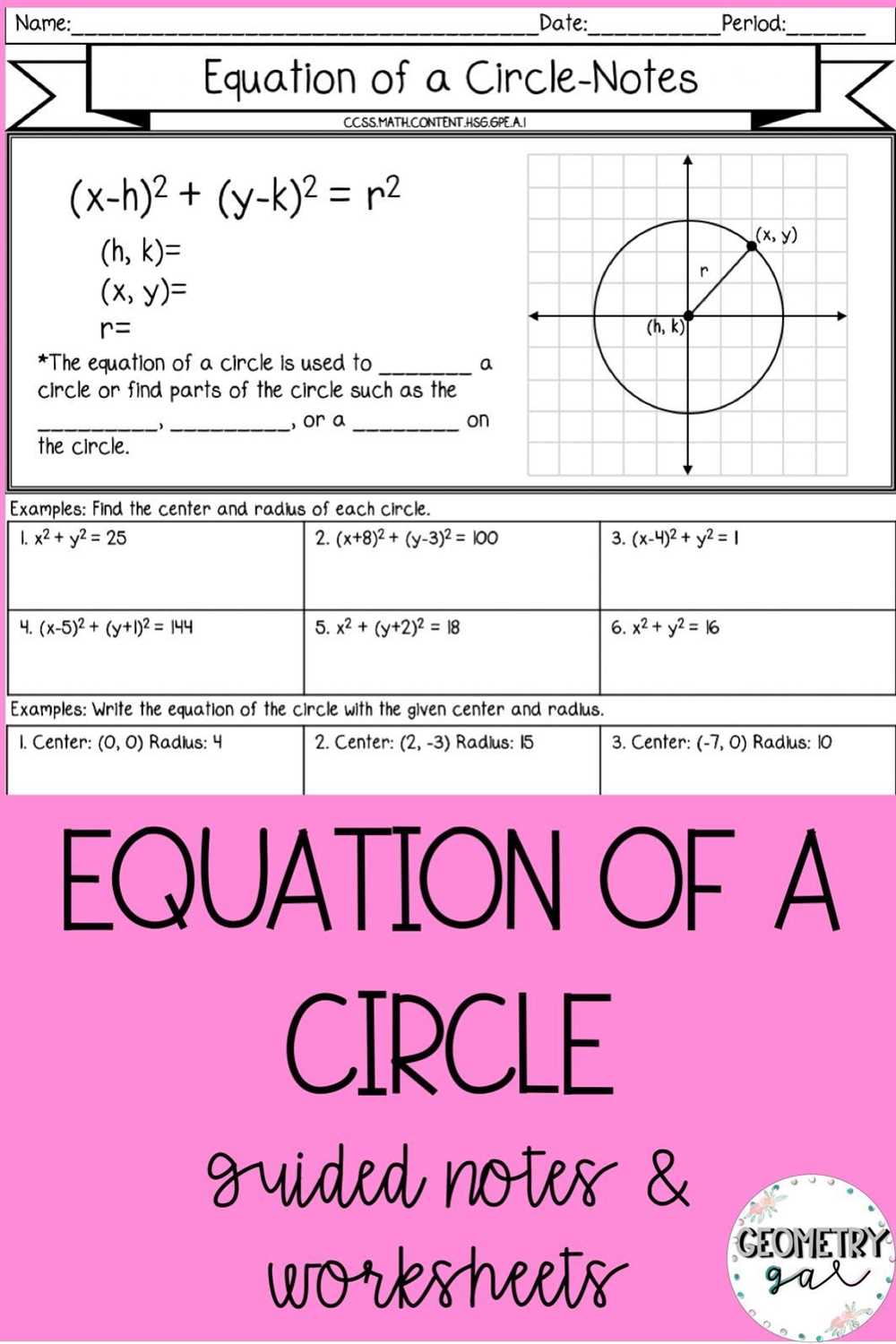
Understanding and mastering the equation of a circle is an essential skill in geometry and algebra. It involves being able to identify the center and radius of a circle based on given information, and vice versa. Here are some tips and tricks to help you become proficient in solving equations of circles.
1. Familiarize yourself with the standard form
The equation of a circle in standard form is (x – h)^2 + (y – k)^2 = r^2, where (h, k) represents the center of the circle and r is the radius. Make sure to remember this form as it will be the basis for all calculations.
2. Identify the center and radius
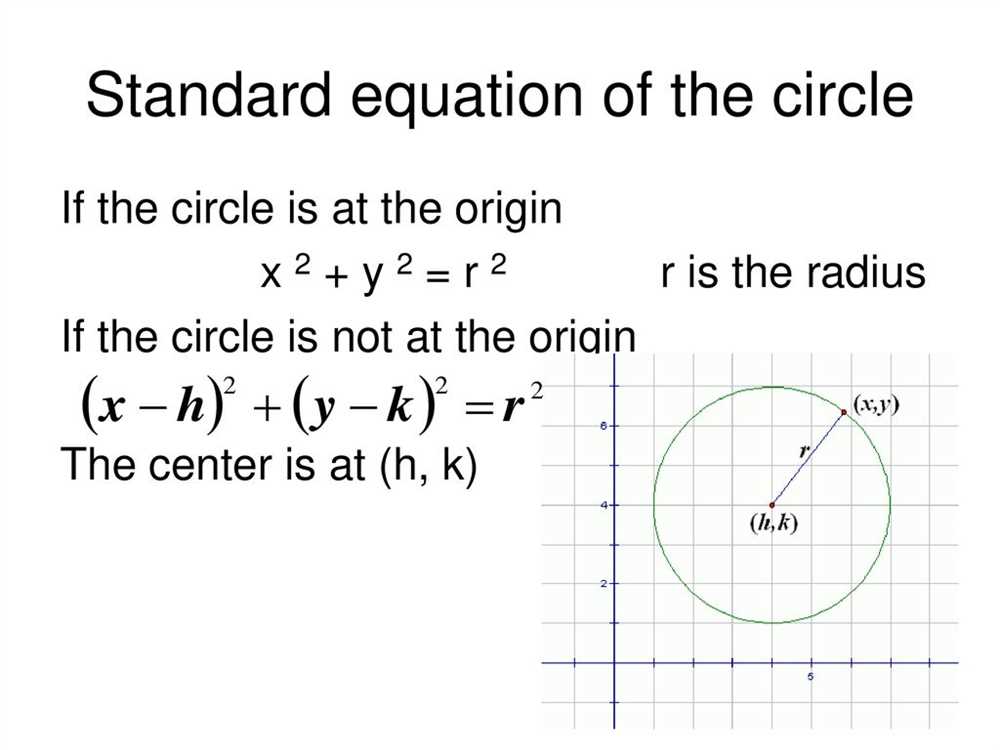
When given an equation of a circle, the first step is to identify the center and radius. Look for any terms in the equation that can be represented as (x – h)^2 or (y – k)^2. Once you have identified these values, it becomes easier to determine the center and radius.
3. Use the distance formula
If the equation of a circle is not in standard form, you can use the distance formula to determine the center and radius. By finding the distance between two points on the circle, you can then use this information to calculate the center and radius.
4. Practice with different examples
The more you practice solving equations of circles, the more comfortable and proficient you will become. Look for worksheets or online resources that provide various examples of equations of circles to practice on. This will help you become familiar with different scenarios and improve your problem-solving abilities.
5. Understand the geometric properties
Understanding the geometric properties of circles can also help you with solving equations. For example, knowing that the radius is always perpendicular to the tangent line at the point of contact can assist you in identifying the center and radius more easily.
By following these tips and tricks, you can build a strong foundation in solving equations of circles. Practice regularly and seek help if needed to ensure you fully grasp this essential concept in geometry and algebra.