Understanding exponent rules is crucial in mastering algebra and higher levels of math. Exponents represent a powerful mathematical tool that simplifies calculations and expresses repeated multiplication in a concise way. To ensure a solid understanding of exponent rules, practice is essential.
This Exponent Rules Review Worksheet provides a comprehensive set of problems that cover the fundamental concepts of exponents. It includes exercises on multiplication and division of exponents, raising a power to a power, simplifying expressions with negative exponents, and more. Each problem is designed to challenge students’ knowledge and reinforce their ability to apply the exponent rules correctly.
Accompanying the worksheet are the answer key and step-by-step explanations, allowing students to check their work and identify any areas that require further practice. The detailed answers serve as a valuable resource for students, helping them understand the conceptual reasoning behind each solution and reinforcing their understanding of exponent rules.
By utilizing this Exponent Rules Review Worksheet with Answers, students can solidify their understanding of exponent rules and enhance their problem-solving skills. Regular practice with these worksheets will enable students to confidently tackle more complex problems involving exponents and lay a strong foundation for future mathematical endeavors.
Exponent Rules Review Worksheet with Answers
Exponents are an important concept in mathematics that allows us to easily represent and manipulate large numbers. However, understanding the rules of exponents is essential for simplifying expressions and solving equations involving exponents. This exponent rules review worksheet with answers is designed to help students practice and reinforce their knowledge of these rules.
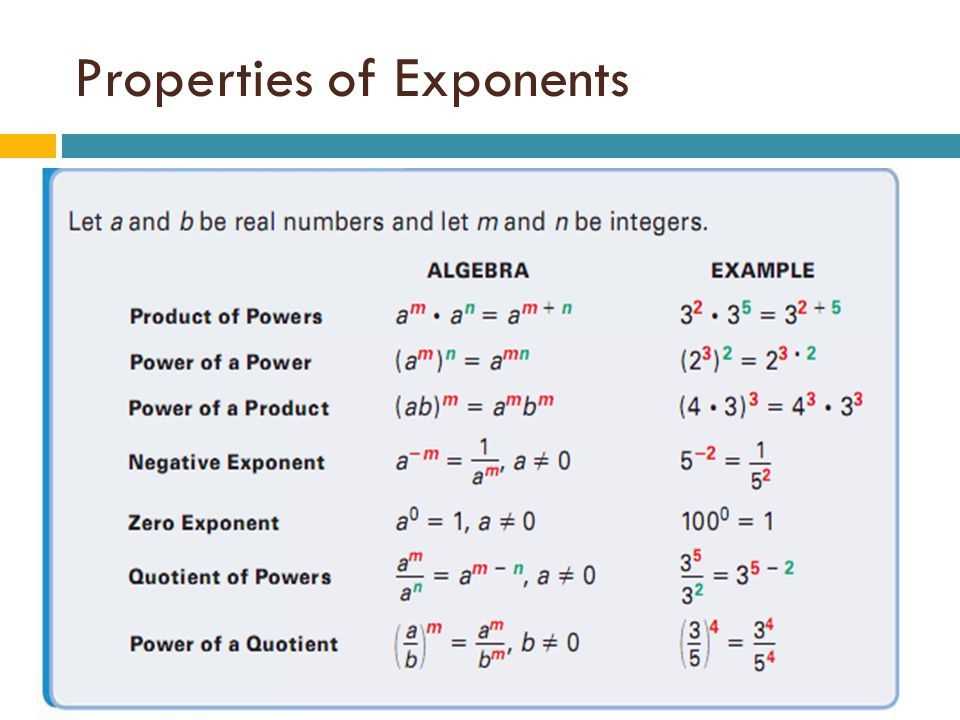
The worksheet starts with a brief recap of the basic exponent rules, such as the product rule, quotient rule, power rule, and zero exponent rule. This serves as a refresher and ensures that students have a solid foundation before moving on to more complex concepts.
- The product rule states that when multiplying two exponential expressions with the same base, you can add their exponents.
- The quotient rule states that when dividing two exponential expressions with the same base, you can subtract their exponents.
- The power rule states that when raising an exponential expression to a power, you can multiply the exponents.
- The zero exponent rule states that any number (except zero) raised to the power of zero is equal to one.
After reviewing these basic rules, the worksheet provides a series of practice problems for students to solve. Each problem requires them to apply one or more of the exponent rules to simplify the given expression. The answers for each problem are provided at the end of the worksheet, allowing students to check their work and identify any mistakes.
This exponent rules review worksheet with answers is a valuable resource for both classroom instruction and independent study. By practicing these rules and working through the problems, students can strengthen their understanding of exponents and improve their problem-solving skills in this area of mathematics.
Multiplying Exponents
Exponents are mathematical notation that represents repeated multiplication of a base number. When multiplying two exponents with the same base, the rule states that we need to add the exponents together. For example, if we have 2^3 * 2^2, we can add the exponents to get 2^5, which is equal to 32. This rule applies to any base number.
When multiplying exponents with different bases, we cannot simply add the exponents together. Instead, we need to multiply the base numbers and then add the exponents. For instance, if we have (3^2) * (4^3), we first multiply the base numbers 3 and 4 to get 12, and then add the exponents 2 and 3 to get 12^5, which is equal to 248,832. This rule ensures that we correctly account for the repeated multiplication of different base numbers.
Summary:
- When multiplying exponents with the same base, add the exponents together.
- When multiplying exponents with different bases, multiply the base numbers and add the exponents.
Understanding the rules for multiplying exponents is essential in simplifying and solving equations that involve exponential notation. Practice and review worksheets, like the “Exponent Rules Review Worksheet with Answers,” can help reinforce these rules and improve your understanding of exponent operations.
Dividing Exponents
When dividing expressions with exponents, we can apply the rule that states when we divide powers with the same base, we subtract the exponents. For example, if we have the expression (a^m / a^n), where (a) is a non-zero number and (m) and (n) are positive integers, the result is (a^{m-n}).
Let’s consider an example to understand this rule better. If we have (5^4 / 5^2), we can subtract the exponents to get (5^{4-2} = 5^2 = 25). In this case, we divide the base number, 5, and subtract the exponents, 4 and 2, to find the result.
It is important to note that this rule only applies when the bases are the same. If we have expressions such as (a^m / b^n), where the bases are different, we cannot directly apply the rule of subtracting exponents. In such cases, we generally simplify the expression as much as possible before dividing.
To summarize, when dividing expressions with the same base, we subtract the exponents. This allows us to simplify the expression and find the result. However, when the bases are different, we need to simplify the expression before dividing.
Power of a Power Rule
The power of a power rule states that when we raise an exponent to another exponent, we simply multiply the exponents. This rule is used to simplify expressions and solve problems involving exponents.
Example: Let’s consider the expression (x^3)^2. By applying the power of a power rule, we can simplify it as x^6. This means that we multiply the exponents 3 and 2 to get 6.
The power of a power rule can also be applied when we have negative exponents. In this case, we evaluate the expression as the reciprocal of the base raised to the product of the exponents.
Example: If we have (a^-2)^3, we can rewrite it as 1/(a^2)^3. Then, using the power of a power rule, we simplify it as 1/a^6.
It is important to note that the power of a power rule only applies when the exponents have the same base. If the bases are different, we cannot use this rule and would need to use other exponent rules to simplify the expression.
Power of a Product Rule
The power of a product rule is a fundamental rule in exponentiation that allows us to simplify expressions involving the multiplication of variables with exponents. The rule states that when you have a product of two or more variables raised to the same exponent, you can simplify it by raising each variable to the exponent individually and then multiplying the results.
Mathematically, the power of a product rule can be expressed as follows:
(ab)^n = a^n * b^n
For example, let’s say we have the expression (2x)^3. By applying the power of a product rule, we can simplify it as follows:
(2x)^3 = 2^3 * x^3 = 8x^3
This rule is particularly useful when dealing with expressions involving variables and exponents. It allows us to break down the expression into smaller components, simplify each component individually, and then combine them back together using multiplication.
It’s important to note that the power of a product rule only applies when the variables being multiplied have the same exponent. If the exponents are different, then the rule cannot be applied and the expression cannot be simplified using this method.
Power of a Quotient Rule

The power of a quotient rule is a fundamental rule in algebra that helps us simplify expressions involving fractions raised to a power. It states that when a fraction is raised to a power, we can distribute the power to both the numerator and the denominator separately.
Mathematically, the power of a quotient rule can be expressed as follows:
(a/b)^n = a^n / b^n
Where a and b are any real numbers, and n is a rational number. This rule allows us to simplify complex expressions by dividing the numerator and the denominator separately and then raising them to the desired power.
For example, let’s say we have the expression (4/2)^3. Applying the power of a quotient rule, we can simplify it as follows:
- 4^3 / 2^3
- 64 / 8
- 8
By using the power of a quotient rule, we were able to simplify the expression to a single number, which makes it easier to work with in further calculations.
This rule is essential in various areas of mathematics, including algebra, calculus, and physics. It allows us to manipulate fractions and exponents, making it easier to solve equations and perform calculations involving powers of fractions.
Zero Exponent Rule
The zero exponent rule is a fundamental concept in exponent rules that applies when a base is raised to the power of zero. According to this rule, any non-zero base raised to the power of zero is equal to one. This means that any number, except zero itself, raised to the power of zero equals one.
The zero exponent rule can be stated as follows: a0 = 1, where “a” is any non-zero number. This rule holds true for all real numbers, as well as complex numbers, when dealing with exponents.
The zero exponent rule can be understood through the concept of repeated multiplication. When a number is raised to the power of zero, we are essentially asking how many times that number should be multiplied by itself. However, since any number multiplied by one equals itself, raising a number to the power of zero results in one.
It is important to note that the zero exponent rule only applies to non-zero bases. When the base is zero, raising it to the power of zero is undefined. This is because any number, except zero, raised to the power of zero is one, but zero itself has no defined value when raised to the power of zero.
Understanding and applying the zero exponent rule is crucial in simplifying expressions and solving equations involving exponents. It allows for easier manipulation of expressions and helps in evaluating various mathematical problems involving exponents.
Negative Exponent Rule
When dealing with exponents, a negative exponent indicates that the base should be divided by itself raised to the positive exponent.
The negative exponent rule states that any number raised to a negative power is equal to one divided by that number raised to the positive power:
a-n = 1 / an
For example, if we have 2-3, it can be rewritten as 1 divided by 2 raised to the power of 3:
2-3 = 1 / 23 = 1 / 8 = 0.125
This rule applies to any base number, whether it is a whole number, a fraction, or a decimal.
It is important to remember that when using negative exponents, the result is always a reciprocal of the positive exponent, meaning it will have the opposite sign.
This rule is useful when simplifying algebraic expressions and solving equations involving negative exponents.