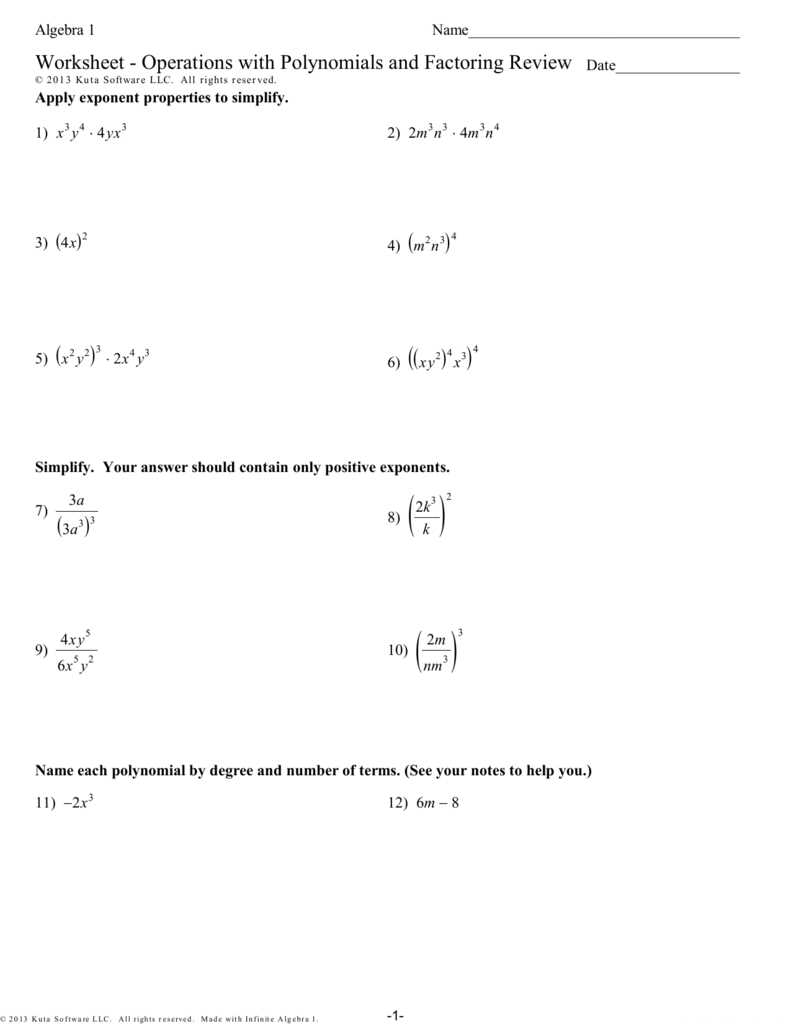
Factoring polynomials is an essential skill in algebra 2 that allows us to simplify complex expressions and solve equations. To help students practice and master this skill, teachers often provide factoring polynomials worksheets. These worksheets are designed to offer a range of problems with varying levels of difficulty, allowing students to gradually build their understanding and proficiency in factoring.
One popular format for these worksheets is the algebra 2 factoring polynomials worksheet with answers in PDF form. These PDF worksheets provide a convenient and printable resource for students to work on factoring polynomials both in and outside of the classroom. They include a variety of polynomial expressions, ranging from simple quadratic equations to more complex higher-degree polynomials.
The answers provided in these worksheets serve as a valuable tool for self-assessment and immediate feedback. Students can compare their answers to the given solutions, identify any mistakes or misconceptions, and work towards correcting them. This helps students to develop a deeper understanding of the concepts involved in factoring polynomials and build their problem-solving skills.
Whether you are a student looking to practice and improve your factoring skills or a teacher searching for additional resources to enhance your algebra 2 curriculum, the factoring polynomials worksheet with answers in PDF format is a valuable tool. It offers a structured and comprehensive approach to mastering this fundamental algebraic skill and provides the necessary guidance for students to achieve success.
Factoring Polynomials Worksheet with Answers Algebra 2 PDF
In algebra, factoring polynomials is a key skill that students need to master. Factoring polynomials worksheet with answers, especially in algebra 2, helps students practice this skill and solidify their understanding of the concept. These worksheets are often available in PDF format, making them easy to download and print.
These worksheets typically include a variety of polynomial expressions that students need to factor. The answers are provided, allowing students to check their work and identify any mistakes they may have made. This immediate feedback is invaluable, as it helps students learn from their errors and improve their problem-solving skills.
Factoring polynomials requires students to break down a polynomial expression into its factors. This process involves identifying any common factors, then using various factoring techniques such as the difference of squares, sum and difference of cubes, or grouping to further factor the expression. The worksheets often include a mix of different factoring techniques to challenge students and ensure a comprehensive understanding of the topic.
By practicing factoring polynomials through worksheets, students can become more confident in their ability to factor expressions and solve more complex problems. These worksheets help students reinforce their understanding of the factoring process, develop their critical thinking skills, and prepare for more advanced algebraic concepts.
Example of a Factoring Polynomials Worksheet:
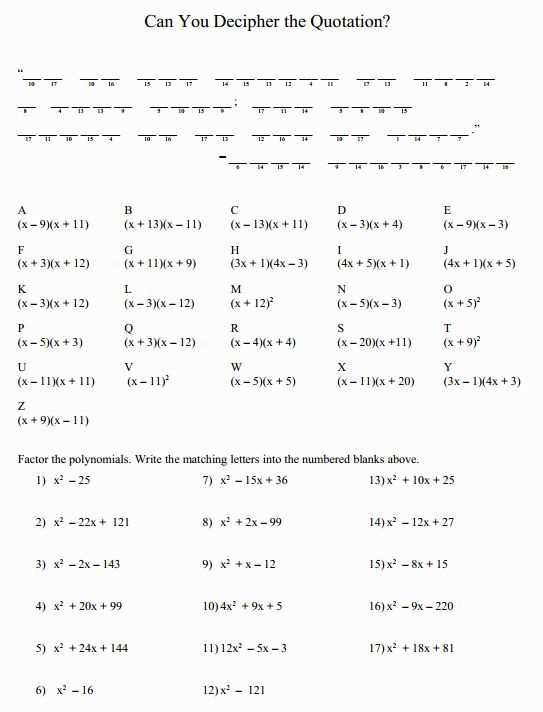
| Polynomial Expression | Factored Form |
|---|---|
| x^2 – 9 | (x – 3)(x + 3) |
| 4x^2 – 25 | (2x – 5)(2x + 5) |
| x^3 + 8 | (x + 2)(x^2 – 2x + 4) |
| 3x^2 + 6x + 3 | 3(x + 1)^2 |
By working through a factoring polynomials worksheet with answers, students can gain confidence and mastery in factoring expressions, setting a solid foundation for further studies in algebra.
Importance of Factoring Polynomials
Factoring polynomials is a fundamental concept in algebra that has numerous real-world applications. It involves breaking down a polynomial equation into its simplest form by identifying its factors. This process is crucial for solving equations, simplifying expressions, and understanding the behavior of polynomial functions.
1. Solving Equations: Factoring polynomials allows us to solve quadratic, cubic, and higher-degree equations. By factoring the polynomial equation, we can set each factor equal to zero and solve for the variables. The solutions obtained through factoring give us the roots of the equation, which are crucial for various mathematical and scientific applications.
2. Simplifying Expressions: Factoring polynomials helps us simplify complex algebraic expressions. By factoring out common factors, we can reduce the complexity of the expression and make it easier to work with. This simplification is especially useful when dealing with fractions, exponents, and irrational numbers.
3. Understanding Polynomial Functions: Factoring polynomials provides insight into the behavior and characteristics of polynomial functions. By factoring, we can determine the x-intercepts (zeros or roots) of the function, which gives us information about the points where the function intersects the x-axis. This knowledge is essential for graphing polynomial functions and analyzing their properties.
4. Applications in Science and Engineering: Factoring polynomials plays a significant role in various scientific and engineering fields. It is used in physics to solve equations of motion, in chemistry to balance chemical equations, and in engineering to analyze electrical circuits. Factoring polynomials helps in modeling and understanding real-world phenomena, making it an essential skill for students pursuing STEM disciplines.
In conclusion, factoring polynomials is a fundamental skill in algebra with broad practical applications. It enables us to solve equations, simplify expressions, understand polynomial functions, and apply mathematical concepts to real-world scenarios. Mastering the art of factoring polynomials empowers students to tackle more complex mathematical problems and opens doors to a wide range of academic and professional opportunities.
Factoring Common Linear Terms
When it comes to factoring polynomials, one common technique is factoring out common linear terms. This involves identifying any common factors within the polynomial expression and simplifying it by factoring them out.
To factor out common linear terms, you need to identify any factors that are present in all terms of the polynomial. For example, consider the polynomial expression 3x + 6. Both terms have a common factor of 3, so you can factor it out to simplify the expression:
3x + 6 = 3(x + 2)
In this case, we factored out the common factor 3 and wrote it outside the parentheses. The remaining expression inside the parentheses, x + 2, is the simplified form of the original polynomial.
This technique can be applied to more complex polynomial expressions as well. For example, consider the polynomial expression 4x^2 + 8x. Both terms have a common factor of 4x, so we can factor it out:
4x^2 + 8x = 4x(x + 2)
In this case, we factored out the common factor 4x and wrote it outside the parentheses. The remaining expression inside the parentheses, x + 2, is the simplified form of the original polynomial.
Factoring out common linear terms can help simplify polynomial expressions and make them easier to work with. It is an important technique in algebra and is often used in various mathematical applications.
Factoring by Grouping
Factoring by grouping is a technique used to factor polynomials by grouping terms with common factors. It is particularly useful when the polynomial has four terms and the common factor is not apparent at first glance. By grouping the terms and factoring out the common factor from each group, we can simplify the polynomial and make the factoring process easier.
To factor a polynomial by grouping, we follow these steps:
- Group the terms in pairs, making sure each pair has a common factor.
- Factor out the common factor from each pair.
- Look for a common factor between the two groups.
- Factor out the common factor from the two groups.
- Combine the factored terms to obtain the final factored form of the polynomial.
Let’s consider an example to illustrate the process. Suppose we have the polynomial 2x + 4 + 3x + 6. We can group the terms as follows: (2x + 4) + (3x + 6). From each group, we can factor out the common factor: 2(x + 2) + 3(x + 2). Now we can see that both groups have a common factor of x + 2. Factoring out this common factor, we obtain the final factored form of the polynomial: (x + 2)(2 + 3).
Factoring by grouping can be a helpful technique for factoring polynomials with four or more terms. It allows us to simplify the polynomial and identify common factors that might not be immediately apparent. By following the steps outlined above, we can efficiently factor polynomials and solve algebraic equations.
Factoring Quadratic Trinomials
A quadratic trinomial is a polynomial expression that contains three terms, with one of the terms being a quadratic term (raised to the power of 2). Factoring quadratic trinomials is the process of breaking down the expression into its factors. It is an important skill in algebra, as it allows us to solve equations and simplify expressions.
When factoring quadratic trinomials, we look for two binomial factors that, when multiplied together, result in the original expression. The goal is to find the correct combination of factors that will lead to the original trinomial when multiplied.
One method for factoring quadratic trinomials is through the process of “guess and check.” We try different combinations of factors and see if they lead to the original trinomial. This can be a time-consuming method, especially for trinomials with large coefficients.
Another more systematic method is to use the quadratic formula or completing the square to find the roots of the trinomial. Once the roots are found, we can express the trinomial as a product of the binomial factors using the fact that (x – root1)(x – root2) will expand to give the original trinomial.
Factoring quadratic trinomials is a fundamental skill in algebra and is often used in various math applications, such as solving word problems and simplifying equations. It is important to practice this skill through worksheets and exercises to become proficient in factoring quadratic trinomials.
Factoring Difference of Squares
Factoring is an important concept in algebra that involves breaking down a polynomial into its constituent parts. One common type of factoring is called the “difference of squares.” This method is used when you have a polynomial that can be expressed as the difference between two perfect squares.
The difference of squares can be factored using a simple formula: a^2 – b^2 = (a + b)(a – b). This means that if you have a polynomial in the form of a difference between two perfect squares, you can factor it by applying the formula to the two terms.
For example, let’s say we have the polynomial x^2 – 9. We can recognize this as a difference of squares because it is the difference between x^2 and 3^2. Applying the formula, we get: x^2 – 9 = (x + 3)(x – 3).
This concept is useful in algebra because it allows us to simplify complex polynomials and solve equations more easily. By factoring a polynomial into its constituent parts, we can often find solutions or simplify expressions that would be otherwise difficult to work with.
When factoring a polynomial, it is important to look for patterns and apply appropriate formulas, such as the difference of squares. With practice, factoring becomes easier and can be a valuable tool for solving algebraic problems.
Factoring Perfect Square Trinomials
Factoring perfect square trinomials is an essential skill in algebra, as it allows us to simplify and solve polynomial equations more efficiently. A perfect square trinomial is a trinomial that can be factored into two identical binomials. In other words, both binomials will have the same factors.
To factor a perfect square trinomial, we can use the formula (a + b)^2 = a^2 + 2ab + b^2. This formula represents the multiplication of a binomial by itself. By identifying the values of a and b in the trinomial, we can easily factor it.
For example, let’s consider the trinomial x^2 + 6x + 9. By comparing it to the formula (a + b)^2, we can see that a is x and b is 3. Therefore, we can factor it as (x + 3)^2. Notice that both binomials x + 3 are the same, indicating that it is a perfect square trinomial.
Factoring perfect square trinomials is useful when solving quadratic equations and simplifying expressions. It allows us to easily identify common factors and simplify complicated equations. By understanding and practicing factoring perfect square trinomials, students can strengthen their algebra skills and solve math problems more efficiently.
Practice Problems: Factoring Polynomials Worksheet with Answers
Factoring polynomials is an important skill in algebra and can be used to simplify expressions and solve equations. To practice this skill, we have prepared a worksheet with a variety of factoring problems along with their answers. This worksheet is designed to help you strengthen your understanding of factoring and improve your problem-solving abilities.
The worksheet includes problems that cover different types of factoring, such as factoring trinomials, binomials, and special cases like the difference of squares and perfect square trinomials. Each problem is accompanied by a step-by-step solution, so you can easily check your work and learn from your mistakes.
To get the most out of this worksheet, it is recommended that you first review the concept of factoring and familiarize yourself with the different methods. Then, work through each problem systematically, applying the appropriate factoring technique. If you get stuck on a problem, refer to the provided solution and try to understand the reasoning behind each step.
By practicing these factoring problems, you will become more confident in your ability to factor polynomials and handle more complex equations in the future. Remember, the key to mastering factoring is practice, so don’t be discouraged if you encounter difficulties along the way. Keep working through the problems, and with time and effort, you will become a skilled factoring expert.
Ready to get started? Download the Factoring Polynomials Worksheet with Answers and begin your factoring journey today!